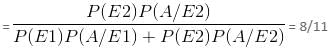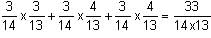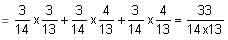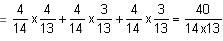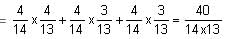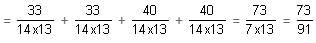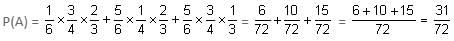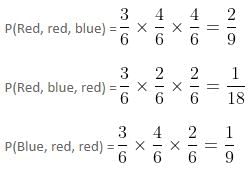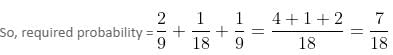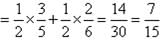This EduRev document offers 10 Multiple Choice Questions (MCQs) from the topic Probability (Level - 3). These questions are of Level - 3 difficulty and will assist you in the preparation of CAT & other MBA exams. You can practice/attempt these CAT Multiple Choice Questions (MCQs) and check the explanations for a better understanding of the topic.
Question for Practice Questions Level 3: Probability - 2
Try yourself:Suppose a girl throws a die. If she gets a 5 or 6, she tosses a coin three times and notes the number of heads. If she gets a 1, 2, 3 or 4, she tosses a coin once and notes whether a head or tail is obtained. If she obtained exactly one head, what is the probability of getting a 1, 2, 3 or 4 on the die?
Explanation
E1 = Getting 5 or 6 in a single throw of a die.
E2 = Getting 1, 2, 3 or 4 in a single throw of a die.
A = Getting exactly one head.
P(E1) = 2/6 = 1/3.
P(E2) = 4/6 = 2/3.
P(A/E1) = Probability of getting exactly one head when a coin is tossed three times = 3C1 × (1/2)1 × (1/2)2 = 3/8.
P(A/E2) = Probability of getting exactly one head when a coin is tossed once only = 1/2.
Now, Required probability = P(E2/A)
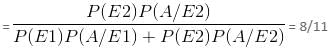
Report a problem
Question for Practice Questions Level 3: Probability - 2
Try yourself:Two balls are drawn at random from a bag containing 3 white, 3 red, 4 green and 4 black balls one by one without replacement. Find the probability that both the balls are of different colours.
Explanation
Report a problem
Question for Practice Questions Level 3: Probability - 2
Try yourself:The probabilities that three men hit a target are 1/6, 1/4 and 1/3. Each man shoots once at the target. What is the probability that exactly one of them hits the target?
Explanation
Probabilities that 3 men hit a target are 1/6 , 1/4 and 1/3 and
Probabilities that the same 3 men do not hit the target are 5/6, 3/4 and 2/3.
Let,
Event (A) = Exactly one of them hits the target 1st person or 2nd person or 3rd person
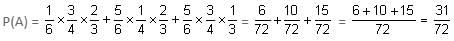
Report a problem
Question for Practice Questions Level 3: Probability - 2
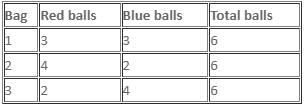
Try yourself:Three bags contain 18 balls of red and blue colours. The first bag contains 3 red and 3 blue balls. The second bag contains 4 red and 2 blue balls and the third bag contains 2 red and 4 blue balls. One ball is taken out at random from each bag. The probability of getting 2 red balls and 1 blue ball is
Explanation
Report a problem
Question for Practice Questions Level 3: Probability - 2
Try yourself:In a tailor shop, tailor A makes 500 shirts, tailor B makes 1200 shirts and tailor C makes 1600 shirts. Out of these, 200, 300 and 300 shirts are defective, respectively. One shirt is drawn at random and if it is found to be defective, then the probability that is made by tailor C is
Explanation
To determine the probability that a randomly selected defective shirt was made by tailor C, we first need to find the total number of shirts and defective shirts made by each tailor.
- Tailor A: 500 shirts, 200 defective
- Tailor B: 1200 shirts, 300 defective
- Tailor C: 1600 shirts, 300 defective
Next, we calculate the total number of shirts and the total number of defective shirts:
- Total shirts: 500 + 1200 + 1600 = 3300 shirts
- Total defective shirts: 200 + 300 + 300 = 800 defective shirts
Now, we focus on the number of defective shirts made by tailor C:
- Defective shirts by C: 300
The probability that a defective shirt is from tailor C is calculated using the formula:
Probability = (Defective shirts by C) / (Total defective shirts)
Substituting the values, we get:
Probability = 300 / 800 = 3/8
Thus, the probability that a defective shirt was made by tailor C is 3/8.
Report a problem
Question for Practice Questions Level 3: Probability - 2
Try yourself:Directions: Study the following information and answer the given question.
Three friends A, B and C do air rifle shooting as a hobby and compete against each other in friendly competitions. On a Sunday morning, they go to an empty tract of land along with their air rifles to shoot at clay practice targets from the distances of 100 m and 200 m. A hits his target 95% of the times from a distance of 100 m and 70% times from a distance of 200 m. The same figures for B are 85% and 80%, respectively. The same figures for C are 90% and 75%, respectively. They put in numbered chits (1, 2 and 3) to be drawn to decide the order of shooting and then each of them shoots at the targets turn-wise according to the chit number, first from 100 m and then from 200 m. They decide on a point system with 1 point for hitting the target from a distance of 100 m and 2 points for hitting it from a distance of 200 m with the winner getting a round of drinks paid by the other two.
Q. What is the probability that the order of shooting is ABC?
Explanation
Total number of possible ways = 3!
Number of favourable cases = 1
Thus, required probability = 1/3! = 1/6
Report a problem
Question for Practice Questions Level 3: Probability - 2
Try yourself:Directions: Study the following information and answer the given question.
Three friends A, B and C do air rifle shooting as a hobby and compete against each other in friendly competitions. On a Sunday morning, they go to an empty tract of land along with their air rifles to shoot at clay practice targets from the distances of 100 m and 200 m. A hits his target 95% of the times from a distance of 100 m and 70% times from a distance of 200 m. The same figures for B are 85% and 80%, respectively. The same figures for C are 90% and 75%, respectively. They put in numbered chits (1, 2 and 3) to be drawn to decide the order of shooting and then each of them shoots at the targets turn-wise according to the chit number, first from 100 m and then from 200 m. They decide on a point system with 1 point for hitting the target from a distance of 100 m and 2 points for hitting it from a distance of 200 m with the winner getting a round of drinks paid by the other two.
Q. What is the probability that all three of them will end up shooting 1 target only, with all three of them shooting the target of the same points weightage?
Explanation
One possibility is that all three of them hit the 1st target and miss out on the 2nd target.
The probability for this is 0.95 × 0.30 × 0.85 × 0.20 × 0.90 × 0.25 = 0.01090125
The 2nd possibility is that all of them hit only the 2nd target and miss out on the 1st.
The probability for the same is 0.05 × 0.7 × 0.15 × 0.80 × 0.10 × 0.75 = 0.000315
Thus, required probability = 0.01090125 + 0.000315 = 0.01121625 ~ 0.011
Report a problem
Question for Practice Questions Level 3: Probability - 2
Try yourself:A bag X contains 3 white and 2 black balls. Another bag Y contains 2 white and 4 black balls. A bag and a ball out of it are picked at random. Find the probability that the ball is of white colour.
Explanation
A white ball can be drawn in two mutually exclusive ways.
(i) Selecting bag X and then drawing a white ball from it.
(ii) Selecting bag Y and then drawing a white ball from it.
Let E1: event of selecting bag X
E2: event of selecting bag Y
A: event of drawing white ball
∴ P(E1) = P(E2) = 1/2
Now, P(A/E1) = Probability of drawing a white ball when bag X is chosen = 3/5
P(A/E2) = Probability of drawing a white ball when bag Y is chosen = 2/6
P(white ball) = P(A) = P(E1) P(A/E1) + P(E2) P(A/E2) (Law of total probability)
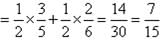
Report a problem
Question for Practice Questions Level 3: Probability - 2
Try yourself:Directions: Read the given information and answer the following:
Two friends A and B play a game of chance. A person tosses a coin and if the coin shows a heads, he rolls a die. He does this twice and the score is the total of the numbers that the die throws up in both the rolls, if at all. If a person ends up with a tails on tossing the coin, he does not roll the die and his score is entered as 0. The winner of the game is the person whose score is the maximum.
Q. What is the probability that A wins the game with a score of 2 and B ends up with a score of 0?
Explanation
There are 3 favourable cases for the same which shall be discussed one by one.
The 1st case is in which A scores a 1 in both the rolls and B does not get to roll the die.
The probability for the same is (1/2)(1/6)(1/2)(1/6)(1/2)(1/2) = 1/576
The 2nd case is in which A gets a score of 2 in his first roll, gets no score in the 2nd roll and B gets no score in either of his rolls.
The probability for the same is (1/2)(1/6)(1/2)(1/2)(1/2) = 1/96
The 3rd case is in which A gets a score of 0 in the 1st roll, gets a score of 2 in the 2nd roll and B gets no score in either of his rolls.
The probability for the same is (1/2)(1/2)(1/6)(1/2)(1/2) = 1/96
Thus, the required probability is 1/96 + 1/96 + 1/576 = 13/576
Report a problem
Question for Practice Questions Level 3: Probability - 2
Try yourself:Directions: Read the given information and answer the following:
Two friends A and B play a game of chance. A person tosses a coin and if the coin shows a heads, he rolls a die. He does this twice and the score is the total of the numbers that the die throws up in both the rolls, if at all. If a person ends up with a tails on tossing the coin, he does not roll the die and his score is entered as 0. The winner of the game is the person whose score is the maximum.
Q. What is the probability that A ends up with an odd score without scoring a 0 in any of his rolls?
Explanation
There shall be two cases for the same.
Case I: A scores an even number in his first roll and an odd number in his second roll, the probability of which is (1/2)(3/6)(1/2)(3/6) = 1/16
Case II: A scores an odd number in his first roll and an even number in his second roll, the probability of which is (1/2)(3/6)(1/2)(3/6) = 1/16
Thus, the required probability is 1/16 + 1/16 = 1/8
Report a problem