Electromagnetic Induction | Electricity & Magnetism - Physics PDF Download
Faraday’s Law
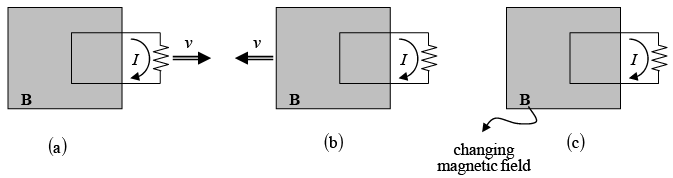
Experiment 1: He pulled a loop of wire to the right through a magnetic field. A current flowed in the loop (Figure a).
Experiment 2: He moved the magnet to the left, holding the loop still. Again, a current flowed in the loop (Figure b).
Experiment 3: With both the loop and the magnet at rest, he changed the strength of the field (he used an electromagnet, and varied the current in the coil). Once again current flowed in the loop (Figure c).
Thus, universal flux rule is that, whenever (and for whatever reason) the magnetic flux through a loop changes, an e.m.f. (ε) will appear in the loop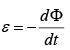
In experiment 2, A changing magnetic field induces an electric field. It is this “induced” electric field that accounts for the e.m.f.
Also the induced e.m.f 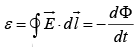 (where magnetic flux
(where magnetic flux 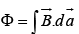 )
)
Then 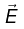 is related to the change in
is related to the change in 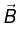 by the equation
by the equation
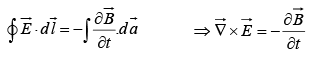
Lenz’s Law
In Faraday’s law negative sign represents the Lenz’s law. (The induced current will flow in such a direction that the flux it produces tends to cancel the change). For example if the magnetic flux is increasing then induced e.m.f will try to reduce and vice versa.
Example 1: A long solenoid, of radius a, is driven by alternating current, so that the field inside is sinusoidal 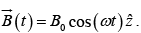 A circular loop of wire, of radius a/2 and resistance R, is placed inside the solenoid, and coaxial with it. Find the current induced in the loop, as a function of time.
A circular loop of wire, of radius a/2 and resistance R, is placed inside the solenoid, and coaxial with it. Find the current induced in the loop, as a function of time.
Magnetic flux through the loop
Induced emf
Induced current
Example 2: A square loop (side a) is mounted on a vertical shaft and rotated at angular velocity ω . A uniform magnetic field 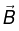 points to the right. Find the induced emf ε( t) for this alternating current generator.
points to the right. Find the induced emf ε( t) for this alternating current generator.
Magnetic flux
Induced emf
Example 3: A metal bar of mass m slides frictionlessly on two parallel conducting rails a distance l apart. A resistor R is connected across the rails and a uniform magnetic field 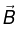 , pointing into the page, fills the entire region.
, pointing into the page, fills the entire region.
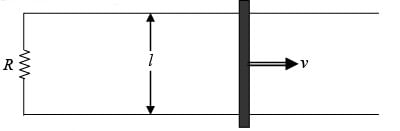
(a) If the bar moves to the right at speed v, what is the current in the resistor? In what direction does it flow?
(b) What is the magnetic force on the bar?
(a)
(b)
Example 4: A square loop of wire (side a) lies on a table, a distance r from a very long straight wire, which carries a current I.
(a) Find the flux of 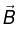 through the loop.
through the loop.
(b) If some one now pulls the loop directly away from the wire, at speed v, what emf is generated? In what direction does the current flow?
(c) What if the loop is pulled to the right at speed v, instead of moving away?
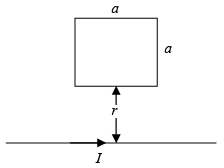
(a)
(b)
(c) Flux is constant so ε = 0
Example 5: A long solenoid, of radius a and n turns per unit length carries a time-dependent current I(t) in the 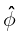 direction. Find the electric field (magnitude and direction) at a distance r from the axis (both inside and outside the solenoid).
direction. Find the electric field (magnitude and direction) at a distance r from the axis (both inside and outside the solenoid).
Field due to solenoid
inside and zero outside.
Inside solenoid ( r <a ):
Outside solenoid ( r >a ):
Example 6: A uniform magnetic field 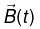 pointing straight up, fills the shaded circular region of figure shown below. if
pointing straight up, fills the shaded circular region of figure shown below. if 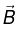 is changing with time, what is the induced electric field?
is changing with time, what is the induced electric field?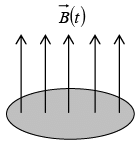
points in the circumferential direction, just like the magnetic field inside a long straight wire carrying a uniform current density.
Draw an Amperian loop of radius r and apply Faraday’s Law:
Thus
Ifis increasing,
runs clockwise, as viewed from above.
Inductance
If a steady current I1 flows in a loop 1, it produces magnetic field 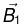 . Some of the field lines pass through loop 2, let Φ2 be the flux of
. Some of the field lines pass through loop 2, let Φ2 be the flux of 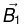 through 2.
through 2.
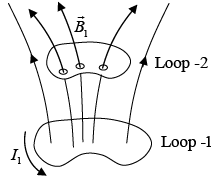
From Biot-Savart law, 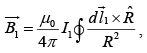
Therefore flux through loop 2 is 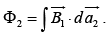
Thus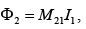 where M21 is the constant of proportionality; it is known as the mutual inductance of the two loops. Now
where M21 is the constant of proportionality; it is known as the mutual inductance of the two loops. Now
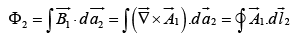
Since
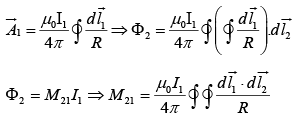
This is the Neumann formula; it involves double line integral-one integration around loop1, the other around loop2.
Thus
(a) M21 is a purely geometrical quantity depends on sizes, shapes and relative position of two loops.
(b) M21 = M12=M
If flux through loop 2, varies then induce emf in loop 2 is
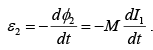
Changing current not only induces an emf in any nearby loops, it also induces an emf in the source loop itself. Again field (and therefore flux) is proportional to the current.
Φ= LI where L is self inductance of the loop
If the current changes, the emf induced in the same loop is
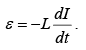
Inductance is measured in henries (H); a henry is a volt-second per ampere. Inductance (like capacitance) is an intrinsically positive quantity. Lenz's law, which is enforced by minus sign, which means the emf is in such a direction to oppose and change in current. For this reason, it is called a back emf. Whenever we try to alter the current, we must fight against this back emf.
Energy Stored in the field
It takes a certain amount of energy to start a current flowing in a circuit. The work done on a unit charge, against the back emf, in one trip around the circuit is −ε (the mines sign is due to the fact that work is being done by us against the emf, not the work done by the emf). The amount of charge per unit time passing down the wire is I. So the total work done per unit time is,
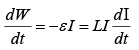
If we start with zero current and build it up to a final value I,
The work done (Integrating the last equation over time) is 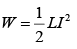
Since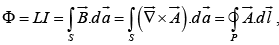 where P is the perimeter of the loop and S is any surface bounded by P.
where P is the perimeter of the loop and S is any surface bounded by P.
Therefore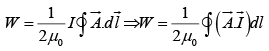
The generalization to volume current is: 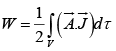
We can simplify above equation as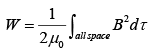
|
82 videos|32 docs|22 tests
|
FAQs on Electromagnetic Induction - Electricity & Magnetism - Physics
| 1. What is electromagnetic induction? |  |
| 2. How does electromagnetic induction work? |  |
| 3. What are the applications of electromagnetic induction? |  |
| 4. What is Faraday's law of electromagnetic induction? |  |
| 5. Can you explain Lenz's law in the context of electromagnetic induction? |  |











 inside and zero outside.
inside and zero outside.


 points in the circumferential direction, just like the magnetic field inside a long straight wire carrying a uniform current density.
points in the circumferential direction, just like the magnetic field inside a long straight wire carrying a uniform current density.

 is increasing,
is increasing,  runs clockwise, as viewed from above.
runs clockwise, as viewed from above.


















