Energy Consideration: A Quantitative Study | Physics for SSS 3 PDF Download
Energy Consideration
We all know that Lenz’s law is consistent with the law of conservation of energy. Lenz’s Law states that, when you induce a current in a wire via a changing magnetic field, the current flows through the wire in such a direction so that its magnetic field opposes the change that produced the current.
Now let us understand energy consideration in a better way
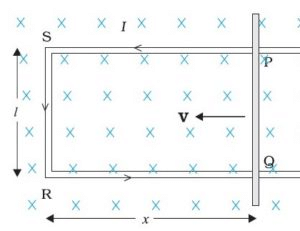
Suppose there is a rectangular conductor. now from the above figure, we can say that the sides of the sides of the rectangular conductor are PQ, RS, QR, and SP. Now in this rectangular conductor, the three sides are fixed, while one of it’s side that is the side PQ is set free.
Let r be that movable resistance of the conductor. So the resistance of the other remaining sides of the rectangular conductor that is the resistance of side RS, SP and QR is very small as compared to this movable resistance. In a constant magnetic field, if we change the flux, an emf is induced. i.e E = d∅/dt
If there is induced emf E and a movable resistance r in the conductor then, we can say that I = Blv/R. As the magnetic field is present, there will also be a force F acting, as F = ILB. This force is directed outwards in the direction opposite to the velocity of the rod, given by F = B2l2v/R
Power = force × velocity = B2l2v/R
Now here the work done is mechanical and this mechanical energy is dissipated as Joule heat. This is given as PJ = I2R = B2l2v/R. Further, the mechanical energy converts into electrical energy and finally into thermal energy. From the Faraday’s law, we have learned that, |E| = Δ∅B/Δt
So we get, |E| = 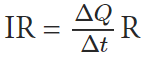
Hence we get, 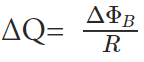
Solved Examples
Q.1. A circular coil of radius 8.0 cm and 20 turns is rotated about its vertical diameter with an angular speed of 50 rads-1 in a uniform horizontal magnetic field of magnitude 3.0 × 10-2 T. Obtain the maximum and average emf induced in the coil. If the coil forms a closed loop of resistance 10, calculate the maximum value of current in the coil. Calculate the average power loss due to Joule heating. Where does this power come from?
Solution: Maximum induced emf e = NwAB,
N = number of turns
w = angular speed
A = Area of the coil
B = Magnetic filed
e = 0.603 V
Over a full cycle, the average emf induced in the coil is zero. Maximum current, I = e/R = 0.0603
Average power loss due to Joule heating is P = eI/2 = 0.018W
Answer: The average power loss due to Joule heating is 0.018W
Q.2. A rectangular wire loop of sides 8 cm and 2 cm with a small cut is moving out of a region of a uniform magnetic field of magnitude 0.3 T directed normal to the loop. What is the emf developed across the cut if the velocity of the loop is in a direction normal to the (a) longer side, (b) shorter side of the loop? For how long does the induced voltage last in each case?
Solution: A = 8 × 2 = 16cm²
emf, e = d(A × B)/dt = 0.32 × 10-4 V
Induced curent, i = e/R = 2 × 10-5 A
Power, P = i²R = 6.4×10-10 W
|
57 videos|199 docs|65 tests
|

|
Explore Courses for SSS 3 exam
|

|

















