NCERT Solutions for Class 9 Maths - Heron’s Formula (Exercise 10.1)
Q1. A traffic signal board, indicating ‘SCHOOL AHEAD’, is an equilateral triangle with side ‘a’. Find the area of the signal board, using Heron’s formula. If its perimeter is 180 cm, what will be the area of the signal board?
Ans: For an equilateral triangle with side ‘a’, area = (√3/4) a2

∵ Each side of the triangle = a cm
Perimeter of the signal board will be
∴ a + a + a = 180 cm
3a = 180 cm
a= (180/3) = 60 cm
Now, Semi-perimeter (s)= (180/2) = 90 cm
∵ Area of triangle 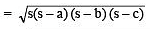
Area of the given triangle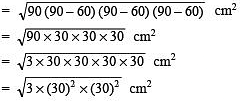
= 30 x 30 x√3 = 900√3 cm2
Thus, the area of the given triangle = 900√3cm2.
Q2. The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 122 m, 22 m and 120 m (see Fig). The advertisements yield an earning of ₹ 5000 per m2 per year. A company hired one of its walls for 3 months. How much rent did it pay?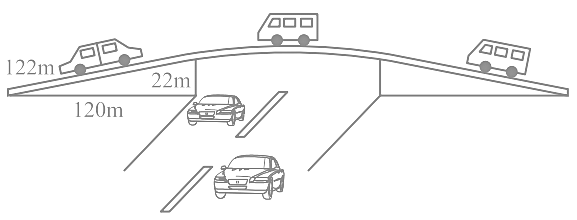 Ans: The sides of the triangular wall are a = 122 m, b = 120 m, c = 22 m.
Ans: The sides of the triangular wall are a = 122 m, b = 120 m, c = 22 m.
Now, the perimeter will be
∴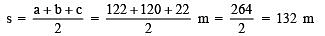
∵ The area of a triangle is given by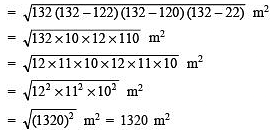
∵ Rent for 1 year (i.e. 12 months) per m2 = Rs 5000
∴ Rent for 3 months per m2 = Rs 5000 x (3/12)
⇒ Rent for 3 months for 1320 m2 = 5000 x (3/12) x 1320 = 5000 x 3 x 110 = Rs16,50,000.
Q3. There is a slide in a park. One of its side walls has been painted in some colour with a message “KEEP THE PARK GREEN AND CLEAN” (see Fig). If the sides of the wall are 15 m, 11 m and 6 m, find the area painted in colour.
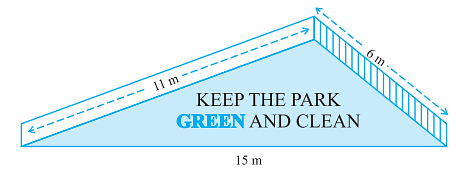
Ans: Sides of the wall a = 15 m, b = 11 m, c = 6 m.
∴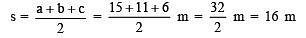
The area of the triangular surface of the wall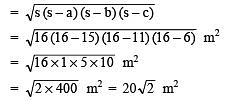
Thus, the required area painted in colour = 20√2 m2.
Q4. Find the area of a triangle, two sides of which are 18 cm and 10 cm and the perimeter is 42 cm.
Ans: Let the sides of the triangle be a = 18 cm, b = 10 cm and c =?
∵ Perimeter (2s) = 42 cm
s = (42/2) = 21 cm
c = 42 – (18 + 10) cm = 14 cm
∵ Area of a triangle = 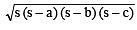
∴ Area of the given triangle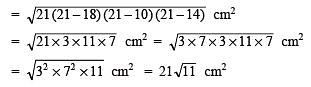
Thus, the required area of the triangle = 21√11 cm2.
Q5. Side of a triangle is in the ratio of 12: 17: 25 and its perimeter is 540 cm. Find its area.
Ans: The perimeter of the triangle = 540 cm.
Semi-perimeter of the triangle, s= (540/2) = 270 cm
∵ The sides are in the ratio of 12: 17: 25.
∴ Let, the side be a = 12x cm, b = 17x cm, c = 25x cm.
12x + 17x + 25x = 540
54x = 540
x = (540/54) = 10
∴ a = 12 x 10 = 120 cm, b = 17 x 10 = 170 cm, c = 25 x 10 = 250 cm.
(s – a) = (270 – 120) cm = 150 cm
(s – b) = (270 – 170) cm = 100 cm
(s – c) = (270 – 250) cm = 20 cm
∴ Area of the triangle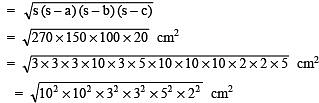
= 10 x 10 x 3 x 3 x 5 x 2 cm2 = 9,000 cm2
Thus, the required area of the triangle = 9,000 cm2.
Q6. An isosceles triangle has a perimeter of 30 cm and each of the equal sides is 12 cm. Find the area of the triangle.
Ans: Equal sides of the triangle are 12 cm each.
Let the third side = x cm.
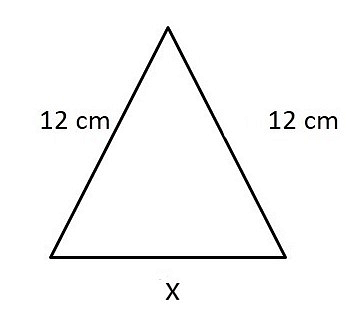 ∵ Perimeter = 30 cm
∵ Perimeter = 30 cm
∴ 12 cm + 12 cm + x cm = 30 cm
x = 30 – 12 – 12 = 6 cm
Semi-perimeter = (30/2) cm = 15 cm
∴ Area of the triangle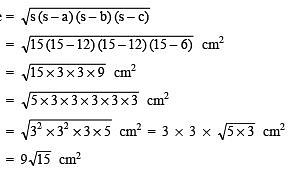
Thus, the required area of the triangle = 9√15 cm2.
|
44 videos|412 docs|54 tests
|
FAQs on NCERT Solutions for Class 9 Maths - Heron’s Formula (Exercise 10.1)
| 1. What is Heron's Formula and how is it used to find the area of a triangle? |  |
| 2. How do you find the semi-perimeter of a triangle? |  |
| 3. Can Heron's Formula be used for triangles with integer side lengths? |  |
| 4. What are the steps to apply Heron’s Formula effectively? |  |
| 5. What types of triangles can Heron's Formula be applied to? |  |

|
Explore Courses for Class 9 exam
|

|


















