NCERT Solutions for Class 9 Maths Chapter 10 - Exercise 10.1 Circles (Old Syllabus)
Q.1. Fill in the blanks:
(i) The centre of a circle lies in ____________ of the circle. (exterior/ interior)
(ii) A point, whose distance from the centre of a circle is greater than its radius lies in __________ of the circle. (exterior/ interior)
(iii) The longest chord of a circle is a _____________ of the circle.
(iv) An arc is a ___________ when its ends are the ends of a diameter.
(v) Segment of a circle is the region between an arc and _____________ of the circle.
(vi) A circle divides the plane, on which it lies, in _____________ parts.
Solution: (i) The centre of a circle lies in interior of the circle.
(ii) A point, whose distance from the centre of a circle is greater than its radius lies in exterior of the circle.
(iii) The longest chord of a circle is a diameter of the circle.
(iv) An arc is a semicircle when its ends are the ends of a diameter.
(v) Segment of a circle is the region between an arc and chord of the circle.
(vi) A circle divides the plane, on which it lies, in 3 (three) parts.
Q.2. Write True or False: Give reasons for your Solutions.
(i) Line segment joining the centre to any point on the circle is a radius of the circle.
(ii) A circle has only finite number of equal chords.
(iii) If a circle is divided into three equal arcs, each is a major arc.
(iv) A chord of a circle, which is twice as long as its radius, is a diameter of the circle.
(v) Sector is the region between the chord and its corresponding arc.
(vi) A circle is a plane figure.
Solution: (i) True. Any line segment drawn from the centre of the circle to any point on it is the radius of the circle and will be of equal length.
(ii) False. There can be infinite numbers of equal chords in a circle.
(iii) False. For unequal arcs, there can be major and minor arcs. So, equal arcs on a circle cannot be said as a major arc or minor arc.
(iv) True. Any chord whose length is twice as long as the radius of the circle always passes through the centre of the circle and thus, it is known as the diameter of the circle.
(v) False. A sector is a region of a circle between the arc and the two radii of the circle.
(vi) True. A circle is a 2d figure and it can be drawn on a plane.
Circle FAQs
Q. 1 What is a circle?
Ans. A circle is defined as a set of all points in a plane which are equally spaced from a fixed point. The fixed point is called the centre of the circle and the distance between any point on the circle and its centre is called the radius of the circle.
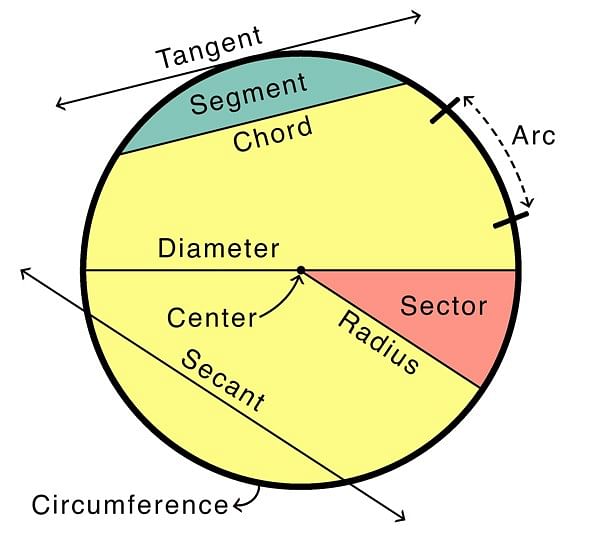
Q.2 Name the various parts of a circle.
Ans.
Based on the positions and their properties a circle has different parts. The important parts of a circle are explained below in detail.
Arc - It is basically the connected curve of a circle.
Sector - A region bounded by two radii and an arc.
Segment - It is the region bounded by a chord and an arc lying between the chord’s endpoints. Points to remember that segments do not contain the centre.
Secant - It is a straight line which cuts the circle at two points. It is also known as an extended chord.
Chord - It is a line segment that joins two points on the circle. The endpoints of the chord lie on the circle.
Tangent - It is a straight line touching the circle at a single point.
Centre - The midpoint of a circle is known as the centre.
 Parts of a circle
Parts of a circle
Q.3 What is the radius and diameter of a circle?
Ans.
A line segment connecting the centre of a circle to any point on the circle itself is known as the radius of the circle. The radius is denoted by R or r.
Diameter is defined as the longest chord of the circle. It is twice the length of radius i.e. d = 2r. The radius of the circle can be derived from the diameter of the circle. Formula for radius of circle is given by r= d/2.
Q.4 What are the various properties of circles?
Ans.
The important basic properties of the circle are as follows:
The outer line of a circle ( which is the circumference ) is at equidistant from the centre.
The diameter of the circle divides the circle into two equal parts.
Two circles are said to be congruent to each other if they have equal radii.
Circles which are different in size or have different radii are known as similar circles.
The diameter is the largest chord of the circle and is double the radius.
|
5 videos|378 docs|164 tests
|
FAQs on NCERT Solutions for Class 9 Maths Chapter 10 - Exercise 10.1 Circles (Old Syllabus)
| 1. What is the formula to find the area of a circle? |  |
| 2. How do you find the circumference of a circle? |  |
| 3. Can the diameter of a circle be equal to its radius? |  |
| 4. What is the relationship between the radius and the diameter of a circle? |  |
| 5. How is the circumference of a circle related to its diameter? |  |

|
Explore Courses for CAT exam
|

|