Fluid Kinematics | Civil Engineering SSC JE (Technical) - Civil Engineering (CE) PDF Download
Chapter 4 Fluid Kinematics
Steady & unsteady flow If the fluid and flow characteristics (such as density, velocity, pressure etc.) at a point do not change with time, the flow is said to be steady flow. If the fluid and flow variables at a point may change with time, the flow will be unsteady.
Uniform and Non-uniform flow If the velocity vector at all points in the flow is same at any instant of time, the flow is uniform flow. If the velocity vector varies from point to point at any instant of time, the flow will be non-uniform laminar flow generally occess at low velocities while turbulent flow occurs at high velocities.
Laminar and Turbulent flow In laminar flow, the particles moves in layers sliding smoothly over the adjacent layers while in turbulent flow particles have the random and erratic movement, intermixing in the adjacent layers.
Streamline
A streamline is an imaginary line drawn in a flow field such that a tangent drawn at any point on this time represents the direction of velocity vector at that point.
There is no velocity component normal to stream lines.
Pathline A pathline is a curve traced by a single fluid particle during its motion. The path can cross each other.
Streakline When a dye is injected in a liquid or smoke in a gas so as to trace the subsequent motion of liquid particles passing a fixed point, the path followed by the dye or smoke is called the streakline.
In a steady flow streamline, pathline & streak lines are same.
Stream tube Stream lines are drawn through a closed curve, they form a boundary surface across which fluid cannot penetrate.
Stream-line equation in 3- D

Fluid Acceleration
Tangential acceleration (as ) = V/t (local acceleration) +V (convective acceleration)
(convective acceleration)
Normal acceleration (an) = Vn/2 (local acceleration) + 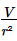 (convective acceleration)
(convective acceleration)
Type of flow | Convective Acceleration | Temporal Acceleration |
Steady & uniform | 0 | 0 |
Steady & non-uniform | Exists | 0 |
Unsteady & uniform | 0 | Exists |
Unsteady & non-uniform | Exists | Exists |
Acceleration in three dimensional-flow


Above equations are valid for steady & unsteady flows uniform & non uniform flow. Continuity Equations For incompressible and steady 3 dimensional flow
For compressible and 3 dimensional unsteady flow
For compressible one dimensional flow
r1 A1 V1 = r2 A2 V2
One dimensional differential form

For incompressible one dimensional flow A1V1 = A2V2
Circulation Circulation is defined as the summation of the product of velocity along the element of curve and element length ds.
G = òv cos qds
- Vorticity
Vorticity is circulation per unit area

Vorticity at a point, x = 
xZ =circulation/area
- Irrotational and Rotational flow
- In irrotational flow, the vorticity is zero, at all points in the flow region while for rotational flow, vorticity is non–zero.
- Flow outside the boundary layer has irrotational characteristic while that within the boundary layer is rotational characteristic.
- Rotational component in x – y plane

W = 1/2 N Hence, w = 0
For irrotational flow in 3 – D Ñ ´ V = 0
- Velocity potential ( f)

- Flow always occurs in the direction of decreasing potential.
- If fexists and satisfies continuity equation, then it represents the possible irrotational flow..
- If fsatisfied Laplace equation then it is the case of steady incompressible and irrotational flow..
- Laplace equation is given by 22

- Streamline function ( y )

- If y exists, then it satisfies continuity equation which can be rotational or irrotational.
- If stream function satisfies Laplace equation then it is case of irrotational flow.
- Flow net: fand y lines intersect orthogonal to each other..
|
2 videos|122 docs|55 tests
|
FAQs on Fluid Kinematics - Civil Engineering SSC JE (Technical) - Civil Engineering (CE)
| 1. What is fluid kinematics in civil engineering? |  |
| 2. How is fluid kinematics important in civil engineering? |  |
| 3. What are the common methods used to analyze fluid kinematics in civil engineering? |  |
| 4. What are the key parameters studied in fluid kinematics? |  |
| 5. How can fluid kinematics be applied in civil engineering projects? |  |
|
2 videos|122 docs|55 tests
|



















