GATE Past Year Questions: Bending of Beams | Strength of Materials (SOM) - Mechanical Engineering PDF Download
Q1: Which of the following beam(s) is/are statically indeterminate? (2024)
(a) 
(b) 
(c) 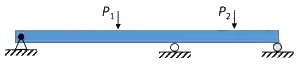
(d) 
Ans: (c,d)
Sol: Statically indeterminate structures are those structures that cannot be analyzed using statics or equations of equilibrium. In such cases, the number unknowns exceeds the number of equilibrium equation available. Checking each option: For option (A), Number of unknown=2 Number of equilibrium equation=2 For option (B), Number of unknown=2 Number of equilibrium equation=2 For option (C), Number of unknown=3 Number of equilibrium equation=2 For option (D), Number of unknown=3 Number of equilibrium equation=3 So, (C) is statically indeterminate structures. Note: Official answer key has been given as option (C) and (D), which can be challenged by students.
Statically indeterminate structures are those structures that cannot be analyzed using statics or equations of equilibrium. In such cases, the number unknowns exceeds the number of equilibrium equation available.
Checking each option:
For option (A),
Number of unknown=2
Number of equilibrium equation=2
For option (B),
Number of unknown=2
Number of equilibrium equation=2
For option (C),
Number of unknown=3
Number of equilibrium equation=2
For option (D),
Number of unknown=3
Number of equilibrium equation=3
So, (C) is statically indeterminate structures. Note: Official answer key has been given as option (C) and (D), which can be challenged by students.
Q2: The effective stiffness of a cantilever beam of length L and flexural rigidity EI subjected to a transverse tip load W is (2023)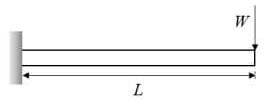
(a) 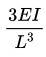
(b) 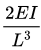
(c) 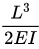
(d) 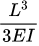
Ans: (a)
Sol: 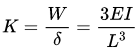
Q3: A cantilever beam with a uniform flexural rigidity 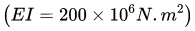 is loaded with a concentrated force at its free end. The area of the bending moment diagram corresponding to the full length of the beam is 10000 N.m2. The magnitude of the slope of the beam at its free end is ____________micro radian (round off to the nearest integer). (2021 Set - 2)
is loaded with a concentrated force at its free end. The area of the bending moment diagram corresponding to the full length of the beam is 10000 N.m2. The magnitude of the slope of the beam at its free end is ____________micro radian (round off to the nearest integer). (2021 Set - 2)
(a) 42
(b) 50
(c) 65
(d) 84
Ans: (b)
Sol: 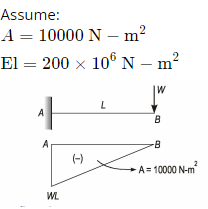
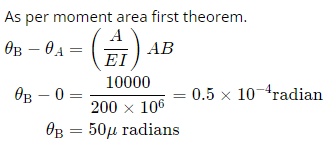
Q4: A plane frame PQR (fixed at P and free at R) is shown in the figure. Both members (PQ and QR) have length, L, and flexural rigidity, EI. Neglecting the effect of axial stress and transverse shear, the horizontal deflection at free end, R, is (2021 Set - 2)
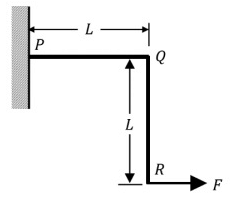
(a) 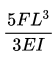
(b) 
(c) 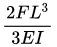
(d) 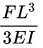
Ans: (b)
Sol: 

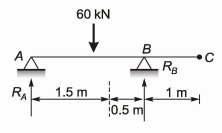
Q5: An overhanging beam PQR is subjected to uniformly distributed load 20 kN/m as shown in the figure. (2021 Set - 1)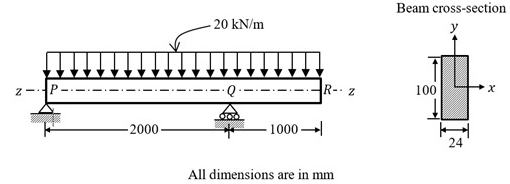
The maximum bending stress developed in the beam is ________MPa (round off to one decimal place).
(a) 125
(b) 250
(c) 325
(d) 450
Ans: (b)
Sol: 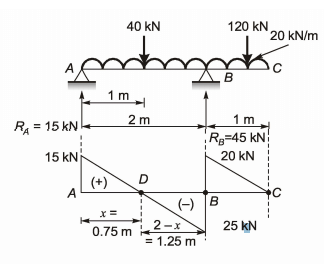
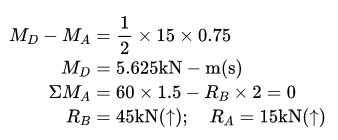

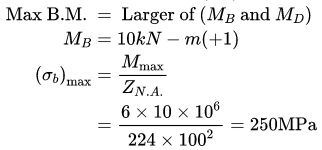
Q6: A cantilever beam of length, L, and flexural rigidity, EI, is subjected to an end moment, M, as shown in the figure. The deflection of the beam at x = L/2 is (2021 Set -1)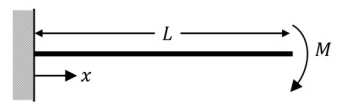
(a) 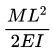
(b) 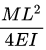
(c) 
(d) 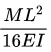
Ans: (c)
Sol: 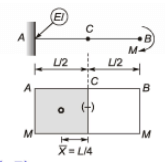
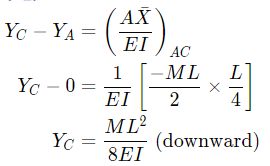
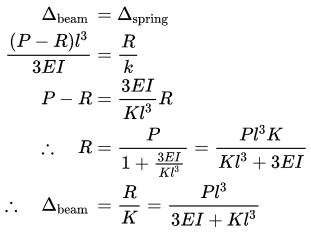
Q7: A cantilever of length l, and flexural rigidity EI, stiffened by a spring of stiffness k, is loaded by transverse force P, as shown
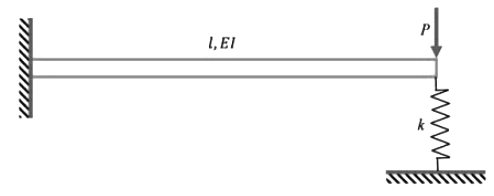
The transverse deflection under the load is (2020 Set - 2)
(a) 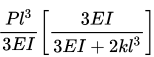
(b) 
(c) 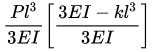
(d) 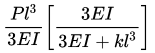
Ans: (d)
Sol: 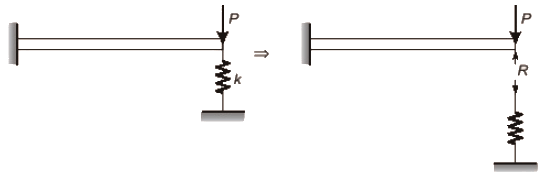
Q8: A horizontal cantilever beam of circular cross-section, length 1.0 m and flexural rigidity EI= 200 N.m2 is subjected to an applied moment MA= 1.0 N⋅m at the free end as shown in the figure. The magnitude of the vertical deflection of the free end is _______mm (round off to one decimal place). (2019 Set -2)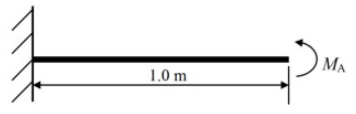
(a) 1.2
(b) 5.3
(c) 8.2
(d) 2.5
Ans: (d)
Sol: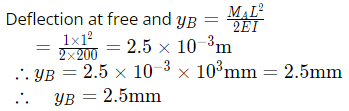
Q9: A prismatic, straight, elastic, cantilever beam is subjected to a linearly distributed transverse load as shown below. If the beam length is L, Young's modulus E, and area moment of inertia I, the magnitude of the maximum deflection is (2019 Set -2)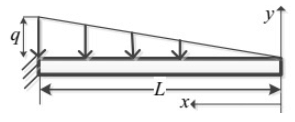
(a) 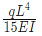
(b) 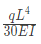
(c) 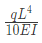
(d) 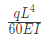
Ans: (b)
Sol: Double Integration method:
Let x be distance from the free end
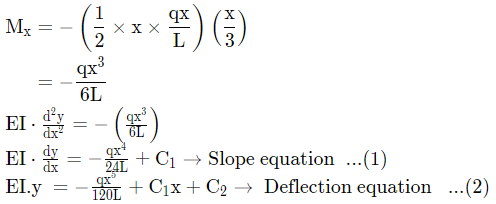
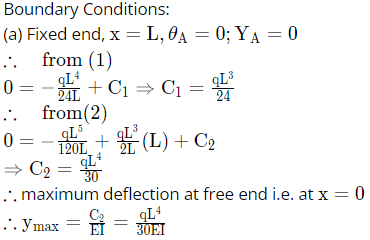
Q10: Consider a prismatic straight beam of length L=πm, pinned at the two ends as shown in the figure. The beam has a square cross-section of side p=6 mm. The Young's modulus E= 200 GPa, and the coefficient of thermal expansion α=3×10-6K-1. The minimum temperature rise required to cause Euler buckling of the beam is ________ K. (2019 Set - 1) (a) 0
(a) 0
(b) 0.5
(c) 1
(d) 2
Ans: (c)
Sol: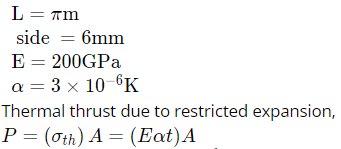
Q11: Consider an elastic straight beam of length L=10πm, with square cross-section of side a=5 mm, and Young's modulus E=200 GPa. This straight beam was bent in such a way that the two ends meet, to form a circle of mean radius R. Assuming that Euler-Bernoulli beam theory is applicable to this bending problem, the maximum tensile bending stress in the bent beam is __________ MPa. (2019 Set-1)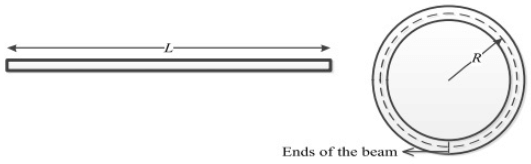
(a) 100
(b) 200
(c) 50
(d)150
Ans: (a)
Sol:
L = 10π m
a = 5 mm
E = 200 GPa
Length of wire: L = πD = 2πR
10π = 2πR
R = 5 m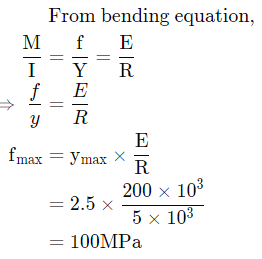
Q12: The minimum axial compressive load, P, required to initiate buckling for a pinned-pinned slender column with bending stiffness EI and length L is (2018 Set -2)
(a) 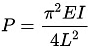
(b) 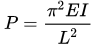
(c) 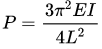
(d) 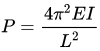
Ans: (b)
Sol:
For both ends hinged buckling load,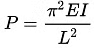
Q13: A steel column of rectangular section (15 mm x 10 mm) and length 1.5 m is simply supported at both ends. Assuming modulus of elasticity, E = 200 GPa for steel, the critical axial load (in kN) is ____ (correct to two decimal places).
(a) 2.25
(b) 1.09
(c) 2.04
(d) 2.68
Ans: (b)
Sol: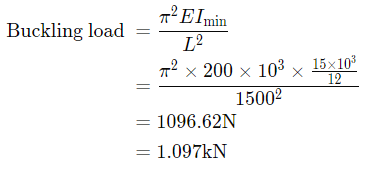
[1988]
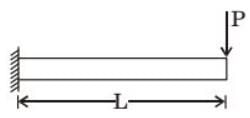
[2003]
[2005]
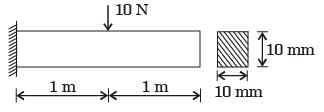
[2007]
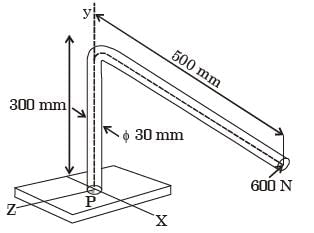
The normal and shear stresses in MPa at point P are respectively
[2007]
[2010]
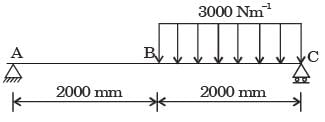
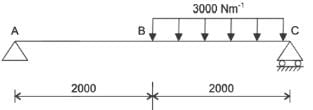
The maximum magnitude of bending stress (in MPa) is given by [2010]
[2011]
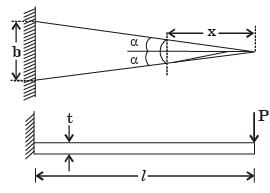
The area moment of inertia about the neutral axis of a cross-section at a distance x measured from the free end is
[2011]
[2014]
[2015]
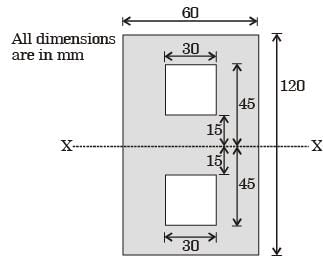
[2016]
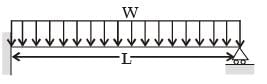
[2017]
[2008]
[2008]
[2008]
|
37 videos|39 docs|45 tests
|
FAQs on GATE Past Year Questions: Bending of Beams - Strength of Materials (SOM) - Mechanical Engineering
| 1. What is the formula for calculating the bending stress in a beam? |  |
| 2. How do you determine the maximum deflection of a beam under a certain load? |  |
| 3. What are the different types of beams based on their support conditions? |  |
| 4. How does the material properties of a beam affect its bending behavior? |  |
| 5. What is the significance of the neutral axis in the bending of beams? |  |

|
Explore Courses for Mechanical Engineering exam
|

|






























 = 6.885 × 106 mm4
= 6.885 × 106 mm4















