Class 9 Maths Chapter 8 HOTS Questions - Quadrilaterals
Q1: Find all the angles of a parallelogram if one angle is 80°.
For a parallelogram ABCD, opposite angles are equal.
So, the angles opposite to the given 80° angle will also be 80°.
It is also known that the sum of angles of any quadrilateral = 360°.
So, if ∠A = ∠C = 80° then,
∠A + ∠B + ∠C + ∠D = 360°
Also, ∠B = ∠D
Thus,
80° + ∠B + 80° + ∠D = 360°
Or, ∠B +∠ D = 200°
Hence, ∠B = ∠D = 100°
Now, all angles of the quadrilateral are found which are:
∠A = 80°
∠B = 100°
∠C = 80°
∠D = 100°
Q2: In the adjoining figure, ABCD is a parallelogram. Find the angles A, B, C, and D.
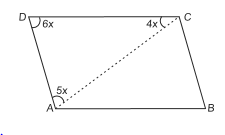 Sol.
Sol.
In ∆ACD, 4x + 5x + 6x = 180°
⇒ 15x = 180°
⇒ x = (180o/15) = 12°
∴ ∠D = 6 × 12° = 72°
⇒ B = 72° [∵ opposite angles of ||gm are equal]
∵ ∠ A + ∠ D = 180° [co-interior angles]
∴ ∠A = 180° – ∠D = 180° – 72° = 180°
⇒ Therefore, ∠ C = 108°
Q3: The sides AD and BC of a quadrilateral are produced as shown in the given figure. Prove that x = ((a+b)/2).
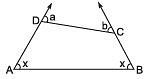
Sol.
We have ∠a + ∠ADC = 180° [linear pair]
Similarly, ∠b + ∠BCD = 180°
Adding (a + b) + ∠ADC + ∠BCD = 360° ...(1)
But x + x + ∠ADC + ∠BCD = 360° ...(2)
From (1) and (2)
x + x + ∠ADC + ∠BCD = a + b + ∠ADC + ∠BCD
⇒ x + x = a + b
⇒ 2x = a + b
⇒ x = ((a+b)/2), hence proved.
Q4: L, M, N, K are midpoints of sides BC, CD, DA and AB respectively of a square ABCD.
Prove that DL, DK, BM and BN enclose a rhombus.
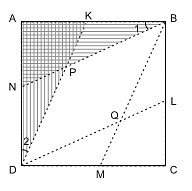
Sol.
BK = DM [halves of equal sides]
∴ BM || DK. Similarly, BN || DL
Also, ∆ ABN ≌ ∆ ADK [SAS congruency]
⇒ ∠ 1 = ∠ 2
Also, ∆PND ≌ ∆PKB [ASA congruency]
⇒ PB = PD
⇒ Therefore, DQBP is a rhombus.
Q5: PQRS is a parallelogram. PS is produced to M so that SM = SR and MR produced meet PQ produced at N. Prove that QN = QR.
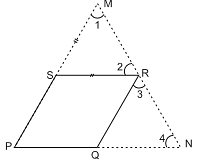 Sol.
Sol.
In ∆SMR, SM = SR
⇒ ∠ 1 = ∠ 2 [Angles opposite to equal sides are equal]
∠1 = ∠3
[∵QR || PM, corresponding angles are equal]
Similarly, ∠2 = ∠4 [corresponding angles]
⇒ ∠ 3 = ∠ 4
⇒ Hence in ∆ QRN, QN = QR
Q6: Calculate all the angles of a quadrilateral if they are in the ratio 2:5:4:1.
Sol.
As the angles are in the ratio 2:5:4:1, they can be written as-
2x, 5x, 4x, and x
Now, as the sum of the angles of a quadrilateral is 360°,
2x + 5x + 4x + x = 360°
Or, x = 30°
Now, all the angles will be,
2x =2 × 30° = 60°
5x = 5 × 30° = 150°
4x = 4 × 30° = 120°, and
x = 30°
Q7: Calculate all the angles of a parallelogram if one of its angles is twice its adjacent angle.
Sol.
Let the angle of the parallelogram given in the question statement be “x”.
Now, its adjacent angle will be 2x.
It is known that the opposite angles of a parallelogram are equal.
So, all the angles of a parallelogram will be x, 2x, x, and 2x
As the sum of interior angles of a parallelogram = 360°,
x + 2x + x + 2x = 360°
Or, x = 60°
Thus, all the angles will be 60°, 120°, 60°, and 120°.
Q8: In a trapezium ABCD, AB∥CD. Calculate ∠C and ∠D if ∠A = 55° and ∠B = 70°
Sol.
In a trapezium ABCD, ∠A + ∠D = 180° and ∠B + ∠C = 180°
So, 55° + ∠D = 180°
Or, ∠D = 125°
Similarly,
70° + ∠C = 180°
Or, ∠C = 110°
Q9: In a rectangle, one diagonal is inclined to one of its sides at 25°. Measure the acute angle between the two diagonals.
Let ABCD be a rectangle where AC and BD are the two diagonals which are intersecting at point O.
Now, assume ∠BDC = 25° (given)
Now, ∠BDA = 90° – 25° = 65°
Also, ∠DAC = ∠BDA, (as diagonals of a rectangle divide the rectangle into two congruent right triangles)
So, ∠BOA = the acute angle between the two diagonals = 180° – 65° – 65° = 50°
Q10: If the bisectors of the angles of a quadrilateral enclose a rectangle, then show that it is a parallelogram.
Sol.
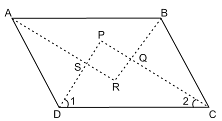
Angle bisectors of the quadrilateral ABCD enclose a rectangle PQRS.
∴ ∠P = 90o
⇒ In Δ PCD, ∠1+∠2 = 90o
But, ∠1 and ∠2 are 1/2∠D and 1/2 ∠C respectively.
⇒ ∠D +∠C = 180o [∵ 2∠1 + 2∠2 = 180o]
⇒ ∠ D and ∠ C form a pair of co-interior supplementary angles AD || BC
Similarly, AB || DC ⇒ ABCD is a parallelogram.
|
44 videos|412 docs|54 tests
|
FAQs on Class 9 Maths Chapter 8 HOTS Questions - Quadrilaterals
| 1. What are the properties of a quadrilateral? |  |
| 2. How many types of quadrilaterals are there? |  |
| 3. What is the difference between a square and a rectangle? |  |
| 4. How do you calculate the area of a quadrilateral? |  |
| 5. What is the sum of the angles in a quadrilateral? |  |

|
Explore Courses for Class 9 exam
|

|


















