Important Concepts: Factors and Multiples | Quantitative for GMAT PDF Download
| Table of contents |

|
| Introduction |

|
| How to Find Factors and Multiples? |

|
| Steps to Find Factors of a Number |

|
| Finding Multiples of a Number |

|
| Common Factors and Multiples |

|
| Properties of Factors and Multiples |

|
Introduction
Factors and multiples have a close connection. A factor of a number divides it entirely, leaving no remainder. When we express a number as the product of two other numbers, like p × q = z, z becomes a multiple of both p and q. This means p and q are factors of z because z can be divided evenly by both. For instance, in 6 × 2 = 12, 6 and 2 are factors of 12, and 12 is a multiple of both 6 and 2.
How to Find Factors and Multiples?
To determine the factors and multiples of a given number, like 'p', we identify the numbers that divide 'p' completely, leaving no remainder. Let's consider the number 28 as an example. How do we discover the factors of 28?
Steps to Find Factors of a Number
- Step 1: List the two numbers such that their product gives 28.
- Step 2: Let's say we take 4 and 7 as the numbers.
- Step 3: Here 28 is divisible by 4 and 7 without leaving any remainder.
- Step 4: Hence, 28 can be factored in as 28 = 7 × 4.
- Step 5: Further, there are finite numbers by which a number can be divided without leaving any remainder. List out the numbers. Here, in the case of 28, the numbers are 1, 2, 4, 7, 14, and 28.
- Step 6: Factors of 28 can be listed as 1, 2, 4, 7, 14, and 28.
1 and the number itself are always the factors of the respective number.
Finding Multiples of a Number
Multiples of a number are values obtained by multiplying that number by whole numbers. Using the example of 28, how do we identify its multiples? They are all the numbers derived from multiplying 28 by other whole numbers. Consider the skip counting method depicted in the image. This technique provides an easy way to determine the multiples of any given number.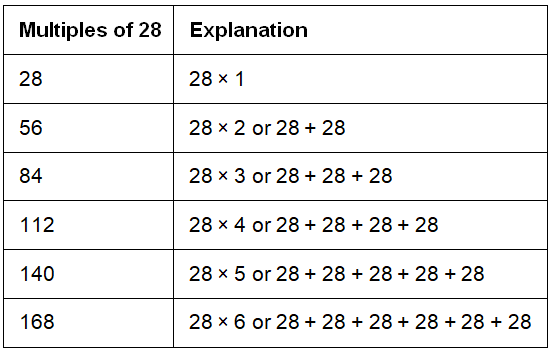
Here, 28, 56, 84, 112, 140, and 168 are a few multiples of 28. There are infinite multiples of any given number.
Common Factors and Multiples
In this section, you will learn how to find common factors and multiples of any number. We know that a factor is a number that exactly divides the given number. Hence, a factor is nothing but a divisor of the given number. To find the factors, we can use the multiplication as well as the division method. To check if two or more numbers have common factors between them we can follow the below steps:
- Write the given numbers.
- Write all the possible factors of the given numbers
- Find the factors which are the same in both the numbers.
- Encircle the common factors.
This way we can easily find common factors between two or more numbers. Let us look at some examples.
Common factors of 30 and 42:

Therefore, the common factors of 30 and 42 are 1, 2, 3, and 6.
Here is another example with three numbers. The common factors of 42, 70, and 84 are shown in the image below.
Hence the common factors of 42, 70, and 84 are 1, 2, 7, and 14. Now let us look at ways to find common multiples.
There are various methods to find common multiples. Two of such ways are:
- Listing multiples method. In this, we list the multiples of each number and then we find their common multiples. It is one of the simplest methods by which we can find the common multiples of two or more numbers.
- Venn diagram method
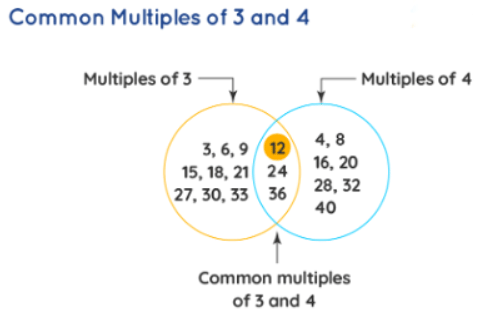
Let us use the Venn diagram method. First, mark the multiples of any 2 numbers, let's say 3 and 4 in two separate circles. Look for the common numbers coming in both circles. The circle of multiples of 3 intersects with the circle of multiples of 4. The intersection part has the common multiples that belong to 3 as well as 4. Please note that there are infinite numbers of common multiples for every pair of numbers. The first three common multiples of 3 and 4 are 12, 24, and 36.
Now, let us look at an example of the listing multiples method. To find the common multiples of 2 and 4, we list their multiples and then find their common multiples.
- Multiples of 2: 2, 4, 6, 8, 10, 12, 14, 16, 18, 20. 22, 24, etc.
- Multiples of 4: 4, 8, 12, 16, 20, 24, 28, 32, 36, 40, etc.
- Common multiples of 2 and 4: 4, 8, 12, 16, 20, 24, etc …
If a number is a factor of another number, then their common multiples are all the multiples of the larger number (as in the above example).
Properties of Factors and Multiples
There are many properties of factors and multiples that will help you to understand this concept properly.
Those properties are listed below:
- Every number has 1 as a factor.
- Every number can be multiplied by 0 to produce a multiple.
- Factors and multiples are concepts exclusively related to whole numbers.
- For any given number, 1 is its smallest factor, and the number itself is the largest factor.
- Each number is a multiple of itself.
- There's an unlimited quantity of multiples for any number, but there's a finite set of factors.
- When a number only has two factors—1 and itself—it's termed a prime number in mathematics.
Important Notes
- Multiples of any number are infinite. Therefore, a set of any two numbers can have an infinite number of common multiples.
- The Least Common Multiple (LCM) is the smallest common multiple of sets of any two or more numbers. For example, the LCM of 3 and 4 is 12.
Solved Examples
Example 1: Find the common factors and multiples of 4, 8, and 12.
Ans: To find the common factors and multiples of 4, 8, and 12 we will use the listing method.
Let us write separately factors of 4,8 and 12. There are 3 factors of 4 which are 1, 2, and 4. There are 4 factors of 8 listed as 1, 2, 4, and 8. There are 6 factors of 12 which are 1, 2, 3, 4, 6, and 12.
The common factors of 4, 8, and 12 are 1, 2, and 4.
Let us make a list of multiples of 4, 8, and 12.
Multiples of 4 = 4, 8, 12, 16, 20, 24, 28, 32, 36, 40, 44, 48, 52, 56, 60, 64, 68, 72 ...
Multiples of 8 = 8, 16, 24, 32, 40, 48, 56, 64, 72, 80 ...
Multiples of 12 = 12, 24, 36, 48, 60, 72, ...
So, the common multiples of 4, 8, and 12 are 24, 48, 72, ...
Example 2: What are the factors and multiples of 60?
Ans: The factors of 60 are all the whole numbers that can divide 60 without leaving any remainder. So, the factors of 60 are 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 30 and 60. The mutiples of 60 can be found by multiplying 60 with whole numbers starting from 1.
60 × 1 = 60
60 × 2 = 120
60 × 3 = 180
60 × 4 = 240
60 × 5 = 300
So, the list of multiples of 60 includes 60, 120, 180, 240, 300, and so on.
Therefore, the factors and multiples of 60 are:
Factors = 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 30 and 60
Multiples = 60, 120, 180, 240, 300, ...
|
115 videos|106 docs|113 tests
|
FAQs on Important Concepts: Factors and Multiples - Quantitative for GMAT
| 1. How do you find the factors of a number? |  |
| 2. Can a number have more than one set of factors? |  |
| 3. How do you find the multiples of a number? |  |
| 4. Can a number have infinite multiples? |  |
| 5. What are common factors and multiples? |  |

|
Explore Courses for GMAT exam
|

|

















