Class 8 Exam > Class 8 Notes > Mathematics (Maths) Class 8 > Important Formulas: Exponents & Powers
Important Formulas: Exponents & Powers | Mathematics (Maths) Class 8 PDF Download
Laws of Exponents
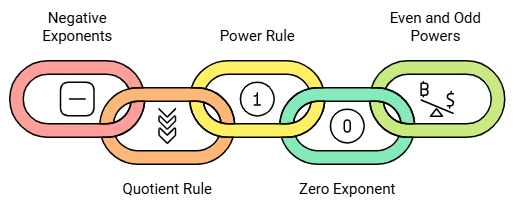
- Here are the laws of exponents when a and b are non-zero integers and m, n are any integers.
- a-m = 1/am
- am / an = am-n
- (am )n = amn
- am x bm = (ab)m
- am / bm = (a/b)m
- a0 =1
- (a/b)-m =(b/a)m
- (1)n = 1 for infinitely many n.
- (-1)p =1 for any even integer p
The document Important Formulas: Exponents & Powers | Mathematics (Maths) Class 8 is a part of the Class 8 Course Mathematics (Maths) Class 8.
All you need of Class 8 at this link: Class 8
|
79 videos|408 docs|31 tests
|
FAQs on Important Formulas: Exponents & Powers - Mathematics (Maths) Class 8
| 1. What is an exponent and how is it related to powers? |  |
Ans. An exponent is a number that represents how many times a base number is multiplied by itself. It is written as a superscript to the right of the base number. Exponents are related to powers because they indicate how many times the base number should be multiplied by itself to get the power. For example, in the expression 2^3, the base is 2 and the exponent is 3, which means 2 is multiplied by itself three times to get the power of 8.
| 2. How do you simplify expressions with exponents and powers? | |
Ans. To simplify expressions with exponents and powers, you can use the rules of exponents. These rules include multiplying powers with the same base by adding their exponents, dividing powers with the same base by subtracting their exponents, and raising a power to a power by multiplying the exponents. For example, to simplify (2^3)^2, you multiply the exponents and get 2^6, which is equal to 64.
| 3. How can I calculate the value of a power with a negative exponent? | |
Ans. When a power has a negative exponent, you can calculate its value by taking the reciprocal of the base number and changing the sign of the exponent to positive. For example, to calculate the value of 2^-3, you take the reciprocal of 2, which is 1/2, and change the exponent to positive, resulting in 1/(2^3) or 1/8.
| 4. Can you explain the zero exponent rule? | |
Ans. The zero exponent rule states that any non-zero number raised to the power of zero is equal to 1. This means that no matter what the base number is, if the exponent is zero, the result is always 1. For example, 5^0 is equal to 1 and 10^0 is also equal to 1.
| 5. How do you solve problems involving negative exponents? | |
Ans. To solve problems involving negative exponents, you can use the rule that states that any non-zero number raised to a negative exponent is equal to the reciprocal of the number raised to the positive exponent. For example, to solve 4^-2, you take the reciprocal of 4^2, which is 1/16. So, 4^-2 is equal to 1/16.
Related Exams
About this Document
|
1.9K Views |
|
4.68/5 Rating |
|
Dec 22, 2024 Last updated |
Document Description: Important Formulas: Exponents & Powers for Class 8 2024 is part of Mathematics (Maths) Class 8 preparation.
The notes and questions for Important Formulas: Exponents & Powers have been prepared according to the Class 8 exam syllabus. Information about Important Formulas: Exponents & Powers covers topics
like and Important Formulas: Exponents & Powers Example, for Class 8 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Important Formulas: Exponents & Powers.
Introduction of Important Formulas: Exponents & Powers in English is available as part of our Mathematics (Maths) Class 8
for Class 8 & Important Formulas: Exponents & Powers in Hindi for Mathematics (Maths) Class 8 course.
Download more important topics related with notes, lectures and mock test series for Class 8
Exam by signing up for free. Class 8: Important Formulas: Exponents & Powers | Mathematics (Maths) Class 8
Description
Full syllabus notes, lecture & questions for Important Formulas: Exponents & Powers | Mathematics (Maths) Class 8 - Class 8 | Plus excerises question with solution to help you revise complete syllabus for Mathematics (Maths) Class 8 | Best notes, free PDF download
Information about Important Formulas: Exponents & Powers
In this doc you can find the meaning of Important Formulas: Exponents & Powers defined & explained in the simplest way possible. Besides explaining types of
Important Formulas: Exponents & Powers theory, EduRev gives you an ample number of questions to practice Important Formulas: Exponents & Powers tests, examples and also practice Class 8
tests
|
Explore Courses for Class 8 exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
Important Formulas: Exponents & Powers | Mathematics (Maths) Class 8
,MCQs
,Extra Questions
,practice quizzes
,Viva Questions
,Important Formulas: Exponents & Powers | Mathematics (Maths) Class 8
,shortcuts and tricks
,ppt
,Summary
,Sample Paper
,Semester Notes
,past year papers
,Previous Year Questions with Solutions
,study material
,Important Formulas: Exponents & Powers | Mathematics (Maths) Class 8
,video lectures
,Objective type Questions
,Exam
,Free
,mock tests for examination
,Important questions
,
Additional Information about Important Formulas: Exponents & Powers for Class 8 Preparation
Important Formulas: Exponents & Powers Free PDF Download
The Important Formulas: Exponents & Powers is an invaluable resource that delves deep into the core of the Class 8 exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Important Formulas: Exponents & Powers now and kickstart your journey towards success in the Class 8 exam.
Importance of Important Formulas: Exponents & Powers
The importance of Important Formulas: Exponents & Powers cannot be overstated, especially for Class 8 aspirants.
This document holds the key to success in the Class 8 exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Important Formulas: Exponents & Powers Notes
Important Formulas: Exponents & Powers Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Important Formulas: Exponents & Powers.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Important Formulas: Exponents & Powers Notes on EduRev are your ultimate resource for success.
Important Formulas: Exponents & Powers Class 8 Questions
The "Important Formulas: Exponents & Powers Class 8 Questions" guide is a valuable resource for all aspiring students preparing for the
Class 8 exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Important Formulas: Exponents & Powers on the App
Students of Class 8 can study Important Formulas: Exponents & Powers alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Important Formulas: Exponents & Powers,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Important Formulas: Exponents & Powers is prepared as per the latest Class 8 syllabus.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup