Important Formulas: Linear Equations | Quantitative Aptitude for SSC CGL PDF Download
Introduction to Formulas of Linear Equations
A linear equation, synonymous with an algebraic equation where each term carries an exponent of one, is graphically represented by a straight line. The standard form of a linear equation is y = mx + b, where x is the variable, and y, m, and b are constants.
Formulas & Definitions for Linear Equations
- A linear equation is an algebraic expression where each term has an exponent of one, and its graph forms a straight line.
- The standard form of a linear equation is y = mx + b, where x is the variable, and y, m, and b are constants.
Forms of Linear Equations
There are mainly 3 forms of Linear Equation :
- Standard Form
- Slope-Intercept Form
- Point-Slope Form
1. Standard Form
The standard form of a linear equation is typically written as:
Ax + By = C
Where:
- A and B are coefficients (constants) representing the coefficients of x and y terms, respectively.
- C is a constant term.
The standard form requires that A and B are both integers and that A is non-negative. Also, A and B should not have any common factors other than 1. This form is commonly used in algebraic manipulation and solving systems of linear equations.
2. Slope-Intercept Form
The slope-intercept form of a linear equation is written as:
y=mx+b
Where:
- m is the slope of the line, representing the rate of change between y and x.
- b is the y-intercept, which is the value of y when x is equal to 0. It represents the point where the line intersects the y-axis.
This form is particularly useful for graphing linear equations and quickly identifying the slope and y-intercept of the line.
3. Point-Slope Form
The point-slope form of a linear equation is given by:
y − y1 = m(x − x 1)
Where:
- m is the slope of the line, as explained in the slope-intercept form.
- (x1 ,y1) represents the coordinates of a point on the line.
This form is useful when you know a specific point on the line and its slope, allowing you to write the equation directly without having to calculate the y-intercept.
Linear equations in one variable
- A Linear Equation in one variable is defined as ax + b = 0
- Where, a and b are constant, a ≠ 0, and x is an unknown variable
- The solution of the equation ax + b = 0 is
 We can also say that
We can also say that  is the root of the linear equation ax + b = 0.
is the root of the linear equation ax + b = 0.
Linear equations in two variable
- A Linear Equation in two variables is defined as ax + by + c = 0
- Where a, b, and c are constants and also, both a and b ≠ 0
Linear equations in three variable
- A Linear Equation in three variables is defined as ax + by + cz = d
- Where a, b, c, and d are constants and also, a, b and c ≠ 0
Formulas and Methods to solve Linear equations
Substitution Method
- Step 1: Solve one of the equations either for x or y.
- Step 2: Substitute the solution from step 1 into the other equation.
- Step 3: Now solve this equation for the second variable.
Elimination Method
- Step 1: Multiply both the equations with such numbers to make the coefficients of one of the two unknowns numerically same.
- Step 2: Subtract the second equation from the first equation.
- Step 3: In either of the two equations, substitute the value of the unknown variable. So, by solving the equation, the value of the other unknown variable is obtained.
Cross-Multiplication Method
Suppose there are two equation,
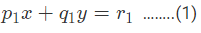
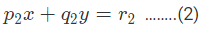
Multiply Equation (1) with p2
Multiply Equation (2) with p1 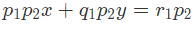
Subtracting,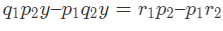
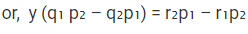
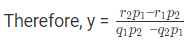
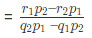
where (p1q2 – p2q1) ≠ 0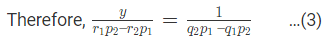
Multiply Equation (1) with q2
Multiply Equation (2) with q1 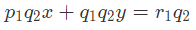
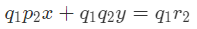
Subtracting,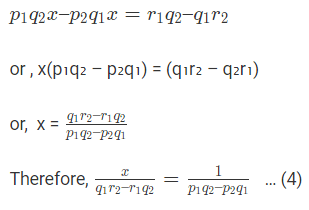
where (p1q2 – p2q1) ≠ 0
From equations (3) and (4), we get,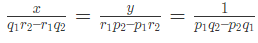
where (p1q2 – p2q1) ≠ 0
Note: Shortcut to solve this equation will be written as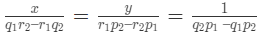
which means,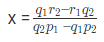
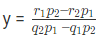
Important Formulas of Linear Equation & key points to Remember
Suppose, there are two linear equations: a1x + b1y = c1 and a2x + b2y = c2
Then,
((a) If 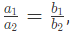 then there will be one solution, and the graphs will have intersecting lines.
then there will be one solution, and the graphs will have intersecting lines.
((b) If 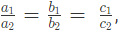 then there will be numerous solutions, and the graphs will have coincident lines.
then there will be numerous solutions, and the graphs will have coincident lines.
((c) If 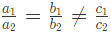 then there will be no solution, and the graphs will have parallel lines.
then there will be no solution, and the graphs will have parallel lines.
Questions on Formulas of Linear Equation
Q1: What is the slope-intercept form of the equation of a line?
(a) y=mx+b
(b) y=mx−b
(c) y=bx+m
(d) y=bx−m
Ans: (a)
The slope-intercept form of a linear equation is y=mx+b, where m represents the slope and b represents the y-intercept.
Q2: What does the standard form of a linear equation look like?
(a) y = mx+b
(b) y = bx+m
(c) Ax + By=C
(d) Ax + By=D
Ans: (c)
The standard form of a linear equation is Ax+By=C, where A and B are coefficients representing the coefficients of x and y, respectively, and C is a constant term.
Q3: How can you eliminate fractions from a linear equation?
(a) Multiply both sides of the equation by a common denominator
(b) Divide both sides of the equation by a common denominator
(c) Add both sides of the equation by a common denominator
(d) Subtract both sides of the equation by a common denominator
Ans: (a)
To eliminate fractions from a linear equation, multiply both sides of the equation by a common denominator. This process will clear the fractions and make the equation easier to solve.
Q4: Which form of a linear equation is useful when you know a specific point on the line and its slope?
(a) Point-Slope Form
(b) Slope-Intercept Form
(c) Standard Form
(d) None of the above
Ans: (a)
The point-slope form of a linear equation is written as y − y1 =m(x − x1), where (1,1)(x1, y1) represents the coordinates of a point on the line, and m is the slope.
Q5: Which of the following is the correct representation of the point-slope form of a linear equation?
(a) y = mx + b
(b) y − y1 = m(x−x1)
(c) y = Ax + By
(d) y = m1x + b
Ans: (b)
The point-slope form of a linear equation is represented as y − y1 = m(x−x1) , where (x1 , y1) represents the coordinates of a point on the line, and m is the slope of the line. This form is useful when you know a specific point on the line and its slope.
|
314 videos|170 docs|185 tests
|
|
314 videos|170 docs|185 tests
|

|
Explore Courses for SSC CGL exam
|

|
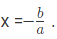 We can also say that
We can also say that 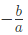 is the root of the linear equation ax + b = 0.
is the root of the linear equation ax + b = 0.
















