Important Formulas: Ratio and Proportion | Quantitative Aptitude (Quant) - CAT PDF Download
In competitive exams, knowing about Ratio and Proportion is really important. These ideas, which come from basic high school math, are super crucial in the Arithmetic section and even show up in other parts like Data Interpretation. Being good at ratio and proportion isn't just helpful by itself; it's like having a super handy tool to solve problems about similar triangles, mixtures, and claims. Getting the hang of these basics isn't just about understanding one thing; it's like having a key that helps solve lots of different questions in various subjects during competitive exams.
Introduction
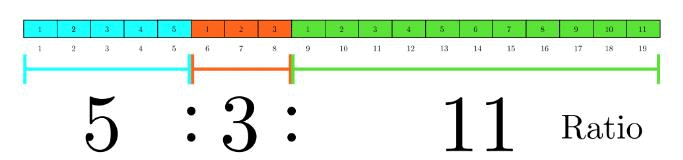
Ratio and Proportion are explained majorly based on fractions. When a fraction is represented in the form of a:b, then it is a ratio whereas a proportion states that two ratios are equal.
- Here, a and b are any two integers.
- The ratio and proportion are the two important concepts, and it is the foundation to understand the various concepts in mathematics as well as in science.

- Ratio and proportions are said to be faces of the same coin. When two ratios are equal in value, then they are said to be in proportion. In simple words, it compares two ratios.
- Proportions are denoted by the symbol ‘::’ or ‘=’.
- In simple words, a comparison of two quantities by division is called a ratio and the equality of two ratios is called proportion. A ratio can be written in different forms like x : y or x/y and is commonly read as, x is to y.
On the other hand, proportion is an equation that says that two ratios are equivalent. A proportion is written as x : y : : z : w, and is read as x is to y as z is to w. Here, x/y = z/w where w & y are not equal to 0.
What is a Ratio?
The ratio is the comparison between similar types of quantities, it is an abstract quantity and does not have any units.
- The ratio of two quantities a and b in the same units is the fraction a/b and we write it as a: b which is equal to a/b

- In the ratio a: b, we call the first term or antecedent and b, the second term or consequent.
- Example 1: The ratio 5 : 9 represents 5/9 with antecedent = 5, consequent = 9.
- Example 2: If we multiply and divide each term of a ratio by the same number (non-zero), it doesn’t affect the ratio like 5:9 = 10:18 = 15:27
Important Properties of Ratio
1. A ratio remains the same if both antecedent and consequent are multiplied or divided by the same non-zero number,
- a/b = pa/pb = qa/qb , p, q ≠0
- a/b = (a/p) / (b/p) = (a/q) / (b/q) , p, q ≠0
2. Two ratios in their fraction notation can be compared just as we compare real numbers.
- a/b = p/q ⟺ aq = bp
- a/b > p/q ⟺ aq > bp
- a/b < p/q ⟺ aq < bp
3. If two ratios a/b and c/d are equal
- a/b = c/d ⟹ b/a = d/c (Invertendo)
- a/b = c/d ⟹ a/c = b/d (Alternendo)
- a/b = c/d ⟹ (a+b)/b = (c+d)/d (Componendo)
- a/b = c/d ⟹ (a-b)/b = (c-d)/d (Dividendo)
4. Key Points to Remember about Ratio:
- The ratio should exist between the quantities of the same kind.
- While comparing two things, the units should be similar.
- There should be significant order of terms.
- The comparison of two ratios can be performed, if the ratios are equivalent like the fractions.
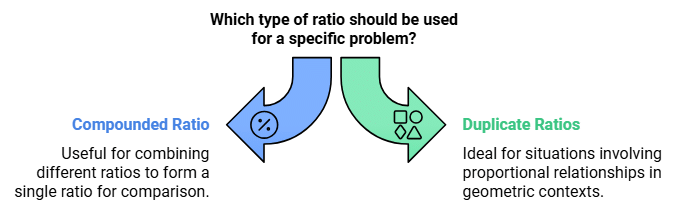
Types of Ratios
1. Compounded Ratio:
The compounded ratio of the ratios:
(a : b), (c : d), (e : f) is (ace : bdf)
2. Duplicate Ratios
- The duplicate ratio of (a : b) is (a2 : b2)
- Sub - duplicate ratio of (a : b) is (√a : √b)
- Triplicate ratio of (a : b) is (a3 : b3)
- Sub - triplicate ratio of (a : b) is (a1/3: b1/3)
If a/b = c/d, then a + b/a - b = c + d/c - d [componendo and dividend]
What is a Proportion?
The equality of two ratios is called proportion i.e. If a/b = c/d, then a, b, c, d are said to be in proportion.
- If a: b = c : d, we write a: b:: c : d and saying that a, b, c, d are in proportion.
- Here a and d are called Extremes, while b and c are called Mean terms.
Product of means = Product of extremes
Thus, a : b :: c : d ⇔ (b x c) = (a x d)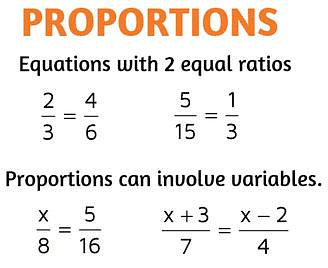
Important Properties of Proportion
If a:b = c:d is a proportion, then
- Product of extremes = product of means i.e., ad = bc
- a, b, c, d,…. are in continued proportion means, a:b = b:c = c:d
- a:b = b:c then b is called mean proportional and b2 = ac
- The third proportional of two numbers, a and b, is c, such that, a:b = b:c
- d is fourth proportional to numbers a, b, c if a:b = c:d
Difference Between Ratio and Proportion
To understand the concept of ratio and proportion, go through the difference between ratio and proportion given here.
| S.No | Ratio | Proportion |
| 1 | The ratio is used to compare the size of two things with the same unit | The proportion is used to express the relation of two ratios |
| 2 | It is expressed using a colon (:), slash (/) | It is expressed using the double colon (::) or equal to the symbol (=) |
| 3 | It is an expression | It is an equation |
| 4 | Keyword to identify ratio in a problem is “to every” | Keyword to identify proportion in a problem is “out of” |
Simple Method to Solve Ratio & Proportion Questions
The LCM process gets very cumbersome when we have to find the ratio out of multiple ratios.
We have the following simple method for that for a chain of ratios of any length.
Suppose you have the ratio train as follows
⇨ A : B = 1 : 2
⇨ B : C = 2 : 3
⇨ C : D = 5 : 6
⇨ D : E = 7 : 8
If we were to find A : B : C : D : E, then the LCM method would have taken quite a long time which is infeasible in examinations of limited hours.
The short cut is as follows:
A : B : C : D : E can be written directly as:
⇨ 1 × 2 × 5 × 7 : 2 × 2 × 5 × 7 : 2 × 3 × 5 × 7 : 2 × 3 × 6 × 7 : 2 × 3 × 6 × 8
⇨ 70 : 140 : 210 : 252 : 288
The thought algorithm for this case goes as:
To get the combined ratio of A : B : C : D : E, from A : B, B : C, C : D, and D : E
In the combined ratio of A : B : C : D : E.
- A will correspond to the product of all numerators (1 × 2 × 5 × 7).
- B will take the first denominator and the last 3 numerators (2 × 2 × 5 × 7).
- C, on the other hand, takes the first two denominators and the last 2 numerators (2 × 3 × 5 × 7).
- D takes the first 3 denominators and the last numerator (2 × 3 × 6 × 7) and E take all the four denominators (2 × 3 × 6 × 8).
Ratio and Proportion Tricks
- If u/v = x/y, then uy = vx
- If u/v = x/y, then u/x = v/y
- If u/v = x/y, then v/u = y/x
- If u/v = x/y, then (u+v)/v = (x+y)/y
- If u/v = x/y, then (u-v)/v = (x-y)/y
- If u/v = x/y, then (u+v)/ (u-v) = (x+y)/(x-y), which is known as Componendo-Dividendo Rule
- If a/(b+c) = b/(c+a) = c/(a+b) and a+b+ c ≠0, then a =b = c
Ratio and Proportion: Important Formulae
| Ratio Formula | a: b ⇒ a/b |
| Proportion Formula | a/b = c/d or a : b :: c : d |
| Fourth, Third and Mean Proportional | If a : b = c : d, then:
|
 1. Ratio:
1. Ratio:
- The ratio is nothing but the simplified form or comparison of two quantities of a similar kind. A ratio is a number, which indicates one quantity as the fraction of other quantity.
- Example: The Ratio of two numbers 5 to 6 is 5:6. It also expresses the no of times one quantity is equal to other quantity.
- “Terms” are the term that indicates numbers forming the ratios. The upper part of the ratio(numerator) is called antecedent and the lower part of the fraction(denominator) is called consequent or descendent.
Example: If the ratio is 4:6, then 4 is called the antecedent and 6 is called the consequent.
2. Proportion:
- Proportion is indicated as ‘::’ or ‘=’. If the ratio x:y is equal to the ratio of a:b, then x,y, a,b are in proportion.
- We mention it using the symbols is as x:y=a:b or x:y:: a:b
- When four terms are in proportion, then the product of two middle values(i.e., 2nd and 3rd values) must be equal to the product of two extremes(i.e., 1st and 4th values)
3. Fourth Proportional:
- If x:y = a:b, then b is called the fourth proportion to x,y and a
- Third Proportional: If x:y = a:b, then a is called the fourth proportion to x,y and b
- Mean Proportional: The mean proportional between x and y is the root(xy).
4. Comparision of Ratios
- We define that x:y>a:b if and only if x/y>a/b
- Compounded Ratios: Compounded Ratio of two ratios: (x:y), (a:b), (c:d) is (xac:ybd)
5. Duplicate Ratios
- The duplicated ratio of (x:y) is ((square(x):square(y)).
- The sub duplicated ratio of (x:y) is ((root(x):root(y)).
- Triplicate ratio of (x:y) is ((cube(x):cube(y)).
- The sub triplicate ratio of (x:y) is ((cuberoot(x):cuberoot(y)).
- Componendo and dividend rule
If x/y=a/b, then x=y/x-y = a+b/a-b.
6. Variations
- We define that a is directly proportional to b, if a=kb for some constant k and we write it as – a is proportional to b.
- We say that a is indirectly proportional to b, if ay=b for some constant k and we write it as – a is proportional to 1/b.
Solved Questions
Q1: In a library, the ratio of the number of story books to that of non-story books was 4:3 and total number of story books was 1248. When some more story books were bought, the ratio became 5:3. Find the number of story books bought.
Ans: Option A
Explanation:
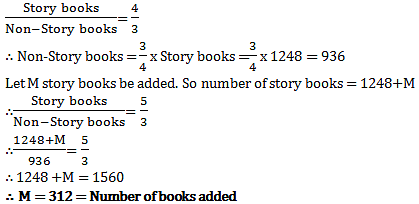
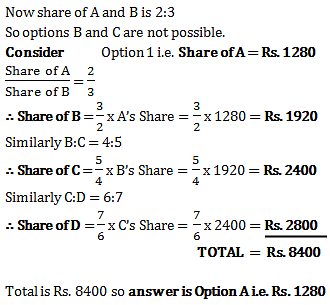
Q2: Rs. 8400 is divided among A, B, C and D in such a way that the shares of A and B, B and C, and C and D are in the ratios of 2:3, 4:5, and 6:7 respectively. The share of A is
Ans: Option A
Explanation:
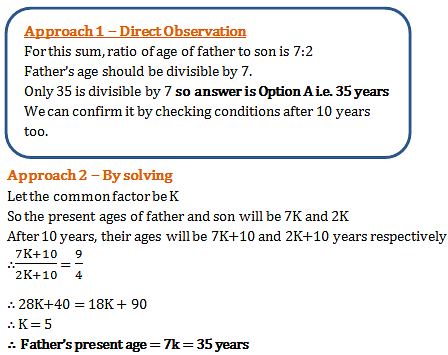
Q3: The ratio of the present age of father to that of son is 7:2. After 10 years their ages will be in the ratio of 9:4. The present ages of the father is
Ans: Option A
Explanation:
Q4: Ajay and Raj together have Rs. 1050. On taking Rs. 150 from Ajay, Ajay will have same amount as what Raj had earlier. Find the ratio of amounts with Ajay and Raj initially.
Ans: Option D
Explanation:

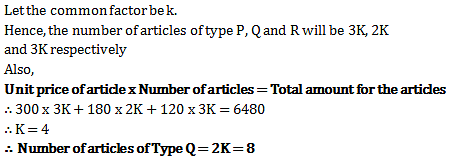
Q5: Price of each article of type P, Q, and R is Rs. 300, Rs. 180 and Rs. 120 respectively. Suresh buys articles of each type in the ratio 3:2:3 in Rs. 6480. How many articles of type Q did he purchase?
a. 8
Ans: Option A
Explanation:
Q6: Divide Rs. 60 in the ratio 1:2 between Mike and John.
Solution: Let Mike’s part be x.
Then John’s part is 2x.
Thus, x+2x = 60
3x = 60
x = (60/3)
x = 20.
Therefore, Mike’s part = x = Rs. 20
John’s part = 3x = Rs. (2*20) = Rs. 40
Q7: Three Jars contain alcohol to water in the ratios 3:5, 1:3, and 1:1. If all the three solutions are mixed, what will be the ratio of alcohol to water in final solution?
Solution: Here we are not given the quantities of the solution in three jars. Only the ratio of alcohol to water is given. If the ratio of the quantity of solution would have been there, we could determine the ratio of alcohol to water in the final solution. Hence, the answer here will be cannot be determined.
Q8: If there are Rs. 495 in a bag in denominations of one-rupee, 50-paisa, and 25-paisa coins which are in the ratio 1:8:16. How many 50 paisa coins are there in bag?
Solution: Assume, you have x numbers of one rupee coin. Now coins are in the ratio 1:8:16. This means that if we have x number of one rupee coins, we have 8x number of 50 paisa coins and 16x number of 25 paisa coins. Here order in which ratios are mentioned in the question is very important. In this case, order is one rupee, 50 paisa and 25 paisa and ratio is 1:8:16. Thus,
Number of 50-paisa coins = 8x
Number of 25- paisa coins = 16x
Now,Total money in the bag = Rs. 495
- x+ (8x/2) + (16x/4) = 495
(50 paisa coins divided by 2 to convert into rupee and 25 paisa coins divided by 4 to convert into rupee)
- 9x = 495
- x = 495/9
- x= 55
Thus, number of 50 paisa coins = 55*8 = 440
|
191 videos|131 docs|110 tests
|
FAQs on Important Formulas: Ratio and Proportion - Quantitative Aptitude (Quant) - CAT
| 1. What are the different types of ratios? |  |
| 2. How can I solve ratio and proportion questions easily? |  |
| 3. What is the difference between ratio and proportion? |  |
| 4. What are some important formulae related to ratio and proportion? |  |
| 5. Can you provide some tricks to remember ratios and proportions? |  |

|
Explore Courses for CAT exam
|

|