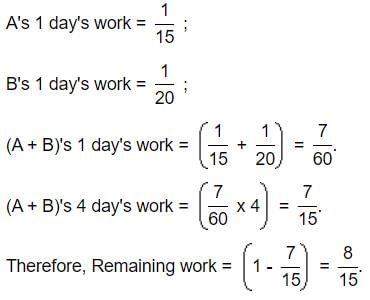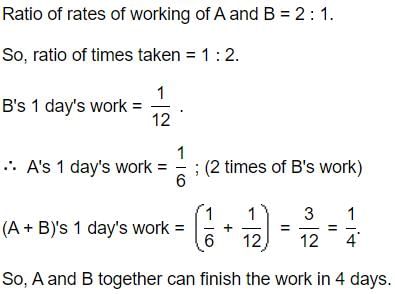Important Formulas & Tips: Time & Work | General Test Preparation for CUET UG - CUET Commerce PDF Download
| Table of contents |

|
| Formulas Used |

|
| Time and work Formula- Based on wages |

|
| Some Tips to Remember |

|
| Time and Work Tricks |

|
| Solved Questions: Time and Work |

|
Before we proceed with the questions and important formulas, it's crucial for candidates to have a good understanding of the concept and the types of questions that can be asked in the exam.
The concept of time and work revolves around how long it takes for an individual or a group of people to finish a task, as well as the effectiveness of their work. It involves understanding how efficiently each person contributes to the overall completion of the work.
Given below are the basic type of questions that may be asked in the exam with respect to the time and work topic:
- Calculating the efficiency of an individual.
- Determining the time required by an individual to complete a specific task.
- Estimating the time needed by a group of individuals to finish a particular job.
- Evaluating the amount of work accomplished by an individual within a given time period.
- Assessing the combined work achieved by a group of individuals within a specified time frame.
Formulas Used
Having knowledge of formulas can instantly connect you to a solution as soon as you encounter a question. Consequently, being familiar with the formula for any numerical ability topic simplifies both the solution process and the associated calculations.
Before you solve the questions, it is important that you are well aware of the basic concept, standard formulas, and types of questions for the same.
- Work Done = Time Taken × Rate of Work
- Rate of Work = 1 / Time Taken
- Time Taken = 1 / Rate of Work
- If A can do a piece of work in n days, work done by A in 1 day = 1/n
If A does 1/n work in a day, A can finish the work in n days. - Total Work Done = Number of Days × Efficiency
- Efficiency and Time are inversely proportional to each other
- A does a particular job in ‘a’ hours and B does the same job in ‘b’ hours, together they will take:
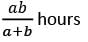
- A does a particular job in ‘a’ hours more than A and B combined whereas B does the same job in ‘b’ hours more than A and B combined, then together they will take
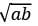 hours to finish the job.
hours to finish the job. - Total Work Done = Number of Days × Efficiency
- x:y is the ratio of the number of men who are required to complete a piece of work, then the ratio of the time taken by them to complete the work will be y:x
- If M1 men can do W1 work in D1 days with T1 working hours/day and M2 men can do W2 work in D2 days with T2 working hours/day, then:
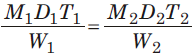
Time and work Formula- Based on wages
1) Total Wage = Total number of days × Wage of a person’s daily wage
2) Wage is directly proportional to the amount of work performed and
3)Wage is directly proportional to the number of hours each person works in a day.
4) Wage is inversely related to the amount of time spent by the employee.
5)If A and B can complete a task in x and y days, respectively, their salaries will be paid out in a y:x ratio. Consequently, A and B’s salaries will be
Total wages y/(x + y) = Wage of A.
Total wages x/(x + y) = Wage of B
Some Tips to Remember
- A does a particular job in ‘a’ hours, B does the same job in ‘b’ hours and C does the same job in ‘c’ hours, then together they will take (abc/ab+bc+ca) hours.
- If A does a particular job in ‘a’ hours and A&B together do the job in ‘t’ hours, the B alone will take (at/a - t) hours.
- If A does a particular job in ‘a’ hours, B does the same job in ‘b’ hours and ABC together do the job in ‘t’ hours, then
⇒ C alone can do it in (abt/ab - at - bt) hours
⇒ A and C together can do it in (bt/b - t) hours
⇒ B and C together can do it in (at/a - t) hours - If the objective is to fill the tank, then the Inlet pipes do positive work whereas the Outlet pipes do negative work.
If the objective is to empty the tank, then the Outlet pipes do positive work whereas the Inlet Pipes do negative work.
Time and Work Tricks
Apart from the basic formulas, Let’s learn some short tricks to solve the problems more quickly based on Time and work.
- If A can do can work in ‘n’ days, Then The efficiency of A is “1/n”
- If individuals can do W1 work in D1 days while putting in T1 hours per day, and M individuals can complete W2 work in D2 days while putting in T2 hours per day, then the relationship between them is If A and B can each complete a piece of work in days, then (A + Bone )’s day work-
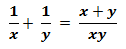
- Time required by (A + B) to finish the task –
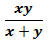
- If there are ‘n’ people (more than two), then their work in one day is equal to where x1, x2, and x3 reflect the number of days it took them to finish the task.
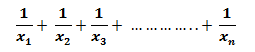
- If A takes days longer than (A + B) to complete a task and B takes y days longer than (A + B) to do the same task, then (A + B) finishes the task in √xy days.
- If A & B can perform a task in x days and A can do so on their own in y days, then the number of days needed by B to finish the task is
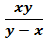
- If A and B can complete a task in x days, B and C can complete the same task in y days, and A and C can complete it in z days, then A, B, and C working together can complete that task in-
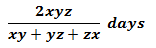
- If A can accomplish a task in days and B can do it more quickly than A, then B will finish the task in
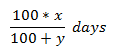
Let's solve some questions on time and work.
Solved Questions: Time and Work
Q 1. A builder appoints three construction workers Akash, Sunil and Rakesh to one of his sites. They take 20, 30 and 60 days respectively to do a piece of work. How many days will it take Akash to complete the entire work if he is assisted by Sunil and Rakesh every third day?
- 10 days
- 15 days
- 25 days
- 30 days
- 45 days
Solution:
Answer: (2) 15 days
Total work done by Akash, Sunil and Rakesh in 1 day = {(1/20) + (1/30) + (1/60)} = 1/10
Work done along by Akash in 2 days = (1/20) × 2 = 1/10
Work Done in 3 days (1 day of all three together + 2 days of Akash’s work) = (1/10) + (1/10) = 1/5
So, work done in 3 days = 1/5
Time taken to complete the work = 5×3 = 15 days
Q 2. To complete a piece of work, Samir takes 6 days and Tanvir takes 8 days alone respectively. Samir and Tanvir took Rs.2400 to do this work. When Amir joined them, the work was done in 3 days. What amount was paid to Amir?
- Rs. 300
- Rs. 400
- Rs. 800
- Rs. 500
- Rs. 100
Solution:
Answer: (1) Rs.300
Total work done by Samir and Tanvir = {(1/6) + (1/8)} = 7/24
Work done by Amir in 1 day = (1/3) – (7/24) = 1/24
Amount distributed between each of them = (1/6) : (1/8) : (1/24) = 4:3:1
Amount paid to Amir = (1/24) × 3 × 2400 = Rs.300
Q 3. Dev completed the school project in 20 days. How many days will Arun take to complete the same work if he is 25% more efficient than Dev?
- 10 days
- 12 days
- 16 days
- 15 days
- 5 days
Solution:
Answer: (3) 16 days
Let the days taken by Arun to complete the work be x
The ratio of time taken by Arun and Dev = 125:100 = 5:4
5:4 :: 20:x
⇒ x = {(4×20) / 5}
⇒ x = 16
Q 4. Time taken by A to finish a piece of work is twice the time taken B and thrice the time taken by C. If all three of them work together, it takes them 2 days to complete the entire work. How much time would B take alone to finish this work?
- 2 days
- 6 days
- 3 days
- 5 days
- Cannot be determined
Solution:
Answer: (2) 6 days
Time taken by A = x days
Time taken by B = x/2 days
Time Taken by C = x/3 days
⇒ {(1/x) + (2/x) + (3/x) = 1/2
⇒ 6/x = 1/2
⇒ x = 12
Time taken by B = x/2 = 12/2 = 6 days
Q 5. Sonal and Preeti started working on a project and they can complete the project in 30 days. Sonal worked for 16 days and Preeti completed the remaining work in 44 days. How many days would Preeti have taken to complete the entire project all by herself?
- 20 days
- 25 days
- 55 days
- 46 days
- 60 days
Solution:
Answer: (5) 60 days
Let the work done by Sonal in 1 day be x
Let the work done by Preeti in 1 day be y
Then, x+y = 1/30 ——— (1)
⇒ 16x + 44y = 1 ——— (2)
Solving equation (1) and (2),
x = 1/60
y = 1/60
Thus, Preeti can complete the entire work in 60 days.
Q 6: It takes 6 hours for pump A, used alone, to fill a tank of water. Pump B used alone takes 8 hours to fill the same tank. We want to use three pumps: A, B and another pump C to fill the tank in 2 hours. What should be the rate of pump C? How long would it take pump C, used alone, to fill the tank?
Solution:
Let the total capacity of the tank be C liters.
Fill rate of pump A, Fa = C/6 liters per hr
Fill rate of pump B, Fb = C/8 liters per hr
2 hrs x [Fa + Fb + Fc] = C => Fc = 5C/24 liters per hr
Let‘t’ be the time taken by only pump C to fill the tank.
‘t’ hrs x 5C/24 = C => t = 24/5 = 4.8 hrs
Q 7: A alone can do a piece of work in 6 days and B alone in 8 days. A and B undertook to do it for Rs.3200. With the help of C, they completed the work in 3 days. How much is to be paid to C?
Solution:
Let the total amount of work to be done be W units.
Productivity of A, Pa = W/6 units per day.
Productivity of B, Pb = W/8 units per day.
3 days x [Pa + Pb + Pc] = W => Pc = W/24 units per day
Ratio of wages of A: B: C = Ratios of their productivities = (W/6): (W/8): (W/24) = 4: 3: 1.
Amount to be paid to C = Rs.3200 x (1/8) = Rs.400
Q 8: A and B can do a work in 3 days; B & C can do it in 4 days and A & C can do it in 6 days. In how many days will A, B & C finish it, if they work together?
Solution:
LCM of 3, 4, and 6 = 12 (Total work)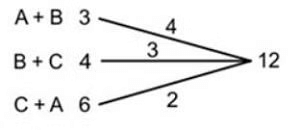
Efficiency of 2(A + B + C) = 9
Efficiency of (A + B + C) = 4.5
Time = 12 / (9/2) = 8/3 days
Q9: P is twice as good as Q and together they finish a piece of work in 36 days. The number of days taken by P alone to finish the work?
Solution: Given,
P is twice as good as Q.
⇒ (P’s 1 day’s work) / (Q’s 1 day’s work) = 2 / 1 Given,
⇒ (P + Q)’s 1 day’s work = 1/36
⇒ P’s 1 day’s work = (1/36) × (2/3) = 1/54
∴ P alone can finish work in 54 days.
Q 10: A and B can complete a piece of work in 15 days and 10 days respectively. They got a contract to complete the work for Rs. 75000. The share of B (in Rs.) in the contracted money will be:
Solution: Ratio of number of days taken by A and B to complete the work = 15 : 10 = 3 : 2
∴ Ratio of efficiency of A and B = 2 : 3 Let their share is in the ratio of 2x and 3x Now,
2x + 3x = 75000
⇒ 5x = 75000
∴ x = 15000
∴ Share of B = 3x = 15000 × 3 = RS. 45000
|
164 videos|626 docs|1130 tests
|
FAQs on Important Formulas & Tips: Time & Work - General Test Preparation for CUET UG - CUET Commerce
| 1. How do you calculate time and work based on wages? |  |
| 2. What are some tips to remember when solving time and work problems? |  |
| 3. What are some common time and work tricks to solve problems efficiently? |  |
| 4. How can one apply the time and work formula in real-life scenarios? |  |
| 5. How can one improve their understanding and proficiency in solving time and work problems? |  |