Important Properties of Conjugate, Modulus & Argument | Mathematics (Maths) Class 11 - Commerce PDF Download
D. Important Properties Of Conjugate / Modulus / Argument
If z , z1 , z2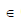 C then ;
C then ;
(a) 



(b)
|z| ≥ 0 ; |z| ≥ Re (z) ; |z| ≥ Im (z) ;




(c)
(i) amp (z1 . z2) = amp z1 + amp z2 + 2 kπ . k ∈I
(ii) amp = amp z1 - amp z2 + 2 kπ ; k∈ I\
(iii) amp(zn) = n amp(z) + 2kπ .
where proper value of k must be chosen so that RHS lies in (- π , π ].
Ex.4 The maximum & minimum values of 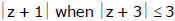 are
are
Sol. 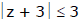 denotes set of points on or inside a circle with centre (- 3, 0) and radius 3.
denotes set of points on or inside a circle with centre (- 3, 0) and radius 3.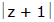 denotes the distance of P from A
denotes the distance of P from A 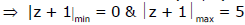
Ex.5 Let z1 , z2 be two complex numbers represented by points on the circle  respectively , then
respectively , then
Sol.
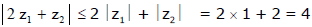
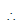 Maximum value of
Maximum value of 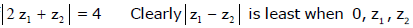 are collinear.
are collinear.


Ex.6 Prove that if z1 and z2 are two complex numbers and c > 0, then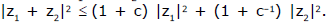
Sol.
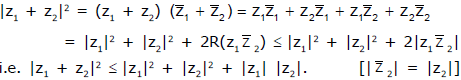
Incorporating the number c > 0, the last term on the RHS can be written




Ex.7 If 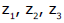 are the points A, B, C in the Argand Plane such that,
are the points A, B, C in the Argand Plane such that,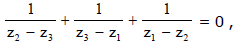 prove that ABC is an equilateral triangle .
prove that ABC is an equilateral triangle .
Sol . Let z2 - z3 = p ; z3 - z1 = q ; z1 - z2 = r ⇒ p + q + r = 0
Given condition, pq + qr + rp = 0 ⇒ p (q + r) + qr = 0 ⇒ p (- p) + qr = 0
⇒ p2 = qr





Ex.8 If z & w are two complex numbers simultaneously satisfying the equations, 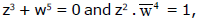 then
then
Sol.
z3 = - w5 ⇒ |z| 3 =|w|5 ⇒ |z| 6 = |w|10 .....(1)





Ex.9 The complex numbers whose real and imaginary parts are integers and satisfy the relation 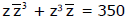 forms a rectangle on the Argand plane, the length of whose diagonal is
forms a rectangle on the Argand plane, the length of whose diagonal is
Sol.
 = 2 (x2 + y2) (x2 - y2) = 350 ⇒ (x2 - y2) (x2 + y2) = 175 = 35.5 = 25.7
= 2 (x2 + y2) (x2 - y2) = 350 ⇒ (x2 - y2) (x2 + y2) = 175 = 35.5 = 25.7
= x2 + y2 = 25 & x2 - y2 = 7 ⇒ x = ± 4 & y = ± 3
Ex.10 Find the area bounded by the curve, arg z 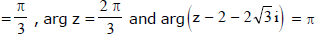 in the complex plane .
in the complex plane .
Sol.
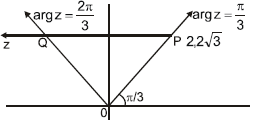
required area, the equilateral triangle OPQ with side 4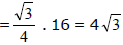
Ex.11
Find the complex number where the curves arg 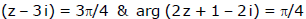 intersect.
intersect.
Sol.




Ex.12 if 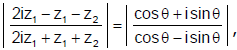 then prove that
then prove that 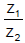 is purely real.
is purely real.
Sol. The given relation can be written as 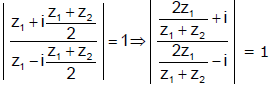




Ex.13 For every real number a ≥ 0 find all the complex numbers z that satisfy the equation 2|z| – 4 az + 1 + ia = 0.
Sol. We have 2|z| – 4 az + 1 + ia = 0
Put z = x + i y,
We get,  = 4 ax – 1 + 4aiy – ia or 4(x2 + y2) = (4 ax – 1)2 .....(1)
= 4 ax – 1 + 4aiy – ia or 4(x2 + y2) = (4 ax – 1)2 .....(1)
and a = 4 ay (by separating imaginary and real parts)
⇒ y =1/2 and 4x2 + 1/4– 16 a2 x2 – 1 + 8 ax = 0 ⇒ x2 (16 - 64 a2) + 32 ax - 3 = 0












|
75 videos|238 docs|91 tests
|
FAQs on Important Properties of Conjugate, Modulus & Argument - Mathematics (Maths) Class 11 - Commerce
| 1. What is the definition of the conjugate of a complex number? |  |
| 2. How do you find the modulus of a complex number? |  |
| 3. What does the argument of a complex number represent? |  |
| 4. How can you find the argument of a complex number? |  |
| 5. What are some important properties of the conjugate, modulus, and argument of complex numbers? |  |

|
Explore Courses for Commerce exam
|

|


















