Integral Calculus and Differential Equations | Mathematics for NDA PDF Download
List of Integral Formulas
The list of basic integral formulas is given below:
∫ 1 dx = x + C
∫ a dx = ax+ C
∫ xn dx = ((xn+1)/(n+1))+C ; n≠1
∫ sin x dx = – cos x + C
∫ cos x dx = sin x + C
∫ sec 2x dx = tan x + C
∫ csc 2x dx = -cot x + C
∫ sec x (tan x) dx = sec x + C
∫ csc x ( cot x) dx = – csc x + C
∫ (1/x) dx = ln |x| + C
∫ ex dx = ex + C
∫ ax dx = (ax/ln a) + C ; a>0, a≠1
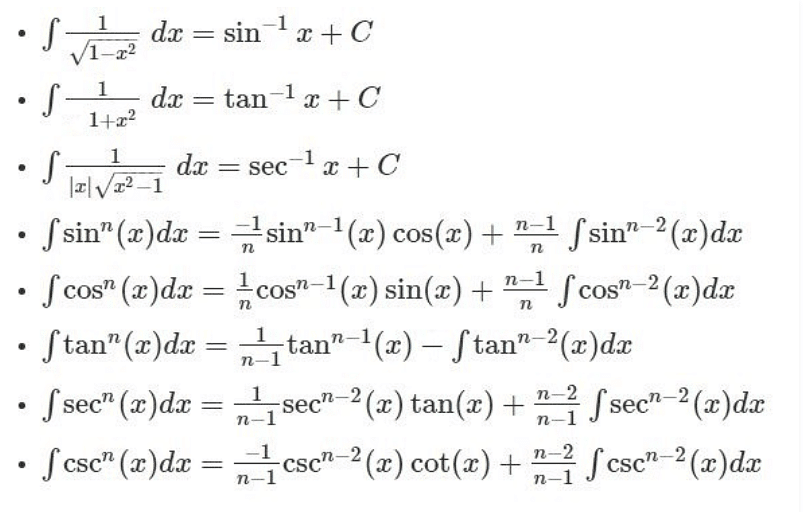
Integral Formulas for Different Functions
Let’s learn all the integration formulas for different functions now.
Rational functions
Below are the integration formulas for rational functions.
∫ 1 dx = x + C
∫ a dx = ax+ C
∫ (1/x) dx = ln |x| + C
Irrational functions
Let’s see the integration formulas for irrational functions.
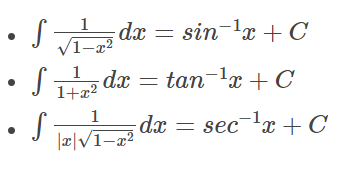
Trigonometric functions
Integration formulas for trigonometric functions are listed below:
∫ sin x dx = – cos x + C
∫ cos x dx = sin x + C
∫ sec 2x dx = tan x + C
∫ csc 2x dx = -cot x + C
∫ sec x (tan x) dx = sec x + C
∫ csc x ( cot x) dx = – csc x + C
Inverse Trigonometric Functions
Go through the integration formulas for inverse trigonometric functions here.

Hyperbolic functions
Below is the list of integration formulas for hyperbolic functions in maths.
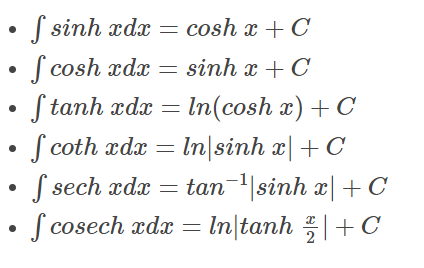
Exponential functions
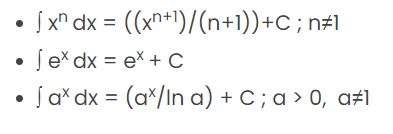
Logarithmic functions
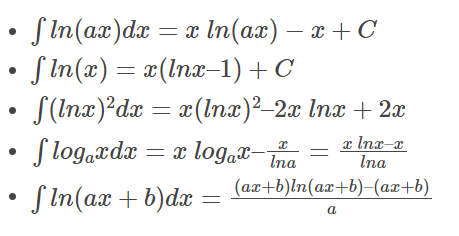
Integral Formulas for Some Special Functions
As we have already gone through integral formulas for exponential functions, logarithmic functions, trigonometric functions and some basic functions. Let’s have a look at the additional integration formulas, i.e. the integral formulas for some special functions listed below
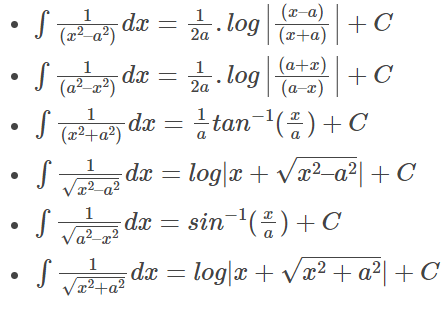
|
277 videos|265 docs|221 tests
|

|
Explore Courses for NDA exam
|

|

















