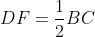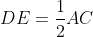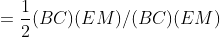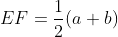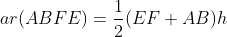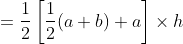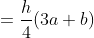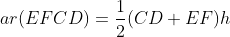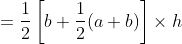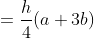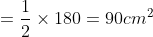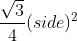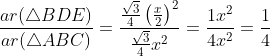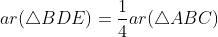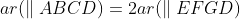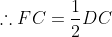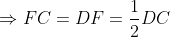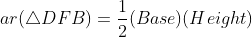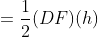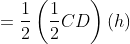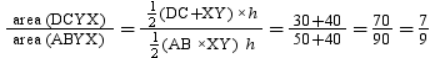NCERT Exemplar Solutions: Area of Parallelograms & Triangles - Class 9 PDF Download
Exercise 9.1
Write the correct answer in each of the following:
Q.1. The median of a triangle divides it into two
(a) triangles of equal area
(b) congruent triangles
(c) right triangles
(d) isosceles triangles
Correct Answer is Option (a)
We know that, a median of a triangle is a line segment joining a vertex to the mid-point of the opposite side. Thus, a median of a triangle divides it into two triangles of equal area.
Q.2. In which of the following figures (Fig. 9.3), you find two polygons on the same base and between the same parallels?
(a) 
(b) 
(c) 
(d) 
Correct Answer is Option (d)
In figures (a), (b) and (c) there are two polygons on the same base but they are not between the same parallels.
In figure (d), there are two polygons (PQRA and BQRS) on the same base and between the same parallels.
Q.3. The figure obtained by joining the mid-points of the adjacent sides of a rectangle of sides 8 cm and 6 cm is:
(a) a rectangle of area 24 cm2
(b) a square of area 25 cm2
(c) a trapezium of area 24 cm2
(d) a rhombus of area 24 cm2
Correct Answer is Option (d)
According to the question,
Let ABCD be the rectangle.
E is the mid-point of the side AB
F is the mid-point of the side BC
G is the mid-point of the side CD
H is the mid-point of the side DA
The figure obtained by joining the midpoints E, F, G and H is rhombus.
Sides of the rectangle is given,
We know that,
Side of the rectangle AB = diagonal of the rhombus FH = 8cm
Similarly,
Side of the rectangle AD = diagonal of the rhombus EG = 6cm
Then,
Area of the rhombus = ½ × EG × FH
= ½ × 6 × 8
= 24 cm2
Q.4. In Fig. 9.4, the area of parallelogram ABCD is: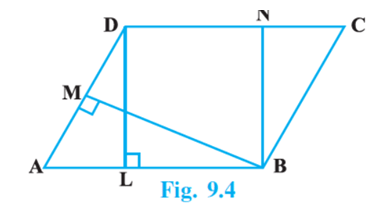 (a) AB × BM
(a) AB × BM
(b) BC × BN
(c) DC × DL
(d) AD × DL
Correct Answer is Option (c)
We know that, area of parallelogram is the product of its any side and the corresponding altitude (or height).
Here, when AB is base, then height is DL.
Area of parallelogram = AB x DL and when AD is base, then height is BM.
Area of parallelogram = AD x BM When DC is base, then height is DL.
Area of parallelogram = DC x DL and when BC is base, then height is not given.
Q.5. In Fig. 9.5, if parallelogram ABCD and rectangle ABEF are of equal area, then: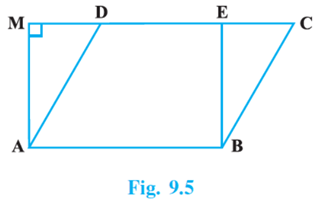 (a) Perimeter of ABCD = Perimeter of ABEM
(a) Perimeter of ABCD = Perimeter of ABEM
(b) Perimeter of ABCD < Perimeter of ABEM
(c) Perimeter of ABCD > Perimeter of ABEM
(d) Perimeter of ABCD = ½ (Perimeter of ABEM)
Correct Answer is Option (c)
In rectangle ABEM,
AB = EM …(eq.1) [sides of rectangle]
In parallelogram ABCD,
CD = AB …(eq.2)
Adding, equations (1) and (2),
We get
AB + CD = EM + AB …(i)
We know that,
Perpendicular distance between two parallel sides of a parallelogram is always less than the length of the other parallel sides.
BE < BC and AM < AD
[because, in a right angled triangle, the hypotenuse is greater than the other side]
On adding both above inequalities, we get
SE + AM <BC + AD or BC + AD> BE + AM
On adding AB + CD both sides, we get
AB + CD + BC + AD> AB + CD + BE + AM
⇒ AB+BC + CD + AD> AB+BE + EM+ AM [∴ CD = AB = EM]
Hence,
We get,
Perimeter of parallelogram ABCD > perimeter of rectangle ABEM
Q.6. The mid-point of the sides of a triangle along with any of the vertices as the fourth point make a parallelogram of area equal to
(a) 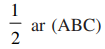
(b) 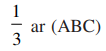
(c) 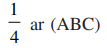
(d) ar (ABC)
Correct Answer is Option (a)
Let the given triangle ABC be shown as below with medians AE, BF, CD.
D is the mid-point of AB, E is the mid-point of BC, F is the mid-point of AC
Mid-point theorem states that the line segment in a triangle joining the midpoint of two sides of the triangle is said to be parallel to its third side and is also half of the length of the third side
So,
DF = BE = EC …(i)
DE = AF = FC …(ii)FE = AD = DB …(iii)
In Δ ADF and Δ FEC
AD = FE (from iii)
DF = EC (from i)
AF = FC (given)
Δ ADF ≅ Δ FEC (SSS congruence)
Similarly, we can prove that,
Δ ADF ≅ Δ FEC ≅ ΔDBE ≅ Δ EFD
So the bigger triangle is divided into 4 smaller congruent triangles.
So area of Δ ABC = 4 (area of one smaller triangle) …(iv)
If we consider the parallelograms formed then we can see that one ║gm consists of two such triangles.
║BDFE = Δ DBE + Δ EFD
║DFCE = Δ FEC + Δ EFD
║ADEF = Δ ADF + Δ EFD
So area of every parallelogram = 2 (area of one smaller triangle) …(v)
Using (iv) and (v),
area of every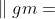
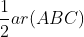
Q.7. Two parallelograms are on equal bases and between the same parallels. The ratio of their areas is
(a) 1 : 2
(b) 1 : 1
(c) 2 : 1
(d) 3 : 1
Correct Answer is Option (b)
Area of a parallelogram is given as the product of its base and corresponding altitude.
Altitude is the perpendicular distance between the two parallel sides.
Now, parallelogram on the same or equal bases and between the same parallels will have the same area. This is because the base is the same, and also as they are between the same bases so the altitude will be the same for them.
So, the ratio of their area is 1 : 1.
Q.8. ABCD is a quadrilateral whose diagonal AC divides it into two parts, equal in area, then ABCD
(a) is a rectangle
(b) is always a rhombus
(c) is a parallelogram
(d) need not be any of (A), (B) or (C)
Correct Answer is Option (d)
Here, ABCD need not be any of rectangle, rhombus and parallelogram because if ABCD is a square, then its diagonal AC also divides it into two parts which are equal in area.
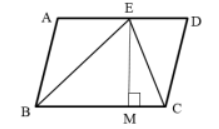
Q.9. If a triangle and a parallelogram are on the same base and between same parallels, then the ratio of the area of the triangle to the area of parallelogram is
(a) 1 : 3
(b) 1 : 2
(c) 3 : 1
(d) 1 : 4
Correct Answer is Option (b)
We know that a triangles and a parallelogram are on the same base and between the same parallels, then the area of the triangle is equal to half the area of the parallelogram. Let us prove the same. We have a parallelogram ABCD and triangle BEC on the same base BC.
Construct EM perpendicular to BC
Area of a triangle is given as: 1/2 (Base) (Height)
Area of Δ BEC = 1/2 (BC) (EM) …(i)
Area of a parallelogram is given as: (Base) (corresponding altitude)
Area of a parallelogram ABCD= (BC) (EM) …(ii)
Ratio of the area of the triangle to the area of parallelogram = (i) : (ii)
= 1/2
Hence the ratio of the area of the triangle to the area of parallelogram is 1 : 2.
Q.10. ABCD is a trapezium with parallel sides AB = a cm and DC = b cm (Fig. 9.6). E and F are the mid-points of the non-parallel sides. The ratio of ar (ABFE) and ar (EFCD) is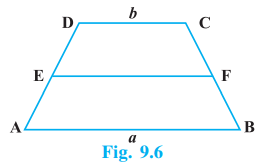 (a) a : b
(a) a : b
(b) (3a + b) : (a + 3b)
(c) (a + 3b) : (3a + b)
(d) (2a + b) : (3a + b)
Correct Answer is Option (b)
Given: ABCD is a trapezium in which AB ║ DC,,
E and F are the mid-points of AD and BC, respectively
(Mid-segment Theorem on Trapezium: the line segment joining the midpoints of the nonparallel sides of a trapezium is half the sum of the lengths of the parallel sides and is also parallel to them.)
So,
Now, ABFE and EFCD are also trapeziums.
Required ratio
So, the required ratio is (3a + b) : (a + 3b)
Exercise 9.2
Write True or False and justify your answer:
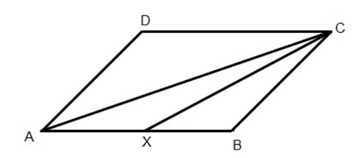
Q.1. ABCD is a parallelogram and X is the mid-point of AB. If ar (AXCD) = 24 cm2, then ar (ABC) = 24 cm2.
False
In ΔABC, X is midpoint of AB
Considering the given question,
Hence ar(ΔAXC) = ar(ΔBXC) = ½ ar(ΔABC)
Therefore,
ar(ΔAXC) = ar(ΔBXC) = 12 cm2
Area of ||gm ABCD = 2 x ar(ΔABC)
= 2 x 24 = 48 cm2
But, according to the question,
area of ||gm ABCD = ar(AXCD) + ar(ΔBXC)
= 24 + 12 = 36 cm2
Hence, we find a contradiction here.
So, If ar (AXCD) = 24 cm2, then ar (ABC) ≠ 24 cm2
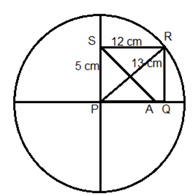
Q.2. PQRS is a rectangle inscribed in a quadrant of a circle of radius 13 cm. A is any point on PQ. If PS = 5 cm, then ar (PAS) = 30 cm2.
True
Since A is any point on PQ, then ar (PAS) ≠ 30 cm2
But, the statement can be true if PA is equal to PS.
Justification:
According to the question,
PQRS is a rectangle inscribed in a quadrant of a circle of radius 13 cm.
Given,
A is any point on PQ
PA < PQ
ar (△PQR) = ½ × PQ × QR
= ½ × 12 × 5
= 30cm2
PS = 5 cm
Suppose PA < PQ,
ar(△PAS) < ar(△PQR)
ar(△PAS) < 30 cm2
Suppose PA = PQ,
ar (△PAS) = ½ × PQ × PS
= ½ × 12 × 5
= 30cm2
Q.3. PQRS is a parallelogram whose area is 180 cm2 and A is any point on the diagonal QS. The area of ∆ ASR = 90 cm2.
False
Given, PQRS is a parallelogram whose area is 180 cm2
We know that diagonal of a parallelogram divides it into two triangles of equals area, so
Given that A is any point on SQ
So, ar (ΔASR) < ar(ΔQRS)
Hence, ar (ΔASR) < 90 cm2
But given that area of ΔASR = 90 cm2
Hence, the given statement is false.
Q.4. ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then ar (BDE) = 1/4 ar ( ABC).
True
Given ΔABC and ΔBDE are two equilateral triangles.
D is the mid-point of BC, so BD = CD =BC
Let each sides of ΔABC be x.
So each side of ΔBDE is x/2.
Area of an equilateral triangle is given as
Now,
Hence,
Therefore the given statement is true.
Q.5. In Fig. 9.8, ABCD and EFGD are two parallelograms and G is the mid-point of CD. Then ar (DPC) = 1/2 ar ( EFGD)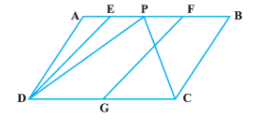
False
Given that ABCD and EFGD are two parallelogram and G is mid-point of CD.
Let perpendicular distance between AB and CD be h
ar (║gm) = (base) (corresponding altitude)
ar (║EFGD) = (GD) (h)
ar (║ABCD) = (CD) (h)
CD = 2 GD (Given that G is mid-point of CD)
So,
∴....(i)
Now, DPDC and parallelogram ABCD are on the same base BC and between the same parallels AB and CD
So,…(ii)
From (i) and (ii)
⇒ ar (ΔDPC) = ar(║EFGD)
Therefore the given statement is false.
Exercise 9.3
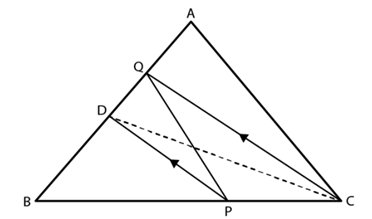
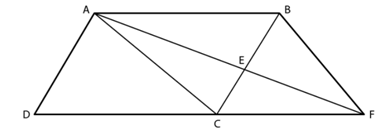
Q.1. In Fig.9.11, PSDA is a parallelogram. Points Q and R are taken on PS such that PQ = QR = RS and PA || QB || RC. Prove that ar (PQE) = ar (CFD).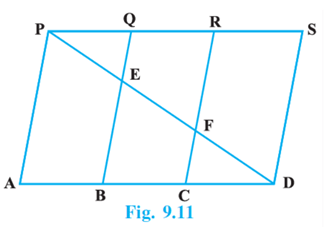
According to the question,
PA║QB║RC║SD & PQ = QR = RS
According to the Equal intercept theorem,
We know that if three or more parallel lines make equal intercept on traversal, then they make equal intercept on any other form of traversal.
Hence, we get,
PE = EF = FD & AB = BC = CD
From ΔPQE & ΔDCF,
We get,
∠PEQ = ∠DFC
PE = DF
∠QPE = ∠CDF
So,
ΔPQE ≅ ΔDCF
Since Congruent figures have equal areas,
We get,
ar ΔPQE = ar ΔDCF
Hence proved.
Q.2. X and Y are points on the side LN of the triangle LMN such that LX = XY = YN. Through X, a line is drawn parallel to LM to meet MN at Z (See Fig. 9.12). Prove that ar (LZY) = ar (MZYX)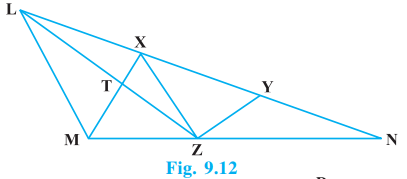
Given X and Y are points on the side LN such that
LX = XY = YN and XZ || LM To prove ar (ΔLZY) = ar (MZYX)
Proof Since, ΔXMZ and ΔXLZ are on the same base XZ and between the same parallel lines LM and XZ.
Then, ar (ΔXMZ) = ar (ΔXLZ) …(i)
On adding ar (ΔXYZ) both sides of Eq. (i), we get
ar (ΔXMZ) + ar (ΔXXZ) = ar (ΔXLZ) + ar (ΔXYZ)
=> ar (MZYX) = ar (ΔLZY) Hence proved.
Q.3. The area of the parallelogram ABCD is 90 cm2 (see Fig.9.13). Find
(i) ar (ABEF)
(ii) ar (ABD)
(iii) ar (BEF)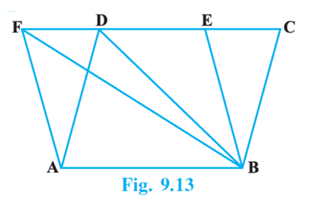
According to the question,
Area of parallelogram, ABCD = 90 cm2
(i) We know that,
Parallelograms on the same base and between the same parallel are equal in areas.
Here,
The parallelograms ABCD and ABEF are on same base AB and between the same parallels AB and CF.
Therefore, ar (ABEF) = ar (ABCD) = 90 cm2
(ii) We know that,
If a triangle and a parallelogram are on the same base and between the same parallels, then area of triangle is equal to half of the area of the parallelogram.
Here,
ΔABD and parallelogram ABCD are on the same base AB and between the same parallels AB and CD.
Therefore, ar (ΔABD) = ½ ar (ABCD)
= ½ x 90 = 45 cm2
(iii) We know that,
If a triangle and a parallelogram are on the same base and between the same parallels, then area of triangle is equal to half of the area of the parallelogram.
Here,
ΔBEF and parallelogram ABEF are on the same base EF and between the same parallels AB and EF.
Therefore, ar (ΔBEF) = ½ ar (ABEF)
= ½ x 90 = 45 cm2
Q.4. In ∆ ABC, D is the mid-point of AB and P is any point on BC. If CQ || PD meets AB in Q (Fig. 9.14), then prove that ar (BPQ) = 1/2 ar (ABC).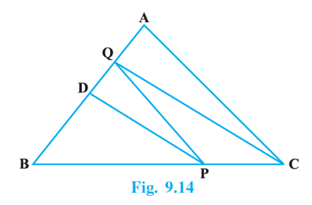
According to the question,
We have the figure,
In △ ABC,
D is the mid-point of AB
P is any point on BC.
If CQ || PD meets AB at Q
To prove: ar (BPQ) = ½ ar (ABC)
Construction: Join DC
Proof: Since D is the mid-point of AB. so, in △ ABC, CD is the median.
ar(△ BCD) = ½ ar (△ ABC) ….. (1)
We know that,
△ PDQ and △ PDC are on the same base PD and between the same parallels lines PD QC.
Therefore, ar(△ PDQ) = ar(△ PDC) ……………. (2)
From (1) and (2)
ar(△ BCD) = ½ ar(△ ABC)
ar(△ BCD = ar(△ BPD) + ar(△ PDC)
= ½ ar(△ ABC)
Area of △ PDC = PDQ
So,
ar(△ BPQ) = ar(△ BPD) + ar(△ PDQ)
= ½ ar(△ ABC)
Therefore, ar(△BPQ) = ½ ar(△ ABC)
Hence Proved
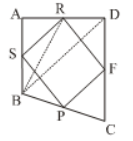
Q.5. ABCD is a square. E and F are respectively the midpoints of BC and CD. If R is the mid-point of EF (Fig. 9.15), prove that ar (AER) = ar (AFR)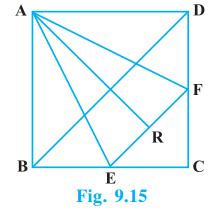
Given that ABCD is a square
E and F are the midpoints of BC and CD
R is the mid-point of EF
Proof: Consider ΔABE and ΔADF
We know that BC = DC (All sides of square are equal)
⇒ BC/2 = DC/2
⇒ EB = DF (Since E and F are mid points of BC and CD)
∠ABE = ∠ADF = 90º (All angles in a square are right angle)
⇒ AB = AD (All sides in a square are equal)
∴ ΔABE ≅ ΔADF (By SAS criterion)
Hence, AE = AF (by C.P.C.T)
Now, ER = RF ( R is the midpoint of EF)
AR = AR (Common)
∴ ΔAER ≅ ΔAFR (By SSS criterion)
Hence, AR divides the triangle into two triangles of equal area.
⇒ ar (ΔAER) = ar (ΔAFR) Hence proved.
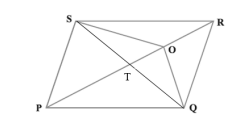
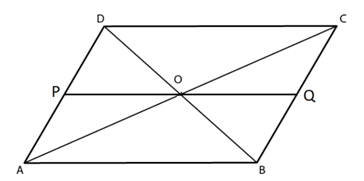
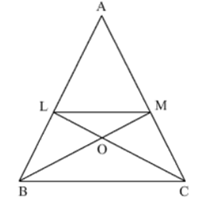
Q.6. O is any point on the diagonal PR of a parallelogram PQRS (Fig. 9.16). Prove that ar (PSO) = ar (PQO).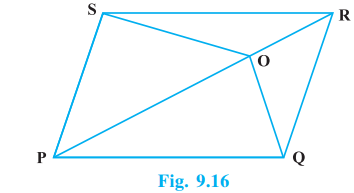
Given: O is any point on the diagonal PR of a parallelogram PQRS
Construction: Join QS
Let diagonals PR and QS intersect each other at T.
We know that diagonals of a parallelogram bisect each other.
∴ T is the mid-point of QS and PR
Since a median of a triangle divides it into two triangle of equal area.
∴ In ΔPQS, PT is the median of side QS
⇒ ar(ΔPTS) = ar(ΔPTQ) …(i)
In ΔSOQ, OT is the median of side QS
⇒ ar(ΔSTO) = ar(ΔQTO) …(ii)
Adding (i) and (ii), we have
ar(ΔPTS) + ar(ΔSTO) = ar(ΔPTQ) + (ΔQTO)
⇒ ar(ΔPSO) = ar(ΔPQO)
Hence proved
Q.7. ABCD is a parallelogram in which BC is produced to E such that CE = BC (Fig. 9.17). AE intersects CD at F. If ar (DFB) = 3 cm2, find the area of the parallelogram ABCD.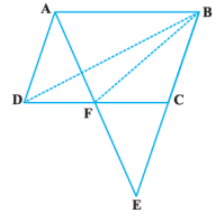
Given: ABCD is a parallelogram.
CE = BC
The lines BC and AF when produced, meet at E.
ar ΔDFB = 3cm2
Now,
BC = EC & AB ║ FC (given)(By mid-point theorem)
Also, AB = DC (opposite sides of a parallelogram are equal)
Now, let perpendicular distance between parallel lines AB and CD be h…(i)
Area of ║ABCD = (Base) (corresponding altitude)
= (CD) (h) …(ii)
From (i) and (ii)
Area of ║ABCD = 4(ar(ΔDFB))
Area of║ABCD = 4(3 cm2) = 12 cm2
Q.8. In trapezium ABCD, AB || DC and L is the mid-point of BC. Through L, a line PQ || AD has been drawn which meets AB in P and DC produced in Q (Fig. 9.18). Prove that ar (ABCD) = ar (APQD)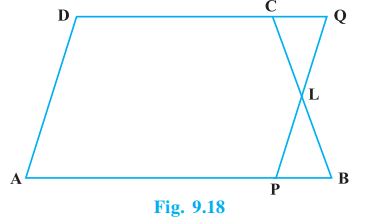
As AB ∥ DC, so AB ∥ DQ
In ΔCLQ and ΔBLP, we have
∴∠QCL = ∠LBP [[Alt. ∠S]
CL = LB [∵ L is the mid-point of BC]
∠CLQ = ∠BLP [ Vertically opposite ∠S]
∴ ΔQCL ≅ ∠BLP [ By ASA congruence rule]
⇒ ar(ΔCLQ) = ar(ΔBLP) .......(1) [Congruence ΔS are equal in area]
Adding ar(APLCD) to both sides of (1), we get
ar(ΔCLQ) + ar(APLCD) = ar(ΔBLP) + ar(APLCD)
⇒ ar(APQD) = ar(ABCD)
Hence, ar(ABCD) = ar(APQD)
Q.9. If the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram so formed will be half of the area of the given quadrilateral (Fig. 9.19). [Hint: Join BD and draw perpendicular from A on BD.]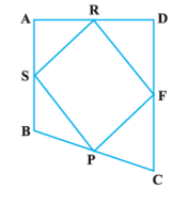
Consider a quadrilateral ABCD
P, F, R and S are the midpoints of the sides BC, CD, AD and AB
PFRS is a parallelogram.
Join BD and BR
We know that the median of a triangle divides it into two triangles of equal areas. BR is the median of the triangle BDA.
So, ar(BRA) = 1/2 ar(BDA) --------------- (1)
RS is the median of the triangle BRA.
So, ar(ASR) = 1/2 ar(BRA) --------------- (2)
From (1) and (2),
ar(ASR) = 1/2 (1/2 ar(BDA)
ar(ASR) = 1/4 ar(BDA) --------------------- (3)
Similarly, ar(CFP) = 1/4 ar(BCD) -------- (4)
Adding (3) and (4),
ar(ASR) + ar(CFP) = 1/4 ar(BDA) + 1/4 ar(BCD)
ar(ASR) + ar(CFP) = 1/4 ar(BCDA) --------- (5)
Similarly, ar(DRF) + ar(BSP) = 1/4 ar(BCDA) ------------ (6)
Adding (5) and (6),
ar(ASR) + ar(CFP) + ar(DRF) + ar(BSP) = 2(1/4 ar(BCDA))
ar(ASR) + ar(CFP) + ar(DRF) + ar(BSP) = 1/2 ar(BCDA) ------------- (7)
From the figure,
ar(ASR) + ar(CFP) + ar(DRF) + ar(BSP) + ar(PFRS) = ar(BCDA)
From (7),
1/2 ar(BCDA) + ar(PFRS) = ar(BCDA)
ar(PFRS) = ar(BCDA) - 1/2 ar(BCDA)
Therefore, ar(PFRS) = 1/2 ar(BCDA)
Exercise 9.4
Q.1. A point E is taken on the side BC of a parallelogram ABCD. AE and DC are produced to meet at F. Prove that ar (ADF) = ar (ABFC)
According to the question,
ABCD is a parallelogram.
E is a point on BC.
AE and DC are produced to meet at F.
To prove: area (ΔADF) = area (ABFC).
Proof: We know that,
Triangles on the same base and between the same parallels are equal in area.
Here,
We have,
ΔABC and ΔABF are on same base AB and between same parallels, AB || CF.
Area (ΔABC) = area (ΔABF) … (1)
We also know that,
Diagonal of a Parallelogram divides it into two triangles of equal area
So,
Area (ΔABC) = area (ΔACD) … (2)
Now,
Area (ΔADF) = area (ΔACD) + area (ΔACF)
∴ Area (ΔADF) = area (ΔABC) + area (ΔACF) … (From equation (2))
⇒ Area (ΔADF) = area (ΔABF) + area (ΔACF) … (From equation (1))
⇒ Area (ΔADF) = area (ABFC)
Q.2. The diagonals of a parallelogram ABCD intersect at a point O. Through O, a line is drawn to intersect AD at P and BC at Q. Show that PQ divides the parallelogram into two parts of equal area.
According to the question,The diagonals of a parallelogram ABCD intersect at a point O.
Through O, a line is drawn to intersect AD at P and BC at Q.
To Prove: Ar (parallelogram PDCQ) = ar (parallelogram PQBA).
Proof: AC is a diagonal of || gm ABCD
∴ ar(ΔABC) = ar(ΔACD)
= ½ ar (||gm ABCD) …(1)
In ΔAOP and ΔCOQ,
AO = CO
Since, diagonals of a parallelogram bisect each other,
We get,
∠AOP = ∠COQ
∠OAP = ∠OCQ (Vertically opposite angles)
∴ ΔAOP = ΔCOQ (Alternate interior angles)
∴ ar(ΔAOP) = ar(ΔCOQ) (By ASA Congruence Rule)
We know that,
Congruent figures have equal areas
So,
ar(ΔAOP) + ar(parallelogram OPDC) = ar(ΔCOQ) + ar(parallelogram OPDC)
⇒ ar(ΔACD) = ar(parallelogram PDCQ)
⇒ ½ ar(|| gm ABCD) = ar(parallelogram PDCQ)
From equation (1),
We get,
ar(parallelogram PQBA) = ar(parallelogram PDCQ)
⇒ ar(parallelogram PDCQ) = ar(parallelogram PQBA).
Hence Proved.
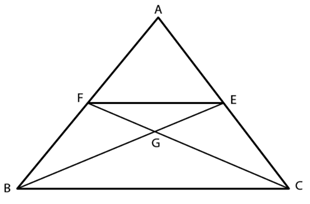
Q.3. The medians BE and CF of a triangle ABC intersect at G. Prove that the area of ∆ GBC = area of the quadrilateral AFGE.
According to the question,
We have,
BE & CF are medians
E is the midpoint of AC
F is the midpoint of AB
∴ ΔBCE = ΔBEA … ( i )
ΔBCF = ΔCAF
Construct:
Join EF,
By midpoint theorem,
We get FE || BC
We know that,
Δ on the same base and between same parallels are equal in area
∴ ΔFBC = ΔBCE
ΔFBC – ΔGBC = ΔBCE – ΔGBC
⇒ ΔFBG = ΔCGE (ΔGBC is common)
⇒ ΔCGE = ΔFBG …( ii )
Subtracting equation (ii) from (i)
We get,
ΔBCE – ΔCGE = ΔBEA – ΔFBG
∴ ΔBGC =quadrilateral AFGE.
Q.4. In Fig. 9.24, CD || AE and CY || BA. Prove that ar (CBX) = ar (AXY)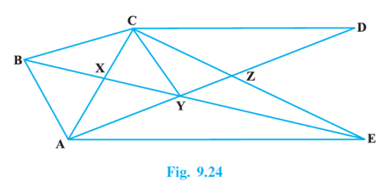
According to the question,
From figure,
We get,
CD||AE
and CY || BA
To prove: ar (ΔCBX) = ar (ΔAXY) .
Proof: We know that,
Triangles on the same base and between the same parallels are equal in areas.
Here,
ΔABY and ΔABC both lie on the same base AB and between the same parallels CY and BA.
ar (ΔABY) = ar (ΔABC)
⇒ ar (ABX) + ar (AXY) = ar (ABX) + ar (CBX)
Solving and cancelling ar (ABX),
We get,
⇒ ar (AXY) = ar (CBX)
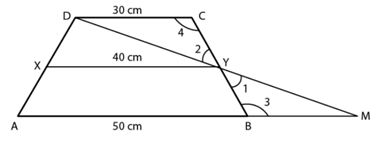
Q.5. ABCD is a trapezium in which AB || DC, DC = 30 cm and AB = 50 cm. If X and Y are, respectively the mid-points of AD and BC, prove that ar (DCYX) = 7/9 ar (XYBA)
According to the question,
We have,
ABCD is a trapezium with AB || DC
Construction: Join DY and produce it to meet AB produced at P.
In ∆BYP and ∆CYD∠BYP = ∠CYD (vertically opposite angles)
Since, alternate opposite angles of DC || AP and BC is the transversal
∠DCY = ∠PBY
Since, Y is the midpoint of BC,
BY = CY
Thus ∆BYP ≅ ∆CYD (by ASA cogence criterion)
So, DY = YP and DC = BP
⇒ Y is the midpoint of AD
∴ XY || AP and XY = ½ AP (by midpoint theorem)
⇒ XY = ½ AP
= ½ (AB + BP)
= ½ (AB + DC)
= ½ (50 + 30)
= ½ × 80 cm
= 40 cm
Since X is the midpoint of AD
And Y is the midpoint of BC
Hence, trapezium DCYX and ABYX are of same height, h, cm
Now
⇒ 9 ar(DCXY) = 7 ar(XYBA)
⇒ ar(DCXY) = 7/9 ar(XYBA)
Hence Proved.
Q.6. In ∆ ABC, if L and M are the points on AB and AC, respectively such that LM || BC. Prove that ar (LOB) = ar (MOC)
Given, ABC is a triangle
L and M are the points on AB and AC
LM || BC
We have to prove that ar(LOB) = ar(MOC)
We know that the area of triangles on the same base and between the same parallel lines are equal.
Triangles LBC and MBC are on the same base BC and between the same parallel lines BC and LM.
So, ar(LBC) = ar(MBC)
From the figure,
ar(LBC) = ar(LOB) + ar(BOC)
Also, ar(MBC) = ar(MOC) + ar(BOC)
Now, ar(LOB) + ar(BOC) = ar(MOC) + ar(BOC)
Cancelling out common terms,
ar(LOB) = ar(MOC)
Therefore, it is proved that ar(LOB) = ar(MOC)
Q.7. In Fig. 9.25, ABCDE is any pentagon. BP drawn parallel to AC meets DC produced at P and EQ drawn parallel to AD meets CD produced at Q. Prove that ar (ABCDE) = ar (APQ)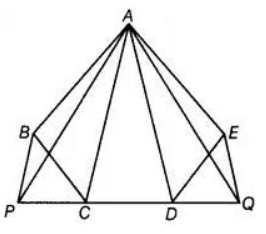
BP ∣∣ AC and AD ∣∣ EQ
Since, triangles on the same base and between the same parallels are equal in area
ar(ΔABC) = ar(ΔAPC).........(1)
and ar(ΔADE) = ar(ΔADQ).........(2)
Adding (1) and (2), we get
ar(ΔABC) + ar(ΔADE) = ar(ΔAPC) = +ar(ΔADQ)
Adding ar(ΔACD) to both sides, we get
ar(ΔABC) + ar(ΔADE) + ar(ΔACD) = ar(ΔAPC) + ar(ΔADQ) + ar(ΔACD)
Hence, ar(ABCDE) = ar(ΔAPQ)
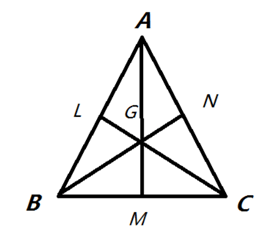
Q.8. If the medians of a ∆ABC intersect at G, show that ar (AGB) = ar (AGC) = ar (BGC) = 1/3 ar (ABC)
Given, ABC is a triangle
The medians of triangle ABC intersect at G
We have to show that ar(AGB) = ar(BGC) = 1/3 ar(ABC)
In triangle ABC,
Since AD is a median ar(ABD) = ar(ACD) --------- (1)
In triangle BGC,
Since GD is a median
ar(GBD) = ar(GCD) ------------ (2)
Subtracting (1) and (2),
ar(ABD) - ar(GBD) = ar(ACD) - ar(GCD)
So, ar(AGB) = ar(AGC) --------- (3)
Similarly,
ar(AGB) = ar(BGC) ----------- (4)
From (3) and (4),
ar(AGB) = ar(BGC) = ar(AGC) ------------- (5)
Now, ar(ABC) = ar(AGB) + ar(BGC) + ar(AGC)
From (5),
ar(ABC) = ar(AGB) + ar(AGB) + ar(AGB)
ar(ABC) = 3ar(AGB)
So, ar(AGB) = 1/3 ar(ABC) -------------- (6)
From (5) and (6),
ar(BGC) = 1/3 ar(ABC)
ar(AGC) = 1/3 ar(ABC)
Therefore, ar(AGB) = ar(BGC) = ar(AGC) = ⅓ ar(ABC)
Q.9. In Fig. 9.26, X and Y are the mid-points of AC and AB respectively, QP || BC and CYQ and BXP are straight lines. Prove that ar (ABP) = ar (ACQ).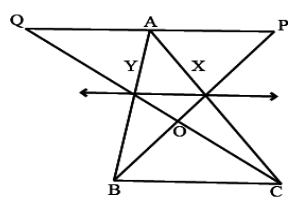
Since X and Y are the mid points AC and AB respectively.
∴ XY ∥ BC
Clearly, triangles BYC and BXC are on the same base BC and between the same parallel XY and BC
∴ ar(△BYC) = ar(△BXC)
⇒ ar(△BYC) − ar(△BOC) = ar(△BXC)−ar(△BOC)
⇒ ar(△BOY) = ar(△COX)
⇒ ar(△BOY) + ar(△XOY) = ar(△COX) + ar(△XOY)
⇒ ar(△BXY) + ar(△CXY)...(i)
⇒ ar(△BYX) + ar(△AXY) = ar(△AXY) + ar(△AYX)
⇒ ar(△BAX) = ar(△CAY)...(ii)
In △BAP, XY= 1/2 AP
In △CAQ, XY= 1/2 AQ
∴ AP = AQ
Clearly, triangles XAP and YAQ are between the same parallels and their bases AP and AQ are equal.
∴ ar(△XAP)=ar(△YAQ).....(iii)
Adding (ii) and (iii), we obtain
ar(△XAP) + ar(△BAX) = ar(△YAQ)ar(△CAY)
⇒ ar(△ABP) = ar(△ACQ)
Hence Proved
Q.10. In Fig. 9.27, ABCD and AEFD are two parallelograms. Prove that ar (PEA) = ar (QFD) [Hint: Join PD]. 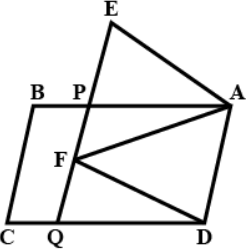
Given, ABCD and AEFD are two parallelograms
We have to prove that ar(PEA) = ar(QFD)
Considering triangles APE and DQF,
We know that the corresponding angles are equal
∠APE = ∠DQF
We know that the opposite sides of a parallelogram are equal
AE = DF
∠AEP = ∠DFQ
The Angle-Side-Angle Postulate (ASA) states that if two angles and the included side of one triangle are congruent to two angles and the included side of another triangle, then the two triangles are congruent.
By ASA criteria, the triangles APE and DQF are congruent.
The Corresponding Parts of Congruent Triangles are Congruent (CPCTC) theorem states that when two triangles are comgruent, then their corresponding sides and angles are also congruent or equal in measurements.
By CPCTC,
PE = FQ
We know that congruent triangles have equal area
So, ar(APE) = ar(DQF)
Therefore, ar (PEA) = ar (QFD)