NCERT Exemplar Solutions: Lines & Angles | Mathematics (Maths) Class 9 PDF Download

Exercise 8.1

Write the correct answer in each of the following:

Q.1. Three angles of a quadrilateral are 75º, 90º and 75º. The fourth angle is
(a) 90º
(b) 95º
(c) 105º
(d) 120º

Correct Answer is Option (d)
According to the question,
Three angles of quadrilateral are 75°, 90° and 75°
Consider the fourth angle to be x.
We know that,
Sum of all angles of a quadrilateral = 360°
⇒ 75° + 90° + 75° + x = 360°
⇒ 240° + x = 360°
⇒ x = 360° – 240°
⇒ x = 120°
Hence, the fourth angle is 120°.
Therefore, option (d) is the correct answer.

Q.2. A diagonal of a rectangle is inclined to one side of the rectangle at 25º. The acute angle between the diagonals is
(a) 55º
(b) 50º

(c) 40º
(d) 25º

Correct Answer is Option (b)
According to the question,
A diagonal of a rectangle is inclined to one side of the rectangle at 25º
i.e., Angle between a side of rectangle and its diagonal = 25°
Consider the acute angle between diagonals to be = x

We know that diagonals of a rectangle are equal in length i.e.,
AC = BD
Dividing RHS and LHS by 2,
⇒ ½ AC = ½ BD
Since, O is mid-point of AC and BD
⇒ OD = OC
Since, angles opposite to equal sides are equal
⇒ ∠y = 25°
We also know that,
Exterior angle is equal to the sum of two opposite interior angles.
So, ∠BOC = ∠ODC + ∠OCD
⇒ ∠x = ∠y + 25°
⇒ ∠x = 25° + 25°
⇒ ∠x = 50°
Hence, the acute angle between diagonals is 50°.
Therefore, option (B) is the correct answer.

Q.3. ABCD is a rhombus such that ∠ACB = 40º. Then ∠ADB is
(a) 40º
(b) 45º
(c) 50º
(d) 60º

Correct Answer is Option (c)

According to the question,
ABCD is a rhombus
∠ACB = 40°

∵ ∠ACB = 40°
⇒ ∠OCB = 40°
∵ AD ∥ BC
⇒ ∠DAC = ∠BCA = 40° [Alternate interior angles]
⇒ ∠DAO = 40°

Since, diagonals of a rhombus are perpendicular to each other
We have,
∠AOD = 90°
We know that,
Sum of all angles of a triangle = 180°
⇒ ∠AOD + ∠ADO + ∠DAO = 180°
⇒ 90° + ∠ADO + 40° = 180°
⇒ 130° + ∠ADO = 180°
⇒ ∠ADO = 180° – 130°
⇒ ∠ADO = 50°
⇒ ∠ADB = 50°
Hence, ∠ADB = 50°
Therefore, option (c) is the correct answer.

Q.4. The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rectangle, if
(a) PQRS is a rectangle
(b) PQRS is a parallelogram
(c) diagonals of PQRS are perpendicular
(d) diagonals of PQRS are equal.

Correct Answer is Option (c)
Let the rectangle be ABCD,
We know that,
Diagonals of rectangle are equal
∴ AC = BD
⇒ PQ = QR
∴ PQRS is a rhombus
Diagonals of a rhombus are perpendicular.
Hence, diagonals of PQRS are perpendicular
Therefore, option (c) is the correct answer.

Q.5. The quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rhombus, if
(a) PQRS is a rhombus
(b) PQRS is a parallelogram
(c) diagonals of PQRS are perpendicular
(d) diagonals of PQRS are equal.

Correct Answer is Option (d)
Since, ABCD is a rhombus
We have,
AB = BC = CD = DA
Now,
Since, D and C are midpoints of PQ and PS
By midpoint theorem,
We have,
DC = ½ QS
Also,
Since, B and C are midpoints of SR and PS
By midpoint theorem

We have,
BC = ½ PR
Now, again, ABCD is a rhombus
∴ BC = CD
⇒ ½ QS = ½ PR
⇒ QS = PR
Hence, diagonals of PQRS are equal
Therefore, option (d) is the correct answer.

Q.6. If angles A, B, C and D of the quadrilateral ABCD, taken in order, are in the ratio 3:7:6:4, then ABCD is a
(a) Rhombus
(b) Parallelogram
(c) Trapezium
(d) Kite

Correct Answer is Option (c)
As angle A, B, C and D of the quadrilateral ABCD, taken in order, are in the ratio 3: 7: 6: 4,
We have the angles A, B, C and D = 3x, 7x, 6x and 4x.
Now, sum of the angle of a quadrilateral = 360o.
3x + 7x + 6x + 4x = 360°
⇒20x = 360°
⇒ x = 360 ÷ 20 =18°
So, the angles A, B, C and D of quadrilateral ABCD are,
∠A = 3×18° = 54°,

∠B = 7×18° = 126°
∠C = 6×18° = 108°
∠D = 4×18° = 72o
AD and BC are two lines cut by a transversal CD
Now, sum of angles ∠C and ∠D on the same side of transversal,
∠C +∠D =108° + 72° =180
Hence, AD|| BC
So, ABCD is a quadrilateral in which one pair of opposite sides are parallel.
Hence, ABCD is a trapezium.
Therefore, option (c) is the correct answer.

Q.7. If bisectors of ∠A and ∠B of a quadrilateral ABCD intersect each other at P, of ∠B and ∠C at Q, of ∠C and ∠D at R and of ∠D and ∠A at S, then PQRS is a
(a) Rectangle
(b) Rhombus
(c) Parallelogram
(d) Quadrilateral whose opposite angles are supplementary

Correct Answer is Option (d)
We know that,
Sum of all angles of a quadrilateral = 360°
⇒ ∠A + ∠B + ∠C + ∠D = 360°
Dividing LHS and RHS by 2,
⇒ ½ (∠A + ∠B + ∠C + ∠D) = ½ × 360° = 180°
Since, AP, PB, RC and RD are bisectors of ∠A, ∠B, ∠C and ∠D
⇒ ∠PAB + ∠ABP + ∠RCD + ∠RDC = 180° … (1)
We also know that,
Sum of all angles of a triangle = 180°
∠PAB + ∠APB + ∠ABP = 180°
⇒ ∠PAB + ∠ABP = 180° – ∠APB …(2)
Similarly,
∴ ∠RDC + ∠RCD + ∠CRD = 180°
⇒ ∠RDC + ∠RCD = 180° – ∠CRD …(3)
Substituting the value of equations (2) and (3) in equation (1),
180° – ∠APB + 180° – ∠CRD = 180°

⇒ 360° – ∠APB – ∠CRD = 180°
⇒ ∠APB + ∠CRD = 360° – 180°
⇒ ∠APB + ∠CRD = 180° …(4)
Now,
∠SPQ = ∠APB [vertically opposite angles]
∠SRQ = ∠DRC [vertically opposite angles]

Substituting in equation (4),
⇒ ∠SPQ + ∠SRQ = 180°
Hence, PQRS is a quadrilateral whose opposite angles are supplementary.
Therefore, option (d) is the correct answer.

Q.8. In Fig. 6.1, if AB || CD || EF, PQ || RS, ∠RQD = 25° and ∠CQP = 60°, then ∠QRS is equal to

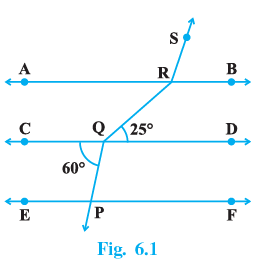
(a) 85°
(b) 135°
(c) 145°
(d) 110°

Correct Answer is Option (c)

Given figure, if AB || CD || EF, PQ || RS, RQD=25o and CQP=60o,

Then ∠SRB = ∠CQP = 60°

OR ∠QRA = ∠RQD = 25°

So ∠ARS +∠SRB = 180°

∴∠ARS= 120 − 60 = 120°

∠QRS = ∠ARS + ∠QRA = 120+25 = 145°

Q.9. If one angle of a triangle is equal to the sum of the other two angles, then the triangle is
(a) an isosceles triangle
(b) an obtuse triangle
(c) an equilateral triangle
(d) a right triangle

Correct Answer is Option (d)

Let the angles of a triabgle be α,β,γ

Given α + β = γ
We now that in a sum of triangles sum of angles is 180°
So, α + β + γ = 180°
⇒ 2γ = 180°

⇒ γ = 90 °

So, it is a right angled triangle.

Q.10. An exterior angle of a triangle is 105° and its two interior opposite angles are equal. Each of these equal angles is
(a) 37(1/2)°
(b) 52(1/2)°
(c) 72(1/2)°
(d) 75°

Correct Answer is Option (b)
By exterior angle theorem,
Exterior angle = Sum of interior opposite angle
Let each interior opposite angles = x
105 = x + x
105 = 2x
x = 52(1/2)°

Q.11. The angles of a triangle are in the ratio 5 : 3 : 7. The triangle is
(a) an acute angled triangle
(b) an obtuse angled triangle
(c) a right triangle
(d) an isosceles triangle

Correct Answer is Option (a)
The angles of the triangle are in the ratio: 5 : 3 : 7
Let the angles be 5x, 3x, 7x
By Angle sum property,
5x + 3x + 7x = 180
15x = 180
x = 12
Thus, angles are, 60°, 36°, 84°
Since, all the angles are less than 90°, it is an acute angled triangle.

Q.12. If one of the angles of a triangle is 130°, then the angle between the bisectors of the other two angles can be
(a) 50°
(b) 65°
(c) 145°
(d) 155°

Correct Answer is Option (d)

Consider a △ABC,such that ∠BAC=130° and bisectors of ∠B and ∠C meet at O.
To find: ∠BOC
Now, in △ABC,
∠BAC + ∠ABC + ∠ACB = 180
130 + ∠ABC+∠ACB = 180 (Angle sum property)
∠ABC +∠ACB = 50
(1/2)(∠ABC + ∠ACB) = 25
∠OBC + ∠OCB = 25 (OB and OC bisect ∠ABC and ∠ACB)
Now, in △OBC,
∠OBC + ∠OCB + ∠BOC = 180
25 + ∠BOC = 180
∠BOC = 155°.

Q.13. In Fig. 6.2, POQ is a line. The value of x is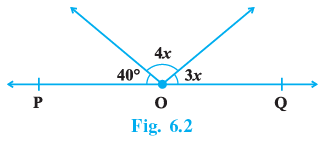

(a) 20°
(b) 25°
(c) 30°
(d) 35°

Correct Answer is Option (a)
Given POQ is a line, POQ = 180°
40° + 4x + 3x = 180°
⇒ 7x = 140°
⇒ x = 20°

Q.14. In Fig. 6.3, if OP||RS, ∠OPQ = 110° and ∠QRS = 130°, then ∠ PQR is equal to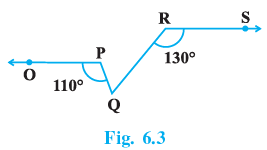

(a) 40°
(b) 50°
(c) 60°
(d) 70°

Correct Answer is Option (c)
Using the fact that OP || RS, we know that
∠RWV = 180° − 130°
1.  ∠RWV = 50°
We know that,
∠PWQ = ∠RWV = 50° (Since, opposite angles of intersecting lines are equal)
Also, for line OP
∠OQP + θ = 180°
θ = 180° − ∠OPQ = 180° − 110°
2.  θ = 70°
Now, we know that sum of angles of a triangle is 180°,
∠PQR + θ + ∠PWQ = 180°
∠PQR = 180° − θ − ∠PWQ = 180° − 70° − 50°
∠PQR = 180° − 120°
∠PQR = 60°

Q.15. Angles of a triangle are in the ratio 2 : 4 : 3. The smallest angle of the triangle is
(a) 60°
(b) 40°
(c) 80°
(d) 20°

Correct Answer is Option (b)
The angles of the triangle are in the ratio: 2 : 4 : 3
Let the angles be 2x, 4x, 3x
By Angle sum property,
2x + 4x + 3x = 180
9x = 180
x = 20
Thus, angles are, 40°, 80° , 60°
Thus, the smallest angle = 40°

Exercise 8.2

Q.1. Diagonals AC and BD of a parallelogram ABCD intersect each other at O. If OA = 3 cm and OD = 2 cm, determine the lengths of AC and BD.

According to the question,
OA = 3 cm
OD = 2 cm
We know that,
Diagonals of parallelogram bisect each other.
Then,
AC = 2AO
AC = 2 × 3 cm
AC = 6 cm
And,
BD = 2OD
BD = 2 × 2 cm
BD = 4 cm
Hence, AC = 6 cm and BD = 4cm

Q.2. Diagonals of a parallelogram are perpendicular to each other. Is this statement true? Give reason for your answer.

The statement “diagonals of a parallelogram are perpendicular to each other” is false.
Justification:
Diagonals of a parallelogram bisect each other but not at 90°.
So, they are not perpendicular to each other.
Hence, this statement is false.

Q.3. Can the angles 110º, 80º, 70º and 95º be the angles of a quadrilateral? Why or why not?

The angles 110º, 80º, 70º and 95º cannot be the angles of a quadrilateral.
Justification:
We know that,
Sum of all angles of a quadrilateral = 360°
Sum of given angles,
110° + 80° + 70° + 95° = 355° ≠ 360°
Hence, 110°, 80°, 70° and 95° cannot be the angles of a quadrilateral.

Q.4. In quadrilateral ABCD, ∠A + ∠D = 180º. What special name can be given to this quadrilateral?

According to the question,
In quadrilateral ABCD, ∠A + ∠D = 180º
We know that,
In a trapezium,
Sum of co-interior angles = 180°

Hence, the given quadrilateral is a trapezium.

Q.5. All the angles of a quadrilateral are equal. What special name is given to this quadrilateral?

According to the question,
All the angles of a quadrilateral are equal.
Suppose all the angles of the quadrilateral = x
We know that,
Sum of all angles of a quadrilateral = 360°
⇒ x + x + x + x = 360°
⇒ 4x = 360°
⇒ x = 360°/4
⇒ x = 90°
Hence, the quadrilateral is a rectangle.

Q.6. Diagonals of a rectangle are equal and perpendicular. Is this statement true? Give reason for your answer.

The statement “diagonals of a rectangle are equal and perpendicular” is false.
We know that,
Diagonals of a rectangle bisect each other.
Therefore, they are equal but they are not perpendicular.
Hence, the statement is not true.

Q.7. Can all the four angles of a quadrilateral be obtuse angles? Give reason for your answer.

All the four angles of a quadrilateral cannot be obtuse angles.
Justification:
We know that,
Sum of all angles of a quadrilateral = 360°
So, at least one angle should be acute angle.
Hence, all the four angles of a quadrilateral cannot be obtuse angles.

Q.8. For what value of x + y in Fig. 6.4 will ABC be a line? Justify your answer.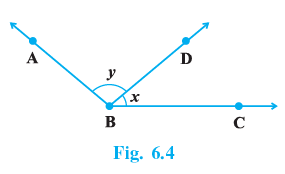

The value of x + y can't be find out with the given data.
No, ABC is not a line as it not straight, it is bent at point B.

Q.9. Can a triangle have all angles less than 60°? Give reason for your answer.

By angle sum property, we know, the sum of all angles of a triangle = 180°.
⇒ 60° + 60° + 60°
= 180°.
If all the these angles is less than 60°, then there sum together would not make 180°.
Say for example, 30°, 40° and 50°  are 3 angles of the triangle.
∴ 30 + 40 + 50 = 120° < 180° .
Therefore, it is not possible.
Hence, the answer is No.

Q.10. Can a triangle have two obtuse angles? Give reason for your answer.

An angle whose measure is more than 90° but less than 180° is called an obtuse angle.
An triangle cannot have two obtuse angles because the sum of all the angles of it cannot be more than 180°. It is always equal to 180°.

Q.11. How many triangles can be drawn having its angles as 45°, 64° and 72°? Give reason for your answer.

The three angles given are 45°, 64°, 72°
Now, sum of angles = 45 + 64 +72
Sum of angles = 181°
Sum of angles of triangle cannot be more than 180°.
Hence, triangle is not possible.

Q.12. How many triangles can be drawn having its angles as 53°, 64° and 63°? Give reason for your answer.

The three angles given are 54°, 64°, 63°
Now, sum of angles = 53 + 64 + 63
Sum of angles = 180°
Sum of angles of triangle is 180°.
Hence, infinitely many triangles can be formed with these three angles.

Q.13. In Fig. 6.5, find the value of x for which the lines l and m are parallel.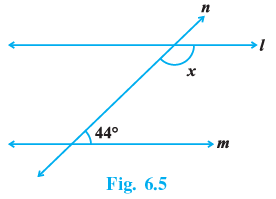

Given, l ∥ m
∠x and 44° are co interior angles. Sum of the co - interior angles is 180° .
Hence, ∠x + 44 = 180
∠x = 136° 

Q.14. Two adjacent angles are equal. Is it necessary that each of these angles will be a right angle? Justify your answer.

No it is not necessary.

Two angles are said to be adjacent when they have common vertex, a common side but do not overlap. They can be of any measure. For example, 33° and 25° can be two adjacent angles.

Q.15. If one of the angles formed by two intersecting lines is a right angle, what can you say about the other three angles? Give reason for your answer.

Let AB and CD be two lines Intersecting at O, such that, ∠AOD = 90°
Now, ∠AOD = ∠COB = 90°  (Vertically opposite angles)
⇒ ∠AOD + ∠DOB = 180° (Angles on a straight line)
⇒ 90 + ∠DOB = 180°
∠DOB = 90°
∠DOB = ∠AOC = 90° (Vertically opposite angles)
Thus, all angles are 90°.

Q.16. In Fig.6.6, which of the two lines are parallel and why?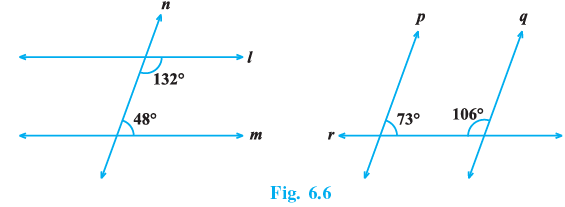

For fig(i) , a transversal intersects two lines such that the sum of interior angles on the same side on the same side of the transversal is 132° +48° = 180°.
Therefore , the line l and m are parallel.
For fig.(ii) , a transversal intersects two lines such that the sum of interior angles on the same side of the transversal is 73°  +106° = 179°.
Therefore , the lines p and q are not parallel.

Q.17. Two lines l and m are perpendicular to the same line n. Are l and m perpendicular to each other? Give reason for your answer.

When two lines l and m are perpendicular to the same line n , each of the two corresponding angles formed by these lines l and m with the line n are equal (each is equal to 90°). Hence , the line l and m are not perpendicular but parallel.

Exercise 8.3

Q.1. One angle of a quadrilateral is of 108º and the remaining three angles are equal. Find each of the three equal angles.

Let the remaining three equal angles be x.
We know,
Sum of all interior angles of a quadrilateral is = 360o
108º  + x + x + x = 360º
108º + 3x = 360º
3x = 360º – 108º
3x = 252º
x = 252/3
x = 84º
Each of three equal angles, x = 84o.

Q.2. ABCD is a trapezium in which AB || DC and ∠A = ∠B = 45º. Find angles C and D of the trapezium.

According to the question,
ABCD is a trapezium
∠A = ∠B = 45º

We know that,
Angles opposite to each other in quadrilateral are supplementary.
Then, we have,
∠A + ∠C = 180º
45º + ∠C = 180º
∠C = 180º – 45º
∠C = 135º
Similarly,
We have,
∠B + ∠D = 180º
45º + ∠D = 180º
∠D = 180º – 45º
∠D = 135º

Q.3. The angle between two altitudes of a parallelogram through the vertex of an obtuse angle of the parallelogram is 60º. Find the angles of the parallelogram.

According to the question,
ABCD is parallelogram,
DP ⊥ AB
DQ ⊥ BC.
∠PDQ = 60º
In quad. DPBQ,
Using angle sum property of a quadrilateral,
We have,
∠PDQ + ∠Q + ∠P + ∠B = 360º
60º + 90º + 90º + ∠B = 360º
240º + ∠B = 360º

∠B = 360º – 240º
∠B = 120º
Since, opposite angles in parallelogram are equal,
We have,
∠B = ∠D = 120º
Since, opposite sides are parallel in parallelogram,
We have,
AB||CD
Also, since sum of adjacent interior angles is 180o,
We have,
∠B + ∠C = 180º
120º + ∠C = 180º
∠C = 180º – 120º
∠C = 60º
Since, opposite angles in parallelogram are equal,
We have,
∠C = ∠A = 60º

Q.4. ABCD is a rhombus in which altitude from D to side AB bisects AB. Find the angles of the rhombus.

According to the question,
We have,
ABCD is a rhombus.
DE is the altitude on AB then AE = EB.
In ΔAED and ΔBED,
We have,
DE = DE (common line)
∠AED = ∠BED (right angle)
AE = EB (DE is an altitude)
∴ ΔAED ≅ ΔBED by SAS property.
∴ AD = BD (by C.P.C.T)
But AD = AB (sides of rhombus are equal)
⇒ AD = AB = BD
∴ ABD is an equilateral triangle.
∴ ∠A = 60º
Since, opposite angles of rhombus are equal, we get,
⇒ ∠A = ∠C = 60º
We also know that,
Sum of adjacent angles of a rhombus = supplementary.
So,
∠ABC + ∠BCD = 180º

∠ABC + 60º = 180º
∠ABC = 180º – 60º = 120º
Since, opposite angles of rhombus are equal, we get,
∠ABC = ∠ADC = 120º
Hence, Angles of rhombus are:
∠A = 60º, ∠C = 60º, ∠B = 120º, ∠D = 120º

Q.5. E and F are points on diagonal AC of a parallelogram ABCD such that AE = CF. Show that BFDE is a parallelogram.

Construction:
Join BD, meeting AC at O.
According to the question,
Since diagonals of a parallelogram bisect each other,
We get,
OA = OC and OD = OB.
And,
OA = OC and AE = CF,
OA – AE = OC – CF
OE = OF
So, BFDE is a quadrilateral whose diagonals bisect each other.
Hence, BFDE is a parallelogram.

Q.6. In Fig. 6.9, OD is the bisector of  ∠AOC, OE is the bisector of ∠BOC and OD ⊥ OE. Show that the points A, O and B are collinear.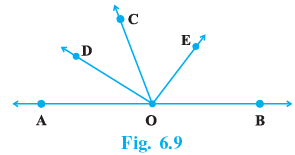

True
Given:
OD is the bisector of ∠AOC
OE is the bisector of ∠BOC
OD ⊥ OE
Proof:
From given we have ∠AOD = ∠DOC .... (1) and ∠BOE = ∠EOC .... (2)
∠DOC + ∠EOC = 90° ... (a)
Substituting from 1 and 2
∠AOD + ∠BOE = 90° ... (b)
(a) + (b)
∠AOD + ∠DOC + ∠EOC + ∠BOE = 90°  + 90°
∠AOD + ∠DOC + ∠EOC + ∠BOE = 180°
∴ ∠AOB = 180°  and A, O and B are Collinear.
∠AOD + ∠DOC = ∠AOC and ∠BOE + ∠EOC = ∠BOC
∴ ∠AOC + ∠BOC = 180°

Q.7. In Fig. 6.10, ∠1 = 60° and ∠6 = 120°. Show that the lines m and n are parallel.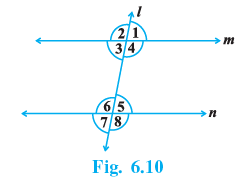

We have,
∠5 + ∠6 = 180° [linear pair Angles]
⇒ ∠5 + 120°  = 180°  ⇒ ∠5 = 180° −120° = 60°
Now, ∠1 = ∠5 [Each = 60°]
But, these are corresponding angles.
Therefore, the lines m and n are parallel.

Q.8. AP and BQ are the bisectors of the two alternate interior angles formed by the intersection of a transversal t with parallel lines l and m (Fig. 6.11). Show that AP || BQ.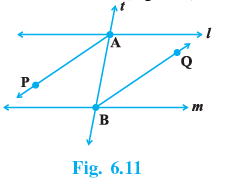

∵ 1 ∣∣ m and t is the transversal
∠MAB = ∠SBA  [Alt. ∠s]
(1/2) ∠MAB = (1/2) ∠SBA ⇒ ∠PAB = ∠QBA
But, ∠PAB and ∠QBA are alternate angles.
Hence, AP ∣∣ BQ.

Q.9. If in Fig. 6.11, bisectors AP and BQ of the alternate interior angles are parallel, then show that l || m.

Given Data.
∠AP is the bisector of ∠LMAB
∠BQ is the bisector of ∠LSBA
And  ∠AB||∠BQ
As AP||BQ
∴ L2 = L3
2 ∠L2 = 2 ∠L3 = L2 + L2 = L3 + L3
= L1 + L2 = L3 + L4
Then, L1 = L2 and L3 = L4
∴ ∠LMAB = ∠LSBA  [Alternate Angles]
∴ l║m
Hence Proved!

Q.10. In Fig. 6.12, BA || ED and BC || EF. Show that ∠ABC = ∠DEF [Hint: Produce DE to intersect BC at P (say)].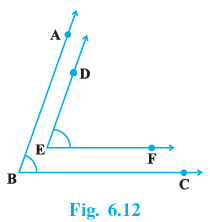

Produce DE to intersect BC at P (say).
EF || BC and DP is the transversal ,
∴ ∠ DEF = ∠ DPC ...(i) [Corres. ∠ s]
Now , AB || DP and BC is the transversal,
∴ ∠ DPC = ∠ ABC ...(ii) [Corres. ∠ s]
From (i) and (ii) , we get
∠ ABC = ∠ DEF
Hence , proved.

Q.11. In Fig. 6.13, BA || ED and BC || EF. Show that ∠ ABC + ∠ DEF = 180°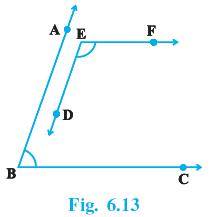

∵BA∣∣ED
∠1=∠2.......(i)
(Corresponding angles)
Also BC || EF
⇒ ∠2 + ∠3 = 180°...(ii)
(Sum of the interior angles on the same side of a transversal is 180°)
From (i) and (ii), we get
∠1 + ∠3 = 180°
i.e. ∠ABC + ∠DEF = 180°

Q.12. In Fig. 6.14, DE || QR and AP and BP are bisectors of ∠ EAB and ∠ RBA, respectively. Find ∠APB.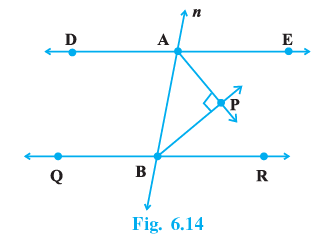

Given: DE∥QR, AP bisects ∠EAB, BP bisects ∠RBA
Since, DE∥QR
∠EAB+∠RBA = 180°
(1/2)(∠EAB+∠RBA) = 90°
∠PAB+∠PBA=90° (AP bisects ∠EAB, BP bisects ∠RBA)
Now, In △PAB
∠PAB + ∠PBA + ∠APB = 180°
90° +∠APB = 180°
∠APB = 90°

Q.13. The angles of a triangle are in the ratio 2 : 3 : 4. Find the angles of the triangle.

Angle sum property of a triangle is 180degrees. It is given that the angles are in the ratio 2:3:4.
So let us consider the angles as 2x,3x and 4x.
2x + 3x + 4x = 9x
Angle sum of triangle is 180∘
so 9x = 180
or x = 180/9 = 20°
∴ 2x = 2 × 20 = 40°
3x = 3 × 20 = 60°
and 4x = 4 × 20° = 80°
∴ the angles are 40°, 60°, 80°.

Q.14. A triangle ABC is right angled at A. L is a point on BC such that AL ⊥ BC. Prove that ∠ BAL = ∠ ACB.

Since Δ ABC is right-angled,
AB² + AC² = BC²
or, AB² + AC² = (BL+ CL)²
or, AB² +AC² = BL² + CL² + 2.BL.CL ...(1).
Again from Δ ALC and Δ ALB we have
AC² = AL² + CL²...(2) and AB² = AL² + BL² ...(3).
Adding (2) and (3) we get,
AC² + AB² = 2.AL² + CL² + BL²
or, BL² + CL² + 2.BL.CL = 2.AL² + BL² + CL²

or, AL² = BL.CL ...(4).
Now  tan ∠ACB = AL/CL ....(5) and tan ∠ABL = AL/BL
⇒ tan(90° − ∠BAL) = (AL/BL)
⇒ cot ∠BAL = (AL/BL) ...(6)
From (4) we have (AL/CL) = (BL/AL)
or, tan ∠ACB = tan ∠BAL [Using (5) and (6)]
or, ∠ACB = ∠BAL.

Q.15. Two lines are respectively perpendicular to two parallel lines. Show that they are parallel to each other.

According to the figure consider the two parallel lines m and n

we know that p ⊥ m and q ⊥ n

so we get

∠1 = ∠2 = 90°

We know that m ∥ n and p is a transversal

from the figure we know that ∠1 and ∠3 are corresponding angles

so we get

∠1 = ∠3

We also know that

∠2 + ∠3 = 90°

We know that ∠2 and ∠3 are corresponding angles when the transversal n cuts p and q

so we get p ∥ q

therefore, it is shown that the two lines which are perpendicular to two parallel lines are parallel to each other.

Exercise 8.4

Q.1. A square is inscribed in an isosceles right triangle so that the square and the triangle have one angle common. Show that the vertex of the square opposite the vertex of the common angle bisects the hypotenuse.

According to the question,
ΔABC with ∠A = 90º and
Since, ABC is an isosceles triangle,
We get,
AB = AC …(i)
Let ADEF be the square inscribed in the isosceles triangle ABC.
Then, we have,
AD = AF = EF = AD …(ii)
Subtracting equation (ii) from (i),
AB – AD = AC – AF
BD = CF
Now,
Considering ΔCFE and ΔEDB,
BD = CF
DE = EF
∠CFE = ∠EDB = 90º (Since, they are the side of a square)
ΔCEF ~ ΔBED (By SAS criteria)
Hence, CE = BE
Therefore, vertex E of the square bisect the hypotenuse BC.

Q.2. In a parallelogram ABCD, AB = 10 cm and AD = 6 cm. The bisector of ∠A meets DC in E. AE and BC produced meet at F. Find the length of CF.

According to the question,
We have,
ABCD is a parallelogram
AB = 10 cm
AD = 6cm.
The bisector of ∠A meets DC at E.
AE and BC produced meet at F.
Since, AF bisects ∠A,
We get,
∠BAE = ∠EAD … (1)
∠EAD = ∠EFB … (2) [Alternate angles]
From equations (1) and (2),
We get,
∠BAE = ∠EFB
Since sides opposite to equal angles are equal,
We get,
BF = AB
Here, AB = 10 cm
So, BF = 10 cm
⇒ BC + CF = 10 cm
6 cm + CF = 10 cm [BC = AD = 6 cm, opposite sides of a parallelogram]
⇒ CF = 10 – 6 cm = 4 cm
⇒ CF = 4 cm

Q.3. P, Q, R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD in which AC = BD. Prove that PQRS is a rhombus.

According to the question,
We have,
P is the mid-point of the sides AB
Q is the mid-point of the sides BC
R is the mid-point of the sides CD
S is the mid-point of the sides DA
Also, we know that,
AC = BD.
In ΔADC, by mid-point theorem,
SR = ½ AC
And, SR||AC
In ΔABC, by mid-point theorem,
PQ = ½ AC
And, PQ||AC
Hence, SR = PQ = ½ AC
Similarly,
In ΔBCD, by mid-point theorem,
RQ = ½ BD
And, RQ||BD
In ΔBAD, by mid-point theorem,
SP = ½ BD
And, SP||BD
So, we get,
SP = RQ = ½ BD = ½ AC
Then,
SR = PQ = SP = RQ
Hence, PQRS is a rhombus.

Q.4. P, Q, R and S are respectively the mid-points of the sides AB, BC, CD and DA of a quadrilateral ABCD such that AC ⊥ BD. Prove that PQRS is a rectangle.

According to the question,
We have,
P is the mid-point of the sides AB
Q is the mid-point of the sides BC
R is the mid-point of the sides CD
S is the mid-point of the sides DA
Also,
AC ⊥ BD
∠COD = ∠AOD = ∠AOB = ∠COB = 90º

In ΔADC, by mid-point theorem,
SR = ½ AC
And, SR||AC
In ΔABC, by mid-point theorem,
PQ = ½ AC
And, PQ||AC
So, we have,
PQ||SR and SR = PQ = ½ AC
Similarly,
SP||RQ and SP = RQ = ½ BD
Now, in quadrilateral EOFR,
OE||FR and OF||ER
So, we get,
∠EOF = ∠ERF = 90º
Hence, PQRS is a rectangle.

Q.5. P, Q, R and S are respectively the mid-points of sides AB, BC, CD and DA of quadrilateral ABCD in which AC = BD and AC ⊥ BD. Prove that PQRS is a square.

According to the question,
We have,
P is the mid-point of the sides AB
Q is the mid-point of the sides BC
R is the mid-point of the sides CD
S is the mid-point of the sides DA
Also,
AC ⊥ BD
And AC = BD

In ΔADC, by mid-point theorem,
SR = ½ AC
And, SR||AC
In ΔABC, by mid-point theorem,
PQ = ½ AC
And, PQ||AC
So, we have,
PQ||SR and PQ = SR = ½ AC
Now, in ΔABD, by mid-point theorem,
SP||BD and SP = ½ BD = ½ AC
In ΔBCD, by mid-point theorem,
RQ||BD and RQ = ½ BD = ½ AC
SP = RQ = ½ AC
PQ = SR = SP = RQ
Thus, we get that,
All four sides are equal.
Considering the quadrilateral EOFR,
OE||FR, OF||ER
∠EOF = ∠ERF = 90º (Opposite angles of parallelogram)
∠QRS = 90º
Hence, PQRS is a square.

Q.6. A diagonal of a parallelogram bisects one of its angles. Show that it is a rhombus.

Let the parallelogram be = ABCD

Diagonal AC bisect ∠A.

∠CAB = ∠CAD

Now,
AB||CD and AC is a transversal.
∠CAB = ∠ACD
Again, AD||BC and AC is a transversal.
∠DAC = ∠ACB
Now,
∠A = ∠C
½ ∠A = ½ ∠C
∠DAC = ∠DCA
AD = CD
But, AB = CD and AD = BC (Opposite sides of parallelograms)
AB = BC = CD = AD
Thus, ABCD is a rhombus.

Q.7. P and Q are the mid-points of the opposite sides AB and CD of a parallelogram ABCD. AQ intersects DP at S and BQ intersects CP at R. Show that PRQS is a parallelogram.

According to the question,
Q is the midpoint of AB
P is the midpoint of CD
Now,
AB||CD,
Also,
AP||QC
And, AB = DC
½ AB = ½ DC
AP = QC
Now,
AP||QC and AP = QC
APCQ is a parallelogram.
AQ||PC or SQ||PR
Again,
AB||DC means ½ AB = ½ DC
BP = QD
Now, BP||QD and BP = QD
BPDQ is a parallelogram
So, PD||BQ or PS||QR
Thus, SQ||RP and PS||QR
PQRS is a parallelogram.

Q.8. ABCD is a quadrilateral in which AB || DC and AD = BC. Prove that ∠A = ∠B and ∠C = ∠D.

According to the question,
We have,
Quadrilateral ABCD
AB||CD and AD = BC.
To prove: ∠A = ∠B and ∠C = ∠D.
Construction: Draw DP ⊥ AB and CQ ⊥ AB.
Proof: In ΔAPD and ΔBQC,
Since ∠1 and ∠2 are equal to 90o
∠1 = ∠2
Distance between parallel line,
AB = BC [Given]
By RHS criterion of congruence,
We have
ΔAPD ≅ ΔBQC [CPCT]
∠A = ∠B
Now, DC||AB
Since, sum of consecutive interior angles is 180o
∠A+∠3 =180 …(1)
And,
∠B +∠4 =180 …(2)
From equations (1) and (2),
We get
∠A + ∠3 = ∠B + ∠4
Since, ∠A = ∠B,
We have,
⇒ ∠3 = ∠4
⇒ ∠C = ∠D
Hence, proved.

Q.9. In Fig. 8.11, AB || DE, AB = DE, AC || DF and AC = DF. Prove that BC || EF and BC = EF.

According to the question,
In quadrilateral ABED,
We have,
AB||DE and AB = DE
ABED is a parallelogram.
AD||BE and AD = BE
In quadrilateral ACFD,
We have,
AC||FD and AC = FD
ACFD is a parallelogram.
AD||CF and AD = CF
AD = BE = CF and CF||BE
In quadrilateral BCFE,
BE = CF and BE||CF.
BCFE is a parallelogram.
BC = EF and BC||EF
Hence proved.

Q.10. If two lines intersect, prove that the vertically opposite angles are equal.

If two lines i.e. AB & CD intersect each other. They have two pair of opp. angles.
i.e.
∠AOC, ∠DOB, ∠AOB, ∠COB.
To prove :- ∠AOC = ∠DOB & ∠AOD = ∠COB
Proof :-
∠AOC + ∠AOD = 180° [linear pair] ___(1)
∠AOD+∠BOD=180° [linear pair] ___(2)
from eq. (1) & (2)
∠AOC + ∠AOD = ∠AOD + ∠BOD
i.e.  [∠AOC = ∠BOD]
Similarly [∠AOD = ∠COB] Hence proved.

Q.10. Bisectors of interior ∠B and exterior ∠ACD of a ∆ ABC intersect at the point T. Prove that ∠BTC = (1/2)∠BAC.

According to the problem ∠TBC = (1/2)∠B ...(1) and
∠TCD = (1/2) ∠ACD ...(2).
Now from Δ ABC we have
∠A + ∠B = ∠ACD ...(3).
And from ΔTBC we have
∠T + ∠TBC = ∠TCD
or, ∠T + (1/2) ∠B = (1/2) ∠ACD[Using (1) and (2)]
or,  ∠T = (1/2) (∠ACD − ∠B)
or, ∠T = (1/2)∠A. [ Using (3)]
or, ∠BTC = (1/2) ∠BAC.

Q.11. A transversal intersects two parallel lines. Prove that the bisectors of any pair of corresponding angles so formed are parallel.

The transversal AD intersects the two lines PQ and RS at points B and C respectively. BE is the bisector of ∠ABQ and CF is the bisector of ∠BCS.
As, BE is the bisector of ∠ABQ, then,
∠ABE = (1/2)∠ABQ
In the same way,
∠BCF = (1/2)∠BCS
Since BE and CF are parallel and AD is the transversal, therefore, by corresponding angle axiom,
∠ABE = ∠BCF
(1/2) ∠ABQ = (1/2)∠BCS
∠ABQ = ∠BCS
Therefore, by the converse of corresponding angle axiom, PQ ∥ RS.

Q.12. Prove that through a given point, we can draw only one perpendicular to a given line. [Hint: Use proof by contradiction].

From the point P , a perpendicular PM is drawn to the given line AB.
∴ ∠PMB = 90°
Let if possible , we can draw another perpendicular PN to the line AB. Then ,
∠PMB = 90°
∴ ∠PMB = ∠PNB , which is possible only when PM and PN coincide with each other.
Hence , through a given point , we can draw only one perpendicular to a given line.

Q.13. Prove that two lines that are respectively perpendicular to two intersecting lines intersect each other. [Hint: Use proof by contradiction].

If n and p are any two intersecting lines.
Draw two lines such that l ⊥ n and m ⊥ p
To prove: Lines l andm will intersect.
Proof:
Let n and p be two intersecting lines.
Let us assume that l and m do not intersect each other.
Now l ⊥ n (Given)
Let us assume that l ⊥ m
Therefore, m ⊥ n ...(1)
But given that m ⊥ p ...(2)
From (1) and (2), we get n ∥ p
But given, lines n and p intersect each other.
Hence, our assumption is wrong.
Therefore l and m intersect each other.

Q.14. Prove that a triangle must have atleast two acute angles.

Consider a triangle ABC
We have to prove that a triangle must have atleast two acute angles.

By angle sum property,
∠A + ∠B + ∠C = 180°___(1)
Case (1): consider ∠B = 90° and ∠C = 90°
From (1),
∠A + 90° + 90° = 180°
∠A + 180° = 180°
∠A = 180° - 180°
∠A = 0°
Therefore, no triangle can be formed.
Case (2): when two angles are obtuse
Consider ∠B and ∠C are greater than 90°
Let ∠B = 100° and ∠C = 95°
From (1),
∠A + 100° + 95° = 180°
∠A + 195° = 180°
∠A = 180° - 195°
∠A = - 15°
Angle A is negative, which is not possible.
Therefore, no triangle can be formed.
Case (3) : when one angle is 90 and other angle is obtuse
Let ∠B = 90° and ∠C = 100°
From (1),
∠A + 90° + 100° = 180°
∠A + 190° = 180°
∠A = 180° - 190°
∠A = -10°
Angle A is negative, which is not possible.
Therefore, no triangle can be formed.
Case (4) : when two angles are acute
Consider ∠B and ∠C are less than 90
Let ∠B = 80° and ∠C = 60°
∠A + 80° + 60° = 180°
∠A + 140° = 180°
∠A = 180° - 140°
∠A = 40°
Verification:
∠A + ∠B + ∠C = 180°
LHS : 40° + 80° + 60°
= 100° + 80°
= 180°
= RHS
Therefore, triangles can be formed.

Q.15. In Fig. 6.17, ∠Q > ∠R, PA is the bisector of ∠QPR and PM ⊥ QR. Prove that∠APM = (1/2) (∠Q – ∠R).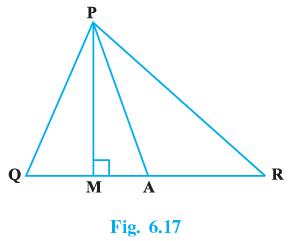

In △PAQ
Sum of two opposite interior angles = Exterior angle
α = θ + ∠θ −(i)
In △PAR
α + θ +∠R = 180°
α = 180° − θ − ∠R −(ii)
In △PMQ
∠MPQ = 180 − ∠Q − 90°
= 90 − ∠Q
∠APM = ∠APQ − ∠MPQ
= θ − (90 − ∠Q)
= θ + ∠Q − 90° −(iii)
∵ (i) = (ii)
⇒ θ + ∠Q = 180 − θ − ∠R
⇒ 2θ = 180 − ∠Q − ∠R
⇒ θ = 90 − (1/2) (∠Q + ∠R)
putting this value of θ in (iii)
∠APM = ∠Q + 90 − (1/2) (∠Q + ∠R) − 90

∴ a = 2