NCERT Exemplar: Work & Energy | Science Class 9 PDF Download
Multiple Choice Questions
Q1: When a body falls freely towards the earth, its total energy
(a) increases
(b) decreases
(c) remains constant
(d) first increases and then decreases
Ans: (c)
Explanation: When a body falls freely towards the earth, its potential energy decreases while its kinetic energy increases. However, the total mechanical energy (the sum of potential energy and kinetic energy) remains constant in the absence of air resistance, according to the law of conservation of energy.
Q2: A car is accelerated on a levelled road and attains a velocity 4 times of its initial velocity. In this process the potential energy of the car
(a) does not change
(b) becomes twice that of the initial
(c) becomes 4 times that of the initial
(d) becomes 16 times that of the initial
Ans: (a)
Explanation: On a levelled road, the height of the car does not change. Since potential energy (PE = mgh) depends on the height (h) above the ground, the potential energy remains constant regardless of the change in velocity.
Q3: In the case of negative work, the angle between the force and displacement is
(a) 0∘
(b) 45∘
(c) 90∘
(d ) 180∘
Ans: (d)
Explanation: Negative work occurs when the force and displacement are in opposite directions. The angle between the force and displacement in this case is 180∘, as the force acts directly opposite to the direction of motion.
Q4: An iron sphere of mass 10 kg has the same diameter as an aluminium sphere of mass 3.5 kg. Both spheres are dropped simultaneously from a tower. When they are 10 m above the ground, they have the same
(a) acceleration
(b) momenta
(c) potential energy
(d) kinetic energy
Ans: (a)
Explanation: Both spheres, regardless of their mass, experience the same acceleration due to gravity (g ≈ 9.8m/s2) when in free fall, provided air resistance is negligible. The acceleration is independent of their masses.
Q5: A girl is carrying a school bag of 3 kg mass on her back and moves 200 m on a levelled road. The work done against the gravitational force will be (g =10 m s–2)
(a) 6 ×103 J
(b) 6 J
(c) 0.6 J
(d) zero
Ans: (d)
Explanation: Work done against gravitational force is given by W = mgh, where h is the vertical displacement. Since the girl is walking on a level road, there is no vertical displacement (h = 0). Therefore, the work done against the gravitational force is zero.
Q6: Which one of the following is not the unit of energy?
(a) joule
(b) newton metre
(c) kilowatt
(d) kilowatt-hour
Ans: (c)
Explanation:
- Joule and Newton metre are both units of energy (1 Newton metre = 1 Joule).
- A kilowatt-hour is also a unit of energy used in electricity (1 kilowatt-hour = 3.6 × 10⁶ Joules).
- Kilowatt, however, is a unit of power (rate of energy transfer), not energy itself.
Q7: The work done on an object does not depend upon the
(a) displacement
(b) force applied
(c) angle between force and displacement
(d) initial velocity of the object
Ans: (d)
Explanation: Work done (W = F⋅d⋅cosθ) depends on: The displacement (d), The force applied (F), and the angle (θ) between the force and displacement. It does not depend on the initial velocity of the object, as work is related to force and displacement, not the object's initial motion.
Q8: Water stored in a dam possesses
(a) no energy
(b) electrical energy
(c) kinetic energy
(d) potential energy
Ans: (d)
Explanation: Water stored in a dam is at a height above the ground. Due to this height, it possesses gravitational potential energy (PE = mgh), which can be converted into kinetic energy and subsequently into electrical energy when released to generate power.
Q9: A body is falling from a height h. After it has fallen from a height h/2, it will possess
(a) only potential energy
(b) only kinetic energy
(c) half potential and half kinetic energy
(d) more kinetic and less potential energy
Ans: (c)
Explanation: So the energy at the point after crossing half the distance is half kinetic energy and half potential energy. The correct answer for this problem is option (C).
Short-answer Questions
Q10: A rocket is moving up with a velocity v. If the velocity of this rocket is suddenly tripled, what will be the ratio of the two kinetic energies?
Ans:
Initial velocity = v
Final Velocity = 3v
Initial kinetic energy = 1/2mv2
Kinetic energy = 1/2mv2
1/2 m (3v)2 = 9 (1/2mv2)
Ratio of final to initial kinetic energy = 9:1
Q11: Avinash can run with a speed of 8 m s–1 against the frictional force of 10 N, and Kapil can move with a speed of 3 m s–1 against the frictional force of 25 N. Who is more powerful and why?
Ans: P = f × v
P1= 10× 8 = 80W
P2 = 25×3 = 75W
Hence, Avinash is more powerful than Kapil
Q12: A boy is moving on a straight road against a frictional force of 5 N. After travelling a distance of 1.5 km he forgot the correct path at a roundabout (Fig. 11.1) of radius 100 m. However, he moves on the circular path for one and half cycle and then he moves forward upto 2.0 km. Calculate the work done by him. Fig. 11.1
Fig. 11.1
Ans:
Distance in 1.5 cycles = 1.5 × 2πr = 1.5 × 2 × 3.14 × 100 = 942 m
Total = 1500 + 942 + 2000 = 4442 m
Work = 5 × 4442 = 22,210 J
Q13: Can any object have mechanical energy even if its momentum is zero? Explain.
Ans: Yes, an object can have mechanical energy even if its momentum is zero.
- Mechanical energy is the sum of potential energy (PE) and kinetic energy (KE).
- If an object is stationary (momentum p = mv = 0, as velocity v = 0), its kinetic energy is also zero (KE = 1/2mv2 = 0).
- However, the object can still have potential energy due to its position (e.g., at a height above the ground, PE = mgh).
Thus, an object at rest with zero momentum can still possess mechanical energy in the form of potential energy.
Q14: Can any object have momentum even if its mechanical energy is zero? Explain.
Ans: No, an object cannot have momentum if its mechanical energy is zero.
Momentum is given by p=mv, where m is the mass and v is the velocity of the object. For an object to have momentum, it must have some velocity.
Mechanical energy is the sum of kinetic energy (K.E.) and potential energy (P.E.).
- If the mechanical energy is zero, both K.E. = 1/2mv2 = 0 (indicating v = 0) and P.E. = 0 (indicating no potential energy).
- With v = 0, momentum (p = mv) must also be zero.
Hence, an object with zero mechanical energy cannot have momentum.
Q15: The power of a motor pump is 2 kW. How much water per minute the pump can raise to a height of 10 m? (Given g = 10 m s–2)
Ans: Power of pump = 2kW = 2000W
Time (t)= 60 sec
Height (h) = 10m g = 10 m/s2
Power = work done per unit time.
Work done = mgh = m × 10 × 10 =100m
⇒ 2000 W = (m ×100) / 60
⇒ 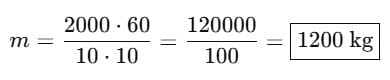 So, the pump can raise 1200kg of water in one minute.
So, the pump can raise 1200kg of water in one minute.
Q16: The weight of a person on planet A is about half that on Earth. He can jump up to 0.4 m in height on the surface of the earth. How high can he jump on planet A?
Ans: The height to which a person can jump is inversely proportional to the gravitational acceleration (g) on a planet, as the jump height is determined by the kinetic energy transferred to potential energy:
mgh = constant for the same jump force.
On planet A, the weight of the person is half that on Earth, meaning the gravitational acceleration on planet A (gA) is half that on Earth (gE):
gA = 1/2 gE.
The height of the jump is inversely proportional to g, so if g decreases by half, the jump height will double. Thus, the person can jump:
hA = 2 x hE = 2 x 0.4m = 0.8m.
So, the person can jump up to 0.8 m on planet A.
Q17: The velocity of a body moving in a straight line is increased by applying a constant force F, for some distance in the direction of the motion. Prove that the increase in the kinetic energy of the body is equal to the work done by the force on the body.
Ans: To prove that the increase in kinetic energy of the body is equal to the work done by the force, we use the work-energy theorem, which states:
Step-by-Step Proof:
(i) Work Done by the Force:
The work done (W) by a constant force F over a displacement d in the direction of motion is given by:
W=F⋅d.
(ii) Newton's Second Law
From Newton's second law, the force F is related to the mass m and acceleration a of the body as:
F = m ⋅ a.
(iii) Kinematic Relation
Using the equation of motion for a body starting at an initial velocity u and reaching a final velocity v under constant acceleration a over displacement d:
v2 = u2 + 2ad.
Rearrange to express d in terms of v, u, and a:

(iii) Substitute into Work Equation
Substituting F = ma and  into W = F . d:
into W = F . d:
(iv) Simplify
Cancel a from the numerator and denominator:
(v) Relate to Kinetic Energy
The change in kinetic energy (ΔKE) of the body is:
Simplify:
(vi) Conclusion
From the above, the work done W equals the change in kinetic energy:
W = ΔKE.
Thus, the increase in kinetic energy of the body is equal to the work done by the force.
Q18: Is it possible that an object is in a state of accelerated motion due to an external force acting on it, but no work is being done by the force? Explain it with an example.
Ans: Yes, an object can be in accelerated motion due to an external force while no work is done by the force.
Work done by a force is given by W = F ⋅ d ⋅ cosθ,
where:
- F is the force applied,
- d is the displacement,
- θ is the angle between the force and the displacement.
For no work to be done, cosθ = 0, which happens when the force is perpendicular to the displacement (θ = 90∘).
Example: Uniform Circular Motion
- When an object moves in a circular path (e.g., a planet orbiting a star or a stone tied to a string and whirled in a circle), the centripetal force acts towards the centre of the circular path.
- The displacement of the object is tangential to the circular path at any point.
- The angle between the centripetal force and the displacement is 90∘.
Since cos 90∘ = 0, the work done by the centripetal force is zero, even though the object is in accelerated motion (changing direction).
Q19: A ball is dropped from a height of 10 m. If the energy of the ball reduces by 40% after striking the ground, how much high can the ball bounce back? (g = 10 m s–2).
Ans: To determine how high the ball can bounce back, we analyze the energy transfer using the conservation of energy and the given energy loss.
Step-by-Step Solution:
- Initial Potential Energy of the Ball: The potential energy (PE) of the ball at the initial height (h1 = 10m) is:
PE= mgh1 = m ⋅ 10 ⋅ 10 = 100m. - Energy Loss After Striking the Ground: The energy of the ball reduces by 40%, so the remaining energy is:
Remaining Energy = 60% of Initial Energy.
Remaining Energy = 0.6 ⋅ 100m = 60m. - Potential Energy at Maximum Height After Bouncing Back: After bouncing back, the remaining energy is converted into potential energy at the new maximum height (h2):
mgh2 = 60m.
Simplify:
So, the ball can bounce back to a height of 6 meters.
Q20: If an electric iron of 1200 W is used for 30 minutes everyday, find electric energy consumed in the month of April.
Ans:
Power of electric iron = 1200W
Usage per day = 30min
= 30/60hrs
= 0.5hrs
Number of days in the month of April = 30 days
Electrical energy consumed, E = P x t 1200 × 0.5 × 30
= 18000 WH
= 18 KWH
= 18 units
Therefore, The Total Electricity consumed in the month of April is 18 units
Long answer Questions
Q21: A light and a heavy object have the same momentum. Find out the ratio of their kinetic energies. Which one has a larger kinetic energy?
Ans: To compare the kinetic energies of a light and a heavy object with the same momentum, we use the formula for kinetic energy and momentum:
Momentum (p) is given by: p = mv,
where m is the mass and v is the velocity of the object.Kinetic energy (KE) is: KE = 1/2mv2.
Substituting v = p/m from the momentum equation into the kinetic energy formula:
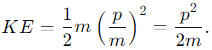
Ratio of Kinetic Energies:
For two objects with masses m1 (light object) and m2 (heavy object) having the same momentum p: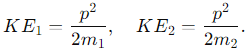 The ratio of their kinetic energies is:
The ratio of their kinetic energies is: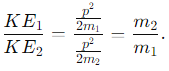 So, the light object (m1) has a larger kinetic energy because its mass is smaller.
So, the light object (m1) has a larger kinetic energy because its mass is smaller.
The ratio of kinetic energies is: KE1 : KE2 = m2 : m1.
Q22: An automobile engine propels a 1000 kg car (A) along a levelled road at a speed of 36 km h–1. Find the power of the opposing frictional force is 100 N. Now, suppose after travelling a distance of 200 m, this car collides with another stationary car (B) of the same mass and comes to rest. Let its engine also stop at the same time. Now, car (B) starts moving on the same level road without getting its engine started. Find the speed of the car (B) just after the collision.
Ans:
m(A)= m(B) = 1000 kg.
v = 36 km/h =10 m/s
Frictional force = 100 N
Car A moves with a uniform speed, which means the engine of the car applies a force equal to the frictional force.
(i) Power = Force × distance / time = F . V
= 100 N × 10 m/s = 1000 W
(ii) After collision mA uA + mB uB = mA vA + mB vB.
1000 × 10 + 1000 × 0 = 1000 × 0 + 1000 × vB
10000 = 1000 vB
vB= 10 m s–1
Q23: A girl having a mass of 35 kg sits on a trolley of mass 5 kg. The trolley is given an initial velocity of 4 m s–1 by applying a force. The trolley comes to rest after traversing a distance of 16 m. (a) How much work is done on the trolley? (b) How much work is done by the girl?
Ans:
Initial velocity of the trolley, u = 4 m/s
Final velocity of the trolley, v = 0 m/s
Mass of the trolley, m = 5 kg
Distance covered by the trolley before coming to rest, s = 16 m
Use the work-energy theorem: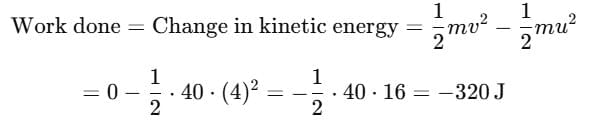
Therefore, –320 J (work is negative because the force opposes motion, e.g., friction).
(b) Since the girl is sitting still on the trolley and does not move relative to it, her displacement is zero.
Work done = Force × Displacement = 0
Q24: Four men lift a 250 kg box to a height of 1 m and hold it without raising or lowering it.
(a) How much work is done by the men in lifting the box?
(b) How much work do they do just in holding it?
(c) Why do they get tired while holding it? (g = 10 m s–2)
Ans: Given:
- Mass of the box (m) = 250 kg
- Height (h) = 1 m
- Gravitational acceleration (g) = 10m/s2
(a) Work done in lifting the box:
The work done in lifting the box is equal to the increase in its gravitational potential energy:
W = m g h
Substituting the values:
W = 250 ⋅ 10 ⋅ 1 = 2500J.
So, the work done by the men in lifting the box is 2500 J.
(b) Work done in holding the box:
When holding the box stationary, there is no displacement. Work is defined as:
W = F ⋅ d ⋅ cosθ,
where d = 0 (no displacement). Therefore:
W = 0.
So, the work done by the men in just holding the box is 0 J.
(c) Why do they get tired while holding the box?
Even though no mechanical work is done while holding the box (as there is no displacement), the men exert a force to counteract the gravitational pull on the box. This involves the continuous contraction and relaxation of muscles, which requires energy. The chemical energy stored in their bodies (in the form of ATP) is consumed, producing fatigue. Therefore, they get tired because their muscles use energy to maintain the force required to hold the box, even though no mechanical work is being done.
Q25: What is power? How do you differentiate kilowatt from kilowatt-hour? The Jog Falls in Karnataka state are nearly 20 m high. 2000 tonnes of water fall from it in a minute. Calculate the equivalent power if all this energy can be utilised? (g = 10 m s–2)
Ans:
Power is the rate of transfer of energy or the rate of doing work. Watt is the unit of power, and kilowatt is 1000 watts.
h = 20 m, and mass = 2000 × 103 kg
= 2 × 106 kg
Power = m g h / t
Power = (2 x 106 x 10 x 20) / 60
Power = 4/6 x 107
Power = 2/3 x 107w = 6.67 x 106W
Q26: How is the power related to the speed at which a body can be lifted? How many kilograms will a man working at the power of 100 W, be able to lift at a constant speed of 1 m s –1 vertically? (g = 10 m s–2)
Ans: Power (P) is related to the speed (v) of lifting by the formula:
P = F × v
where F is the force needed (equal to weight mg when lifting vertically at constant speed).
Given:
P = 100W, v = 1m/s, g = 10m/s2.
Force, F = m × g = m × 10
Substitute into the power formula: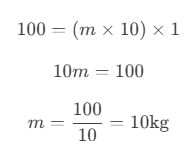
The man can lift 10 kg at a constant speed of 1 m/s vertically.
Q27: Define watt. Express kilowatt in terms of joules per second. A 150 kg car engine develops 500 W for each kg. What force does it exert in moving the car at a speed of 20 m s–1?
Ans: A watt is the unit of power.
1 watt is defined as the power when 1 joule of work is done in 1 second.
1 watt = 1joule/second
Also, 1 kilowatt = 1000 watts = 1000J/sec
Mass of car = 150 kg
Power for each kg = 500 x 150 =75000 w
Speed = 20m/s
Power = Force x v
∴ Force = Power/v = 75000/20
= 3750N
Q28: Compare the power at which each of the following is moving upwards against the force of gravity? (given g = 10 m s–2)
(i) a butterfly of mass 1.0 g that flies upward at a rate of 0.5 m s–1.
(ii) a 250 g squirrel climbing up a tree at a rate of 0.5 m s–1.
Ans: To calculate the power for each case, we use the formula:
P = F × v
where:
- P is the power,
- F is the force acting against gravity (F = mg).
- v is the velocity.
Given:
- Gravitational acceleration (g) = 10m/s2,
- Velocity (v) = 0.5m/s.
(i) Butterfly:
- Mass of butterfly (m) = 1.0 g = 0.001kg,
- Force (F = mg) = 0.001 × 10 = 0.01N,
- Power (P) = F ⋅ v = 0.01 × 0.5 = 0.005W.
Power for the butterfly: 0.005W.
(ii) Squirrel:
- Mass of squirrel (m) = 50g = 0.25kg,
- Force (F = mg) = 0.25 × 10 = 2.5N,
- Power (P) = F⋅v = 2.5 × 0.5 = 1.25W.
Power for the squirrel: 1.25W.
Comparison:
- Butterfly's power: 0.005W,
- Squirrel's power: 1.25W.
The squirrel requires significantly more power than the butterfly to move upwards at the same velocity.
|
84 videos|478 docs|60 tests
|
FAQs on NCERT Exemplar: Work & Energy - Science Class 9
| 1. What is the principle of conservation of energy? |  |
| 2. How is work defined in physics, and what is its formula? |  |
| 3. What are the different forms of energy discussed in the context of work and energy? |  |
| 4. What is the relationship between work and energy? |  |
| 5. How can we calculate the kinetic energy of an object? |  |






















