NCERT Solutions Class 11 Maths Chapter 3 - Trigonometric Functions
Question 1: Prove that: 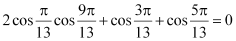
ANSWER : L.H.S.
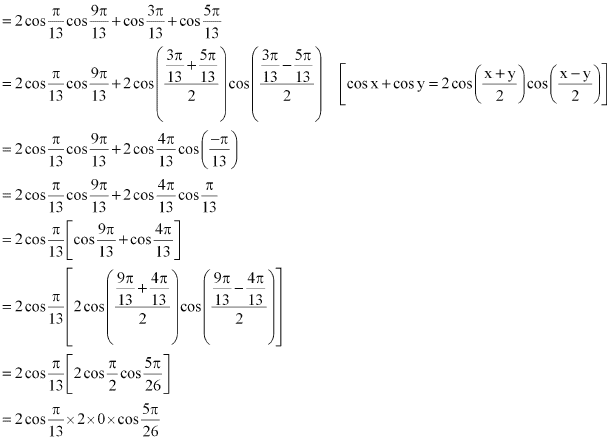
= 0 = R.H.S
Question 2: Prove that: (sin 3x + sin x) sin x +(cos 3x – cos x) cos x = 0
ANSWER : L.H.S.
= (sin 3x + sin x) sin x + (cos 3x – cos x) cos x
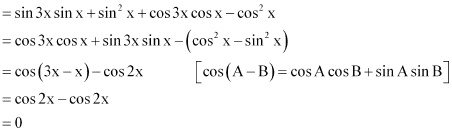
= RH.S.
Question 3: Prove that: 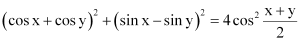
ANSWER : L.H.S. = 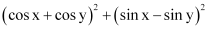
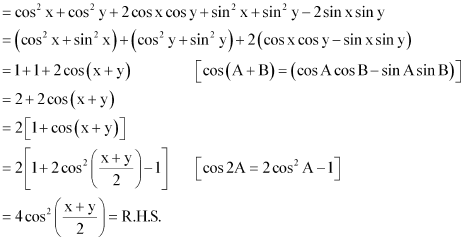
Question 4: Prove that: 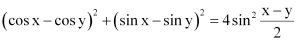
ANSWER : L.H.S. = 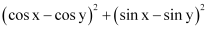
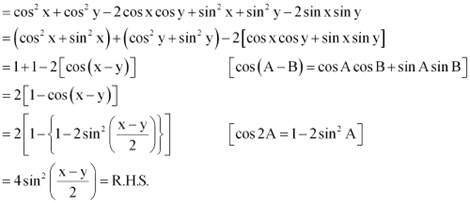
Question 5: Prove that: 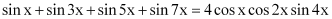
ANSWER : It is known that 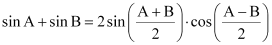 .
.
∴L.H.S. = 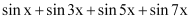
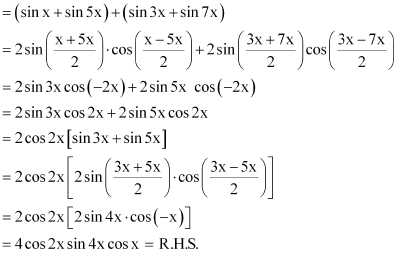
Question 6: Prove that: 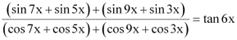
ANSWER : It is known that
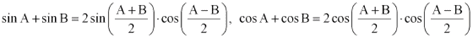 .
.
L.H.S. = 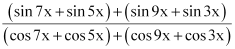
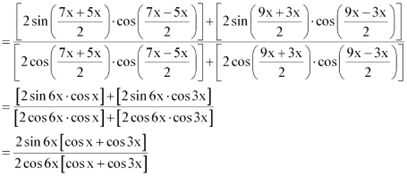
= tan 6x
= R.H.S.
Question 7: Prove that: 
ANSWER : L.H.S. = 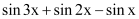
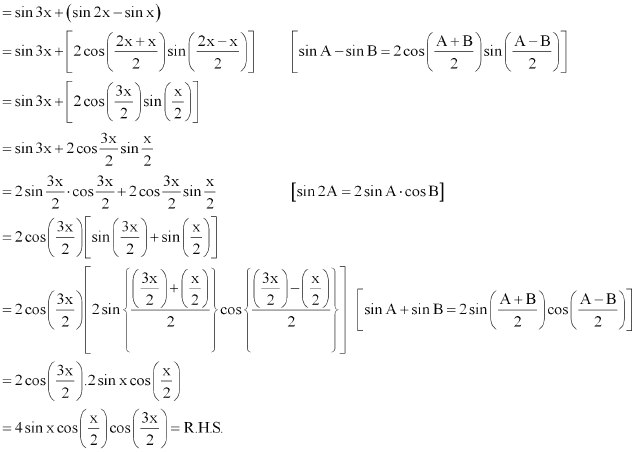
Question 8: 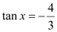 , x in quadrant II
, x in quadrant II
ANSWER : Here, x is in quadrant II.
i.e., 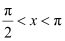
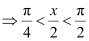
Therefore, 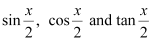 are all positive.
are all positive.
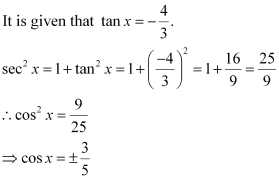
As x is in quadrant II, cosx is negative.
∴ 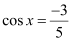
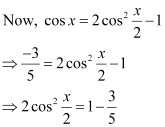
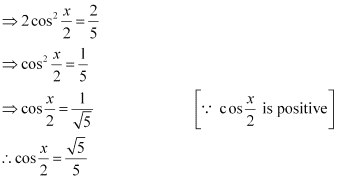
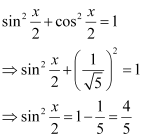
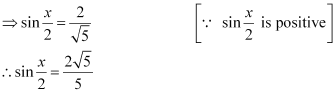
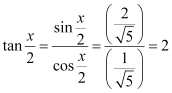
Thus, the respective values of 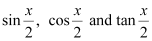 are
are 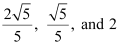 .
.
Question 9: Find 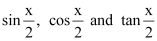 for
for 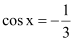 , x in quadrant III
, x in quadrant III
ANSWER : Here, x is in quadrant III.
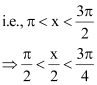
Therefore, 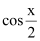 and
and 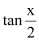 are negative, whereas
are negative, whereas 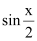 is positive.
is positive.
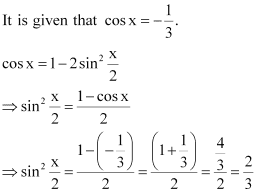
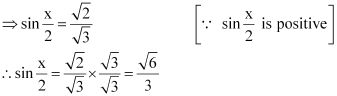
Now, 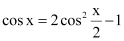
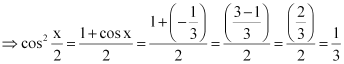
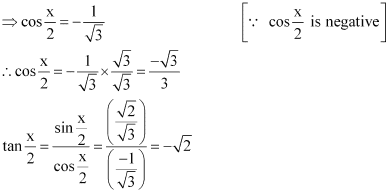
Thus, the respective values of  are
are 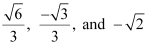
.
Question 10: Find  for
for 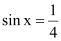 , x in quadrant II
, x in quadrant II
ANSWER : Here, x is in quadrant II.
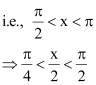
Therefore, 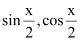 , and
, and 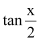 are all positive.
are all positive.
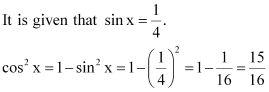
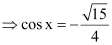 [cosx is negative in quadrant II]
[cosx is negative in quadrant II]
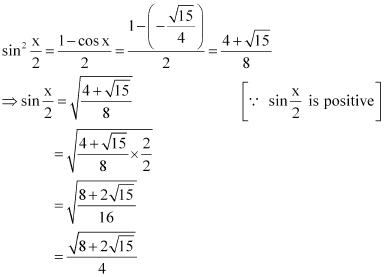
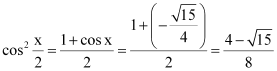
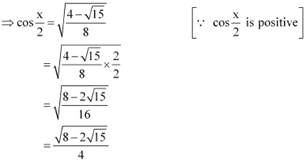
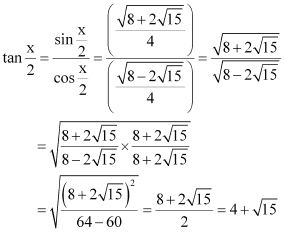
Thus, the respective values of 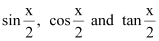 are
are 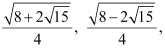 .
.
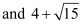
|
75 videos|238 docs|91 tests
|
FAQs on NCERT Solutions Class 11 Maths Chapter 3 - Trigonometric Functions
| 1. What are trigonometric functions? |  |
| 2. How are trigonometric functions used in real-life applications? |  |
| 3. What is the unit circle and how is it related to trigonometric functions? |  |
| 4. How can I find the values of trigonometric functions for specific angles? |  |
| 5. Are there any important identities or formulas related to trigonometric functions? |  |

|
Explore Courses for Commerce exam
|

|
















