Exercise Miscellaneous - Linear Inequalities NCERT Solutions | Mathematics (Maths) Class 11 - Commerce PDF Download

Q1: Solve the inequalities: 2 ≤ 3x – 4 ≤ 5
Ans: According to the question,
The inequality given is,
2 ≤ 3x – 4 ≤ 5
⇒ 2 ≤ 3x – 4 ≤ 5
⇒ 2 + 4 ≤ 3x – 4 + 4 ≤ 5 + 4
⇒ 6 ≤ 3x ≤ 9
⇒ 6/3 ≤ 3x/3 ≤ 9/3
⇒ 2 ≤ x ≤ 3
Hence, all real numbers x greater than or equal to 2, but less than or equal to 3 are solutions of given equality.
x ∈ [2, 3]

Q2: Solve the inequalities: 6 ≤ –3 (2x – 4) < 12
Ans: According to the question,
The inequality given is,
6 ≤ –3 (2x – 4) < 12
⇒ 6 ≤ -3 (2x – 4) < 12
Dividing the inequality by 3, we get.
⇒ 2 ≤ – (2x – 4) < 4
Multiplying the inequality by -1,
⇒ -2 ≥ 2x – 4 > -4 [multiplying the inequality with -1 changes the inequality sign.]
⇒ -2 + 4 ≥ 2x – 4 + 4 > -4 + 4
⇒ 2 ≥ 2x > 0
Dividing the inequality by 2,
⇒ 0 < x ≤ 1
Hence, all real numbers x greater than 0, but less than or equal to 1 are solutions of given equality.
x ∈ (0, 1]

Q3: Solve the inequalities: – 3 ≤ 4 – 7x/2 ≤ 18
Ans: According to the question,
The inequality given is,
– 3 ≤ 4 – 7x/2 ≤ 18
⇒ – 3 – 4 ≤ 4 – 7x/2 – 4 ≤ 18 – 4
⇒ – 7 ≤ – 7x/2 ≤ 18 – 14
Multiplying the inequality by -2,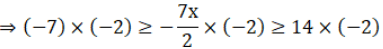

⇒ 14 ≥ 7x ≥ -28
⇒ -28 ≤ 7x ≤ 14
Dividing the inequality by 7,
⇒ -4 ≤ x ≤ 2
Hence, all real numbers x greater than or equal to -4, but less than or equal to 2 are solutions of given equality.
x ∈ [-4, 2]

Q4: Solve the inequalities: – 15 ≤ 3(x – 2)/5 ≤ 0
Ans: According to the question,
The inequality given is,
– 15 ≤ 3(x – 2)/5 ≤ 0
⇒ – 15 < 3(x – 2)/5 ≤ 0
Multiplying the inequality by 5,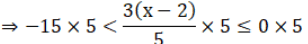

⇒ -75 < 3(x – 2) ≤ 0

Dividing the inequality by 3, we get,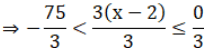

⇒ -25 < x – 2 ≤ 0
⇒ – 25 + 2 < x – 2 + 2 ≤ 0 + 2
⇒ – 23 < x ≤ 2
Hence, all real numbers x greater than -23, but less than or equal to 2 are solutions of given equality.
x ∈ (-23, 2]

Q5: Solve the inequalities: – 12 < 4 – 3x/ (-5) ≤ 2
Ans: According to the question,
The inequality given is,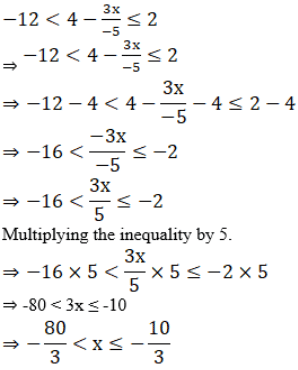

Hence, all real numbers x greater than -80/3, but less than or equal to -10/3 are solutions of given equality.
x ∈ (-80/3, -10/3]

Q6: Solve the inequalities: 7 ≤ (3x + 11)/2 ≤ 11
Ans: According to the question,
The inequality given is,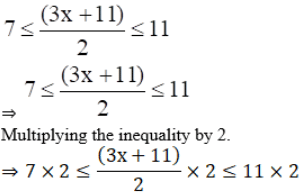

⇒ 14 ≤ 3x + 11 ≤ 22
⇒ 14 – 11 ≤ 3x + 11 – 11 ≤ 22 – 11
⇒ 3 ≤ 3x ≤ 11
⇒ 1 ≤ x ≤ 11/3
Hence, all real numbers x greater than or equal to -4, but less than or equal to 2 are solutions of given equality.
x ∈ [1, 11/3].

Solve the inequalities in Q. 7 to 11 and represent the solution graphically on number line.
Q7: 5x + 1 > – 24, 5x – 1 < 24
Ans: According to the question,
The inequalities given are,
5x + 1 > -24 and 5x – 1 < 24
5x + 1 > -24
⇒ 5x > -24 – 1
⇒ 5x > -25
⇒ x > -5 ……… (i)
5x – 1 < 24
⇒ 5x < 24 + 1
⇒ 5x < 25
⇒ x < 5 ……….(ii)
From equations (i) and (ii),
We can infer that the solution of given inequalities is (-5, 5).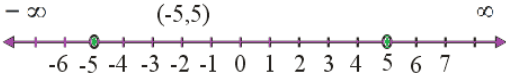


Q8: 2 (x – 1) < x + 5, 3 (x + 2) > 2 – x
Ans: According to the question,
The inequalities given are,
2 (x – 1) < x + 5 and 3 (x + 2) > 2 – x
2 (x – 1) < x + 5
⇒ 2x – 2 < x + 5
⇒ 2x – x < 5 + 2
⇒ x < 7 ……… (i)
3 (x + 2) > 2 – x
⇒ 3x + 6 > 2 – x
⇒ 3x + x > 2 – 6
⇒ 4x > -4
⇒ x > -1 ………. (ii)
From equations (i) and (ii),
We can infer that the solution of given inequalities is (-1, 7).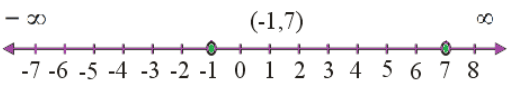


Q9: 3x – 7 > 2(x – 6), 6 – x > 11 – 2x
Ans: According to the question,
The inequalities given are,
3x – 7 > 2(x – 6) and 6 – x > 11 – 2x
3x – 7 > 2(x – 6)
⇒ 3x – 7 > 2x – 12
⇒ 3x – 2x > 7 – 12
⇒ x > -5 ………… (i)
6 – x > 11 – 2x
⇒ 2x – x > 11 – 6
⇒ x > 5 ……….(ii)
From equations (i) and (ii),
We can infer that the solution of given inequalities is (5, ∞).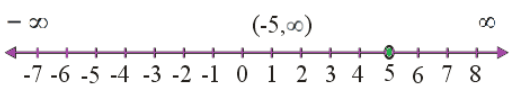


Q10: 5(2x – 7) – 3(2x + 3) ≤ 0, 2x + 19 ≤ 6x + 47
Ans: According to the question,
The inequalities given are,
5(2x – 7) – 3(2x + 3) ≤ 0 and 2x + 19 ≤ 6x + 47
5(2x – 7) – 3(2x + 3) ≤ 0
⇒ 10x – 35 – 6x – 9 ≤ 0
⇒ 4x – 44 ≤ 0
⇒ 4x ≤ 44
⇒ x ≤ 11 ……(i)
2x + 19 ≤ 6x +47
⇒ 6x – 2x ≥ 19 – 47
⇒ 4x ≥ -28
⇒ x ≥ -7 ……….(ii)
From equations (i) and (ii),
We can infer that the solution of given inequalities is (-7, 11).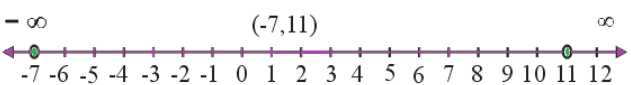


Q11: A solution is to be kept between 68° F and 77° F. What is the range in temperature in degree Celsius (C) if the Celsius / Fahrenheit (F) conversion formula is given by F = (9/5) C + 32?
Ans: According to the question,
The solution has to be kept between 68° F and 77° F
So, we get, 68° < F < 77°
Substituting,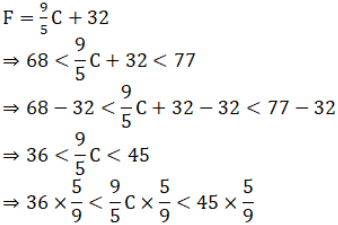

⇒ 20 < C < 25
Hence, we get,
The range of temperature in degree Celsius is between 20° C to 25° C.

Q12: A solution of 8% boric acid is to be diluted by adding a 2% boric acid solution to it. The resulting mixture is to be more than 4%, but less than 6% boric acid. If we have 640 litres of the 8% solution, how many litres of the 2% solution will have to be added?
Ans: According to the question,
8% of solution of boric acid = 640 litres
Let the amount of 2% boric acid solution added = x litres
Then we have,
Total mixture = x + 640 litres
We know that,
The resulting mixture has to be more than 4% but less than 6% boric acid.
∴ 2% of x + 8% of 640 > 4% of (x + 640) and
2% of x + 8% of 640 < 6% of (x + 640)
2% of x + 8% of 640 > 4% of (x + 640)
⇒ (2/100) × x + (8/100) × 640 > (4/100) × (x + 640)
⇒ 2x + 5120 > 4x + 2560
⇒ 5120 – 2560 > 4x – 2x
⇒ 2560 > 2x
⇒ x < 1280 ….(i)
2% of x + 8% of 640 < 6% of (x + 640)
⇒ (2/100) × x + (8/100) × 640 < (6/100) × (x + 640)
⇒ 2x + 5120 < 6x + 3840
⇒ 6x – 2x > 5120 – 3840
⇒ 4x > 1280
⇒ x > 320 ……….(i)
From (i) and (ii)
320 < x < 1280
Therefore, the number of litres of 2% of boric acid solution that has to be added will be more than 320 litres but less than 1280 litres.

Q13: How many litres of water will have to be added to 1125 litres of the 45% solution of acid so that the resulting mixture will contain more than 25% but less than 30% acid content?
Ans: According to the question,
45% of solution of acid = 1125 litres
Let the amount of water added = x litres
Resulting mixture = x + 1125 litres
We know that,
The resulting mixture has to be more than 25% but less than 30% acid content.
Amount of acid in resulting mixture = 45% of 1125 litres.
∴ 45% of 1125 < 30% of (x + 1125) and 45% of 1125 > 25% of (x + 1125)
45% of 1125 < 30% of (x + 1125)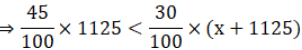

⇒ 45 × 1125 < 30x + 30 × 1125
⇒ (45 – 30) × 1125 < 30x
⇒ 15 × 1125 < 30x
⇒ x > 562.5 ………..(i)
45% of 1125 > 25% of (x + 1125)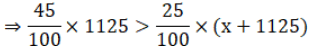

⇒ 45 × 1125 > 25x + 25 × 1125
⇒ (45 – 25) × 1125 > 25x
⇒ 25x < 20 × 1125
⇒ x < 900 …..(ii)
∴ 562.5 < x < 900
Therefore, the number of litres of water that has to be added will have to be more than 562.5 litres but less than 900 litres.

Q14: IQ of a person is given by the formula
Where MA is mental age and CA is chronological age. If 80 ≤ IQ ≤ 140 for a group of 12-year-old children, find the range of their mental age.
Ans: According to the question,
Chronological age = CA = 12 years
IQ for age group of 12 is 80 ≤ IQ ≤ 140.
We get that,
80 ≤ IQ ≤ 140
Substituting,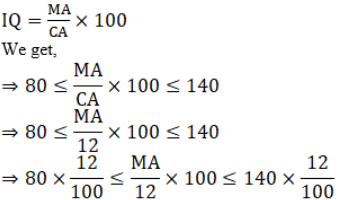

⇒ 9.6 ≤ MA ≤ 16.8
∴ Range of mental age of the group of 12 year-old-children is 9.6 ≤ MA ≤ 16.8.