NCERT Solutions for Class 8 Maths Chapter 9 - Mensuration - 2 (Exercise 9.2)
Surface Area of Cube, Cuboid and Cylinder
Cube: A cube has 6 faces, all of which are congruent squares.
| Total surface area of a cube having length of one side as ‘l’ = 6l2 |
Remember
- For a cube, length, breadth and height are all equal.
- If the length of the edge of a cube is doubled, it surface area increases four times
Cuboid: A cuboid too have 6 faces but they may or may not be equal. All the six faces are rectangular and the opposite faces are identical, Thus, in a cuboid, we get 3 pairs of identical faces.
For a cuboid having length, breadth and height as l, b and h respectively, we have:
| Total surface area = 2(lb + bh + hl) |
Note: Area of 4 walls of a room = [perimeter of the room] *height of the room
Cylinder: A cylinder has three surfaces: (i) curved surface, (ii) base surface and (iii) Top surface.
Note:
- The top and bottom (base) surfaces are circular.
- The top surface and bottom surface are identical.
| Total surface area of a cylinder = 2πr[h + r] |
Remember
If the cylinder is open then the total surface area is equal to the curved surface area of the cylinder
Exercise 9.2
Q1. There are two cuboidal boxes as shown in the adjoining figure. Which box requires the lesser amount of material to make?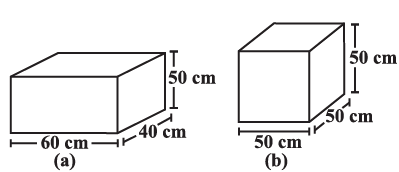
Ans:
(a) Given: Length of cuboidal box (l) = 60 cm
Breadth of cuboidal box (b) = 40 cm
Height of cuboidal box (h) = 50 cm
Total surface area of cuboidal box = 2 × (lb + bh + hl)
= 2 × (60 × 40 + 40 × 50 + 50 × 60)
= 2 × (2400 + 2000 + 3000)
= 14800 cm2
(b) Length of cubical box (l) = 50 cm
Breadth of cubicalbox (b) = 50 cm
Height of cubicalbox (h) = 50 cm
Total surface area of cubical box = 6(side)2
= 6(50 × 50)
= 6 × 2500
= 15000
Surface area of the cubical box is 15000 cm2
From the result of (a) and (b), cuboidal box requires the lesser amount of material to make.
Q2. A suitcase with measures 80 cm × 48 cm × 24 cm is to be covered with a tarpaulin cloth. How many metres of tarpaulin of width 96 cm is required to cover 100 such suitcases?
Ans: Length of suitcase box, l = 80 cm,
Breadth of suitcase box, b= 48 cm
And Height of cuboidal box , h = 24 cm
Total surface area of suitcase box = 2(lb+bh+hl)
= 2(80 × 48 + 48 × 24 + 24 × 80)
= 2 (3840 + 1152 + 1920)
= 2 × 6912
= 13824
Total surface area of suitcase box is 13824 cm2
Area of Tarpaulin cloth = Surface area of suitcase
l × b = 13824
l × 96 = 13824
l = 144
Required tarpaulin for 100 suitcases = 144 × 100 = 14400 cm = 144 m
Hence tarpaulin cloth required to cover 100 suitcases is 144 m.
Q3. Find the side of a cube whose surface area is 600 cm2.
Ans: Surface area of cube = 600 cm2 (Given)
Formula for surface area of a cube = 6(side)2
Substituting the values, we get
6(side)2 = 600
(side)2 = 100
Or side = ±10
Since side cannot be negative, the measure of each side of a cube is 10 cm
Q4. Rukhsar painted the outside of the cabinet of measure 1 m × 2 m × 1.5 m. How much surface area did she cover if she painted all except the bottom of the cabinet.
Ans: Length of cabinet, l = 2 m, Breadth of cabinet, b = 1 m and Height of cabinet, h = 1.5 m
Area painted = Total surface area of the cabinet – Area of bottom
Total surface area of the cabinet = 2(lb + bh + hl )
= 2(2 × 1 + 1 × 1.5 + 1.5 × 2)
= 2(2 + 1.5 + 3.0)
= 13 m2
Area of bottom = Length × Breadth
= 2 × 1
= 2 m2
Area painted = 13 – 2 = 11 m2
The required surface area of the cabinet is 11m2.
Q5. Daniel is painting the walls and ceiling of a cuboidal hall with length, breadth and height of 15 m, 10 m and 7 m respectively. From each can of paint 100 m2 of area is painted. How many cans of paint will she need to paint the room?
Ans: Area to be painted = [Area of 4-walls] + Area of ceiling
= 2[Perimeter × Height] + l × b
= 2[(15 + 10) × 7] + 15 × 10 m2
= 2[25 × 7] + 150 m2
= 2 × 175 + 150 m2
350 + 150 m2 = 500 m2
Since 1 can of paint covers 100 m2 area.
∴ Number of cans required = 500/100 = 5
Q6. Describe how the two figures at the right are alike and how they are different. Which box has larger lateral surface area?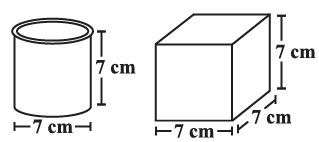 Ans:
Ans:
Similarity
Both figures have the same length and the same height
Difference
The first figure has circular bottom and top
The second figure has square bottom and top
The first figure is a cylinder and the second figure is a cube
Diameter of cylinder = 7 cm (Given)
Radius of cylinder, r = 7/2 cm
Height of cylinder, h = 7 cm
Lateral surface area of cylinder = 2πrh
= 2 × (22/7) × (7/2) × 7 = 154
So, Lateral surface area of cylinder is 154 cm2
Now, lateral surface area of cube = 4 (side)2 =4 × 72 = 4 × 49 = 196
Lateral surface area of cube is 196 cm2
Hence, the cube has a larger lateral surface area.
Q7. A closed cylindrical tank of radius 7 m and height 3 m is made from a sheet of metal. How much sheet of metal is required?
Ans: 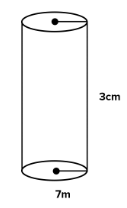 Radius of cylindrical tank, r = 7 m
Radius of cylindrical tank, r = 7 m
Height of cylindrical tank , h = 3 m
Total surface area of cylindrical tank = 2πr(h+r)
= 2 × (22/7) × 7(3 + 7)
= 44 × 10 = 440
Therefore, 440 m2 metal sheet is required.
Q8. The lateral surface area of a hollow cylinder is 4224 cm2. It is cut along its height and formed a rectangular sheet of width 33 cm. Find the perimeter of rectangular sheet?
Ans: Lateral surface area of hollow cylinder = 4224 cm2
Width of rectangular sheet = 33 cm and say l be the length of the rectangular sheet
Lateral surface area of cylinder = Area of the rectangular sheet
4224 = b × l
4224 = 33 × l
l = 4224/33 = 128 cm
So the length of the rectangular sheet is 128 cm.
Also, Perimeter of rectangular sheet = 2(l + b)
= 2(128 + 33)
= 322 cm
The perimeter of the rectangular sheet is 322 cm.
Q9. A road roller takes 750 complete revolutions to move once over to level a road. Find the area of the road if the diameter of a road roller is 84 cm and length is 1 m. Ans: Diameter of road roller, d = 84 cm
Ans: Diameter of road roller, d = 84 cm
Radius of road roller, r = d/2 = 84/2 = 42 cm
Length of road roller, h = 1 m = 100 cm
Formula for Curved surface area of road roller = 2πrh
= 2 × (22/7) × 42 × 100 = 26400
Curved surface area of the road roller is 26400 cm2
Again, Area covered by the road roller in 750 revolutions = 26400 × 750cm2
= 1,98,00,000cm2
= 1980 m2 [∵ 1 m2= 10,000 cm2]
Hence the area of the road is 1980 m2.
Q10. A company packages its milk powder in cylindrical container whose base has a diameter of 14 cm and height 20 cm. Company places a label around the surface of the container (as shown in the figure). If the label is placed 2 cm from top and bottom, what is the area of the label.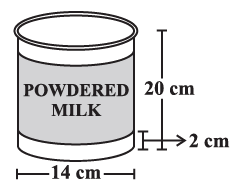 Ans: Diameter of the cylindrical container, d = 14 cm
Ans: Diameter of the cylindrical container, d = 14 cm
Radius of cylindrical container, r = d/2 = 14/2 = 7 cm
Height of cylindrical container = 20 cm
Height of the label, say h = 20 – 2 – 2 (from the figure)
= 16 cm
Curved surface area of label = 2πrh
= 2 × (22/7) × 7 × 16
= 704
Hence, the area of the label is 704 cm2.
Extra Questions
Q1. Why is it incorrect to call the solid shown here a cylinder?
Ans: We know that a cylinder has two identical (congruent) circular faces, parallel to each other. Therefore, it is incorrect to call the given solid as a cylinder, because its opposite faces are not congruent.
Q2. Find the total surface area of the following cuboids
Ans: (i) ∵ l = 6 cm, b = 4 cm and h = 2 cm
∴ Total surface area of the cuboids = 2(lb + bh + hl)
= 2(6 * 4 + 4 * 2 + 2 * 6cm2
= 2(24 + 8 + 12) cm2
= 2* 44 cm2 = 88 cm2
(ii) ∵ l = 4 cm, b = 4 cm and h = 10 cm
∴ Total surface area of the cuboid = 2(lb + bh + hl)
= 2(4*4 + 4*10 + 10*4) cm2
= 2(16 + 40 + 40) cm2
= 2*96 cm2 = 192 cm2
Q3. Can we say that the total surface area of cuboid = lateral surface area + 2 * area of base
Ans: Yes, or a cuboid,
Total surface area = Lateral surface area + 2 * Area of the base [∵ Opposite faces are congruent]
Q4. If we interchange the lengths of the base and the height of a cuboid [Fig. (i)] to get another cuboid [Fig. (ii)], will its lateral surface area change?
Solution: Lateral surface area of figure (i) = 2(l + b) * h
Lateral surface area of figure (ii) = 2(h + b) * l
Since, the two results are different.
∴ By changing the position of a cuboid, its lateral surface will change.
Q5. Find the surface area of cube A and lateral surface area of cube B.
Ans: For cube A:
Side (l) = 10 cm
∴ Total surface area of the cube = 6l2
= 6 * (10)2 cm2 = 600 cm2
For cube B:
Side (l) = 8 cm
∴ Total surface area of the cube = 6l2
= 6 * (8)2 cm2
= 6 * 64 cm2 = 256 cm2
Q6. Two cubes each with side b are joined to form a cuboid figure. What is the surface area of this cuboid? Is it 12b2? Is the surface area of cuboid formed by joining three such cubes, 18b2? Why?
Ans: When 2 cubes are joined end to end:
Length (L) = b + b = 2b
Breadth (B) = b
Height (H) = b (∵ Height and breadth do not chang.)
∴ Total surface area = 2[LB + BH + HL]
= 2[(2b * b) + (b * b) + (b * 2b)]
= 2[2b2 + b2 + 2b2]
= 2[5b2] = 10b2
∴ The total surface area is not 12b2.
When 3 cubes are joined end to end:
Length (L) = 3b
Breadth (B) = b
Height (H) = b (Here too breadth and height do not change.)
∴ Total surface area of the cuboid = 2[LB + BH + HL]
= 2[(3b * b) + (b * b) + (b * 3b)]
= 2[3b2 + b2 + 3b2]
= 2[7b2] = 14b2
∴ The surface area is not 18b2.
Q7. How will you arrange 12 cubes of equal length to form a cuboid of smallest surface area?
Ans: Case I
Length (L) = 12b
Breadth (B) = b
Height (H) = b
∴ Total surface area of the cuboid = 2[LB + BH + HL]
= 2(12b * b) + (b * b) + (b * 12b)]
= 2[12b2 + b2 + 12b2]
= 2[25b2] = 50b2
Case II
Length (L) = 6b
Breadth (B) = 2b
Height (H) = b
∴ Surface area of the cuboid = 2[LB + BH + HL]
= 2[(6b * 2b) + (2b * b) + (b * 6b)]
= 2[12b2 + 2b2 + 6b2]
= 2[20b2] = 40b2
Case III
Length (L) = 3b
Breadth (B) = 2b
Height (H) = 2b
∴ Surface area of the cuboid = 2(LB + BH + HL)
= 2[(3b * 2b) + (2b * 2b) + (2b * 3b)
= 2[6b2 + 4b2 + 6b2]
= 2[16b2] = 32b2
Obviously, the case III arrangement of 12 cubes, has the minimum surface area.
Q8. After the surface area of cube is painted, the cube is cut into 64 smaller cubes of same dimensions. How many have no face painted? 1 face painted? 2 faces painted? 3 faces painted?
Ans: In the given figure, we have:
(a) Number of cubes having no face painted = 16
(b) Number of cubes having 1 face painted = 16
(c) Number of cubes having 2 faces painted = 24
(d) Number of cubes having 3 faces painted = 8
Q9. Find total surface area of the following cylinders.
Ans:
(i) We have
Radius (r) = 14 cm
Height (h) = 8 cm
∴ Total surface area = 2πr(r + h)
(ii) We have r = 2/2 1 m and h = 2 m
∴ Total surface area = 2πr(r + h)
|
276 docs|155 tests
|

















