NCERT Solutions for Class 9 Maths Chapter 8 - Quadrilaterals (Exercise 8.2)
Q1. ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA (see Fig). AC is a diagonal.
Show that:
(i) SR || AC and SR = (1/2) AC
(ii) PQ = SR
(iii) PQRS is a parallelogram.

Ans: We have P as the mid-point of AB, Q as the mid-point of BC, R as the mid-point of CD, S as the mid-point of DA, and AC as the diagonal of quadrilateral ABCD.
(i) To prove that SR =(1/2) AC and SR || AC.
In ΔACD, we have
S as the mid-point of AD, R as the mid-point of CD.
∵ The line segment joining the mid-point of any two sides of a triangle is parallel to the third side and half of it.
∴ SR = (1/2)AC and SR || AC
(ii) To prove that PQ = SR.
In ΔABC, we have P is the mid-point of AB, Q is the mid-point of BC.
∴ PQ = (1/2) AC …(1)
Also, SR = (1/2) AC [Proved] …(2)
From (1) and (2), PQ = SR
(iii) To prove that PQRS is a parallelogram.
In ΔABC, P and Q are the mid-points of AB and BC.
∴ PQ = (1/2)AC and PQ || AC ...(3)
In ΔACD, S and R are the mid-points of DA and CD.
∴ SR = (1/2)AC and SR || AC …(4)
From (3) and (4), we get
PQ =(1/2)AC = SR and PQ || AC || SR
⇒ PQ = SR and PQ || SR
i.e. One pair of opposite sides in quadrilateral PQRS is equal and parallel.
∴ PQRS is a parallelogram.
Q2. ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
Ans: We have P as the mid-point of AB, Q as the midpoint of BC, R as the mid-point of CD, S as the mid-point of DS.

We have to prove that PQRS is a rectangle.
Let us join AC.
∵ In ΔABC, P and Q are the mid-points of AB and BC.
∴ PQ =(1/2)AC and PQ || AC …(1)
Also in ΔADC, R and S are the mid-points of CD and DA.
∴ SR = (1/2)AC and SR || AC
From (1) and (2), we get
PQ =(1/2) AC = SR and PQ || AC || SR
⇒ PQ = SR and PQ || SR
i.e. One pair of opposite sides of quadrilateral PQRS is equal and parallel.
∴ PQRS is a parallelogram.
Now, in ΔERC and ΔEQC, ∠1= ∠2 [∵ The diagonal of a rhombus bisects the opposite angles]
CR = CQ [Each is equal to(1/2) of a side of rhombus]CE = CE [Common]
∴ ΔERC ≌ ΔEQC [SAS criteria]
⇒ ∠3= ∠4[c.p.c.t.]
But ∠3 + ∠4 = 180º [Linear pair] ⇒ ∠3= ∠4 = 90°
But ∠5= ∠3 [Vertically opposite angles]
∴ ∠5 = 90º PQ || AC
⇒ PQ || EF
∴ PQEF is a quadrilateral having a pair of opposite sides parallel and one of the angles is 90º.
∴ PQEF is a rectangle.
⇒ ∠RQP = 90º
∴ One angle of parallelogram PQRS is 90º.
Thus, PQRS is a rectangle.
Question 3. ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
Ans: 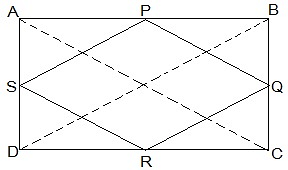 Given in the question,
Given in the question,
ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA, respectively.
Construction,
Join AC and BD.
To Prove,
PQRS is a rhombus.
Proof:
In ΔABC
P and Q are the mid-points of AB and BC, respectively
, PQ || AC and PQ = ½ AC (Midpoint theorem) — (i)
In ΔADC,
SR || AC and SR = ½ AC (Midpoint theorem) — (ii)
So, PQ || SR and PQ = SR
As in quadrilateral PQRS one pair of opposite sides is equal and parallel to each other, so, it is a parallelogram.
, PS || QR and PS = QR (Opposite sides of parallelogram) — (iii)
Now,
In ΔBCD,
Q and R are mid points of side BC and CD, respectively.
, QR || BD and QR = ½ BD (Midpoint theorem) — (iv)
AC = BD (Diagonals of a rectangle are equal) — (v)
From equations (i), (ii), (iii), (iv) and (v),
PQ = QR = SR = PS
So, PQRS is a rhombus.
Hence Proved
Q4. ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F (see Fig). Show that F is the mid-point of BC.
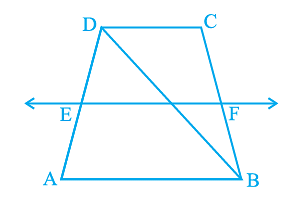
Ans: Given that,
ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD.
To prove,
F is the mid-point of BC.
Proof,
BD intersected EF at G.
In ΔBAD,
E is the mid point of AD and also EG || AB.
Thus, G is the mid point of BD (Converse of mid point theorem)
Now,
In ΔBDC,
G is the mid point of BD and also GF || AB || DC.
Thus, F is the mid point of BC (Converse of mid point theorem)
Q5. In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively (see Fig). Show that the line segments AF and EC trisect the diagonal BD.
Ans: We have ABCD is a parallelogram such that: E is the mid-point of AB and F is the mid-point of CD.

Let us join the opposite vertices B and D.
Since, the opposite sides of a parallelogram are parallel and equal.
∴ AB || DC ⇒ AE || FC …(1)
Also AB = DC
or (1/2) AB =(1/2)DC ⇒ AE = FC …(2)
From (1) and (2),
we can say that AECF is quadrilateral having a pair of the opposite sides as parallel and equal.
∴ AEFC is a parallelogram.
⇒ AE || CF
Now, in DABC, F is the mid-point of DC [Given]
and FP || CQ [∵ AF || CE]
⇒ P is the mid-point of DQ [Converse of mid-point theorem]
⇒ DP = PQ …(3)
Similarly, in DBAP, BQ = PQ …(4)
∴ From (3) and (4), we have
DP = PQ = BQ
⇒ The line segments AF and EC trisect the diagonal BD.
Q6. ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that
(i) D is the mid-point of AC
(ii) MD ⊥ AC
(iii) CM = MA = 1/2 AB
Ans: We have a triangle ABC, such that ∠C = 90º M is the mid-point of AB and MD || BC
 (i) To prove that D is the mid-point of AC.
(i) To prove that D is the mid-point of AC.
In ΔACB, we have M as the mid-point of AB. [Given]
MD || BC [Given]
∴ Using the converse of mid-point theorem, D is the mid-point of AC.
(ii) To prove that MD ⊥ AC.
Since, MD || BC [Given] and AC is a transversal.
∴ ∠ MDA = ∠BCA [Corresponding angles]
But ∠BCA = 90º [Given]
∴ ∠MDA = 90º ⇒ MD ⊥ AC.
(iii) To prove that CM = MA =(1/2) AB
In ΔADM and ΔCDM, we have
∠ADM = ∠CDM [Each = 90º]
MD = MD [Common]
AD = CD [∵ M is the mid-point of AC (Proved)]
∴ ΔADM ≌ ΔCOM [SAS criteria]
⇒ MA = MC [c.p.c.t.] …(1)
∵ M is the mid-point AB. [Given]
∴ MA =(1/2)AB …(2)
From (1) and (2), we have
CM = MA = (1/2) AB
|
5 videos|378 docs|164 tests
|
FAQs on NCERT Solutions for Class 9 Maths Chapter 8 - Quadrilaterals (Exercise 8.2)
| 1. What are the properties of a quadrilateral? |  |
| 2. How many diagonals does a quadrilateral have? |  |
| 3. What are the types of quadrilaterals? |  |
| 4. How can we determine if a quadrilateral is a parallelogram? |  |
| 5. What is the formula to find the area of a quadrilateral? |  |

|
Explore Courses for CAT exam
|

|


















