Navier Stokes Equations | Fluid Mechanics for Mechanical Engineering PDF Download
A general way of deriving the Navier-Stokes equations from the basic laws of physics
- Consider a general flow field as represented in Fig. 25.1.
- Imagine a closed control volume,
 within the flow field. The control volume is fixed in space and the fluid is moving through it. The control volume occupies reasonably large finite region of the flow field.
within the flow field. The control volume is fixed in space and the fluid is moving through it. The control volume occupies reasonably large finite region of the flow field. - A control surface ,
 is defined as the surface which bounds the volume
is defined as the surface which bounds the volume  .
. - According to Reynolds transport theorem, "The rate of change of momentum for a system equals the sum of the rate of change of momentum inside the control volume and the rate of efflux of momentum across the control surface".
- The rate of change of momentum for a system (in our case, the control volume boundary and the system boundary are same) is equal to the net external force acting on it.
Now, we shall transform these statements into equation by accounting for each term,

Rate of change of momentum inside the control volume
 (25.1)
(25.1)
 (25.2)
(25.2)
 (25.3)
(25.3)
- Body force acting on the control volume

 is the body force per unit mass.
is the body force per unit mass.
- Finally, we get,

or
 ( 25.5)
( 25.5)
We know that  is the general form of mass conservation equation (popularly known as the continuity equation), valid for both compressible and incompressible flows.
is the general form of mass conservation equation (popularly known as the continuity equation), valid for both compressible and incompressible flows.
- Invoking this relationship in Eq. (25.5), we obtain

- Equation (25.6) is referred to as Cauchy's equation of motion in equation
 is the stress tensor
is the stress tensor - After having substituted
 we get
we get
 (25.8)
(25.8)
From Stokes's hypothesis we get, (25.9)
(25.9)
Invoking above two relationships into Eq.( 25.6) we get
 (25.10)
(25.10)
This is the most general form of Navier-Stokes equation.
Exact Solutions Of Navier-Stokes Equations
Consider a class of flow termed as parallel flow in which only one velocity term is nontrivial and all the fluid particles move in one direction only.
- We choose
 to be the direction along which all fluid particles travel , i.e.
to be the direction along which all fluid particles travel , i.e.  . Invoking this in continuity equation, we get
. Invoking this in continuity equation, we get


- Now. Navier-Stokes equations for incompressible flow become

So, we obtain
 (25.11)
(25.11)
|
56 videos|104 docs|75 tests
|
FAQs on Navier Stokes Equations - Fluid Mechanics for Mechanical Engineering
| 1. What are the Navier-Stokes equations? |  |
| 2. How are the Navier-Stokes equations derived? |  |
| 3. What are the applications of the Navier-Stokes equations in mechanical engineering? |  |
| 4. Are the Navier-Stokes equations solvable for all fluid flow problems? |  |
| 5. What are the limitations of the Navier-Stokes equations? |  |
|
56 videos|104 docs|75 tests
|

|
Explore Courses for Mechanical Engineering exam
|

|
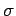
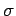
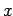 to be the direction along which all fluid particles travel , i.e.
to be the direction along which all fluid particles travel , i.e. 
















