Olympiad Notes: Ratio and Proportion | Maths Olympiad Class 6 PDF Download
| Table of contents |

|
| Introduction |

|
| Ratio |

|
| Proportion |

|
| Unitary Method |

|
| Practice Questions |

|
Introduction
- When we want to compare quantities of the same type, we have different ways to do it.
- Subtracting one quantity from another is a common method, but dividing them is often more insightful.
- Division helps us see how many times one quantity can fit into another.
- By using ratios and proportions, we can systematically explore these relationships in a way that's easy to understand.
Ratio
- If we compare two quantities using division then it is called ratio.
- It compares quantities in terms of ‘How many times’.
- The symbol to represent ratio is “:”.

- It reads as “4 is to 3”
- It can also be written as 4/3.
Example: If there are 35 boys and 25 girls in a class, then what is the ratio of
(i) Number of boys to total students
(ii) Number of girls to total students.
Sol: In the ratio, we want the total number of students.
Total number of students = Number of boys + Number of girls = 35 + 25 = 60
(i) Ratio of number of boys to total number of students
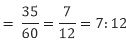
(ii) The ratio of the number of girls to the total number of students
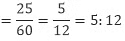
The unit must be same to compare two quantities
- To compare two quantities with different units, it's important to make them comparable.
- This involves converting both quantities into the same unit.
- Once they're in the same unit, you can easily compare them using a ratio.
- This helps ensure a fair and accurate comparison between the quantities.
Example: What is the ratio of the height of Raman and Radha if the height of Raman is 175 cm and Radha is 1.35 m?
Sol: The unit of the height of Raman and Radha is not same so convert them in the same unit.
Height of Radha is 1.35 m = 1.35 × 100 cm = 135 cm
The ratio of the height of Raman and Radha

Equivalent Ratios
- Multiplying or dividing both the numerator and denominator of a ratio by the same number results in an equivalent ratio.
- Equivalent ratios can have different numerical values but represent the same proportion.
- These ratios depict the same portion or fraction of the total quantity, despite their numerical differences.
Example: Find two equivalent ratios of 2/4.
Sol: 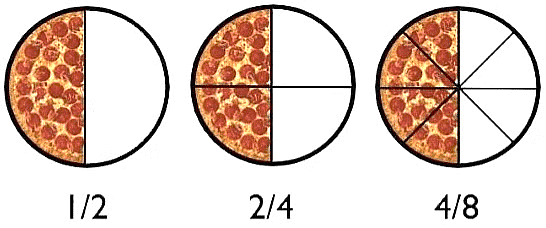
To get the equivalent ratio we multiply both the numerator and denominator with 2.
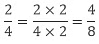
To get another equivalent ratio we divide both the numerator and denominator with 2.
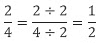
From the above figure, we can see that in all the equivalent ratios only the number of equal parts is changing but all the ratios are representing the half part of the circle only.
The Lowest form of the Ratio
If there is no common factor of numerator and denominator except one, then it is the lowest form of the ratio.
Example: Find the lowest form of the ratio 25: 100.
Sol: The common factor of 25 and 100 is 25, so divide both the numerator and denominator with 25.
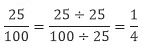
Hence the lowest ratio of 25: 100 is 1: 4.
Proportion
- When two ratios are the same, they make a proportion.
- Proportions are written as "a is to b as c is to d" or a: b = c: d.
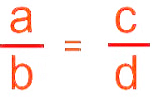
- This means you can compare quantities using proportions.
- It's a straightforward way to relate different ratios to each other.
Example: If a man runs at a speed of 20 km in 2 hours then with the same speed would he be able
to cross 40 km in 4 hours?
Sol: Here the ratio of the distances given is 20/40 = 1/2 = 1: 2
And the ratio of the time taken by them is also 2/4 = 1/2 = 1: 2
Hence the four numbers are in proportion.
We can write them in proportion as 20: 40 : : 2: 4
And reads as “20 is to 40 as 2 is to 4”.
Extreme Terms and Middle Terms of Proportion
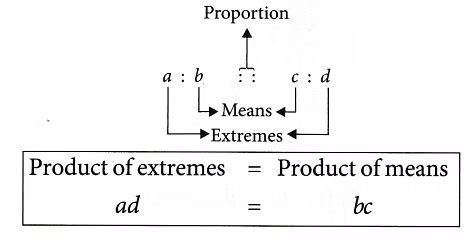
- The first and fourth terms in a proportion are referred to as the extreme terms.
- The second and third terms are known as the middle or mean terms.
- When stating a proportion, the four terms written in order are called the respective terms.
- If two ratios are not equal, they are not considered to be in proportion.
Example 1: Check whether the terms 30,99,20,66 are in proportion or not.
Sol: (i) To check the numbers are in proportion or not we have to equate the ratios.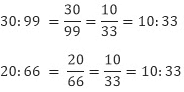
As both the ratios are equal so the four terms are in proportion, 30: 99 :: 20: 66
(ii) We can check with the product of extremes and the product of means.
In the respective terms 30, 99, 20, 66
- 30 and 66 are the extremes.
- 99 and 20 are the means.
To be in proportion the product of extremes must be equal to the product of means.
30 × 66 = 1980
99 × 20 = 1980
Here, product of extremes = product of means. So, these terms are in proportion.
Example 2: Find the ratio 30 cm to 4 m is proportion to 25 cm to 5 m or not.
Sol: As the unit is different so we have to convert them into the same unit.
4 m = 4 × 100 cm = 400 cm
The ratio of 30 cm to 400 cm is 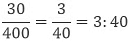 5 m = 5 × 100 cm = 500 cm
5 m = 5 × 100 cm = 500 cm
Ratio of 25 cm to 500 cm is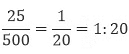 Here the two ratios are not equal so these ratios are not in proportion.3: 40 ≠ 1: 20
Here the two ratios are not equal so these ratios are not in proportion.3: 40 ≠ 1: 20
Unitary Method
- The Unitary method involves figuring out the value of one unit first.
- Then, you use this value to find the total for the required number of units.
- It's a helpful technique for solving problems involving proportions and rates.
- By dealing with one unit at a time, the Unitary method makes calculations easier to understand and manage.
Example 1: If the cost of 3 books is 320 Rs. then what will be the cost of 6 books?

Sol: Cost of 3 books = Rs. 320
Cost of 1 book = 320/3 Rs.
Cost of 6 books = (320/3) × 6 = 640 Rs.
Hence, the cost of 6 books is Rs. 640.
Q2: A car travels 90 km in 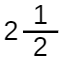 hours.
hours.
(a) How much time is required to cover 30 km with the same speed?
(b) Find the distance covered in 2 hours with the same speed.
Sol: (a) In this case, time is unknown and distance is known.
Therefore, 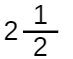 hours =
hours = 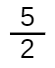 hours =
hours = 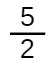 × 60 minutes = 150 minutes.
× 60 minutes = 150 minutes.
Since, 90 km is covered in 150 minutes.
1 km can be covered in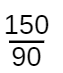 minutes.
minutes.
∴ 30 km can be covered in 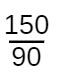 x 30 = 50 minutes.
x 30 = 50 minutes.
Thus, 30 km can be covered in 50 minutes.
(b) In this case, distance is unknown and time is known.
Distance covered in 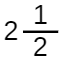 hours (5/2 hours) = 90 km
hours (5/2 hours) = 90 km
Distance covered in 1 hour = 90 ÷ 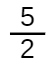 = 90 x
= 90 x 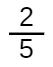 = 36 km
= 36 km
∴ Distance covered in 2 hours = 36 × 2 = 72 km.
Thus, in 2 hours, distance covered is 72 km.
Practice Questions
Q1: The cost of 5 eggs is ₹ 27.50 and cost of 5 omelettes is ₹ 60. Find the ratio of cost of one egg to the cost of one omelette.
Sol: Given, Cost of 5 eggs = ₹ 27.50
∴ Cost of 1 egg = ₹ 27.50/5 = 5.50
Cost of 5 omelettes = ₹ 60
∴ Cost of 1 omelette = ₹ 60/5 = ₹12
Now, Ratio of the cost of one egg to the cost of one omelette = Cost of one egg : Cost of one omelette
∴ Required ratio = 5.50 : 12.
Q2: The weight of three friends are in the ratio 2 : 5 : 7. If their total weight is 126 kg 350 g, then find the weight of the heaviest person.
Sol: Given,
The ratio of their weights is 2 : 5 : 7.
The total weight of the three friends is 126 kg 350 g.
Let's represent the weights of the three friends as 2x, 5x, 7x respectively, where x is a common multiplier.
According to question,
2x + 5x + 7x = 126kg 350g
14x=126kg 350g
Now, we need to convert the grams into kilograms:
126kg 350g = 126kg + 0.350kg = 126.350 kg
Solving for x:
14x=126.350kg
x = 126.350/14 kg
x ≈ 9.025kg
Finding the weights of three friends:
Weight of the 1st friend = 2 x 9.025 = 18.05kg
Weight of the 2nd friend = 5 x 9.025 = 45.125kg
Weight of the 3rd friend = 7 x 9.025 = 63.175kg
On comparing, we get weight of the 3rd friend is greater.
Hence, weight of the heaviest person = 63.175kg.
Q3: In the word BEAUTIFUL, find the ratio of number of consonants to the number of vowels.
Sol: The word BEAUTIFUL has:
(i) 4 consonants (B, T, F, L)
(ii) 4 vowels (E, A, U, I).
Required ratio = 
= 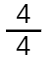
= 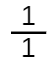
∴ Required ratio = 1:1
Q4: If the given figure is made up of a square and four identical rectangles, then find the ratio of shaded area to the area of the whole figure.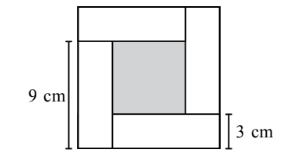
Sol: 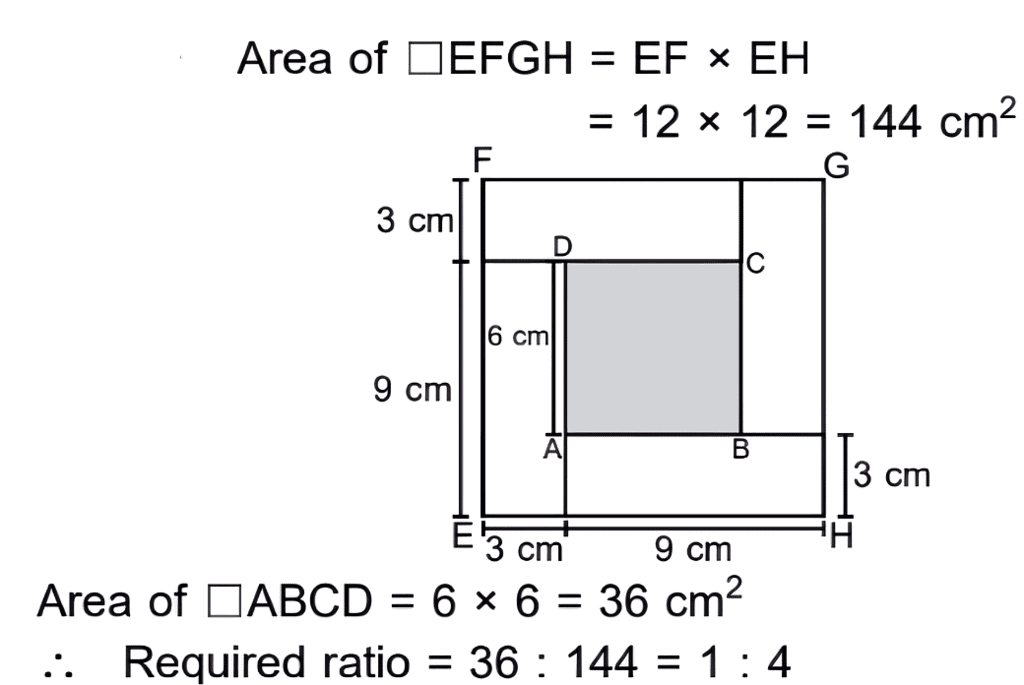
|
5 videos|117 docs|60 tests
|
FAQs on Olympiad Notes: Ratio and Proportion - Maths Olympiad Class 6
| 1. What is the difference between ratio and proportion? |  |
| 2. How can the unitary method be used to solve problems related to ratio and proportion? |  |
| 3. How can ratios be simplified or reduced to their simplest form? |  |
| 4. How are ratios and proportions used in real-life situations? |  |
| 5. Can ratios and proportions be used in geometry and algebra problems? |  |















