Percentage Change | Mathematics for Digital SAT PDF Download

The percentage change (or) the percentage change of a quantity is the ratio of the difference in the quantity to its initial value multiplied by 100. There is always a change in percentage change (or) the percent change of a quantity when the percent of its initial value is either increased or decreased to obtain its final value.

Definition of Percentage Change

Percentage Change is the difference coming after subtracting the old value from the new value and then divide by the old value and the final answer will be multiplied by 100 to show it as a percentage. Generally, to convert a fraction into a percent, we multiply it by 100.
For example, 3/4 × 100 = 75%
In the same way, we multiply the fraction (ratio) of the difference in quantity to its initial value by 100 to get the percentage change. The percentage change gives the difference in quantity out of 100.

Understanding Percentage Change with help of an example.

Robert started a business with an initial investment of Rs. 30,000 and in one year, it grew to Rs. 70,000. The growth of Robert is 40000 (70000 - 30000). Michael started a business at the same time as Robert with an initial investment of Rs. 25,000 and in one year, it grew to Rs. 65,000. The growth of Robert is 40000 (65000 - 25000). Whose business grew rapidly?
We can say that the growth value of both businesses is 40000, but the growth rate is not the same.
The growth rate should always be calculated with respect to the initial value and only then the rates can be compared. The percent change gives the difference in a quantity with respect to its initial value. It gives the growth/decay rate. This helps us in comparing the quantities.

Percentage Change Formula

The formula for the percentage change is defined as the ratio of the difference of final value and initial value to the initial value. The formula of percentage change is mathematically expressed as:

How to Calculate Percentage Change?

There are two methods to calculate the percent change. One is using the above formula and the other is using proportions.
Let us apply both methods to solve the following example.
For example, If 64 is increased to 120, find the percent change.
To perform these methods, we need to identify the initial and final values.

• Initial Value = 64
• Final Value = 120

Method 1:
(a) Subtract the initial value from the final value
Final Value - Initial Value =120 - 64 = 56
(b) Divide this by the absolute value of the initial value
Final Value - Initial Value ÷ |Initial Value| = 56 ÷ |64| = 0.875
(c) Multiply the result by 100
(Final Value - Initial Value) ÷ | Initial Value | × 100
= 0.875 × 100 = 87.5%
Since the answer is positive, it is a percent increase.
Percent Increase = 87.5%
Let's understand this process easily using the following image.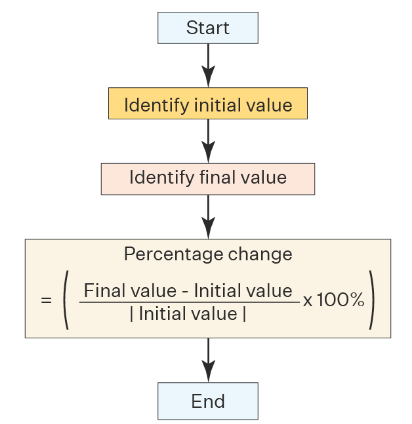

Method 2:
In this method, we assume that

• the initial value corresponds to 100%
• the final value corresponds to x%

Proportion = 64 / 100 = 120 / x

Solve for x by cross multiplication.

64x = 12000

x = 187.5%

This is the percent of the final value when compared to the initial value.

To find the percent change, we just subtract 100% from this.

Percent change = 187.5% - 100% = 87.5%
Since it is positive, it is a percent increase.

Percent Increase = 87.5%

Let's understand this process easily using the following image.
Percent Difference Formula

Important Notes

• The formula for percentage change is: [(Final Value - Initial Value) ÷ | Initial Value |] × 100
• If a quantity is increased by r%, then its final value is obtained by multiplying its initial value by 1 + r / 100
• If a quantity is decreased by r%, then its final value is obtained by multiplying its initial value by 1 - r / 100

Example: A number 35 is misread to be 53. Find the percentage change in both numbers.
Solution: Original number = 35
Misread number = 53
The percentage change is calculated using:
[(Misread number - Original number) ÷ Original Number] × 100
= [(53 - 35) ÷ 35] × 100
= (18 ÷ 35) ×100
= 51%