Points to Remember: Squares & Square Roots | Mathematics (Maths) Class 8 PDF Download
| Table of contents |

|
| Facts that Matter |

|
| We know that |

|
| Remember |

|
| Properties of Square Number |

|
| Some Interesting Patterns |

|
| Solved Examples |

|
Facts that Matter
- A natural number ‘n’ is a perfect square, if m2 = n for a natural number m.
- A number ending in 2, 3, 7, or 8 is never a perfect square.
- The squares of even numbers are even.
- The squares of odd numbers are odd.
- A number ending in an odd number of zeros is never a perfect square.
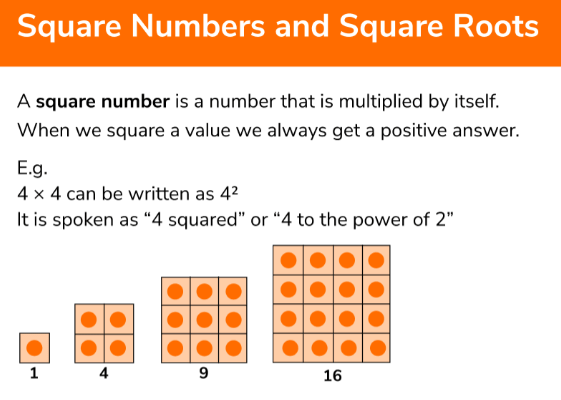
- There are 2n non-perfect square numbers between the squares of the numbers n and n + 1.
- For any natural number ‘n’ greater than 1, 2n, (n2 – 1), and (n2 + 1) form a Pythagorean triplet.
- Finding a square root is the inverse operation of squaring a number.
- If ‘n’ be the number of digits of a square number then the number of digits in its square root is given by n/2 (for ‘n’ is even) and (n+1) /2 (for ‘n’ is odd).
We know that
- If a whole number is multiplied by itself, the product is called the square of that number.
Example: 3 * 3 = 9 = 32
i.e. the square of 3 is 9.
Example: 5 * 5 = 25 = 52
i.e. the square of 5 is 25.
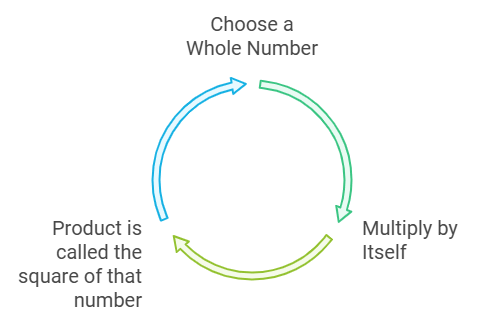
- A natural number is called a perfect square or a square number if it is the square of some natural number.
Example: 16 is the square of 4, therefore, 16 is a perfect square.
Remember
- All-natural numbers are not perfect squares or square numbers, 32 is not a square number. In general, if a natural number ‘m’ can be expressed as n2, where n is also a natural number, then ‘m’ is the perfect square. The numbers like 1, 4, 9, 16, 25, and 36 are called square numbers.
Table: Square of numbers from 1 and 10.
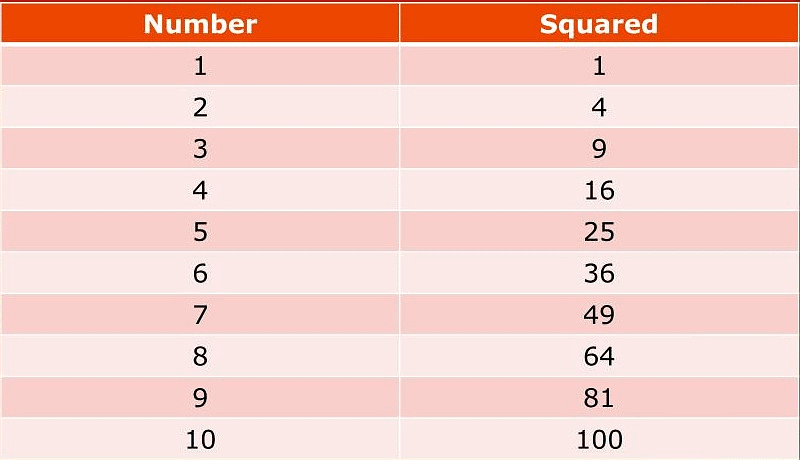
Properties of Square Number
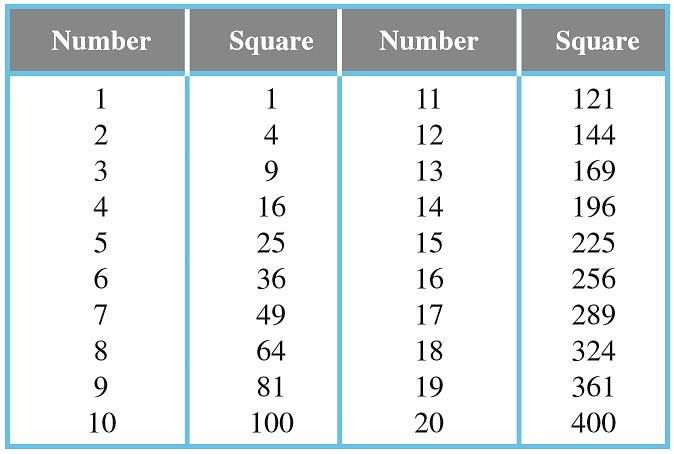
From the table, we conclude that:
Property 1: “The ending digits (the digits in the one’s place) of a square number is 0, 1, 4, 5, 6 or 9 only.”
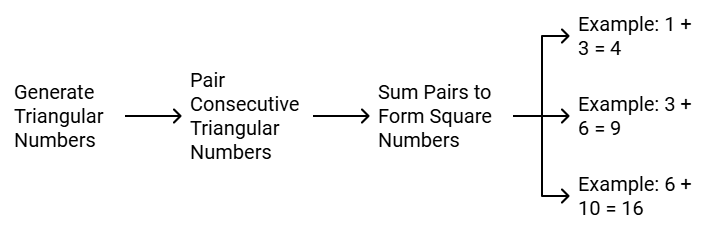
Some Interesting Patterns
- Triangular numbers are: 1, 3, 6, 10, 15, 21, etc. If we combine two consecutive triangular numbers, we get a square number.
1 + 3 = 4, ‘4’ is a square number
3 + 6 = 9, ‘9’ is a square number
6 + 10 = 16, ‘16’ is a square number
and so on.
- 12 =1
112 = 121
1112 = 12321
11112 = 1234321 - 72 = 49
672 = 4489
6672 = 444889
66672 = 44448889 and so on.
Solved Examples
Problem: What will be the unit’s digit in the square of the following numbers?1. 12487
2. 1324
3. 91478
4. 1251
Solution: The unit’s digit in the square of the following is:
1. 12487 is 9 (as 72 = 49, 9 in the unit’s place).
2. 1324 is 6 (as 42 = 16, 6 in the unit’s place).
3. 91478 is 4 (as 82 = 64, 4 in the unit’s place).
4. 1251 is 1 (as 12 = 1, 1 in the unit’s place).
Problem: Comment on the square of an even number and of an odd number.
Solution: The square of an even number is always an even number and the square of an odd number is always an odd number. The square of an even number will always have 4, 6, or even zeros in its unit’s place. And the square of an odd number will always have 1, 5, or 9 in its unit’s place.
|
79 videos|408 docs|31 tests
|
FAQs on Points to Remember: Squares & Square Roots - Mathematics (Maths) Class 8
| 1. What are some properties of square numbers? |  |
| 2. What are some interesting patterns related to square numbers? |  |
| 3. How can square numbers be used to solve problems? |  |
| 4. How do square numbers relate to square roots? |  |
| 5. Can you provide some examples of problems involving square numbers? |  |

|
Explore Courses for Class 8 exam
|

|



















