Class 9 Science Chapter 9 Practice Question Answers - Gravitation
Gravitation Topic is a fundamental concept that has been studied for centuries. It is a force that exists between any two objects in the universe, and it governs the motion of planets, stars, and galaxies. In the context of Class 9 Physics, the study of gravitation introduces students to the basic principles of the force, its effects on objects, and its application in various scenarios. Let us see someNumericals Practice Questions of this capter.

Q1. Let us find force of attraction between two block lying 1m apart. Let the mass of each block is 40 kg.
Sol.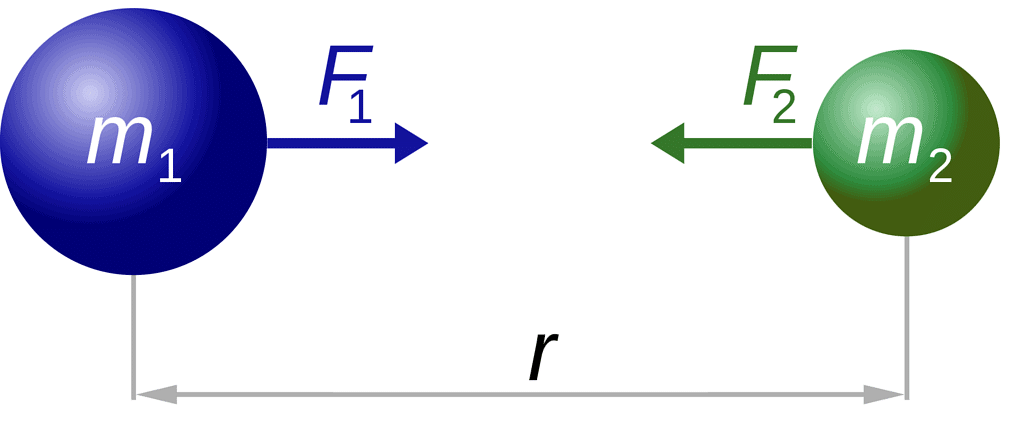 The formula for the gravitational force between two objects is:
The formula for the gravitational force between two objects is: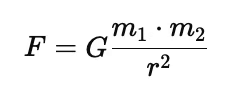
Where:
F is the gravitational force.
G is the universal gravitational constant (6.67430 x 10-11 N·m2/kg2).
m1 and m2 are the masses of the two objects.
r is the distance between the centers of the two objects.
Substitute the given values: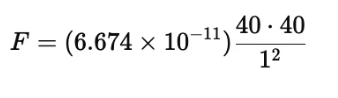

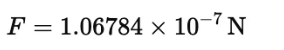
The force of attraction between the two blocks is 1.06784×10−7N.
Q2. The gravitational force between two object is 49 N. How much distance between these objects be decreased so that the force between them becomes double?
Sol. Step-1 : Here, F = 49 N
Let r = original distance between the objects.
m1and m2= masses of the objects
F = G m1 × m2/r² …(1)
Let new distance is R and then force is 2F,
We get, 2F = G m1 × m2/R²…(2)
Dividing equation (2) by (1)
2 = r²/R²
R² = r²/2
R = r/√2
Hence decrease in distance = r-r/√2 = r (√2 - 1)/√2
Q3. Two bodies A and B having masses 2 kg and 4 kg respectively are seperated by 2m. Where should a body of mass 1 kg be placed so that the gravitational force on this body due to bodies A and B is zero?
Sol. Let a body of mass 1 kg be placed at point P at a distance x from body A. Therefore, the distance of mass 1 kg from a body B = (2 x) m.
Gravitaional force on 1 kg due to body A is given by
F = N(along PA) = N (along PA)
Gravitational force on 1 kg due to body B is
F' = N (along PB).
Since gravitational force on 1 kg due to bodies A and B is zero, therefore,
F = F ' or (2 x)22x2or (2 x) = x = 1.414 x
or 2.414 x = 2 or x = 0.83 m.
Q4. Calculate the force of gravitation due to a child of mass 25 kg on his fat mother of mass 75 kg if the distance between their centres is 1m from each other. Given G = (20/3) × 10-11 Nm2 kg-2.
Sol.Here m1 = 25 kg ; m2 = 75 kg ; d = 1 m ; G = 20/3 × 10-11 Nm2 kg-2
Using F = Gm1m2/d2
F = 20/3 x 10-11 x 75 x 25
F = 12,500 × 10-11
or F = 1.25 × 10-7N
Q5. A boy drops a stone from the edge of the roof. If passes a window 2m high in 0.1s. How far is the roof above the top of the window ?
Sol.Let a stone be dropped from the edge of the roof A. Let it passes over B with a velocity say u. Consider motion BC.
u = ?, a = 9.8 ms_2; s = h = 2m ; t = 0.1 s
Using s = ut gt2, we have
2 = u(0.1) × 9.8 (0.1)2
2 = 0.1u 0.049
0.1u = 2 / 0.049
or u = = 19.51 ms_1
This initial velocity at B in motion BC is the final velocity in motion AB. Considering motion AB, we have
u = 0 ; v = 19.51 ms_1; s = ? ; a = 9.8 ms_2
Using v2- u2= 2as, we have
(19.51)2- (0)2= 2 × 9.8 s
or s = 19.4 m
Roof is 19.4 m above the window.
Q6.A ball thrown up is caught by the thrower after 4 s. With what velocity was it thrown up? How high did it go? Where was it after 3 s ? (g = 9.8 m s_2)
Sol.Since the time of going up is the same as that of commg down, therefore time of going up = 4/2 = 2s. Let it starts upward with velocity u.
Here u = ?; a = - 9.8 m s_2; t = 2s; v = 0 (at the top); s = h
Using v = u at
or 0 = u _ 9.8 × 2
or u = 19.6 m s_1
Again v2- u2= 2as
0 - (19.6)2= 2 (-9.8) h
h = 19.6 m
After 2s, it starts coming downwards (starting with u = 0). Considering downward motion.
u = 0; a = 9.8 m s_2; t = 3 - 2 = 1s; s = ?
s = ut at2
or s = 0 × 9.8 (1)2= 4.9 m from top.
Q7. Coconut is hanging on a tree at a height of 15 m from the ground. A boy launches a projectile vertically upwards with a velocity of 20 m s_1. After what time the projectile pass by coconut? Explain the two answers in this problem.
Sol.Here u = 20 m s_1; a = - 10 m s_2; s = 15 m ; t = ?
Using s = ut at2, we have
15 = 20t (-10) t2
Dividing throughout by 5, we have
3 = 4t - t2
or t2- 4t 3 = 0
or (t - 1) (t - 3) =t - 1 = 0 or t = 1s
or t -3 = 0 or t = 3s
After 1s, it will cross coconut while going up and after 3 s while coming down.
Q8. Fig. (a) shows how to calculate pressure exerted by a brick of mass 3 kg : (a) when standing on end; (b) when lying flat. The total force or thrust exerted is the same in both the cases.
Sol.In Fig. (a), A1= 5 cm × 10 cm = 50 cm2= 50 × 10_4m2and
F1= 3 kg wt = 3 kg × 10 m/s2= 30 N
Thus, pressure exerted, P1= = 6 × 103N/m2= 6 × 103Pa
In Fig. (b), A2= 10 cm × 20 cm = 200 cm2= 200 × 10_4m2and
F2= 3 kg wt = 3 kg × 10 m/s2= 30 N
Thus, pressure exerted, P2= = 1·5 × 103N/m2= 1·5 × 103Pa
Q9. What is the force of gravitation between two point masses of 1 kg and 2 kg kept 1 m apart?
Sol.
To calculate the gravitational force (F) between two point masses (m1 and m2) separated by a distance (r), use the formula:
F = (G * m1 * m2) / (r2)
Where:
- F is the gravitational force.
- G is the universal gravitational constant (6.67430 x 10-11 Nm2/kg2).
- m1 and m2 are the masses of the two point masses.
- r is the distance between their centers.
Here, m1 = 1 kg, m2 = 2 kg, and r = 1 m. G is also provided.
Now, substitute these values into the formula to calculate the gravitational force:
F = (6.67430 x 10-11 Nm2/kg2 * 1 kg * 2 kg) / (1 m2)
First, calculate the product of the masses: m1 * m2 = 1 kg * 2 kg = 2 kg2
Now, calculate the force: F = (6.67430 x 10-11 Nm2/kg2 * 2 kg2) / (1 m2)
Next, simplify: F = (6.67430 x 10-11 Nm2/kg2 * 2 kg2) / (1 m2)
Now, perform the calculation: F = 6.67430 10-11 Nm2/kg2 * 2 kg2
Now, multiply the constants: F = 13.34860 x 10-11 Nm2/kg2
So, the gravitational force between the two point masses of 1 kg and 2 kg, kept 1 meter apart, is approximately 13.34860 x 10-11 Nm2/kg2
Q10. Calculate the force of gravitation between the Earth and the sun.Mass of Earth = 6 × 1024 kg, Mass of Sun = 2 × 1030 kg. The average distance between the two is 1.5 × 1011m.
Sol. To calculate the gravitational force (F) between the Earth and the Sun, we can use Newton's law of universal gravitation, which is given by the formula:
F = (G * m1 * m2) / (r2)
Where:
- F is the gravitational force.
- G is the universal gravitational constant (6.67430 x 10-11 Nm2/kg2).
- m1 is the mass of the Earth (6 x 1024 kg).
- m2 is the mass of the Sun (2 x 1030 kg).
- r is the distance between the centers of the Earth and the Sun (1.5 x 1011 m).
Now, substitute these values into the formula to calculate the gravitational force:
F = (6.67430 x 10-11 Nm2/kg2* 6 x 1024 kg * 2 x 1030 kg) / (1.5 x 1011 m)2
First, calculate the product of the masses: m1 * m2 =6 x 1024 kg * 2 x 1030 kg = 1.2 x 1055 kg2
Now, calculate the force: F = (6.67430 x 10-11 Nm2/kg2 * 1.2 x 1055 kg2) / (1.5 x 1011 m)2
Next, simplify: F = (6.67430 x 10-11 Nm2/kg2 * 1.2 x 1055 kg2) / (1.5 x 1011 m)2
Now, perform the calculation: F = (8.00916 x 1043 Nm2) / (2.25 x 1022 m2)
Now, divide to get the value of F: F = 3.5596296 x 1021 N
So, the gravitational force between the Earth and the Sun is approximately 3.5596296 x 1021 Newtons.
Q11. Write down the expression for acceleration experienced by a particle on the surface of the moon due to gravitational force on the moon. Find the ratio of this acceleration to that experienced by the same particle on the surface of the earth. If the acceleration due to gravity on the earth is 9.8 ms_2, what is the acceleration of a particle on the moon's surface? Mass of moon = 7.3 × 1022 kg; Mass of Earth = 6 × 1024 kg. Radius of moon= 1.74 × 106 m, Radius of earth = 6.4 × 106 m.
Sol. The expression for acceleration (am) experienced by a particle on the surface of the Moon due to the gravitational force on the Moon can be calculated using Newton's law of universal gravitation:
am = (G * Mm) / (Rm^2)
Where:
- am is the acceleration on the surface of the Moon.
- G is the universal gravitational constant (6.67430 x 10^-11 Nm^2/kg^2).
- Mm is the mass of the Moon (7.3 x 10^22 kg).
- Rm is the radius of the Moon (1.74 x 10^6 m).
Now, let's calculate am:
am = (6.67430 x 10^-11 Nm^2/kg^2 * 7.3 x 10^22 kg) / (1.74 x 10^6 m)^2
First, calculate the product of the constants:
G * Mm = (6.67430 x 10^-11 Nm^2/kg^2) * (7.3 x 10^22 kg)
Now, calculate Rm^2:
(1.74 x 10^6 m)^2 = 3.0276 x 10^12 m^2
Now, calculate am:
am = (6.67430 x 10^-11 Nm^2/kg^2 * 7.3 x 10^22 kg) / (3.0276 x 10^12 m^2)
Now, perform the calculation:
am ≈ 1.625 m/s^2
So, the acceleration experienced by a particle on the surface of the Moon (am) is approximately 1.625 m/s^2.
To find the ratio of this acceleration to that experienced by the same particle on the surface of the Earth, we can use the same formula for acceleration (ae) on the Earth's surface:
ae = (G * Me) / (Re^2)
Where:
- ae is the acceleration on the surface of the Earth.
- Me is the mass of the Earth (6 x 10^24 kg).
- Re is the radius of the Earth (6.4 x 10^6 m).
Now, let's calculate ae:
ae = (6.67430 x 10^-11 Nm^2/kg^2 * 6 x 10^24 kg) / (6.4 x 10^6 m)^2
First, calculate the product of the constants:
G * Me = (6.67430 x 10^-11 Nm^2/kg^2) * (6 x 10^24 kg)
Now, calculate Re^2:
(6.4 x 10^6 m)^2 = 4.096 x 10^13 m^2
Now, calculate ae:
ae = (6.67430 x 10^-11 Nm^2/kg^2 * 6 x 10^24 kg) / (4.096 x 10^13 m^2)
Now, perform the calculation:
ae ≈ 9.81 m/s^2
So, the acceleration experienced by a particle on the surface of the Earth (ae) is approximately 9.81 m/s^2.
Now, to find the ratio of am to ae:
am / ae ≈ 1.625 m/s^2 / 9.81 m/s^2 ≈ 0.1656
Therefore, the ratio of the acceleration on the surface of the Moon to that on the surface of the Earth is approximately 0.1656.
gm=0.16 × gE= 0.16 × 9.8 = 1.57 ms_2
Q12. Find the value of acceleration due to gravity at a height of (a) 6400 km, (b) 12,800 km from the surface of the earth. Radius of earth is 6400 km.
Sol.
We know that the acceleration due to gravity at the surface of the Earth is 9.8 m/s².
To find the value of acceleration due to gravity at a height of 6400 km above the surface of the Earth, we can use the formula:
g' = (g*R^2) / (r + h)^2
where g is the acceleration due to gravity at the surface of the Earth (9.8 m/s²), R is the radius of the Earth (6400 km), r is the distance from the center of the Earth (also 6400 km), and h is the height above the surface of the Earth.
(a) When h = 6400 km:
g' = (9.8*6400^2) / (6400+6400)^2 = 2.45 m/s²
Therefore, the acceleration due to gravity at a height of 6400 km above the surface of the Earth is 2.45 m/s².
(b)When h = 12800 km:
g' = (9.8*6400^2) / (6400+12800)^2 = 0.61 m/s²
Therefore, the acceleration due to gravity at a height of 12800 km above the surface of the Earth is 0.61 m/s².
Q13. A particle is thrown up vertically with a velocity of 50 m/s. (a) What will be its velocity at the highest point of its journey? (b) How high would the particle rise? (c) What time would it take to reach the highest point.
Sol.At the highest point the velocity will be zero.
Considering activity A to B
Using v = u at
0 = 50 -9.8 × t
t = 5.1 s
Also v2- u2= 2as
02-(50)2= 2 (-9.8) × s
5 = 127.5 m
Q14. With reference to the above sample problem, (a) Find the time the particle takes from the highest point back to the initial point (b) Find the velocity with which the particle reaches the initial point.
Sol.The data is given in the adjacent figure. Considering activity B to A
Using v2 - u2= 2as
v2- 02= 5(9.8)(127.5)
v = 50 m/s
Also v = u at
50 = 0 9.8 (t)
t = 5.1 s
Q15.A ball is dropped from the top of a tower 40 m high. What is its velocity when it has covered 20 m ? What would be its velocity when it hits the ground? Take g= 10 m/s2.
Sol.Let the point B be at a height of 20 m.
Activity from A to B :
u1= 0, a1= 10 ms_2, s1= 20 m, v1= ?
v12- u12= 2a1s1
v12- 02= 2 (10) (20)
v12=202
v1= 20 m/s
Activity from A to C : C is a point on the ground
u2= 0, a2= 10 ms_2, s2= 40 m, v2= ?
v22- u22= 2a2s2
v22- 02= 2 (10) (40)
v22= 800
v2= 28.28 ms_1
Q16.A body is thrown up with a speed 29.4 ms_1.
(a) What is its speed after (i) t = 1 s, (ii) t = 2 s and (iii) t = 3 s.
(b) What is its height after (i) t = 1 s, (ii) t = 2 s and (iii) t = 3 s.
Sol.(a) (i) u = 29.4 ms_1, a = -9.8 ms_2, t1= 1 s, v1= ?
v1= u at1
= 29.4 (-9.8) x 1 = 19.6 ms_1
(ii) u = 29.4 ms, a = -9.8 ms_2, t2 = 2 s, v2 = ?
v2= u at2
= 29.4 (_9.8) × 2 = 9.8 ms_1
(iii) u = 29.4 ms_1, a = -9.8 ms_2, t3 = 3 s, v3 = ?
v3= u at3
= 29.4 (_9.8) × 3 = 0
(b) (i) u = 29.4 ms_1, a = -9.8 ms_2, s1 = h1 , t1 = 1s
h1= ut1at12=29.4 × 1 (-9.8) × 1 =24.5m
(ii) u = 29.4 ms_1, a = -9.8 ms_2, s2 = h2, t2 = 2 s
h2= ut2at22= 29.4 × 2 (-9.8) × 22= 39.2 m
(iii) u = 29.4 ms_1, a = -9.8 ms_2, s3 = h3, t3 = 3 s
h3= ut3at32= 29.4 x 3 (-9.8) × 32= 44.1 m
Q17.What is the weight of a person whose mass is 50 kg.
Sol.The weight of the person
W = mg = 50 x 9.8 = 490 N
Note:The gravitational unit of force is kg-f (kilogram force) or kg-wt (kilogram weight) 1 kg-wt = 9.8 N = 1 kg-f
490 N = 50 kg-f
Q18.Weight of a girl is 294 N. Find her mass.
Sol. Weight (W) = Mass (m) × Acceleration due to gravity (g)
Given that the weight of the girl is 294 N and the acceleration due to gravity on Earth is approximately 9.81 m/s², you can rearrange the formula to solve for mass (m):
m = W / g
Substitute the values:
m = 294 N / 9.81 m/s²
Now, calculate the mass:
m ≈ 29.96 kg
So, the mass of the girl is approximately 29.96 kilograms.
Q19. Weight of an object is 294 N on the surface of the earth. What is its weight at a height of 200 km from the surface of the earth. Radius of the earth= 6400 km.
Sol.Weight at a height of 200 km
Wh= mg
Here mg = 294 N, R = 6400 km, h= 200 km
Wh= 294
= 276.45 N
Note:Weight decreases with increase of height from the surface of the earth.
Q20. The gravitational force between two objects is F. How will this force change when
(i) distance between them is reduced to half ?
(ii) the mass of each object is quadrupled ?
Sol. (i) According to Newton's law of gravitation, gravitational force F between two objects distance r apart is
When distance between them is reduced to half, i.e., r' = r/2, the force,
Thus, F' = 4F
i.e., force becomes 4 times its previous value. "
(ii) Again, according to Newton's law of gravitation, the gravitational force F between two objects of masses m1 and m2 is
F µ m1m2
When mass of each object is quadrupled,
m'1= 4m1
and m'2= 4m2
The force, F ' µ m'1m'2
or F' = 16 F
i.e., force becomes 16 times its previous value.
Q21. A sphere of mass 40 kg is attracted by a second sphere of mass 15 kg when their centres are 20 cm apart, with a force of 0·1 milligram weight. Calculate the value of gravitational constant.
Sol.Here, m1= 40 kg, m2= 15 kg
From r = 20cm =2 × 10_1m
F = 0·1 milligram weight = 0·1 × 10_3gram weight
= 10_4× 10_3kg wt = 10_7× 9·8 N (1 kg wt = 9.8 N)
From G = 6·53 × 10_11Nm2/kg2
Q22. Calculate the force of gravity acting on your friend of mass 60 kg. Given mass of earth = 6 x 1024 kg and radius of Earth = 6·4 x 106 m.
Sol.Here, m = 60 kg, M = 6 × 1024kg
R = 6·4 × 106m, F = ?
G = 6·67 × 10_11Nm2/kg2
Thus, F = 58·62 N
Q23.A particle is thrown up vertically with a velocity of 50 m/s. What will be its velocity at the highest point of the journey? How high would the particle rise ? What time would it take to reach the highest point? Take g = 10 m/s2.
Sol.Here, initial velocity, u = 50 m/s
final velocity, v = ?
height covered, h = ?
time taken, t = ?
g = 10 m/s2
At the highest point, final velocity v = 0
From v2- u2= 2 gh, where g = - 10 m/s2for upward journey,
0 - (50)2= 2 (-10) h
h = 125 m
From v = u gt,
or 0 = 50 (-10) t
t = 50/10 = 5 s
Q24.A force of 15 N is uniformly distributed over an area of 150 m2. Find the pressure in pascals.
Sol.
Pressure (P) = Force (F) / Area (A)
Given:
- Force (F) = 15 N
- Area (A) = 150 m²
Now, simply plug these values into the formula: P = 15 N / 150 m²
Now, calculate the pressure: P = 0.1 Pa
So, the pressure is 0.1 Pascals (Pa).
Q25.How much force should be applied on an area of 1 cm2 to get a pressure of 15 Pa?
Sol.Here, area, A = 1 cm2= 10_4m2
pressure, P = 15 Pa = 15 N/m2
As P = , F = P × A = (15 N/m2) × (10_4m2) = 1·5 x 10_3N
Q26.A block weighing 1·0 kg is in the shape of a cube of length 10 cm. It is kept on a horizontal table. Find the pressure on the portion of the table where the block is kept.
Sol.Given:
- Mass of the block (m) = 1.0 kg
- Acceleration due to gravity (g) ≈ 9.81 m/s²
- Length of one side of the cube (L) = 10 cm = 0.1 m
First, calculate the force (weight) of the block: Weight (F) = m * g
F = 1.0 kg * 9.81 m/s² ≈ 9.81 N
Now, calculate the area (A) of the bottom face of the cube:
A = Length (L) * Width (W)
A = 0.1 m * 0.1 m = 0.01 m²
Now, calculate the pressure (P):
P = F / A
P = 9.81 N / 0.01 m²
P = 981 Pascals (Pa)
Also Check at EduRev
Match the Column
Q1. Cloumn-I Column-II Ans:(A → s), (B → p), (C → q), (D → r)
Ans:(A → s), (B → p), (C → q), (D → r) Q2. Cloumn-I Column-II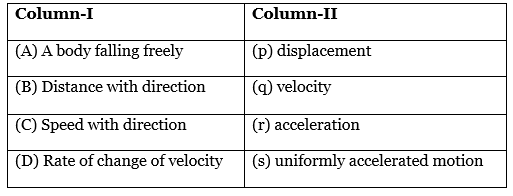 Ans:(A → s), (B → p), (C → q), (D → r)
Ans:(A → s), (B → p), (C → q), (D → r)
Q3. Cloumn-I Column-II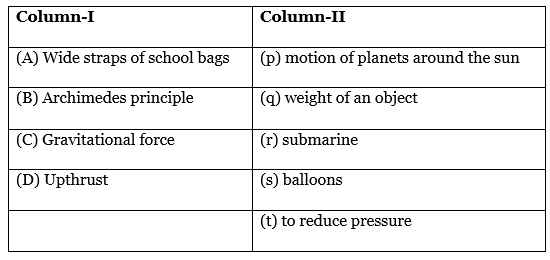 Ans: (A → t), (B → r, s), (C → p, q) (D → r, s)
Ans: (A → t), (B → r, s), (C → p, q) (D → r, s)
Q4. Cloumn-I Column-II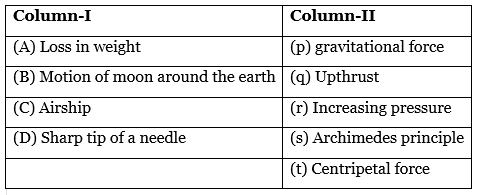 Ans: (A → q, s), (B → p,t), (C → q, s), (D → r)
Ans: (A → q, s), (B → p,t), (C → q, s), (D → r)
1. False 2. False 3. True 4. True 5. True 6. False 7. True
8. True 9. True 10. True 11. True 12. True 13. False 14. False
True & False
Q1: The force of attraction between two bodies is called gravity.Ans: False
Q2: The value of G depends upon the mass of two objects.
Ans: False
Q3: If a spring balance, holding a heavy object is released, it will read zero weight.
Ans: True
Q4: The value of G is high if the radius of the body is more and less if radius is less.
Ans: True
Q5: The centre of mass and centre of gravity for a small body lie at the same point.
Ans: True
Q6: The gravitational force between two bodies changes if a material body is placed between them.
Ans: False
Q7: The acceleration of a body thrown up is numerically the same as the acceleration of a downward falling body but opposite is sign.
Ans: True
Q8: The value of g is zero at the centre of the earth.
Ans: True
Q9: The inertia of an object depends upon its mass.
Ans: True
Q10: All objects attract each other along the line joining their centre of mass.
Ans: True
Q11: Acceleration due to gravity, g = , where symbols have their usual meanings.
Ans: True
Q12: Archimedes' principle does not apply to gases.
Ans: True
Fill in the Blanks
Q1: ________ is the force of attraction between any two bodies in the universe.Ans: Gravitational force is the force of attraction between any two bodies in the universe.
Q2: ________ is the force of attraction between a body and a planet.
Ans: Weight is the force of attraction between a body and a planet.
Q3: Acceleration due to gravity ________ with height from the surface of the earth.
Ans: Acceleration due to gravity decreases with height from the surface of the earth.
Q4: Acceleration due to gravity ________ with depth from the surface of the earth.
Ans: Acceleration due to gravity decreases with depth from the surface of the earth.
Q5: Acceleration due to gravity is a maximum at the ________ .
Ans: Acceleration due to gravity is a maximum at the surface of the earth.
Q6: ________ of a body changes from place to place but its ________ remains constant.
Ans: The weight of a body changes from place to place, but its mass remains constant.
Q7: The upward force experienced by a body immersed partially or fully in a fluid is called ________.
Ans: The upward force experienced by a body immersed partially or fully in a fluid is called upthrust.
Q8: The combined weight of the sinker and cork is ________ than the weight of the sinker alone.
Ans: The combined weight of the sinker and cork is greater than the weight of the sinker alone.
Q9: The centre of ________ is a point, where the total upthrust, due to fluid displaced by part ________ of body acts.
Ans: The center of buoyancy is a point where the total upthrust, due to the fluid displaced by part immersed of the body, acts.
Q10: A fish ________ by squeezing out air, from its ________.
Ans: A fish sinks by squeezing out air from its air bladder.
Q11: When a body is partly or wholly immersed in a ________ , it experience an ________.
Ans: When a body is partly or wholly immersed in a fluid, it experiences an upthrust.
Q12: An iceberg floats with ________ of its volume below ________.
Ans: An iceberg floats with 90% of its volume below the water surface.
Q13: The unit of upthrust in SI is ________.
Ans: The unit of upthrust in SI is newton.
Q14: Lactometer is used to measure ________ of ________.
Ans: Lactometer is used to measure the density of milk.
Q15: A hydrometer sinks ________ in water than in pure milk.
Ans: A hydrometer sinks deeper in water than in pure milk.
|
88 videos|369 docs|67 tests
|
FAQs on Class 9 Science Chapter 9 Practice Question Answers - Gravitation
| 1. What is the law of universal gravitation? |  |
| 2. How does gravity affect the motion of planets? |  |
| 3. What is the gravitational field strength and how is it calculated? |  |
| 4. What role does gravitational potential energy play in gravitation? |  |
| 5. How does distance affect gravitational force between two objects? |  |

|
Explore Courses for Class 9 exam
|

|


















