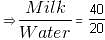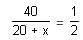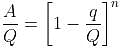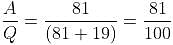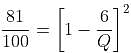This EduRev document offers 10 Multiple Choice Questions (MCQs) from the topic Mixtures & Alligations (Level - 1). These questions are of Level - 1 difficulty and will assist you in the preparation of CAT & other MBA exams. You can practice/attempt these CAT Multiple Choice Questions (MCQs) and check the explanations for a better understanding of the topic.
Question for Practice Questions Level 1: Mixtures & Alligations - 1
Try yourself:In a mixture of 60 litres, the ratio of milk to water is 2 : 1. If the ratio of milk to water needs to be 1 : 2, then the amount of water (in litres) to be added further is
Explanation
Amount of mixture = 60 litres
Ratio of milk to water = 2 : 1

Let the amount of added water be x litres to make the ratio of milk to water 1 : 2.

⇒ x = 60 litres
Amount of water to be added = 60 litres
Report a problem
Question for Practice Questions Level 1: Mixtures & Alligations - 1
Try yourself:If 20 litres of mixture containing 15% alcohol is mixed with 30 litres of mixture containing 18% alcohol, what is the concentration of the resulting solution?
Explanation
Amount of alcohol in the resulting mixture = 20 × 0.15 + 30 × 0.18 = 8.4 litres
Total amount of the resulting mixture = 50 litres
Concentration of alcohol in the resulting mixture = 8.4 × 100/50 = 16.8%
Report a problem
Question for Practice Questions Level 1: Mixtures & Alligations - 1
Try yourself:A milkman mixes 20 litres of water with 80 litres of milk. After selling one-fourth of this mixture, he adds water to replenish the quantity that he has sold. What is the current proportion of water to milk?
Explanation
Total mixture = 100 litres
Quantity of milk = 80 litres
Quantity of water = 20 litres
¼ of the mixture removed = 25 litres
Quantity of milk removed = 25 × 4/5 = 20 litres
Quantity of water removed = 25 × 1/5 = 5 litres
After adding 25 litres of water, total quantity of milk = 80 - 20 = 60 litres
Total quantity of water = 20 - 5 + 25 = 40 litres
Hence, required ratio of water to milk = 2 : 3
Report a problem
Question for Practice Questions Level 1: Mixtures & Alligations - 1
Try yourself:A vessel is full of a mixture of liquor and water with 18% liquor. Eight litres are drawn of and then the vessel is filled with water. How much does the vessel hold if the liquor is now 15%?
Explanation
Let the total volume of vessel be 'x' litres.
Initially, the %age of liquor in the mixture = 18%
After the replacement of 8 litres of the mixture with water, liquor %age = 15%
∴ 0.18x - 8 18/100 = 0.15x
⇒ 0.03x = 1.44
⇒ x = 48 litres
Hence, volume of vessel = 48 litres
Report a problem
Question for Practice Questions Level 1: Mixtures & Alligations - 1
Try yourself:Six gallons of wine is drawn from a cask and is replaced by six gallons of water. Six gallons of the mixture is drawn and again replaced by six gallons of water. If the ratio of wine to water in the cask is now 81 : 19, then how much wine was in the cask at first?
Explanation
Report a problem
Question for Practice Questions Level 1: Mixtures & Alligations - 1
Try yourself:We have two solutions of milk - A and B. Solution A contains milk and water in the ratio of 1 : 3 and solution B contains the same in the ratio of 2 : 3. If we mix both in equal quantities, then what is the ratio of milk and water in the new solution?
Explanation
Suppose, we mix 1 litre of each solution.
So, the quantity of milk in the new solution would be 1/4 + 2/5 = 13/20
And, the quantity of water in the new solution would be 3/4 + 3/5 = 27/20
The ratio of milk and water would be (13/20) : (27/20) = 13 : 27
Report a problem
Question for Practice Questions Level 1: Mixtures & Alligations - 1
Try yourself:A lump of two metals weighing 18 grams is worth Rs. 74, but if their weights are interchanged, it would be worth Rs. 60.10. If the price of gold is Rs. 7.20 per gram, then find the weight of the other metal in the mixture.
Explanation
Let the weight of gold be x g and weight of the other metal be (18 - x) g.
Let the price of the other metal be Rs. y
Now, according to the question
7.20x + y(18 - x) = 74
7.20(18 - x) + yx = 60.10
Solving these two equations simultaneously,
x = 10 and y = 0.25
Quantity of the other metal = (18 - 10) g = 8 g
Report a problem
Question for Practice Questions Level 1: Mixtures & Alligations - 1
Try yourself:We have two solutions of milk - A and B. Solution A contains milk and water in the ratio of 1 : 3 and solution B contains the same in the ratio of 2 : 3. If we mix both in equal quantities, then what is the ratio of milk and water in the new solution?
Explanation
Suppose, we mix 1 litre of each solution.
So, the quantity of milk in the new solution would be 1/4 + 2/5 = 13/20
And, the quantity of water in the new solution would be 3/4 + 3/5 = 27/20
The ratio of milk and water would be (13/20) : (27/20) = 13 : 27
Report a problem
Question for Practice Questions Level 1: Mixtures & Alligations - 1
Try yourself:An alloy contains zinc and tin in the ratio of 3 : 4. Another alloy contains zinc and silver in the ratio of 4 : 3. If both of these alloys are melted and mixed in equal ratios, then what will be the ratio of zinc and tin in the new alloy?
Explanation
Let the weights of first and second alloys be 7x and 7y, respectively.
According to the question, both are mixed in equal ratios.
So, x = y
In first alloy, zinc and tin are 3x and 4x, respectively.
In second alloy, zinc and silver are 4x and 3x, respectively.
After mixing these two alloys, new alloy contains:
Zinc = 7x, tin = 4x and silver = 3x
Hence, ratio of zinc and tin = 7 : 4
Report a problem
Question for Practice Questions Level 1: Mixtures & Alligations - 1
Try yourself:Two liquids A and B are in the ratio 5 : 1 in container X and in the ratio 1 : 3 in container Y. In what ratio should the contents of the two containers be mixed so as to obtain a mixture of A and B in the ratio 1 : 1?
Explanation
Let X units of liquid be taken from container X.
So, liquid A taken = (5/6)X
Liquid B taken = (1/6)X
Let Y units of liquid be taken from container Y.
So, liquid A taken = (Y/4)
Liquid B taken = (3/4)Y
So, as the ratio is 1 : 1, we get
(5/6)X + (Y/4) = (X/6) + (3/4)Y
Or X/Y = 3/4
Hence, the required ratio is 3 : 4.
Report a problem