This EduRev document offers 20 Multiple Choice Questions (MCQs) from the topic Speed, Time & Distance (Level - 2). These questions are of Level - 2 difficulty and will assist you in the preparation of CAT & other MBA exams. You can practice/attempt these CAT Multiple Choice Questions (MCQs) and check the explanations for a better understanding of the topic.
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:A, B and C take part in a race over a distance of d metres at uniform speed. If A can beat B by 20 metres, B can beat C by 10 metres and A can beat C by 28 metres, then what is the value of d?
Explanation
Let the speed of B be VB m/s and that of C be VC m/s.
Now, in the same time as A runs d metres, B runs (d - 20) metres and this time is (d - 20)/VB ..(i)
In the same time that A runs d metres, C runs (d - 28) metres and this time is (d - 28)/VC ...(ii)
Equating the 2 times from the expressions (i) and (ii), we get (d - 20)/VB = (d - 28)/VC..(iii)
Also, in the time that B runs d m, C runs (d - 10) m, which is (d/VB) = (d - 10)/VC ...(iv)
Now, from equation (iv), we get VB = VCd/(d - 10)..(v)
Plugging in this value of VB from equation (v) into equation (iii), we get ((d - 20)(d - 10))/(VCd) = (d - 28)/VC
Solving for d, we get d = 100 metres
Thus, answer option (3) is correct.
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:Mr. Racer started from Hyderabad to Bangalore with a speed of 60 kmph. After some time, he changed his speed to 80 kmph and reached Bangalore. He returned with the same speed and changed his speed to 60 kmph at the place where he had initially changed his speed in the onward journey, and came back to Hyderabad. What was his average speed for the entire journey? (The distance between Hyderabad and Bangalore is 600 km).
Explanation
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:A boat P travels 40 km upstream from point A to point B in 10 hours and from point B to point A downstream in 5 hours. Another boat Q can travel from point A to a point C, 30 km upstream, in 5 hours. At 10 am, P starts from A and goes towards B. At the same time, Q starts from C and goes towards A.
At what time will they meet each other?
Explanation
Let P be the speed of boat P and Q be that of boat Q.
Let s be the speed of stream.
We have P - s = 40/10 = 4 km/hr and P + s = 40/5 = 8 km/hr
Solving, we get P = 6 and s = 2
Again, Q - s = 30/5 = 6
Since s = 2, Q = 8 km/hr
Therefore, downstream speed of Q = 2 + 8 = 10 km/hr
Hence, time taken = 30/(10 + 4) = 30/14 = 15/7 hours = 2 hrs 8 mins (approx.)
Time at which both boats meet each other = 12:08 pm
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:Ramesh takes 6 hours for a 300 km journey from Pune to Mumbai, if 180 km is travelled by train and the rest by bike. It takes 15 minutes more, if 200 km is travelled by train and the rest by bike. The ratio of the speed of the train to that of the bike is
Explanation
Let the speed of the train be x kmph and that of the bike be y kmph.
Then, according to the question,
(180/x) + (120/y) = 6 ---------(1)
(200/x) + (100/y) = (6 hrs + 15 min) = 25/4 ---------(2)
On solving (1) and (2), we get
x = 40 and y = 80
Therefore, ratio = 40 : 80 = 1 : 2
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:Mani leaves home for office at 7 a.m. and reaches at 7:40 a.m. On the other hand, his brother Vikram leaves the same office at 7:20 a.m. and reaches their common home at 7:50 am. At what time do they cross on the way, if they follow the same route?
Explanation
Let the speed of Vikram be 4vm/min.
Speed of Mani is 3vm/min.
As Vikram takes 30 minutes to cover the distance, distance to office = d m = 4v × 30 m ...(i)
Let the two meet at t minutes after 7:20.
By that time Mani has traveled for (t + 20) minutes and has covered a distance of d1m = 3v(t + 20) m ...(ii)
Vikram has traveled a distance of d2 m = 4vt m ...(iii)
Between the two of them, they together have covered a distance of the total one way distance from home to office or vice versa.
Thus, d m = d1 m + d2 m ...(iv)
Plugging in the values of d, d1 and d2 from equations (i), (ii) and (iii) into (iv) in terms of v and t, and then solving for t, we get t = 9 (approx.)
Thus, they cross each other at (20 + 9) min after 7:00 a.m. or at 7:29 a.m.
Thus, option 3 is correct.
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:A ship develops a leak 12 km from the shore. Despite the leak, the ship is able to move towards the shore at a speed of 8 km/hr. However, the ship can stay afloat only for 20 minutes. If a rescue vessel were to leave from the shore towards the ship and it takes 4 minutes to evacuate the crew and passengers of the ship, what should be the minimum speed of the rescue vessel in order to be able to successfully rescue the people aboard the ship?
Explanation
The distance between the rescue vessel and the ship, which is 12 km, has to be covered in 16 minutes (the ship can stay afloat for only 20 minutes and it takes 4 minutes to evacuate the people aboard the ship).
Therefore, the ship and the rescue vessel should move towards each other at a combined speed of 12 × (60/16) km/hr = 45 km/hr.
The ship is moving at a speed of 8 km/hr. Therefore, the rescue vessel should move at a speed of 45 - 8 = 37 km/hr.
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:Two guns are fired from the same place at an interval of 12 minutes, but a person in a train approaching the place hears the second shot 10 minutes after the first. Find the speed of the train if the speed of sound is 330 m/s.
Explanation
If the person had not moved towards the source of the gunfire, he would have heard the second shot 12 minutes after the first shot.
Since the person is actually moving towards the source, the shot is now heard after 10 minutes.
It means the sound would have taken 2 more minutes to reach the initial position of the person, but this very distance was travelled by the train in 10 minutes.
It means that speed of the train is 1/5 of the speed of sound.
Or 330/5 m/s = 66 m/s
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:A boat P travels 40 km upstream from point A to B in 10 hours and from point B to A downstream in 5 hours. Another boat Q can travel from point A to a point C 30 km upstream in 5 hours. At 10 am, P starts from A and goes towards B. At the same time, Q starts from C and proceeds towards A. At what distance from B will they meet each other?
Explanation
Let the speed of boat P in still water be M.
Let the speed of water be x.
Therefore;
While going upstream:
M - x = 40/10 = 4 …................................. (1)
While going downstream:
M + x = 40/5 = 8 …................................. (2)
From (1) and (2), we get
M = 6, x = 2
For boat Q:
Let the speed of boat Q be S.
While going upstream:
S - 2 = 30/5 = 6
Therefore, S = 8
Distance between points B and C is 10 km.
Boats P and Q started from A and C, respectively.
Let the distance covered by boat P from point A be y and that by boat Q be (30 - y).
The time taken by both the boats is the same as they have started at the same time, so
y/4 = (30 - y)/10 (as boat P is going upstream and boat Q is going downstream)
Hence, y = 8.6
Therefore, the distance of their meeting point from B is 10 + (30 - 8.6) = 10 + 21.4 = 31.4 km.
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
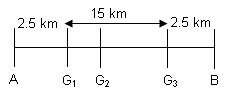
Try yourself:In a 20 km long tunnel connecting two cities A and B, there are three gutters. The distance between gutters 1 and 2 is half the distance between gutters 2 and 3. The distance from city A to its nearest gutter - gutter 1 - is equal to the distance of city B from gutter 3. On a particular day, the hospital in city A receives information that an accident has occurred at the third gutter. The victim can be saved only if an operation is started within 40 minutes. An ambulance starts from city A at 30 km/hr and crosses the first gutter after 5 minutes. If the driver doubles the speed after that, then what is the maximum amount of time the doctor will get to attend the patient at the hospital before starting the operation. Assume 1 minute elapses in taking the patient into and out of the ambulance.
Explanation
AG1 = 5 min at 30 km/hr = 2.5 km
G3B = 2.5 km
G1G3 = 20 - 2.5 - 2.5 = 15 km
Time for AG1 = 5 min
Time for G1G3 = 15 km at 60 km/hr = 15 min
Time for G3 A = 17.5 km at 60 km/hr = 17.5 min
Total time = 15 + 17.5 + 5 = 37.5 min
1 min is taken in transferring the patient into and out of the ambulance.
Hence, (40 – 37.5 – 1) = 1.5 min remain.

Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:The J&K Express from Delhi to Srinagar was delayed by snowfall for 16 minutes and made up for the delay on a section of 80 km travelling with a speed 10 km per hour higher than its normal speed. Find the original speed of the J&K Express (according to the schedule)
Explanation
By travelling at 10 kmph higher than the original speed, the train is able to make up 16 minutes while traveling 80 km. This condition is only satisfied at an initial speed of 50 (and a new speed of 60 kmph).
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:Amitabh covered a distance of 96 km two hours faster than he had planned to. This he achieved by travelling 1 km more every hour than he intended to cover every 1 hour 15 minutes. What was the speed at which Amitabh travelled during the journey?
Explanation
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:A pedestrian and a cyclist start simultaneously towards each other from Aurangabad and Paithan which are 40 km apart and meet 2 hours after the start. Then they resumed their trips and the cyclist arrives at Aurangabad 7 hours 30 minutes earlier than the pedestrian arrives at Paithan. Which of these could be the speed of the pedestrian?
Explanation
The relative speed is 20 kmph. Also, the pedestrian should take 7:30 hours more than the cyclist.
Using option (a) the speeds of the two people are 4km/hr and 16 km/hr respectively.
At this speed, the respective times would be 10 hrs and 2:30 hours, giving the required answer.
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:Two motorists met at 10 a.m. at the Dadar railway station. After their meeting, one of them proceeded in the East direction while the other proceeded in the North direction. Exactly at noon, they were 60 km apart. Find the speed of the slower motorist if the difference of their speeds is 6 km/h.
Explanation

The distance between the motorists will be shown on the hypotenuse.
Using the 3,4,5 Pythagoras triplet and the condition that the two speeds are 6 kmph different from each other, you will get the triplet as: 18, 24, 30.
Hence, the slower motorist travelled at 18 kmph.
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:Two ants start simultaneously from two ant holes towards each other. The first ant coveres 8% of the distance between the two ant holes in 3 hours, the second ant covered 7/120 of the distance in 2 hours 30 minutes. Find the speed (feet/h) of the second ant if the first ant travelled 800 feet to the meeting point.
Explanation
Since the second ant covers 7/120 of the distance in 2 hours 30 minutes, we can infer that is covers 8.4/120 = 7% of the distance in 3 hours.
Thus, in 3 hours both ants together cover 15% of the distance → 5% per hour → they will meet in 20 hours.
Also, ratio of speeds = 8 : 7.
So, the second ant would cover 700 ft to the meeting point in 20 hours and its speed would be 35 feet/hr.
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:A racetrack is in the form of a right triangle. The longer of the legs of the track is 2 km more than the shorter of the legs (both these legs being on a highway). The start and end points are also connected to each other through a side road. The escort vehicle for the race took the side road and rode with a speed of 30 km/h and then covered the two intervals along the highway during the same time with a speed of 42 km/h. Find the length of the racetrack.
Explanation
The requisite conditions are met on a Pythagoras triplet 6,8,10.
Since the racetrack only consists of the legs of the right triangle the length must be 6 + 8 = 14 km.
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:Karim, a tourist leaves Ellora on a bicycle. Having travelled for 1.5 h at 16 km/h, he makes a stop for 1.5 h and then pedals on with the same speed. Four hours after Karim started, his friend and local guide Rahim leaves Ellora on a motorcycle and rides with a speed of 28 km/h in the same direction as Karim had gone. What distance will they cover before Rahim overtakes Karim?
Explanation
When Rahim starts, Karim would have covered 40 km.
Also, their relative speed is 12 kmph and the distance between the two would get to 0 in 40/12 = 3.33 hours.
Distance covered = 28 × 3.33 = 93.33 km.
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:An ant climbing up a vertical pole ascends 12 meters and slips down 5 meters in every alternate hour. If the pole is 63 meters high how long will it take it to reach the top?
Explanation
The ant would cover 7 × 8 = 56 meters in 16 hours.
Further, it would require 7/12 of the 17th hour to reach the top.
Thus time required = 16 hours 35 minutes
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:An ant moved for several seconds and covered 3 mm in the first second and 4 mm more in each successive second than in its predecessor. If the ant had covered 1 mm in the first second and 8 mm more in each successive second, then the difference between the path it would cover during the same time and the actual path would be more than 6 mm but less than 30 mm. Find the time for which the ant moved (in seconds).
Explanation
The movement of the ant in the two cases would be 3, 7, 11, 15, 19, 23 and 1, 9, 17, 25, 33, 41.
It can be seen that after 3 seconds the difference is 6mm, after 4 seconds, the difference is 16mm and after 5 seconds the difference is 30 mm.
Thus, it is clearly seen that the ant moved for 4 seconds.
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:The Sabarmati Express left Ahmedabad for Mumbai. Having travelled 300 km, which constitutes 66.666 per cent of the distance between Ahmedabad and Mumbai, the train was stopped by a red signal. Half an hour later, the track was cleared and the enginedriver, having increased the speed by 15 km per hour, arrived at Mumbai on time. Find the initial speed of the Sabarmati Express.
Explanation
When the signal happened distance left was 150 km.
150/(s) – 150/(s + 15) = 1/2 hours → s = 60.
Report a problem
Question for Practice Questions Level 2: Speed, Time & Distance - 2
Try yourself:Read the following and answer the question that follow.
A naughty bird is sitting on top of a car. It sees another car approaching it at a distance of 12 km. The speed of the two cars is 60 kmph each. The bird starts flying from the first car and moves towards the second car, reaches the second car and comes back to the first car and so on. If the speed at which the bird flies is 120 kmph then answer the following questions. Assume that the two cars have a crash.
Q. The total distance travelled by the bird before the crash is
Explanation
The total distance the bird would travel would be dependent on the time that the cars crash with each other.
Also, the speed of the bird is the same as the relative speed of the cars.
Hence, the answer to question 41 will be 12 km.
Report a problem


 +
+ 
 speed per 75 minutes
speed per 75 minutes  New speed.
New speed.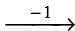 Speed per 75 minutes
Speed per 75 minutes 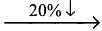 We need to use this process to check the option.
We need to use this process to check the option.