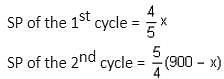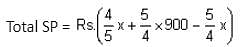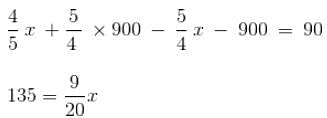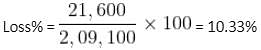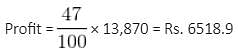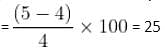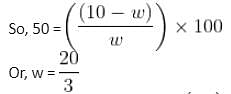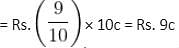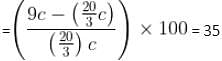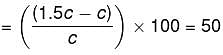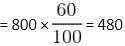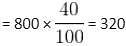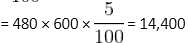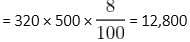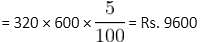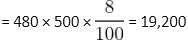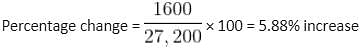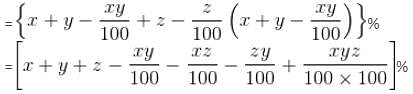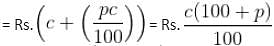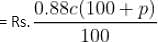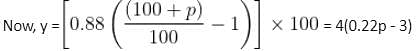This EduRev document offers 10 Multiple Choice Questions (MCQs) from the topic Profit, Loss & Discount (Level - 3). These questions are of Level - 3 difficulty and will assist you in the preparation of CAT & other MBA exams. You can practice/attempt these CAT Multiple Choice Questions (MCQs) and check the explanations for a better understanding of the topic.
Question for Practice Questions Level 3: Profit, Loss & Discount - 2
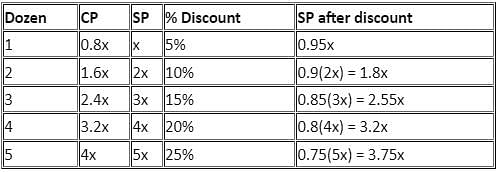
Try yourself:Discounts offered by the fruit vendor on the selling price of mangoes are in the following way.
5% discount on the selling price of 1 dozen mangoes
10% discount on the selling price of 2 dozen mangoes
15% discount on the selling price of 3 dozen mangoes and so on.
Find the maximum percentage discount a fruit vendor can give to a customer so as to have no loss.
(Note: A fruit vendor gets 25% profit on selling 1 dozen mangoes without giving discount)
Explanation

When a fruit vendor sells 5 dozen mangoes to the customers, then the selling price after giving 25% becomes Rs. 3.75x, which is less than its cost price (Rs. 4x).
Therefore, fruit vendor can give a maximum of 20% discount to the customer.
Report a problem
Question for Practice Questions Level 3: Profit, Loss & Discount - 2
Try yourself:A man buys two cycles at a total cost of Rs. 900. By selling one cycle for 4/5th of its cost and the other for 5/4th of its cost, he makes a profit of Rs. 90 on the whole transaction. Find the cost prices of both the cycles.
Explanation
Let CP of the 1st cycle be Rs. x.
Then, CP of the 2nd cycle = Rs. 900 – x


Total CP = Rs. 900
Profit = Rs. 90
SP – CP = Profit

x = 300
Thus, cost price of the 1st cycle = x = Rs. 300
And cost price of the 2nd cycle = Rs. 600
Report a problem
Question for Practice Questions Level 3: Profit, Loss & Discount - 2
Try yourself:Hotel Aditya has 10 single AC rooms, 5 double AC rooms and 18 non-AC rooms. The fixed monthly rent of the hotel is Rs. 1,50,000. The per day maintenance cost is Rs. 100 for double AC room, Rs. 75 for single AC room and Rs. 40 for non-AC room. All the rooms are maintained everyday, irrespective of occupancy. The per day charges are Rs. 600 for double AC room, Rs. 400 for single AC room and Rs. 250 for non-AC room. In April 2003, the occupancy rate of non-AC room was 50%, that of single AC room was 70% and that of double AC room was 40%. Find the profit or loss percent for that particular month.
Explanation
Monthly rent = Rs. 1,50,000
Maintenance = Rs. 100 × 5 × 30 + 75 × 10 × 30 + 40 × 18 × 30
= 15,000 + 22,500 + 21,600 = Rs. 59,100
Total cost = 2,09,100
Amount received = 9 × 250 × 30 + 7 × 400 × 30 + 2 × 600 × 30
= 67,500 + 84,000 + 36,000 = 1,87,500

Report a problem
Question for Practice Questions Level 3: Profit, Loss & Discount - 2
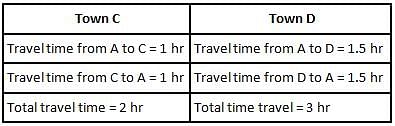
Try yourself:Vishal lives in town A. He leaves his house for exactly 10 hours everyday to sell fruits. He purchases his fruits from either local Town A market or from any of the three neighbouring towns B, C or D. It takes him 1 hour to reach C and 1.5 hours to reach D from A. The time required for travelling from B to C, C to D or B to D is 1 hour. In 1 hour, he sells, exactly 1 kg of fruits. The rates of fruits in the four towns are as follows:
A = Rs. 20/kg, B = Rs. 25/kg, C = Rs. 10/kg, D = Rs. 11/kg
He completes each day without any stock at the end. Assume that the buying and selling price are both the same.
The maximum profit Vishal can make by selling in town A only is
Explanation
To make the profit by selling in town A, Vishal has to buy either from town C or town D as the prices are low in these town only.

Vishal leaves his house exactly for 10 hours to sell fruits.
∴ In case of buying the fruits from C, he is left with 8 hours.
In 8 hours, he can sell 8 kg of fruits.
Hence, profit = (selling price at town A) - (buying price from town C) x 8 = (20 - 10) 8 = Rs. 80
In case of buying the fruits from D, he is left with 7 hours.
In 7 hours, he can sell 7 kg of fruits.
Hence, profit = (selling price at town A) - (buying price from town D) x 7 = (20 - 11) 7 = Rs. 63
Hence, Rs. 80 is the maximum.
Report a problem
Question for Practice Questions Level 3: Profit, Loss & Discount - 2
Try yourself:Vikram buys certain watches worth Rs. 13,870. He decides to make a profit of 10% on the sale of watches worth Rs. 3870 and increase the profit percent to 16% for sales of watches worth Rs. 6000 and then, increase the profit percent for the sale of remaining lot such that he is able to make a profit of 47% on the sale of the full lot. Find the profit that he makes on the third lot of watches.
Explanation
CP of watches for Vikram = Rs. 13,870
He earns 47% profit on the lot.

Now, this profit is made by earning 10% on Rs. 3870, 16% on Rs. 6000 and say Rs. x profit on the remaining lot of Rs. 4000.
So, 6518.9 = 0.1 × 3870 + 0.16 × 6000 + x
6518.9 = 387 + 960 + x
x = Rs. 5171.9, which is profit from sale of the third lot.
Report a problem
Question for Practice Questions Level 3: Profit, Loss & Discount - 2
Try yourself:Kambli is a very shrewd and dishonest spice merchant. Being an electronics genius and a gadget guru, he adjusts the electronic weighing scale at his godown in such a manner that the error percentage of the scale is directly proportional to the displayed weight (in kg) of the commodity being transacted. However, to maintain his falsely portrayed honest image and goodwill in the market, he gives a discount of 10% on every transaction. The weighing scale displays 5 kg for 4 kg. If he sells the commodity at its cost price, what is the actual profit percentage when the displayed weight is 10 kg?
Explanation
Let the cost price of the commodity sold incurred by Kambli be Rs. c/kg.
Error percentage in the 5-4 weight

So, 25 = a × 5; where, a is a constant.
Or, a = 5
So, when the displayed weight is 10 kg, the error percent is 5 × 10 = 50.
Let the actual weight be w kg.

Thus, the cost price is Rs. (20/3)c
Sales revenue

Thus, profit percentage

Hence, answer option 1 is correct.
Report a problem
Question for Practice Questions Level 3: Profit, Loss & Discount - 2
Try yourself:Simran, a vegetable vendor, has a faulty weighing balance, the right pan of which weighs 200 gm more than the left pan. He has a weight of 1 kg only. While buying goods, he keeps the weight on the right pan. While selling the vegetables, he keeps the weight on the left pan. If he sells the vegetables at their cost price, what is his percentage gain?
Explanation
Let the cost price of a vegetable be Rs. c/kg in the market from where Simran buys.
So, for Rs. c he is getting 1.2 kg of vegetables.
Now, because of the faulty balance, he is selling .800 kg of the vegetable for Rs. c.
Or, he is selling 1.2 kg of vegetables for Rs. (1.2/0.8)c = Rs. 1.5c.
Therefore, his profit percentage

Hence, answer option 3 is correct.
Report a problem
Question for Practice Questions Level 3: Profit, Loss & Discount - 2
Try yourself:There were 800 waiters in a hotel; of which 60% were males and the rest were females. The male waiters got a tip of 5% of the bill and the female waiters got a tip of 8% of the bill. Find the % change in the total tip amount, if the ratio of male to female gets reversed next year, but the total number of waiters remained the same. (You can assume that each male waiter gets an order of Rs. 600 and each female waiter gets an order of Rs. 500).
Explanation
Report a problem
Question for Practice Questions Level 3: Profit, Loss & Discount - 2
Try yourself:Shopkeepers A and B mark a particular item at the same price. A gives three successive discounts of x%, y% and z% on the item. B gives a single discount of (x + y + z)% on the item. Then, which of the following is true?
Explanation
As no data about the cost price of that particular item is given, we cannot determine whether they made a profit or loss. Thus, [1] and [2] are false.
Now, the discount given by B = (x + y + z)%, whereas discount given by A

Thus, the discount given by A is lesser than that of B.
So, the selling price of A is higher than that of B.
Report a problem
Question for Practice Questions Level 3: Profit, Loss & Discount - 2
Try yourself:On ties, a Raymonds showroom gives a discount of 12%. If the percentage above the cost price by which the storeowners must mark up the price of the ties is denoted by p (where p is an integer), and the profit then made by them on selling a tie is y%, which of the following cannot be a possible value of y?
Explanation
Report a problem