Practice Questions: Number System | Quantitative Aptitude (Quant) - CAT PDF Download
Q1: Consider six distinct natural numbers such that the average of the two smallest numbers is 14, and the average of the two largest numbers is 28. Then, the maximum possible value of the average of these six numbers is
(a) 23
(b) 24
(c) 23.5
(d) 22.5
Ans: (d)
Sol: Let the six numbers be a, b, c, d, e, f in ascending order
a + b = 28
e + f = 56
If we want to maximise the average then we have to maximise the value of c and d and maximise e and minimise f
e + f = 56
As e and f are distinct natural numbers so possible values are 27 and 29
Therefore c and d will be 25 and 26 respecitively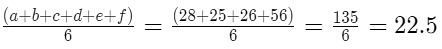
Q2: For some natural number n, assume that (15,000)! is divisible by (n!)!. The largest possible value of n is
(a) 4
(b) 7
(c) 6
(d) 5
Ans: (b)
Sol: To find the largest possible value of n, we need to find the value of n such that n! is less than 15000.
7! = 5040
8! = 40320 > 15000
This implies 15000! is not divisible by 40320!
Therefore, maximum value n can take is 7.
The answer is option B.
Q3: A school has less than 5000 students and if the students are divided equally into teams of either 9 or 10 or 12 or 25 each, exactly 4 are always left out. However, if they are divided into teams of 11 each, no one is left out. The maximum number of teams of 12 each that can be formed out of the students in the school is
Sol: Since the total number of students, when divided by either 9 or 10 or 12 or 25 each, gives a remainder of 4, the number will be in the form of LCM(9,10,12,25)k + 4 = 900k + 4.
It is given that the value of 900k + 4 is less than 5000.
Also, it is given that 900k + 4 is divided by 11.
It is only possible when k = 2 and total students = 1804.
So, the number of 12 students group = 1800/12 = 150.
Q4: For a 4-digit number, the sum of its digits in the thousands, hundreds and tens places is 14, the sum of its digits in the hundreds, tens and units places is 15, and the tens place digit is 4 more than the units place digit. Then the highest possible 4-digit number satisfying the above conditions is
Sol: Given the 4 digit number:
Considering the number in thousands digit is a number in the hundredth digit is b, number in tens digit is c, number in the units digit is d.
Let the number be abcd.
Given that a+b+c = 14. (1)
b+c+d = 15. (2)
c = d+4. (3).
In order to find the maximum number which satisfies the condition, we need to have abcd such that a is maximum which is the digit in thousands place in order to maximize the value of the number. b, c, and d are less than 9 each as they are single-digit numbers.
Substituting (3) in (2) we have b + d + 4 + d = 15, b+2*d = 11. (4)
Subtracting (2) and (1) : (2) - (1) = d = a + 1. (5)
Since c cannot be greater than 9 considering c to be the maximum value 9 the value of d is 5.
If d = 5, using d = a + 1, a = 4.
Hence the maximum value of a = 4 when c = 9, d = 5.
Substituting b + 2*d = 11. b = 1.
The highest four-digit number satisfying the condition is 4195
Q5: How many 3-digit numbers are there, for which the product of their digits is more than 2 but less than 7?
Sol: Let the number be 'abc'. Then, 2 < a × b × c < 7. The product can be 3, 4, 5, 6.
We can obtain each of these as products with the combination 1,1, x where x = 3, 4, 5, 6.
Each number can be arranged in 3 ways, and we have 4 such numbers: hence, a total of 12 numbers fulfilling the criteria.
We can factories 4 as 2*2 and the combination 2, 2, 1 can be used to form 3 more distinct numbers.
We can factorize 6 as 2*3 and the combination 1, 2, 3 can be used to form 6 additional distinct numbers.
Thus a total of 12 + 3 + 6 = 21 such numbers can be formed.
Q6: How many 4-digit numbers, each greater than 1000 and each having all four digits distinct, are there with 7 coming before 3?
Sol: Here there are two cases possible
Case 1: When 7 is at the left extreme
In that case 3 can occupy any of the three remaining places and the remaining two places can be taken by (0, 1, 2, 4, 5, 6, 8, 9)
So total ways 3(8)(7)= 168
Case 2: When 7 is not at the extremes
Here there are 3 cases possible. And the remaining two places can be filled in 7(7) ways.(Remember 0 can't come on the extreme left)
Hence in total 3(7)(7)=147 ways
Total ways 168 + 147=315 ways
Q7: How many integers in the set {100, 101, 102, ..., 999} have at least one digit repeated?
Sol: Total number of numbers from 100 to 999 = 900
The number of three digits numbers with unique digits:
_ _ _
The hundredth's place can be filled in 9 ways ( Number 0 cannot be selected)
Ten's place can be filled in 9 ways
One's place can be filled in 8 ways
Total number of numbers = 9*9*8 = 648
Number of integers in the set {100, 101, 102, ..., 999} have at least one digit repeated = 900 - 648 = 252
Q8: In a six-digit number, the sixth, that is, the rightmost, digit is the sum of the first three digits, the fifth digit is the sum of first two digits, the third digit is equal to the first digit, the second digit is twice the first digit and the fourth digit is the sum of fifth and sixth digits. Then, the largest possible value of the fourth digit is
Sol: Let the six-digit number be ABCDEF
F = A + B + C, E= A + B, C=A, B= 2A, D= E + F.
Therefore D = 2A + 2B + C = 2A + 4A + A= 7A.
A cannot be 0 as the number is a 6 digit number.
A cannot be 2 as D would become 2 digit number.
Therefore A is 1 and D is 7.
Q9: Of 128 boxes of oranges, each box contains at least 120 and at most 144 oranges. X is the maximum number of boxes containing the same number of oranges. What is the minimum value of X?
(a) 5
(b) 103
(c) 6
(d) Cannot be determined
Ans: (c)
Sol: Each box contains at least 120 and at most 144 oranges.
So boxes may contain 25 different numbers of oranges among 120, 121, 122, .... 144.
Lets start counting.
1st 25 boxes contain different numbers of oranges and this is repeated till 5 sets as 25*5=125.
Now we have accounted for 125 boxes. Still 3 boxes are remaining. These 3 boxes can have any number of oranges from 120 to 144.
Already every number is in 5 boxes. Even if these 3 boxes have different number of oranges, some number of oranges will be in 6 boxes.
Hence the number of boxes containing the same number of oranges is at least 6.
Q10: Anita had to do a multiplication. In stead of taking 35 as one of the multipliers, she took 53. As a result, the product went up by 540. What is the new product?
(a) 1050
(b) 540
(c) 1440
(d) 1590
Ans: (a)
Sol: Let the number be X.
From the given information, 53x - 35x = 540 => 18x = 540 => x = 30
So, new product = 35*30 = 1050
Q11: Convert the number 1982 from base 10 to base 12. The result is:
(a) 1182
(b) 1912
(c) 1192
(d) 1292
Ans: (c)
Sol: Quotient of 1982/12 = 165, remainder = 2
Quotient of 165/12 = 13, remainder = 9
Quotient of 13/12 = 1, remainder = 1
Remainder of 1/12 = 1
So, the required number in base 12 = 1192
Q12: A is the set of positive integers such that when divided by 2, 3, 4, 5, 6 leaves the remainders 1, 2, 3, 4, 5 respectively. How many integers between 0 and 100 belong to set A?
(a) 0
(b) 1
(c) 2
(d) None of these
Ans: (b)
Sol: Let the number 'n' belong to the set A.
Hence, the remainder when n is divided by 2 is 1
The remainder when n is divided by 3 is 2
The remainder when n is divided by 4 is 3
The remainder when n is divided by 5 is 4 and
The remainder when n is divided by 6 is 5
So, when (n+1) is divisible by 2,3,4,5 and 6.
Hence, (n+1) is of the form 60k for some natural number k.
And n is of the form 60k-1
Between numbers 0 and 100, only 59 is of the form above.
Q13: How many five-digit numbers can be formed using the digits 2, 3, 8, 7, 5 exactly once such that the number is divisible by 125?
(a) 0
(b) 1
(c) 4
(d) 3
Ans: (c)
Sol: As we know for a number to be divisible by 125, its last three digits should be divisible by 125
So for a five digit number, with digits 2,3,8,7,5 its last three digits should be 875 and 375
Hence only 4 numbers are possible with its three digits as 875 and 375
I.e. 23875, 32875, 28375, 82375
Q14: A student instead of finding the value of 7/8 of a number, found the value of 7/18 of the number. If his answer differed from the actual one by 770, find the number.
(a) 1584
(b) 2520
(c) 1728
(d) 1656
Ans: (a)
Sol: let's say number is x.
So, 7x/8 - 7x/18 = 770
or x = 1584
Q15: P and Q are two positive integers such that PQ = 64. Which of the following cannot be the value of P + Q?
(a) 20
(b) 65
(c) 16
(d) 35
Ans: (d)
Sol: When PQ = 64
Possible values of P and Q are as follows:
64 and 1 ; 32 and 2 ; 16 and 4 ; 8 and 8
So possible sums are 65,34,20 and 16
Hence answer would be (d)
Q16: If a number 774958A96B is to be divisible by 8 and 9, the respective values of A and B will be
(a) 7 and 8
(b) 8 and 0
(c) 5 and 8
(d) None of these
Ans: (b)
Sol: According to the divisible rule of 9, the sum of all digits should be divisible by 9.
i.e. 55+A+B = 9k
So sum can be either 63 or 72.
For 63, A+B should be 8.
In given options, option B has values of A and B whose sum is 8 and by putting them we are having a number which divisible by both 9 and 8.
Hence answer will be B.
Q17: Three times the first of three consecutive odd integers is 3 more than twice the third. What is the third integer?
(a) 15
(b) 9
(c) 11
(d) 5
Ans: (a)
Sol: Suppose consecutive odd integers are: (a-2), a, (a+2)
Hence, 3a-6 = 2(a+2) + 3 => a=13
a+2 = 15
Q18: The sum of two integers is 10 and the sum of their reciprocals is 5/12. Then the larger of these integers is
(a) 2
(b) 4
(c) 6
(d) 8
Ans: (c)
Sol: let's say integers are x and y
so x + y = 10 ⇒ y = 10 - x
and 1/x + 1/y = 5/12
1/x + 1/10-x = 5/12
⇒ (10 - x + x)*12 = 5*x(10-x)
⇒ 120 = 50x - 5x2
⇒ 24 = 10x - x2
⇒ x = 4, 6
⇒ y = 6 or 4
The bigger of the two numbers is 6.
Q19: In Sivakasi, each boy's quota of match sticks to fill into boxes is not more than 200 per session. If he reduces the number of sticks per box by 25, he can fill 3 more boxes with the total number of sticks assigned to him. Which of the following is the possible number of sticks assigned to each boy?
(a) 200
(b) 150
(c) 125
(d) 175
Ans: (b)
Sol: Let the number of sticks assigned to each boy be N.
Let the number of boxes be M.
So, number of sticks per box = N/M
Now, if he reduces the number of sticks in each box, the equation becomes N/(M+3) = N/M - 25
So, 25 = N/M - N/(M+3)
From the options, if N = 150, then, we get 25 = 150 [ 1/M - 1/(M+3) ]
=> 1/6 = 1/M - 1/(M+3) => M = 3
So, the number of sticks assigned to each boy = 150
Q20: If 8 + 12 = 2, 7 + 14 = 3 then 10 + 18 = ?
(a) 10
(b) 4
(c) 6
(d) 18
Ans: (a)
Sol: 8+12 =( 20 = 2+0) = 2
7 + 14 =( 21 = 2+1) = 3
10 + 18= (28= 2+8) = 10
|
191 videos|131 docs|110 tests
|
FAQs on Practice Questions: Number System - Quantitative Aptitude (Quant) - CAT
| 1. What is the number system? |  |
| 2. What are the different types of number systems? |  |
| 3. How do you convert numbers between different number systems? |  |
| 4. What advantages does the binary number system have in computing? |  |
| 5. How can number systems be applied in real-life scenarios? |  |

|
Explore Courses for CAT exam
|

|

















