Class 9 Science Chapter 11 Practice Question Answers - Sound
Solved Examples
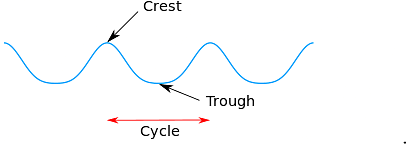
Q1: A source of wave produces 40 crests and 40 troughs in 0.4 seconds. Find the frequency of the wave.
 View Answer
View AnswerAns: Since there are 40 crests and 40 troughs in 0.4 seconds, there are 40 complete cycles in 0.4 seconds.
As we know that frequency is defined as the number of cycles per second
Hence, To find frequency, we can divide the number of cycles by the time in seconds:
Frequency = (Number of cycles) / (Time in seconds)
Frequency = 40 cycles / 0.4 seconds
Frequency = 100 cycles per second (Hz)
The frequency of the wave is 100 Hz.
Q2. A person has a hearing range from 20 Hz to 20 kHz. What are the typical wavelengths of sound waves in air corresponding to these two frequencies? Take the speed of sound in the air as 344 m s-1
 View Answer
View AnswerAns: Hearing range = 20 Hz to 20 kHz (= 20,000 Hz)
Speed of sound in the air = 344 ms-1
Since, for a wave, Velocity = Wavelength ✖ Frequency
Therefore,
- For, v = 20 Hz = 20s-1
λ = 344 / 20 = 17.2 m - For, v = 20,000 Hz = 20,000 s-1
λ = 344 / 20000 = 0.0172 m = 1.72 cm
Q3: Calculate the wavelength of a sound wave whose frequency is 220 Hz and speed is 440 m/s in a given medium.
 View Answer
View AnswerAns: Given: Frequency, f = 220 Hz, Speed of sound, v = 440 ms-1.
To Find: Wavelegth of sound wave
Since, for a sound wave, Velocity = Wavelength ✖ Frequency
⇒ 440 ms-1 = λ × 220 Hz = λ × 220 s-1
⇒ λ = 440 / 220 = 2 m
Thus, the wavelength of the sound wave is 2 m.
Q4: A person is listening to a sound of 50 Hz sitting at a distance of 450 m from the source of the sound. What is the time interval between successive compressions from the source reaches him?
 View Answer
View AnswerAns: Frequency of the sound, f = 50 Hz = 50 s-1 , Distance from the source, λ = 450 m.
As we know that, the time between the successive compressions is equal to the time taken by the sound to travel a distance equal to its wavelength.
⇒ Time period, T = 1/Frequency
⇒ T = 1/50 = 0.02 s
Thus, the successive compressions will reach the person after every 0.02 s.
Q5: A human heart, on average, is found to beat 75 times a minute. Calculate its frequency.
 View Answer
View AnswerAns: No. of beats of human heart = 75 min-1.
∵ 1 min = 60 sec.
⇒ No. of beats of human heart = 75/60 = 1.25 s-1
Thus, the average frequency of human heart beating = 1.25 s-1
Q6: A boat at anchor is rocked by waves whose consecutive crests are 100 m apart. The wave velocity of the moving crests is 20 m/s. What is the frequency of rocking of the boat?
 View Answer
View AnswerAns: Distance between two consecutive crests = wavelength (λ) = 100 m
Wave velocity, v = 20 ms-1
The distance between two consecutive crests is equal to the wavelength of the wave(λ).
⇒ Frequency = Velocity/Wavelength.
⇒ f = 20/100 = 0.2 s-1
Thus, the frequency of rocking of the boat is 0.2 s-1
Q7: A longitudinal wave is produced on a toy slinky. The wave travels at a speed of 30 cm/ s and the frequency of the wave is 20 Hz. What is the minimum separation between the consecutive compressions of the slinky
 View Answer
View AnswerAns: Wave speed, v = 30 cm/ s, Frequency of the wave, f = 20 Hz = 20 s-1.
The minimum separation between the consecutive compressions is equal to the wavelength (λ).
Since , for a wave, Velocity = Frequency ✖ Wavelength
⇒ Therefore, Wavelength, λ = 30/20 = 1.5 cm
Thus the minimum separation between the consecutive compression of the slinky is 1.5 cm.
Q8: A bat can hear a sound at frequencies up to 120 kHz. Determine the wavelength of sound in the air at this frequency. Take the speed of sound in the air as 344 m/s.
 View Answer
View AnswerAns: Frequency, f = 120 kHz = 120 × 103 Hz = 120 × 103 s-1
Velocity of sound in the air, v = 344 ms-1
∵ Wavelength, λ = Velocity/Frequency
⇒ λ = 344 / (120 x 103) = 2.87 × 10-3 m = 0.29 cm
Q9: A gun is fired in the air at a distance of 660 m, from a person. He hears the sound of the gun after 2s. What is the speed of sound?
 View Answer
View AnswerAns: Distance travelled by sound, λ = 660 m, Time taken by the sound, T = 2 s.
∵ Speed of sound, v = Distance travelled by sound /time taken by the sound
⇒ v = 660/2 = 330 m/s
Thus, The speed of sound in the air is 330 m/s.
Q10: A child hears an echo from a cliff 4 seconds after the sound from a powerful cracker is produced. How far away is the cliff from the child? The velocity of sound in air at 20°C is 344m/s.
 View Answer
View AnswerAns: Let the distance between the child and the cliff be d.
⇒ Total distance travelled by the sound = 2d
Total time taken by the sound, T = 4s,
Take, Velocity of sound, v = 344m/s
⇒ 2d = 344 × 4
d = 688 m
Thus, the cliff is at a distance of 688 m from the child.
Q11: A ship sends on a high-frequency sound wave and receives an echo after 1 second. What is the depth of the sea? Speed of sound in water is 1500 m/s.
 View Answer
View AnswerAns: Let, Depth of the sea = d
⇒ Total distance travelled by the sound wave = 2d
Time taken by sound to travel both ways, T = 1s
∵ Speed of the sound = 1500m/s.
⇒ 1500 m/s = 2d/1
⇒ d = (1500 x 1) / 2 = 750 m
Thus, the depth of the sea is 750 meters.
Q12: A sonar echo takes 2.2 s to return from a whale. How far away is the whale?
 View Answer
View AnswerAns: Total time taken by the signal = 2.2 s
⇒ Time taken the signal to reach the whale, T = 1.1 s
Let, Distance of the whale = d
From the literature, Speed of sound in seawater at 25°C, v = 1533 ms-1
∵ Distance of the whale, d = Speed of the signal x Time taken
⇒ d = 1533 ms-1 × 1.1 s = 1686.3 m.
Q13: Using the SONAR, sound pulses are emitted at the surface of water. These pulses, after being reflected from the bottom, are detected. If the time interval from the emission to the detection of the sound pulses is 2 seconds, find the depth of the water. Velocity of sound in water = 1498 m/s.
 View Answer
View AnswerAns: Let, depth of the water from the earth's surface = d.
⇒ Total distance travelled by the pulse = 2d
Total time taken by the pulse, T = 2 s.
⇒ Velocity of the sound, v = 2d/2 = d ms-1
∵ Velocity = 1498 m/s.
⇒ d = 1498 m/s × 1s = 1498 m
Thus, the depth of water is 1498 m.
Q14. A wave moves a distance of 8 m in 0.05 s.
(a) Find the velocity of the wave.
(b) What is the wavelength of the wave if its frequency is 200 Hz?
 View Answer
View AnswerAns: Distance covered by wave = 8 m, Time taken = 0.05 s.
(a) Velocity = 8 / 0.05 = 160 ms-1
(b) λ = v/f = 0.8m
Q15. Two children are at opposite ends of an iron pipe. One strikes his end of the iron pipe with a stone. Find the ratio of times taken by the sound waves in the air and in iron to reach the other child. Given the velocity of sound in air is 344 ms-1 and that in iron is 5130 ms-1.
 View Answer
View AnswerAns: The distance travelled by sound waves through air and iron is the same, which is equal to the thickness (s) of the rod.
Let, The distance travelled = d.
For air, jet the speed be v1 and time be t1.
⇒ d = v1t1----(i)
For iron, let the speed be v2 and time be t2.
⇒ d = v2t2----(ii)
From (i) and (ii), v1t1 = v2t2
⇒ t1/t2 = 5130 / 344 = 14.9
Q16. A boat at anchor is rocked by waves whose consecutive crests are 100 m apart. The wave velocity of the moving crests is 20 m/s. What is the frequency of rocking of the boat?
 View Answer
View AnswerAns: Wavelength, λ = 100 m, velocity, v = 20 m/s.
∵ Frequency = Velocity/ Wavelength.
⇒ v = 20/100 = 0.2 Hz
Q17. A stone is dropped into a well 44.1 m deep. The splash is heard 3.13 seconds after the stone is dropped. Find the velocity of sound in air.
 View Answer
View AnswerAns: For the downward motion of the stone:
s = ut + (1/2) at2
Here, a = acceleration due to gravity, g = 9.8m/s
⇒ 44.1 = (1/2) x 9.8 x t2
⇒ t = 3 s
For reflected sound wave:
Time taken to travel reflected sound wave = 3.13-3 = 0.13 s
d = vt
⇒ 44.1 = v ✖ 0.13
⇒ v = 339.23 ms-1
Q18. Using sonar, sound pulses are emitted at the surface of water. These pulses, after being reflected from water bottom, are detected. If the time interval from the emission to the detection of the sound pulses is 2 seconds, find the depth of the water. [speed of sound in water = 1531 m/s given].
 View Answer
View AnswerAns: Let's assume depth = d
Distance travelled by the sound between emission and detection will be two times of depth, i.e. 2d.
⇒ 2d = vt, where v = velocity of sound, t = total time
⇒ d = vt/2
⇒ d = (1531 x 2)/2 = 1531 m.
|
88 videos|369 docs|67 tests
|
FAQs on Class 9 Science Chapter 11 Practice Question Answers - Sound
| 1. What is sound? |  |
| 2. How does sound travel? |  |
| 3. What is the speed of sound? |  |
| 4. How does sound change with distance? |  |
| 5. How does sound travel through different mediums? |  |

|
Explore Courses for Class 9 exam
|

|


















