Q1: An analysis of the monthly incentives received by 5 salesmen: The mean and median of the incentives is $7000. The only mode among the observations is $12,000. Incentives paid to each salesman were in full thousands. What is the difference between the highest and the lowest incentive received by the 5 salesmen in the month?
(a) $4000
(b) $13,000
(c) $9000
(d) $5000
(e) $11,000
Ans: (e)
Explanation: Step 1 of solving this GMAT Statistics Question: Understanding the given data
The arithmetic mean of the incentives is $7000.
The median of the incentives is also $7000.
There is only one mode and the mode is $12,000.
Step 2 of solving this GMAT Statistics Question: Decoding Mean and Median
Let their incentives be a, b, c, d, and e such that a ≤ b ≤ c ≤ d ≤ e
Therefore, the median of these values is 'c'.
The median incentive is $7000. So, c = $7000.
Essentially, the incentives are __ __ 7000 __ __
The arithmetic mean of the incentives is $7000.
So, the sum of their incentives a + b + c + d + e = 5 * 7000 = $35,000
Step 3 of solving this GMAT Statistics Question: Decoding Mode
There is only one mode amongst these 5 observations.
The mode is that value that appears with the maximum frequency.
Hence, $12,000 is the incentive received by the most number of salesmen.
So, the incentives are __ __ 7000, 12000, 12000
Step 4 of solving this GMAT Statistics Question: Putting it all together
The incentive that c has got is $7000
The incentive received by d and e are 12,000 each
Therefore, c + d + e = 7000 + 12,000 + 12,000 = $31,000
Hence, a + b = 35,000 - 31,000 = $4000
As there is only one mode, the incentives received by a and b have to be different.
So, a received $1000 and b received $3000.
Maximum incentive: $12,000
Minimum incentive: $1000
Difference between maximum and minimum incentive: $11,000
Example 2: If the average of 5 positive integers is 40 and the difference between the largest and the smallest of these 5 numbers is 10, what is the maximum value possible for the largest of these 5 integers?
(a) 50
(b) 52
(c) 49
(d) 48
(e) 44
Ans: (d)
Explanation: The average of 5 positive integers is 40. i.e., the sum of these integers = 5 × 40 = 200
Let the least of these 5 numbers be x.
Because the range of the set is 10, the largest of these 5 numbers will be x + 10.
If we have to maximize the largest of these numbers, we have to minimize all the other numbers.
That is 4 of these numbers are all at the least value possible = x.
So, x + x + x + x + x + 10 = 200
Or x = 38.
So, the maximum value possible for the largest of these 5 integers is 48.
Example 3: Positive integers from 1 to 45, inclusive are placed in 5 groups of 9 each. What is the highest possible average of the medians of these 5 groups?
(a) 25
(b) 31
(c) 15
(d) 26
(e) 23
Ans: (b)
Explanation: We are dividing the numbers into sets of 9. So, we will have 5 sets.
The median of each set is the middle number when the 9 numbers are arranged in ascending order.
So, the median is the 5th number when the numbers in a set are written in ascending order.
Therefore, we will have 4 numbers that are greater than the median in each set.
We need to maximize the median in each group in order to maximize the average of all the medians.
The highest possible median is 41 as there should be 4 numbers greater than the median in a group of 9.
So, if we have a group that has a, b, c, d, 41, 42, 43, 44, 45, the median will be 41.
In this set, it is essential not to expend any more high values on a, b, c, or d as these do not affect the median.
For example, the median of a group that comprises 1, 2, 3, 4, 41, 42, 43, 44, 45 will be 41.
The next group can be 5, 6, 7, 8, 36, 37, 38, 39, 40. The median will be 36.
The first 4 numbers in any set is not of much consequence. Our focus is on the numbers upward of the median in any set.
Extrapolating the findings in the two sets listed above, to maximize medians in all the 5 groups, the medians of the 5 groups will have to be 21, 26, 31, 36, and 41.
The average of the highest possible medians will be the average of these 5 numbers = 31.
Example 4: If m, s are the average and standard deviation of integers a, b, c, and d, is s > 0?
I. m > a
II. a + b + c + d = 0
(a) Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient to answer the question asked.
(b) Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient to answer the question asked.
(c) BOTH statements (1) and (2) TOGETHER are sufficient to answer the question asked, but NEITHER statement ALONE is sufficient to answer the question asked.
(d) EACH statement ALONE is sufficient to answer the question asked.
(e) Statements (1) and (2) TOGETHER are NOT sufficient to answer the question asked, and additional data specific to the problem are needed.
Ans: (a)
Explanation: Step 1 of solving this GMAT DS question: Understand the Question Stem
What kind of an answer will the question fetch?
The question is an "Is" question. Answer to an "is" questions is either YES or NO.
When is the data sufficient?
The data is sufficient if we are able to get a DEFINITE Yes or No.
If the statements do not have adequate data to determine whether the standard deviation is greater than 0, the data is NOT sufficient.
When will the standard deviation be 0?
The standard deviation, s, will be 0 in two instances.
when all the elements in the set are the same.
the set contains only one element, which in this case is not possible.
Step 2 of solving this GMAT DS question:
Evaluating Statement (1) ALONE: m > a
If a = b = c = d, the average m will be the same as a.
Since m > a, all the elements in the set cannot be the same, and therefore, s > 0.
Statement 1 ALONE is sufficient.
Eliminate choices B, C and E. Choices narrow down to A or D.
Step 3 of solving this GMAT DS question:
Evaluating Statement (2) ALONE: a + b + c + d = 0
Approach: Look for a counter example
Example: When a = b = c = d = 0, s = 0
Counter Example: When a = -4, b = 0, c = 0, and d = 4, s > 0
Statement 2 ALONE is NOT sufficient.
Eliminate choice D.
Statement 1 ALONE is sufficient. Choice A is the answer.
Example 5: The average age of a group of 10 students was 20. The average age increased by 2 years when two new students joined the group. What is the average age of the two new students who joined the group?
(a) 22 years
(b) 30 years
(c) 44 years
(d) 32 years
(e) None of these
Ans: (d)
Explanation:
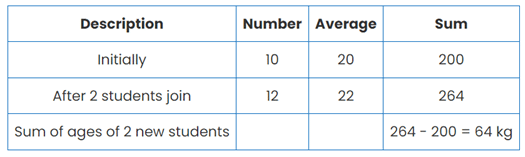
The average age of a group of 10 students is 20.
Therefore, the sum of the ages of all 10 of them = 10 * 20 = 200
When two new students join the group, the average age increases by 2. New average = 22.
Now, there are 12 students.
Therefore, the sum of the ages of all 12 of them = 12 × 22 = 264
Therefore, the sum of the ages of the two new students who joined = 264 - 200 = 64
And the average age of each of the two new students = 64/2 = 32 years.
Example 6: The average of 5 numbers is 6. The average of 3 of them is 8. What is the average of the remaining two numbers?
(a) 4
(b) 5
(c) 3
(d) 3.5
(e) 0.5
Ans: (c)
Explanation: The average of 5 quantities is 6.
Therefore, the sum of the 5 numbers is 5 × 6 = 30.
The average of three of these 5 numbers is 8.
Therefore, the sum of these three numbers = 3 × 8 = 24
The sum of the remaining two numbers = (Sum of all 5 - Sum of 3 numbers) = 30 - 24 = 6.
Average of these two remaining numbers = 6/2 = 3
Example 7: The average wages of a worker during a fortnight comprising 15 consecutive working days was $90 per day. During the first 7 days, his average wages was $87/day and the average wages during the last 7 days was $92 /day. What was his wage on the 8th day?
(a) $83
(b) $92
(c) $90
(d) $97
(e) $104
Ans: (d)
Explanation:
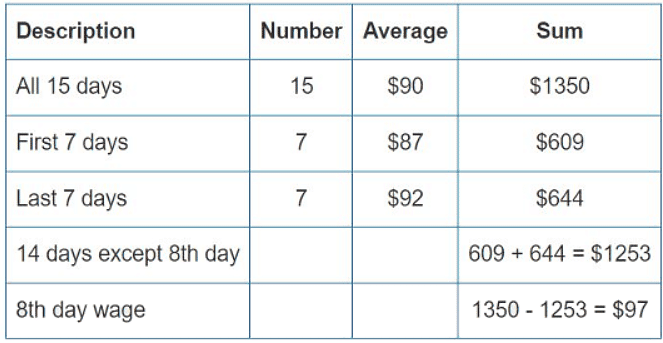
Fill in the data given in the question in the standard framework as shown above. Most questions in averages can be solved by completing this standard framework. The sum of wages is computed as the product of the number of days and the average wage earned per day.
The sum of wages earned during the 15 days that the worker worked = 15 × 90 = $1350.
The sum of wages earned during the first 7 days = 7 × 87 = $609.
The sum of wages earned during the last 7 days = 7 × 92 = $644.
Sum of wages earned for all 15 days = wages during first 7 days + wage on 8th day + wages during the last 7 days.
Or, 1350 = 609 + wage on 8th day + 644
Wage on 8th day = 1350 - 609 - 644 = $97.
Example 8: The average weight of a group of 30 friends increases by 1 kg when the weight of their football coach was added. If average weight of the group after including the weight of the football coach is 31 kg, what is the weight of their football coach?
(a) 31 kg
(b) 61 kg
(c) 60 kg
(d) 62 kg
(e) 91 kg
Ans: (b)
Explanation: The group comprises 30 friends.
When the football coach is also included, the average weight of the group becomes 31 kg.
As the new average is 1 kg more than the old average, old average without including the football coach = 30 kg.
Standard Framework to Solve Averages Questions
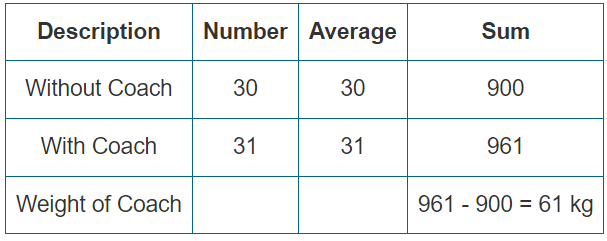
The total weight of the 30 friends without including the football coach = 30 * 30 = 900.
After including the football coach, the number of people in the group increases to 31 and the average weight of the group increases by 1 kg.
Therefore, the total weight of the group after including the weight of the football coach = 31 * 31 = 961 kg.
Therefore, the weight of the football coach = 961 - 900 = 61 kg.
Example 9: The arithmetic mean of the 5 consecutive integers starting with 's' is 'a'. What is the arithmetic mean of 9 consecutive integers that start with s + 2?
(a) 2 + s + a
(b) 22 + a
(c) 2s
(d) 2a + 2
(e) 4 + a
Ans: (e)
Explanation: Step 1 of solving this GMAT Statistics Question: Understanding the given data
The first sequence of 5 consecutive numbers starts with 's' and its mean is 'a'
The mean of 5 consecutive numbers is the 3rd term - the middle term.
Hence, 'a' the mean is the middle (3rd) term.
Step 2 of solving this GMAT Averages Question: Relating 'a' to 's'
The first sequence starts with 's'
Hence, the terms are s, s + 1, s + 2, s + 3, and s + 4
The middle term is s + 2
Therefore, a = s + 2
Step 3 of solving this GMAT Averages Question: The second series and its mean
The second series of 9 consecutive numbers starts from s + 2
The terms will therefore, be s + 2, s + 3, s + 4, s + 5, s + 6, s + 7, s + 8, s + 9, and s + 10
The average of these 9 numbers is the middle term of the second series. i.e., the 5th term = s + 6
If a = s + 2, then s + 6 will be a + 4
The average of the second sequence is a + 4
Alternative Approach
The fastest way to solve such questions is to assume a value for 's'.
Let s be 1
Therefore, the 5 consecutive integers that start with 1 are 1, 2, 3, 4, and 5
The average of these 5 numbers is the middle term, which is 3. Hence, a = 3
9 consecutive integers that start with s + 2 will start from 1 + 2 = 3
The second sequence is therefore, 3, 4, 5, 6, 7, 8, 9, 10, and 11
The average of these 9 number is the middle term, which is 7
If the average of the first sequence 3 = a, the average of the second sequence 7 = 4 + a.
Example 10: If the mean of numbers 28, x, 42, 78 and 104 is 62, what is the mean of 48, 62, 98, 124 and x?
(a) 78
(b) 58
(c) 390
(d) 310
(e) 66
Ans: (a)
Explanation: Step 1 of solving this GMAT Statistics Question: Find the sum of both the series
x is common to both the series. So, x is not going to make a difference to the average.
Only the remaining 4 numbers will contribute to the difference in average between the two series.
Sum of the 4 numbers, excluding x, of the first series is 28 + 42 + 78 + 104 = 252
Sum of the 4 numbers, excluding x, of the second series is 48 + 62 + 98 + 124 = 332
Step 2 of solving this GMAT Statistics Question: Difference in the sum of the two series
The difference between the sum of the two sets of numbers = 332 - 252 = 80
Step 3 of solving this GMAT Statistics Question: Calculating the average of the second series
The sum of the second series is 80 more than the sum of the first series.
If the sum of the second series is 80 more than that of the first series, the average of the second series will be 80/5 = 16 more than the first series.
Therefore, the average of the second series = 62 + 16 = 78.
Alternative Approach
Observe that each number in the new series, with the exception of "x", has increased by 20
This will increase the overall sum of the second series by 4 × 20 = 80
The overall sum increasing by 80 is the equivalent of each number in the series increasing by 80/5 = 16
If each number in the series increases by 16 the average will increase by 16 to 78.
























