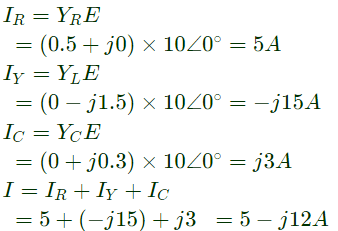Q1: The value of parameters of the circuit shown in the figure are
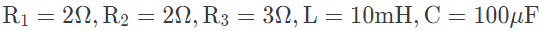 For time t < 0, the circuit is at steady state with the switch 'K' in closed condition. If the switch is opened at t = 0, the value of the voltage across the inductor (VL) at t = 0+in Volts is _____ (Round off to 1 decimal place). (2023)
For time t < 0, the circuit is at steady state with the switch 'K' in closed condition. If the switch is opened at t = 0, the value of the voltage across the inductor (VL) at t = 0+in Volts is _____ (Round off to 1 decimal place). (2023)
 (a) 6.5
(a) 6.5
(b) 8
(c) 12.5
(d) 16
Ans: (b)
Sol: Case (i) t < 1
At steady state, capacitor behaves as open circuit and inductor behaves as short circuit.
Redraw the circuit:
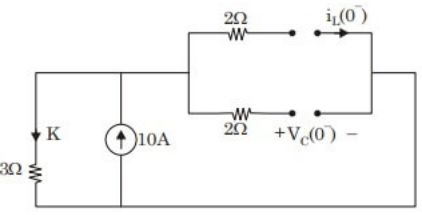 Using current division,
Using current division,
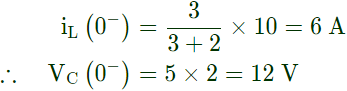 Case (ii) t > 0 :
Case (ii) t > 0 :
Switch is opened.
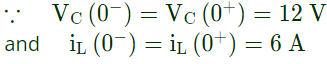 Redraw the circuit t = 0+:
Redraw the circuit t = 0+:
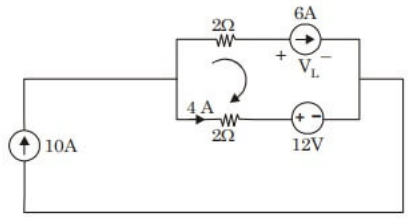 Apply KVL in loop,
Apply KVL in loop, 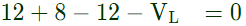
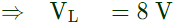
Q2: The network shown below has a resonant frequency of 150 kHz and a bandwidth of 600 Hz. The Q-factor of the network is __________. (round off to nearest integer) (2022)
(a) 250
(b) 100
(c) 150
(d) 450
Ans: (a)
Sol: 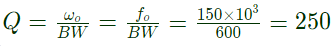
Q3: An inductor having a Q-factor of 60 is connected in series with a capacitor having a Q-factor of 240. The overall Q-factor of the circuit is ________. (round off to nearest integer) (2022)
(a) 12
(b) 24
(c) 48
(d) 96
Ans: (c)
Sol: We have, overall Q-factor of given circuit is,

Q4: A 0.1 μF capacitor charged to 100 V is discharged through a 1 kΩ resistor. The time in ms (round off to two decimal places) required for the voltage across the capacitor to drop to 1V is ______ (2019)
(a) 0.25
(b) 0.65
(c) 0.45
(d) 0.85
Ans: (c)
Sol: 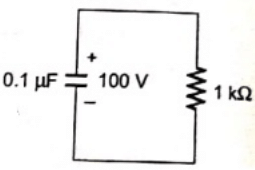
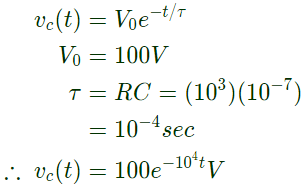 Let the time required by the voltage across the capacitor to drop to 1 V is t1,
Let the time required by the voltage across the capacitor to drop to 1 V is t1,
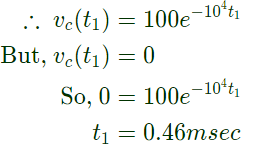
Q5: The voltage across the circuit in the figure, and the current through it, are given by the following expressions:
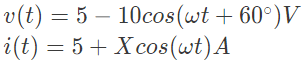 where ω = 100π radian/s. If the average power delivered to the circuit is zero, then the value of X (in Ampere) is _____ (up to 2 decimal places). (2018)
where ω = 100π radian/s. If the average power delivered to the circuit is zero, then the value of X (in Ampere) is _____ (up to 2 decimal places). (2018)
 (a) 5
(a) 5
(b) 10
(c) 15
(d) 20
Ans: (b)
Sol: Given that,
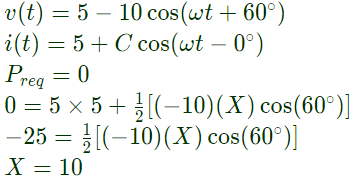
Q6: In the figure, the voltages are
v1(t) = 100cos(ωt),
v2(t) = 100cos(ωt + π/18) and
v3(t) = 100cos(ωt + π/36).
The circuit is in sinusoidal steady state, and R<<ωL. P1, P2 and P3 are the average power outputs. Which one of the following statements is true? (2018)
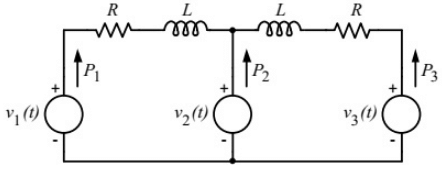
(a) P1 = P2 = P3 = 0
(b) P1 < 0, P2 > 0, P3 > 0
(c) P1 < 0, P2 > 0, P3 < 0
(d) P1 > 0, P2 < 0, P3 > 0
Ans: (c)
Sol: 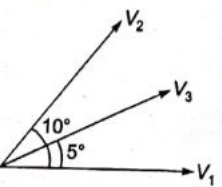
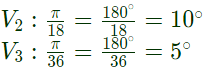 V2 leads V1 and V3,
V2 leads V1 and V3,
So, V2 is a source, V1 and V3 are absorbing.
Hence, P2 > 0 and P1, P3 < 0
Q7: The voltage (V) and current (A) across a load are as follows.
v(t) = 100sin(ωt),
i(t) = 10sin(ωt − 60°) + 2sin(3ωt) + 5sin(5ωt).
The average power consumed by the load, in W, is___________. (SET-2 (2016))
(a) 100
(b) 150
(c) 200
(d) 250
Ans: (d)
Sol: The average power consumed by the load = 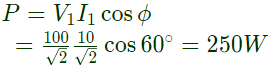
Q8: A resistance and a coil are connected in series and supplied from a single phase, 100 V, 50 Hz ac source as shown in the figure below. The rms values of plausible voltages across the resistance (VR) and coil (VC) respectively, in volts, are (SET-2 (2016))
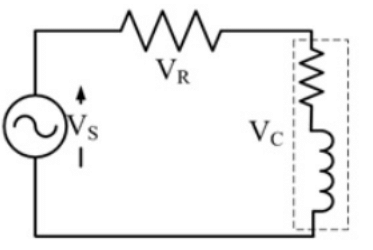 (a) 65, 35
(a) 65, 35
(b) 50, 50
(c) 60, 90
(d) 60, 80
Ans: (d)
Sol: It is a series RL circuit and
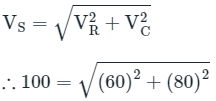
Q9: In the circuit shown below, the supply voltage is 10sin (1000t) volts. The peak value of the steady state current through the 1Ω resistor, in amperes, is ______. (SET-1 (2016))
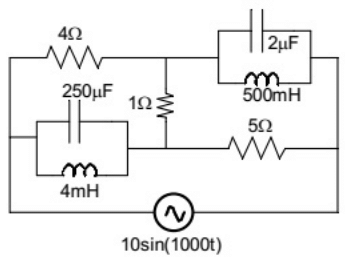 (a) 0.5
(a) 0.5
(b) 1
(c) 2
(d) 3
Ans: (b)
Sol: If we observe the parallel LC combination, we get that at ω = 1000 rad/sec the parallel LC is at resonance, thus it is open circuited.
The circuit given in question can be redrawn as
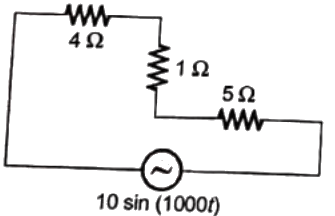 So,
So, 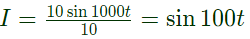 So, peak value is 1 Amp.
So, peak value is 1 Amp.
Q10: A symmetrical square wave of 50% duty cycle has amplitude of ±15 V and time period of 0.4 π ms. This square wave is applied across a series RLC circuit with R = 5 Ω, L = 10 mH, and C = 4 μF. The amplitude of the 5000 rad/s component of the capacitor voltage (in Volt) is ______. (SET-2 (2015))
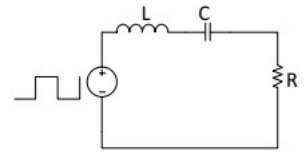 (a) 100
(a) 100
(b) 140
(c) 192
(d) 212
Ans: (c)
Q11: The total power dissipated in the circuit, shown in the figure, is 1 kW.
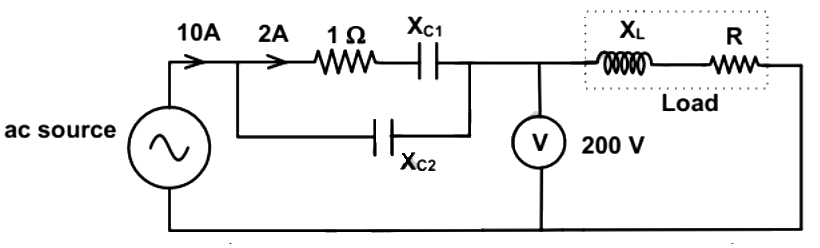 The voltmeter, across the load, reads 200 V. The value of XL is _____. (SET-2 (2014)
The voltmeter, across the load, reads 200 V. The value of XL is _____. (SET-2 (2014)
(a) 8.5
(b) 17.3
(c) 22.4
(d) 28.6
Ans: (b)
Sol: 
Given, total power dissipated in the circuit = 1kW =1000 Watt
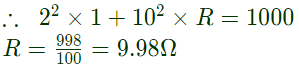 Also, voltage drop across R,
Also, voltage drop across R,
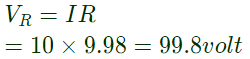 Voltage drop across load,
Voltage drop across load,
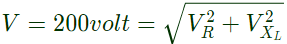 ∴ voltage drop across inductor,
∴ voltage drop across inductor,
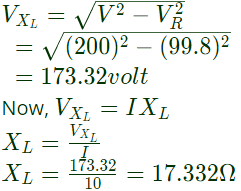
Q12: In the circuit shown, the three voltmeter readings are V1 = 220 V , V2 = 122 V , V3 = 136 V.
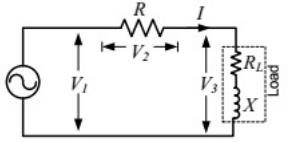 If RL = 5Ω, the approximate power consumption in the load is (2012)
If RL = 5Ω, the approximate power consumption in the load is (2012)
(a) 700 W
(b) 750 W
(c) 800 W
(d) 850 W
Ans: (b)
Sol: Given,
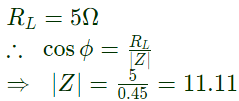 Power consumed by load,
Power consumed by load,
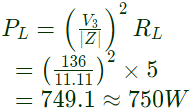
Q13: In the circuit shown, the three voltmeter readings are V1 = 220 V , V2 = 122 V , V3 = 136 V.
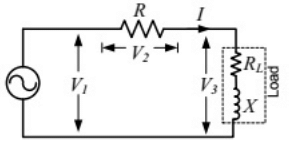 The power factor of the load is (2012)
The power factor of the load is (2012)
(a) 0.45
(b) 0.5
(c) 0.55
(d) 0.6
Ans: (a)
Sol: 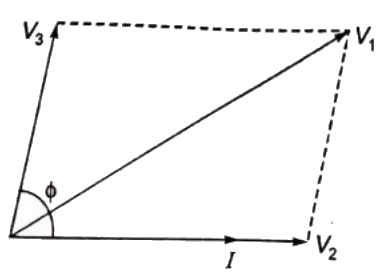
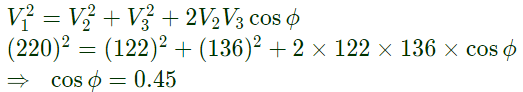
Q14: The average power delivered to an impedance (4 − j3)Ω by a current 5cos(100 πt+100)A is (2012)
(a) 44.2 W
(b) 50 W
(c) 62.5 W
(d) 125 W
Ans: (b)
Sol: 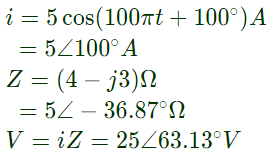 The average power is
The average power is
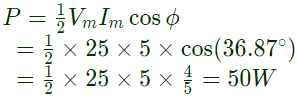 Alternate Method:
Alternate Method:
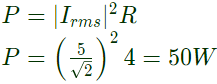
Q15: An RLC circuit with relevant data is given below.
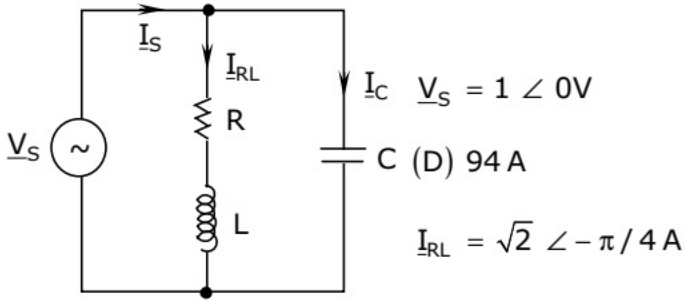 The current IC in the figure above is (2011)
The current IC in the figure above is (2011)
(a) -j2A
(b) 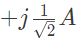
(c) 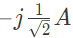
(d) +j2A
Ans: (d)
Sol: Using KCL,
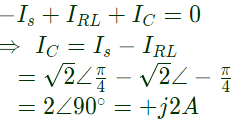
Q16: An RLC circuit with relevant data is given below.
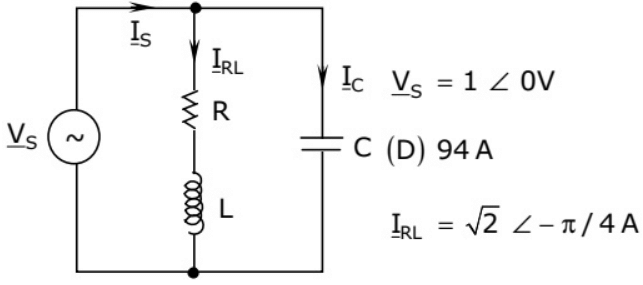 The power dissipated in the resistor R is (2011)
The power dissipated in the resistor R is (2011)
(a) 0.5 W
(b) 1 W
(c) 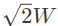
(d) 2W
Ans: (b)
Sol: Power supplied by the source = VsIs cosϕ
Where, ϕ= angle between Vs and Is = π/4
Inductor and capacitor do not consume power. Therefore, power dissipated in R = Power supplied by the source
PR = VSIS cosϕ
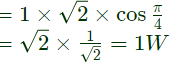
Q17: The voltage applied to a circuit is 100√2𝑐𝑜𝑠(100𝜋𝑡) volts and the circuit draws a current of 10√2sin(100πt + π/4) amperes. Taking the voltage as the reference phasor, the phasor representation of the current in amperes is (2011)
(a) 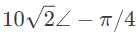
(b) 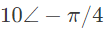
(c) 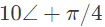
(d) 
Ans: (b)
Sol: 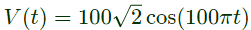 Voltage represented in phasor form:
Voltage represented in phasor form:
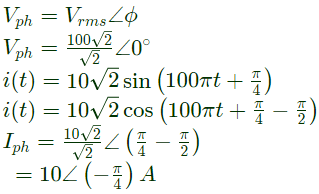
Q18: The r.m.s value of the current i(t) in the circuit shown below is (2011)
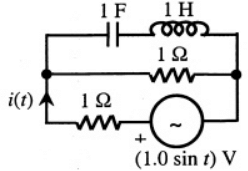 (a) 1/2 A
(a) 1/2 A
(b) 1/√2 A
(c) 1 A
(d) √2 A
Ans: (b)
Sol: 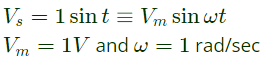 Impedance of the branch containing inductor and capacitor
Impedance of the branch containing inductor and capacitor
 So, this branch is short circuit and the whole current flow through it
So, this branch is short circuit and the whole current flow through it
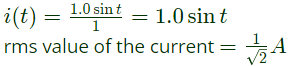
Q19: An energy meter connected to an immersion heater (resistive) operating on an AC 230 V, 50 Hz, AC single phase source reads 2.3 units (kWh) in 1 hour. The heater is removed from the supply and now connected to a 400 V peak to peak square wave source of 150 Hz. The power in kW dissipated by the heater will be (2006)
(a) 3.478
(b) 1.739
(c) 1.54
(d) 0.87
Ans: (b)
Sol: Assuming resistance of the heater = R
(i) When heater connected to 230 V, 50 Hz source, energy consumed by the heater = 2.3 units or 2.3 kWh in 1 hour
Power consumed by the heater
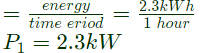 rms value of the input voltage
rms value of the input voltage
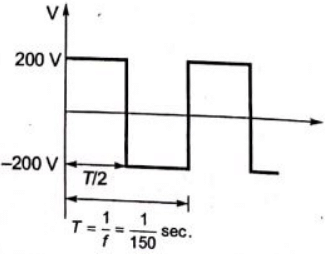
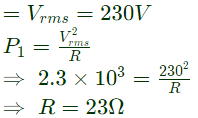 (ii) When heater connected to 400 V (peak to peak) square wave source of 150Hz
(ii) When heater connected to 400 V (peak to peak) square wave source of 150Hz
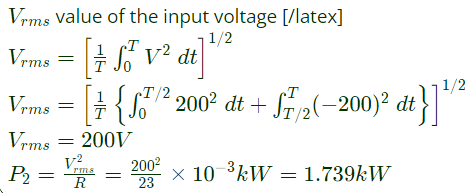
Q20: The RL circuit of the figure is fed from a constant magnitude, variable frequency sinusoidal voltage source VIN. At 100 Hz, the R and L elements each have a voltage drop μRMS. If the frequency of the source is changed to 50 Hz, then new voltage drop across R is (2005)
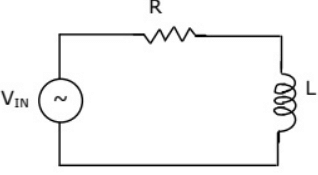 (a)
(a) 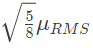
(b) 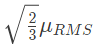
(c) 
(d) 
Ans: (c)
Sol: At f = 100Hz
∣VR∣ = ∣VL∣
as R and L are series connected, current through R and L is same, so
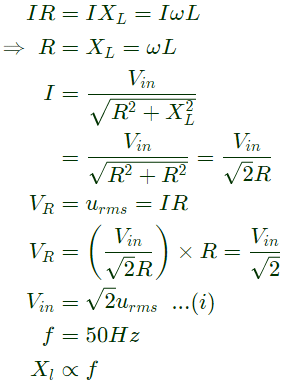
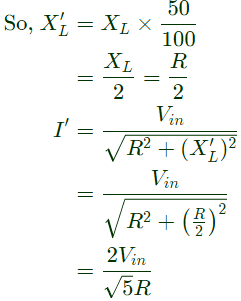
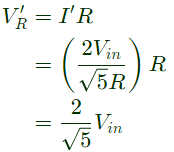
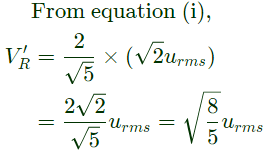
Q21: The RMS value of the voltage u(t)= 3 + 4 cos (3t) is (2005)
(a) √17 V
(b) 5V
(c) 7V
(d) (3+2√2)V
Ans: (a)
Sol: R.M.S. value of d.c voltage 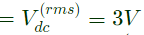 R.M.S. value of a.c. voltage
R.M.S. value of a.c. voltage
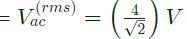 Therefore, R.M.S. value of the voltage
Therefore, R.M.S. value of the voltage
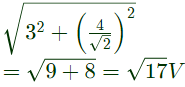
Q22: In figure, the admittance values of the elements in Siemens are YR = 0.5 + j0, YL = 0 - j1.5, YC = 0 + j0.3 respectively. The value of I as a phasor when the voltage E across the elements is 10∠0∘ V is (2004)
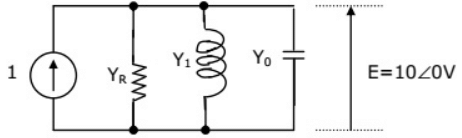 (a) 1.5 + j0.5
(a) 1.5 + j0.5
(b) 5 - j18
(c) 0.5 + j1.8
(d) 5 - j12
Ans: (d)
Sol: 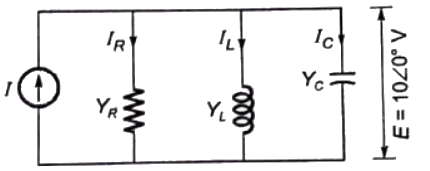
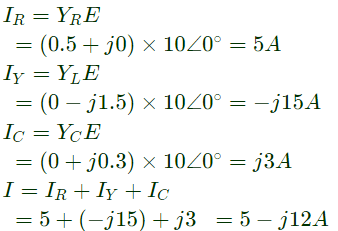
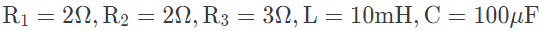 For time t < 0, the circuit is at steady state with the switch 'K' in closed condition. If the switch is opened at t = 0, the value of the voltage across the inductor (VL) at t = 0+in Volts is _____ (Round off to 1 decimal place). (2023)
For time t < 0, the circuit is at steady state with the switch 'K' in closed condition. If the switch is opened at t = 0, the value of the voltage across the inductor (VL) at t = 0+in Volts is _____ (Round off to 1 decimal place). (2023) (a) 6.5
(a) 6.5 Using current division,
Using current division,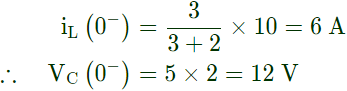 Case (ii) t > 0 :
Case (ii) t > 0 :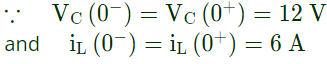 Redraw the circuit t = 0+:
Redraw the circuit t = 0+: Apply KVL in loop,
Apply KVL in loop, 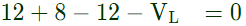
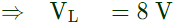
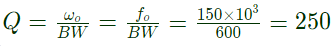


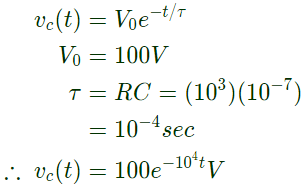 Let the time required by the voltage across the capacitor to drop to 1 V is t1,
Let the time required by the voltage across the capacitor to drop to 1 V is t1,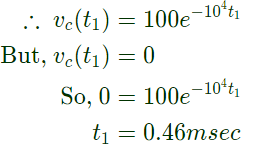
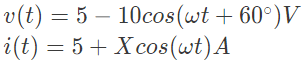 where ω = 100π radian/s. If the average power delivered to the circuit is zero, then the value of X (in Ampere) is _____ (up to 2 decimal places). (2018)
where ω = 100π radian/s. If the average power delivered to the circuit is zero, then the value of X (in Ampere) is _____ (up to 2 decimal places). (2018) (a) 5
(a) 5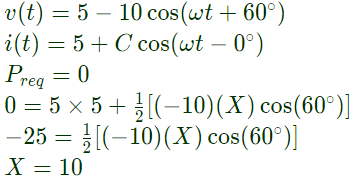


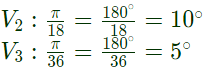 V2 leads V1 and V3,
V2 leads V1 and V3,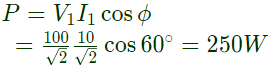
 (a) 65, 35
(a) 65, 35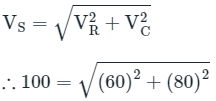
 (a) 0.5
(a) 0.5 So,
So, 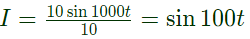 So, peak value is 1 Amp.
So, peak value is 1 Amp. (a) 100
(a) 100 The voltmeter, across the load, reads 200 V. The value of XL is _____. (SET-2 (2014)
The voltmeter, across the load, reads 200 V. The value of XL is _____. (SET-2 (2014)
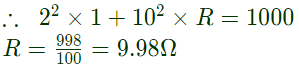 Also, voltage drop across R,
Also, voltage drop across R,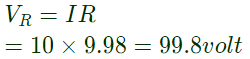 Voltage drop across load,
Voltage drop across load,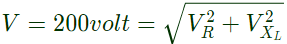 ∴ voltage drop across inductor,
∴ voltage drop across inductor,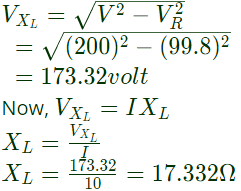
 If RL = 5Ω, the approximate power consumption in the load is (2012)
If RL = 5Ω, the approximate power consumption in the load is (2012)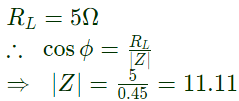 Power consumed by load,
Power consumed by load,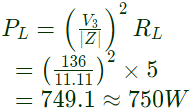
 The power factor of the load is (2012)
The power factor of the load is (2012)
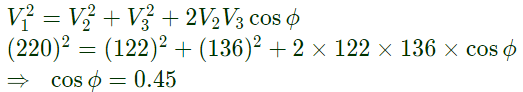
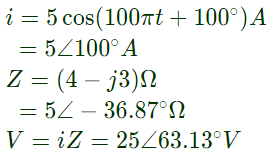 The average power is
The average power is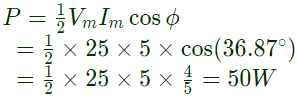 Alternate Method:
Alternate Method: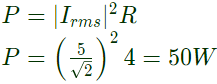
 The current IC in the figure above is (2011)
The current IC in the figure above is (2011)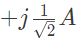
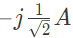
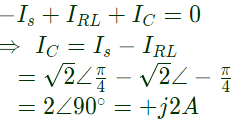
 The power dissipated in the resistor R is (2011)
The power dissipated in the resistor R is (2011)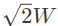
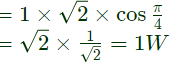
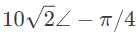
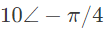
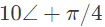

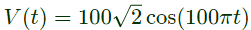 Voltage represented in phasor form:
Voltage represented in phasor form: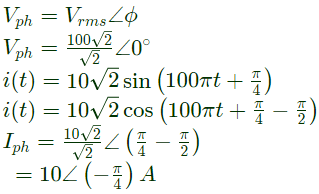
 (a) 1/2 A
(a) 1/2 A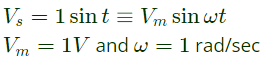 Impedance of the branch containing inductor and capacitor
Impedance of the branch containing inductor and capacitor So, this branch is short circuit and the whole current flow through it
So, this branch is short circuit and the whole current flow through it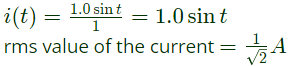
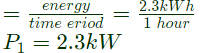 rms value of the input voltage
rms value of the input voltage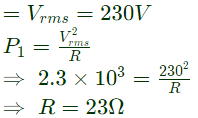 (ii) When heater connected to 400 V (peak to peak) square wave source of 150Hz
(ii) When heater connected to 400 V (peak to peak) square wave source of 150Hz
 (a)
(a) 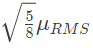
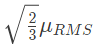


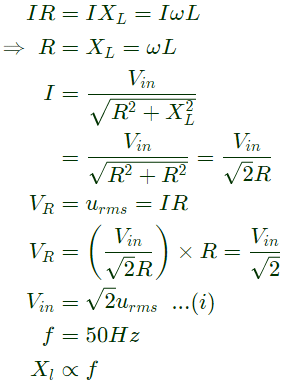
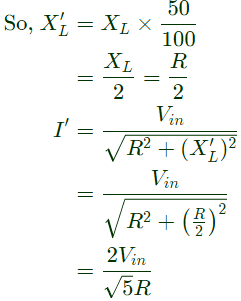
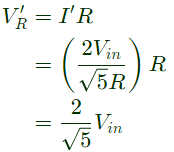
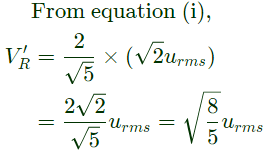
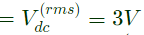 R.M.S. value of a.c. voltage
R.M.S. value of a.c. voltage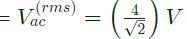 Therefore, R.M.S. value of the voltage
Therefore, R.M.S. value of the voltage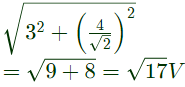
 (a) 1.5 + j0.5
(a) 1.5 + j0.5