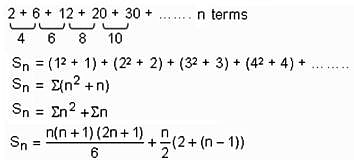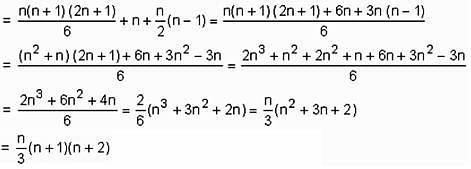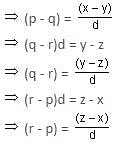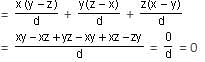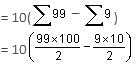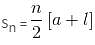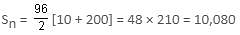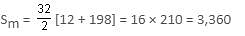This EduRev document offers 10 Multiple Choice Questions (MCQs) from the topic Progressions, Sequences & Series (Level - 1). These questions are of Level - 1 difficulty and will assist you in the preparation of CAT & other MBA exams. You can practice/attempt these CAT Multiple Choice Questions (MCQs) and check the explanations for a better understanding of the topic.
Question for Practice Questions Level 1: Progressions, Sequences & Series - 2
Try yourself:Four positive numbers are in geometric progression. Their sum is 156. The sum of the first and the third numbers is 1/5th of the sum of the second and the fourth numbers. Find the first number.
Explanation
a, ar, ar2, ar3
a + ar + ar2 + ar3 = 156
a + ar2 = 1/5 (ar + ar3)
5a + 5ar2 = ar + ar3
5 + 5r2 = r + r3
r3 - 5r2 + r - 5 = 0
r2 (r - 5) + 1(r - 5) = 0
r = 5, r = ±1
a + 5a + 25a + 125a = 156
156a = 156
a = 1
Report a problem
Question for Practice Questions Level 1: Progressions, Sequences & Series - 2
Try yourself:If a, b, c, d, e and f are AMs between 2 and 12, then a + b + c + d + e + f is equal to
Explanation
As, 2, a, b, c, d, e, f, 12 are in AP
So, 2 + a + b + c + d + e + f + 12 = 8/2 (2 + 12)
2 + a + b + c + d + e + f + 12 = 4 × (2 + 12)
a + b + c + d + e + f = 4 × (2 + 12) - 2 - 12
a + b + c + d + e + f = 42
Report a problem
Question for Practice Questions Level 1: Progressions, Sequences & Series - 2
Try yourself:2 + 6 + 12 + 20 + 30 + … up to n terms equals
Explanation
Report a problem
Question for Practice Questions Level 1: Progressions, Sequences & Series - 2
Try yourself:The sum of 3rd and 15th terms of an arithmetic progression is equal to the sum of 6th, 11th and 13th terms of the same progression. Which term of the given progression should necessarily be equal to zero?
Explanation
Let the AP be a, a + d, a + 2d, …
∵ Tn = a + (n - 1)d
T3 + T15 = T6 + T11 + T13
2a + 2d + 14d = 3a + 5d + 10d + 12d
0 = a + 11d
0 = T12
Thus, the 12th term of the AP is 0.
Report a problem
Question for Practice Questions Level 1: Progressions, Sequences & Series - 2
Try yourself:If the pth, qth, rth terms of an AP are x, y, z, respectively, then x(q - r) + y(r - p) + z(p - q) is equal to
Explanation
a + (p - 1)d = x … (i)
a + (q - 1)d = y … (ii)
a + (r - 1)d = z … (iii)
Subtracting (ii) from (i), (ii) from (iii) & (iii) from (i), we get
⇒ (p - q)d = x - y

Given: x(q - r) + y(r - p) + z(p - r)

Report a problem
Question for Practice Questions Level 1: Progressions, Sequences & Series - 2
Try yourself:What is the sum of all 3-digit numbers, which end with zero?
Explanation
100 + 110 + 120 + ……… + 990
= 10(10 + 11 + …… + 99)

= 10(4950 – 45) = 49,050
Report a problem
Question for Practice Questions Level 1: Progressions, Sequences & Series - 2
Try yourself:If the fifth term of an AP is twice its third term, then the first term of the AP is
Explanation
t5 = 2t3
(a + 4d) = 2(a + 2d)
⇒ a = 0
Report a problem
Question for Practice Questions Level 1: Progressions, Sequences & Series - 2
Try yourself:If a, b, c are in AP and x, y, z are in GP, then xb - c. yc - a. za - b equals
Explanation
Since a, b, c are in AP, therefore
b - a = c - b = d and c - a = 2d.
∴ xb - c × yc - a × za - b = x-d × y2d × z-d
= (xz)-d × y2d = y-2d × y2d = 1. (∵ y2 = xz)
Report a problem
Question for Practice Questions Level 1: Progressions, Sequences & Series - 2
Try yourself:The sum of 3rd and 15th terms of an arithmetic progression is equal to the sum of 6th, 11th and 13th terms of the same progression. Which term of the given progression should necessarily be equal to zero?
Explanation
Let the AP be a, a + d, a + 2d, …
∵ Tn = a + (n - 1)d
T3 + T15 = T6 + T11 + T13
2a + 2d + 14d = 3a + 5d + 10d + 12d
0 = a + 11d
0 = T12
Thus, the 12th term of the AP is 0.
Report a problem
Question for Practice Questions Level 1: Progressions, Sequences & Series - 2
Try yourself:Find the sum of all even numbers from 10 to 200 (inclusive), excluding those, which are multiples of 6.
Explanation
Report a problem