Class 8 Exam > Class 8 Notes > RD Sharma Solutions for Class 8 Mathematics > RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math
Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math RD Sharma Solutions | RD Sharma Solutions for Class 8 Mathematics PDF Download
Question 8: By what number should (−5) −1 be multiplied so that the product is (−8) −1
Answer 8:
Let the number be x.
According to the question,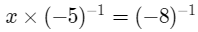 
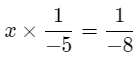
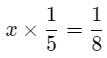
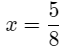
Question 9: By what number should (15) −1 be multiplied so that the product may be equal to (−5) −1 ?
Answer 9:
Let the required number be x.
Therefore,
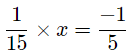
x=−3
Hence, the required number is −3.
Question 10:
By what number should (−15)−1 be divided so that the quotient may be equal to (−5)−1?
Answer 10:
Expressing in fractional form, we get:
(−15)−1 = −1/15, ---> (a−1 = 1/a)
and
(−5)−1 = −1/5 ---> (a−1 = 1/a)
We have to find a number x such that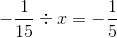
Solving this equation, we get: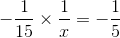
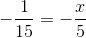
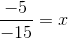
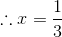Hence, (−15)−1 should be divided by 1/3 to obtain (−5)−1.
Question 11:
By what number should (5/3)−2 be multiplied so that the product may be (73)−1?
Answer 11:
Expressing as a positive exponent, we have: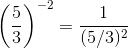
---> (a−1 = 1/a) 
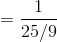---> ((a/b)n = (an)/(bn)) 
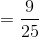and(7/3)−1 = 3/7. ---> (a−1 = 1/a)
We have to find a number x such that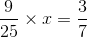
Multiplying both sides by 25/9, we get:
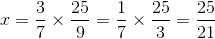Hence, (5/3)−2 should be multiplied by 25/21 to obtain (7/3)−1.
Question 12:
Find x, if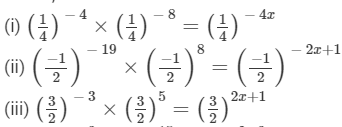
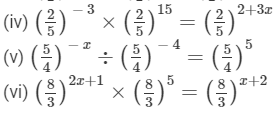
Answer 12:
(i) We have:
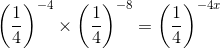
(am×an = am+n)
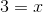
x = 3
(ii) We have:
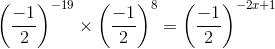
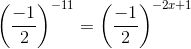(am×an = am+n)
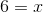
x = 6
(iii) We have:
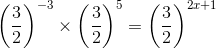
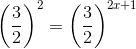
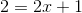
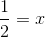
x = 1/2
(iv) We have:
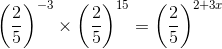
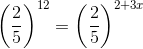
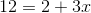
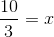
x = 10/3
(v) We have:
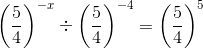
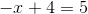
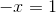
(vi) We have:
Question 13:
Answer 13:
(i) First, we have to find x.
x = 322×23-4 = Hence, x−2 is:
(ii) First, we have to find x.
Hence, the value of x−1 is:
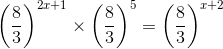
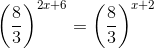
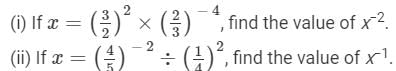
(i) First, we have to find x.
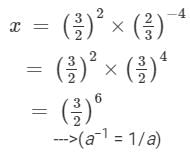x = 322×23-4 = Hence, x−2 is: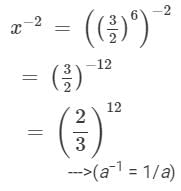
(ii) First, we have to find x.
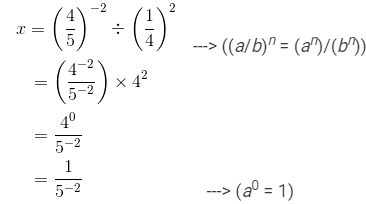
Hence, the value of x−1 is:
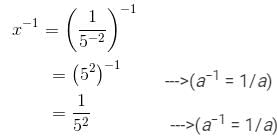
Question 14: Find the value of x for which 52x ÷ 5−3 = 55.
Answer 14:
We have: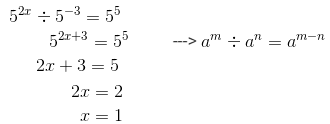
hence, x is 1.
The document Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math RD Sharma Solutions | RD Sharma Solutions for Class 8 Mathematics is a part of the Class 8 Course RD Sharma Solutions for Class 8 Mathematics.
All you need of Class 8 at this link: Class 8
FAQs on Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math RD Sharma Solutions - RD Sharma Solutions for Class 8 Mathematics
| 1. How do you find the value of a number raised to a power? |  |
Ans. To find the value of a number raised to a power, you need to multiply the number by itself as many times as the power indicates. For example, if you have to find the value of 2 raised to the power of 3 (2^3), you would multiply 2 by itself three times: 2 x 2 x 2 = 8. Therefore, 2^3 = 8.
| 2. What is the meaning of a negative exponent? | |
Ans. A negative exponent indicates the reciprocal of a number raised to a positive exponent. For example, if you have 2 raised to the power of -3 (2^-3), it means the reciprocal of 2^3, which is 1/2^3 or 1/8. Therefore, 2^-3 = 1/8.
| 3. How do you simplify expressions with exponents? | |
Ans. To simplify expressions with exponents, you can use the rules of exponents. These rules include multiplying exponents with the same base, dividing exponents with the same base, and raising a power to another power. By applying these rules, you can simplify complex expressions and make them easier to evaluate.
| 4. What is the difference between the exponent and the base of a power? | |
Ans. In a power, the base is the number that is multiplied by itself, while the exponent represents the number of times the base is multiplied. For example, in 3^2, 3 is the base and 2 is the exponent. The base is the number being raised to a power, and the exponent tells us how many times the base is multiplied.
| 5. How are powers used in real-life applications? | |
Ans. Powers are used in various real-life applications such as scientific calculations, engineering, finance, and computer programming. They help in modeling exponential growth and decay, calculating compound interest, analyzing population growth, and understanding physical quantities like distance, energy, and volume. Powers provide a concise way to express repeated multiplication and are essential in many fields of study and applications.
Related Exams
About this Document
|
4.94/5 Rating |
|
Nov 24, 2024 Last updated |
Document Description: RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math for Class 8 2024 is part of RD Sharma Solutions for Class 8 Mathematics preparation.
The notes and questions for RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math have been prepared according to the Class 8 exam syllabus. Information about RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math covers topics
like and RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math Example, for Class 8 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math.
Introduction of RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math in English is available as part of our RD Sharma Solutions for Class 8 Mathematics
for Class 8 & RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math in Hindi for RD Sharma Solutions for Class 8 Mathematics course.
Download more important topics related with notes, lectures and mock test series for Class 8
Exam by signing up for free. Class 8: Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math RD Sharma Solutions | RD Sharma Solutions for Class 8 Mathematics
Description
Full syllabus notes, lecture & questions for Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math RD Sharma Solutions | RD Sharma Solutions for Class 8 Mathematics - Class 8 | Plus excerises question with solution to help you revise complete syllabus for RD Sharma Solutions for Class 8 Mathematics | Best notes, free PDF download
Information about RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math
In this doc you can find the meaning of RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math defined & explained in the simplest way possible. Besides explaining types of
RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math theory, EduRev gives you an ample number of questions to practice RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math tests, examples and also practice Class 8
tests
|
Explore Courses for Class 8 exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
shortcuts and tricks
,video lectures
,Summary
,practice quizzes
,Semester Notes
,Viva Questions
,MCQs
,Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math RD Sharma Solutions | RD Sharma Solutions for Class 8 Mathematics
,Sample Paper
,Important questions
,Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math RD Sharma Solutions | RD Sharma Solutions for Class 8 Mathematics
,Objective type Questions
,Previous Year Questions with Solutions
,ppt
,Extra Questions
,Exam
,past year papers
,mock tests for examination
,study material
,Free
,Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math RD Sharma Solutions | RD Sharma Solutions for Class 8 Mathematics
;
Additional Information about RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math for Class 8 Preparation
RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math Free PDF Download
The RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math is an invaluable resource that delves deep into the core of the Class 8 exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math now and kickstart your journey towards success in the Class 8 exam.
Importance of RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math
The importance of RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math cannot be overstated, especially for Class 8 aspirants.
This document holds the key to success in the Class 8 exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math Notes
RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math Notes on EduRev are your ultimate resource for success.
RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math Class 8 Questions
The "RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math Class 8 Questions" guide is a valuable resource for all aspiring students preparing for the
Class 8 exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math on the App
Students of Class 8 can study RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of RD Sharma Solutions - Chapter 2 - Powers (Ex-2.2) Part- 2 Class 8 Math is prepared as per the latest Class 8 syllabus.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup