RD Sharma Solutions Ex-14.2, Quadrilaterals, Class 9, Maths | RD Sharma Solutions for Class 9 Mathematics PDF Download
Q.1. Two opposite angles of a parallelogram are (3x-2)0 and (50-x)0. Find the measure of each angle of the parallelogram.
Solution:
We know that,
Opposite sides of a parallelogram are equal.
(3x-2)0 = (50-x)0
⇒ 3x + x = 50 + 2
⇒ 4x = 52
⇒ x = 130
Therefore, (3x-2)0 = (3*13-2) = 370
(50-x)0 = (50-13) = 370
Adjacent angles of a parallelogram are supplementary.
∴ x+37 = 1800
∴ x = 1800−370 = 1430
Hence, four angles are : 370, 1430, 370, 1430.
Q.2. If an angle of a parallelogram is two-third of its adjacent angle, find the angles of the parallelogram.
Solution:
Let the measure of the angle be x.
Therefore, the measure of the angle adjacent is 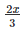
We know that the adjacent angle of a parallelogram is supplementary.
Hence 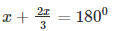
2x + 3x = 5400
⇒ 5x = 5400
⇒ x = 1080
Adjacent angles are supplementary
⇒ x + 1080 = 1800
⇒ x = 1800 – 1080 = 720
⇒ x = 720
Hence, four angles are 1800, 720, 1800, 720
Q.3. Find the measure of all the angles of a parallelogram, if one angle is 240 less than twice the smallest angle.
Solution:
x + 2x – 24 = 1800
⇒ 3x – 24 = 1800
⇒ 3x = 1080 + 24
⇒ 3x = 2040
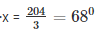
⇒ x = 680
⇒ 2x – 240 = 2*680 – 240 = 1120
Hence, four angles are 680, 1120, 680, 1120.
Q.4. The perimeter of a parallelogram is 22cm. If the longer side measures 6.5cm what is the measure of the shorter side?
Solution:
Let the shorter side be ‘x’.
Therefore, perimeter = x + 6.5 + 6.5 + x [Sum of all sides]
22 = 2 (x + 6.5)
11 = x + 6.5
⇒ x = 11 – 6.5 = 4.5cm
Therefore, shorter side = 4.5cm
Q.5. In a parallelogram ABCD, ∠D = 1350. Determine the measures of ∠Aand∠B.
Solution:
In a parallelogram ABCD
Adjacent angles are supplementary
So, ∠D+∠C = 1800
∠C = 1800−1350
∠C = 450
In a parallelogram opposite sides are equal.
∠A =∠C = 450
∠B = ∠D = 1350
Q. 6. ABCD is a parallelogram in which ∠A = 700. Compute ∠B,∠Cand∠D.
Solution:
In a parallelogram ABCD
∠A = 700
∠A+∠B = 1800 [ Since, adjacent angles are supplementary ]
700+∠B = 1800 [∵∠ A = 70°]
∠B = 1800−700
∠B =1100
In a parallelogram opposite sides are equal.
∠A = ∠C = 700
∠B = ∠D = 1100
Q.7. In Figure 14.34, ABCD is a parallelogram in which ∠A = 600. If the bisectors of ∠A,and∠B meet at P, prove that AD = DP, PC = BC and DC = 2AD.
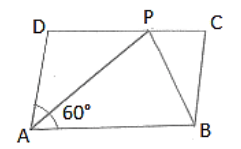
Solution:
AP bisects ∠A
Then, ∠DAP = ∠PAB = 300
Adjacent angles are supplementary
Then, ∠A+∠B = 1800
∠B+600 = 1800
∠B = 1800−600
∠B = 1200
BP bisects ∠B
Then, ∠PBA = ∠PBC = 300
∠PAB = ∠APD = 300 [Alternate interior angles]
Therefore, AD = DP [Sides opposite to equal angles are in equal length]
Similarly
∠PBA = ∠BPC = 600 [Alternate interior angles]
Therefore, PC = BC
DC = DP + PC
DC = AD + BC [Since, DP = AD and PC = BC]
DC = 2AD [Since, AD = BC, opposite sides of a parallelogram are equal]
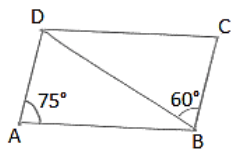
Q.8. In figure 14.35, ABCD is a parallelogram in which ∠DAB = 750 and ∠DBC = 600. Compute ∠CDB, and ∠ADB.
Solution:
To find ∠CDB and ∠ADB
∠CBD = ∠ABD = 600 [Alternate interior angle. AD║BC and BD is the transversal]
In ∠BDC
∠CBD+∠C+∠CDB = 1800 [Angle sum property]
⇒ 600+750+∠CDB =1800
⇒ ∠CDB = 1800−(600+750)
⇒ ∠CDB = 450
Hence, ∠CDB = 450,∠ADB = 600
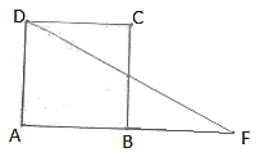
Q. 9. In figure 14.36, ABCD is a parallelogram and E is the mid-point of side BC. If DE and AB when produced meet at F, prove that AF = 2AB.
Solution:
In ΔBEFandΔCED
∠BEF = ∠CED [Verified opposite angle]
BE = CE [Since, E is the mid-point of BC]
∠EBF = ∠ECD [Since, Alternate interior angles are equal]
∴ ΔBEF ≅ ΔCED [ASA congruence]
∴ BF = CD[CPCT]
AF = AB + AF
AF = AB + AB
AF = 2AB.
Hence proved.
Q.10. Which of the following statements are true (T) and which are false (F)?
(i) In a parallelogram, the diagonals are equal.
(ii) In a parallelogram, the diagonals bisect each other.
(iii) In a parallelogram, the diagonals intersect each other at right angles.
(iv) In any quadrilateral, if a pair of opposite sides is equal, it is a parallelogram.
(v) If all the angles of a quadrilateral are equal, it is a parallelogram.
(vi) If three sides of a quadrilateral are equal, it is a parallelogram.
(vii) If three angles of a quadrilateral are equal, it is a parallelogram.
(viii) If all the sides of a quadrilateral are equal, it is a parallelogram.
Solution:
(i) False
(ii) True
(iii) False
(iv) False
(v) True
(vi) False
(vii) False
(viii) True
FAQs on RD Sharma Solutions Ex-14.2, Quadrilaterals, Class 9, Maths - RD Sharma Solutions for Class 9 Mathematics
| 1. What are the types of quadrilaterals covered in RD Sharma Solutions Ex-14.2? |  |
| 2. How do you prove that a quadrilateral is a parallelogram? |  |
| 3. What are the properties of a rectangle? |  |
| 4. How do you find the area of a square using RD Sharma Solutions Ex-14.2? |  |
| 5. How do you prove that a quadrilateral is a rhombus? |  |
















