Class 9 Exam > Class 9 Notes > RD Sharma Solutions for Class 9 Mathematics > RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths
RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths | RD Sharma Solutions for Class 9 Mathematics PDF Download
1. Assuming that x,y,z are positive real numbers, simplify each of the following
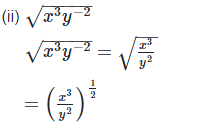
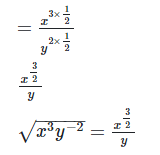
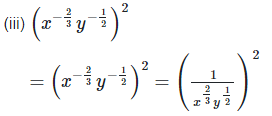
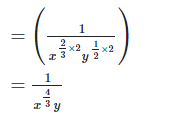
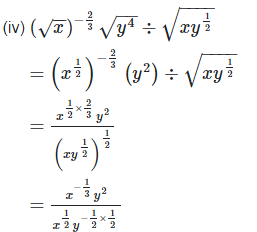
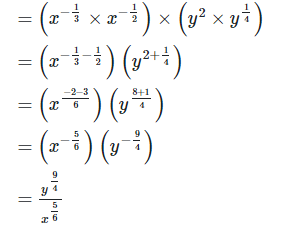
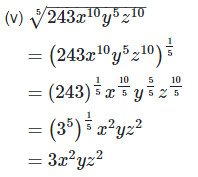
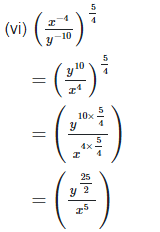
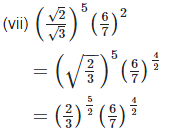
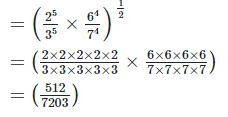
2. Simplify
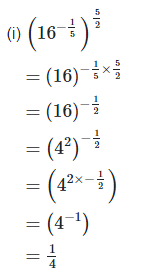
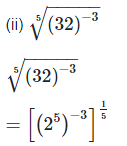
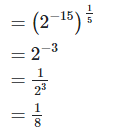
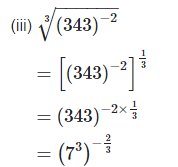
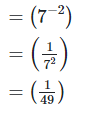
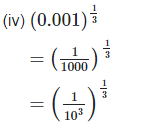
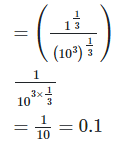
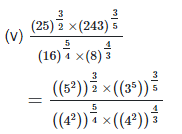
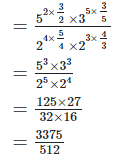
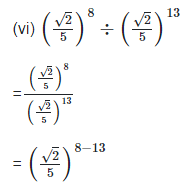
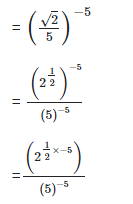
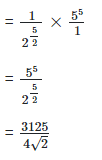
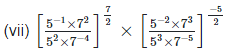
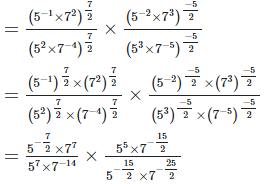
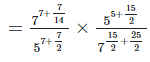
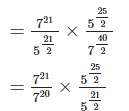
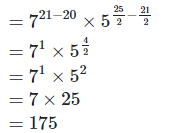
3. Prove that
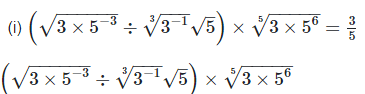
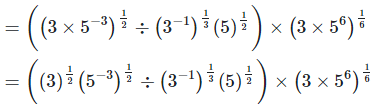
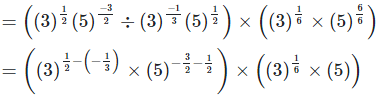
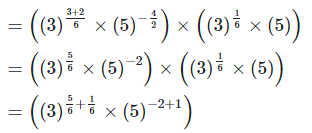
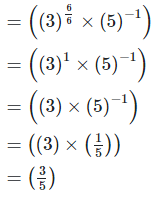
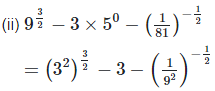
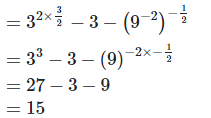
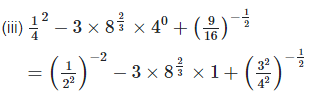
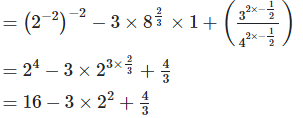
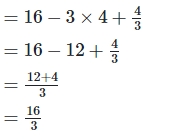
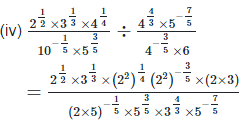
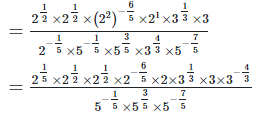
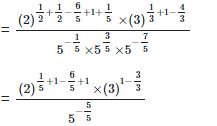
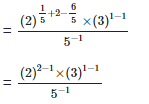
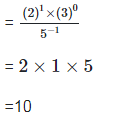
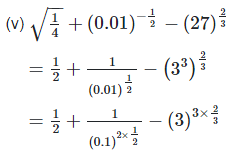
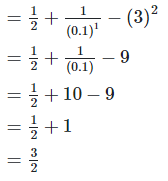
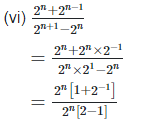
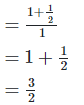
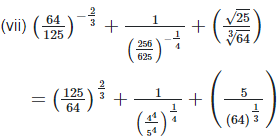
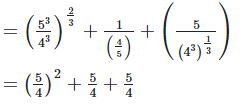
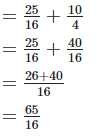
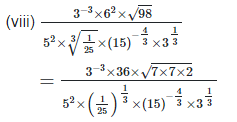
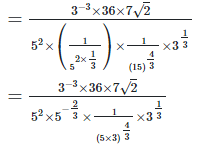
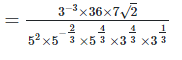
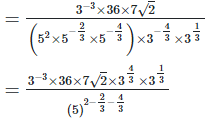
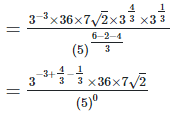
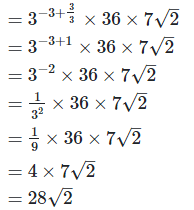
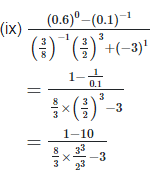
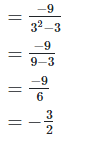
4. Show that
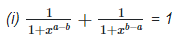
Left hand side (LHS) = Right hand side (RHS)
Considering LHS,
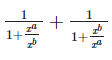
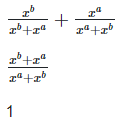
Therefore, LHS = RHS
Hence proved
(ii)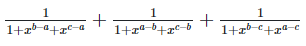 
Left hand side (LHS) = Right hand side (RHS)
Considering LHS,
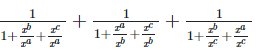
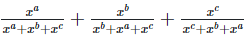
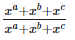
1
Therefore, LHS = RHS
Hence proved
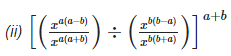
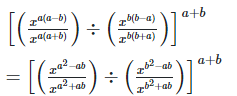
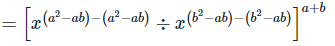
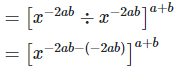
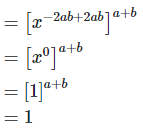
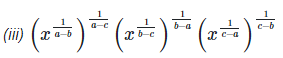
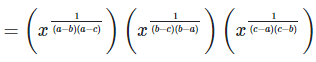
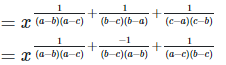
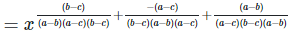
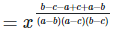
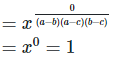
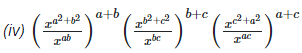
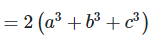
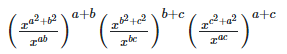
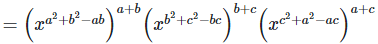
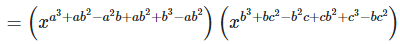
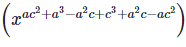
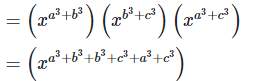
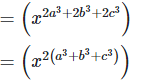
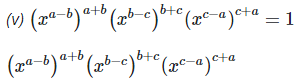
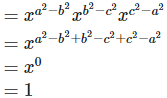
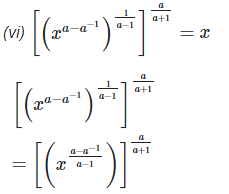
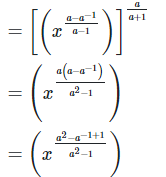
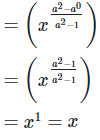
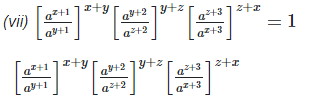
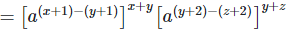
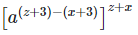
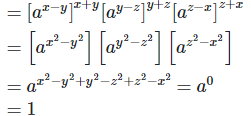
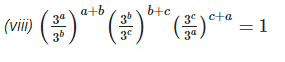
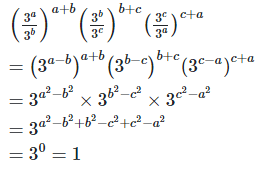
5. If 2x = 3y = 12z ,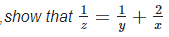 
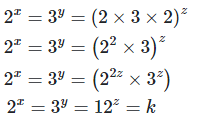
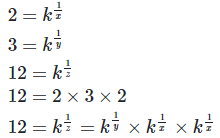
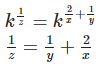
6. If 2x = 3y = 6−z ,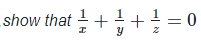 
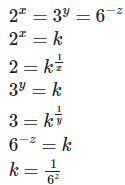
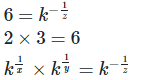
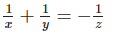[by equating exponents]
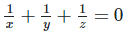
7. If ax = by = cz and b2 = ac , then show that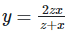 
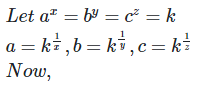
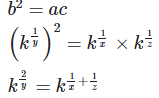
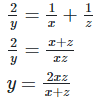
8. If 3x = 5y = (75)z, show that
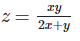
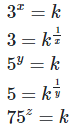
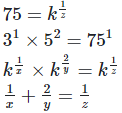
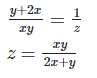
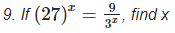
We have,
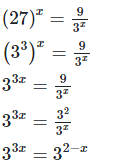
3x = 2 − x [On equating exponents]
3x + x = 2
4x = 2
x = 2/4
x = 12
Here the value of x is 1/2
10. Find the values of x in each of the following
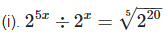
We have
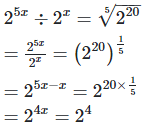
= 4x = 4 [On equatinge xponent]
x = 1
Hence the value of x is 1
(ii). (23)4 = (22)x
We have
(23)4 = (22)x
= 23×4 = 22×x
12 = 2x
2x = 12 [On equating exponents]
x = 6
Hence the value of x is 6
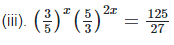
We have
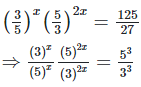
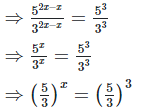
x = 3 [on equating exponents]
Hence the value of x is 3
(iv) 5x−2×32x−3 = 135
We have,
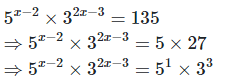
⇒ x − 2 = 1,2x − 3 = 3 [On equating exponents]
⇒ x = 2 + 1,2x = 3 + 3
⇒ x = 3, 2x = 6 ⇒ x = 3
Hence the value of x is 3
(v). 2x−7×5x−4 = 1250
We have
2x−7×5x−4 = 1250
⇒ 2x−7×5x−4 = 2×625
⇒ 2x−7×5x−4 = 2×54
⇒ x−7 = 1 ⇒ x = 8,x−4 = 4 ⇒ x = 8
Hence the value of x is 8
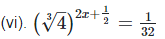
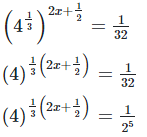
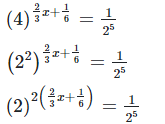
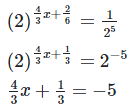
4x+1 = −15
4x = −15−1
4x = −16
x =−16/4
x = −4
Hence the value of x is 4
(vii). 52x+3 = 1
52x+3 = 1×50
2x+3 = 0 [By equatinge xponents]
2x = −3
x = −3/2
Hence the value of x is −3/2
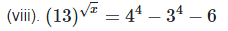
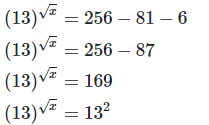
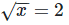[By equating exponents]
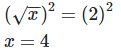
Hence the value of x is 4
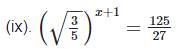
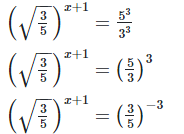
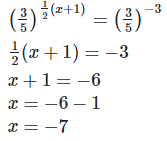
Hence the value of x is 7
The document RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths | RD Sharma Solutions for Class 9 Mathematics is a part of the Class 9 Course RD Sharma Solutions for Class 9 Mathematics.
All you need of Class 9 at this link: Class 9
FAQs on RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths - RD Sharma Solutions for Class 9 Mathematics
| 1. What are exponents of real numbers? |  |
Ans. Exponents of real numbers are a way to represent repeated multiplication of a number by itself. An exponent tells us how many times the base number is being multiplied by itself. For example, in the expression 2^3, the base number is 2 and the exponent is 3. It means that we multiply 2 by itself 3 times, resulting in 2^3 = 2 x 2 x 2 = 8.
| 2. How do you simplify expressions with exponents? | |
Ans. To simplify expressions with exponents, we use the properties of exponents. Here are some important properties:
- Product Rule: When multiplying two numbers with the same base, we add their exponents. For example, a^m * a^n = a^(m+n).
- Quotient Rule: When dividing two numbers with the same base, we subtract their exponents. For example, a^m / a^n = a^(m-n).
- Power Rule: When raising a number with an exponent to another exponent, we multiply the exponents. For example, (a^m)^n = a^(m*n).
- Zero Rule: Any number (except zero) raised to the power of zero is equal to 1. For example, a^0 = 1.
| 3. What is the difference between a positive exponent and a negative exponent? | |
Ans. A positive exponent indicates that the base number is being multiplied by itself a certain number of times. For example, 2^3 means multiplying 2 by itself 3 times, which is equal to 2 x 2 x 2 = 8.
On the other hand, a negative exponent indicates that the base number is being divided by itself a certain number of times. For example, 2^-3 means dividing 1 by 2 three times, which is equal to 1 / (2 x 2 x 2) = 1/8.
So, while a positive exponent leads to a larger value, a negative exponent leads to a smaller value.
| 4. How do you simplify expressions with negative exponents? | |
Ans. To simplify expressions with negative exponents, we can use the reciprocal property of exponents. When a number with a negative exponent is in the numerator, we can move it to the denominator and change the sign of the exponent to positive. Similarly, when a number with a negative exponent is in the denominator, we can move it to the numerator and change the sign of the exponent to positive.
For example, if we have 3^(-2), we can rewrite it as 1 / (3^2) = 1/9. And if we have 1 / 2^(-3), we can rewrite it as 2^3 = 8.
| 5. Can exponents of real numbers be fractions or decimals? | |
Ans. Yes, exponents of real numbers can be fractions or decimals. The rules of exponents apply to any real number, not just whole numbers. For example, 2^(1/2) is the square root of 2, which is approximately 1.414. Similarly, 2^0.5 is also the square root of 2.
Fractional or decimal exponents represent roots or powers that are not whole numbers. They can be used to calculate values like cube roots, fourth roots, and so on. The properties of exponents, such as the product rule and quotient rule, still apply to these fractional or decimal exponents.
Related Exams
About this Document
|
3.1K Views |
|
4.98/5 Rating |
|
Dec 23, 2024 Last updated |
Document Description: RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths for Class 9 2024 is part of RD Sharma Solutions for Class 9 Mathematics preparation.
The notes and questions for RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths have been prepared according to the Class 9 exam syllabus. Information about RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths covers topics
like and RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths Example, for Class 9 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths.
Introduction of RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths in English is available as part of our RD Sharma Solutions for Class 9 Mathematics
for Class 9 & RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths in Hindi for RD Sharma Solutions for Class 9 Mathematics course.
Download more important topics related with notes, lectures and mock test series for Class 9
Exam by signing up for free. Class 9: RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths | RD Sharma Solutions for Class 9 Mathematics
Description
Full syllabus notes, lecture & questions for RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths | RD Sharma Solutions for Class 9 Mathematics - Class 9 | Plus excerises question with solution to help you revise complete syllabus for RD Sharma Solutions for Class 9 Mathematics | Best notes, free PDF download
Information about RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths
In this doc you can find the meaning of RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths defined & explained in the simplest way possible. Besides explaining types of
RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths theory, EduRev gives you an ample number of questions to practice RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths tests, examples and also practice Class 9
tests
|
Explore Courses for Class 9 exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
Class 9
,Free
,Previous Year Questions with Solutions
,ppt
,video lectures
,RD Sharma Solutions Ex-2.2
,RD Sharma Solutions Ex-2.2
,study material
,shortcuts and tricks
,Sample Paper
,MCQs
,Exponents Of Real Numbers
,Class 9
,(Part - 1)
,Exponents Of Real Numbers
,(Part - 1)
,practice quizzes
,Exam
,past year papers
,Maths | RD Sharma Solutions for Class 9 Mathematics
,Summary
,Maths | RD Sharma Solutions for Class 9 Mathematics
,RD Sharma Solutions Ex-2.2
,Important questions
,(Part - 1)
,Viva Questions
,Exponents Of Real Numbers
,Semester Notes
,mock tests for examination
,Maths | RD Sharma Solutions for Class 9 Mathematics
,Extra Questions
,Class 9
,Objective type Questions
;
Additional Information about RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths for Class 9 Preparation
RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths Free PDF Download
The RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths is an invaluable resource that delves deep into the core of the Class 9 exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths now and kickstart your journey towards success in the Class 9 exam.
Importance of RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths
The importance of RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths cannot be overstated, especially for Class 9 aspirants.
This document holds the key to success in the Class 9 exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths Notes
RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths Notes on EduRev are your ultimate resource for success.
RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths Class 9 Questions
The "RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths Class 9 Questions" guide is a valuable resource for all aspiring students preparing for the
Class 9 exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths on the App
Students of Class 9 can study RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of RD Sharma Solutions Ex-2.2, (Part - 1), Exponents Of Real Numbers, Class 9, Maths is prepared as per the latest Class 9 syllabus.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup