Exercise 2.1 - Powers RD Sharma Solutions | Mathematics (Maths) Class 8 PDF Download
Q.1. Express each of the following as a rational number of the form 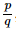 , where p and q are integers and q ≠ 0.
, where p and q are integers and q ≠ 0.
(i) 2−3
(ii) (−4)−2
(iii)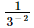
(iv)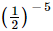
(v)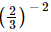
Ans: We know that a−n = 1/an. Therefore,
(i)
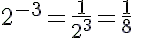
(ii)
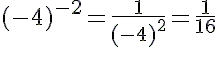
(iii)
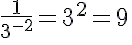
(iv)
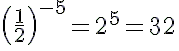
(v)
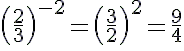
Q.2. Find the value of each of the following:
(i) 3−1 + 4−1
(ii) (30 + 4−1) × 22
(iii) (3−1 + 4−1 + 5−1)0
(iv) {(13)−1−(14)−1}−1
Ans:
(i) We know from the property of powers that for every natural number a, a−1 = 1/a. Then:
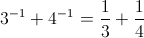 ---> (a−1 = 1/a)
---> (a−1 = 1/a)
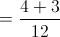
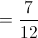
(ii) We know from the property of powers that for every natural number a, a−1 = 1/a.
Moreover, a0 is 1 for every natural number a not equal to 0. Then:
(30+4−1)×22
= 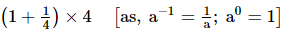
= 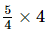
= 5
(iii) We know from the property of powers that for every natural number a, a−1 = 1/a.
Moreover, a0 is 1 for every natural number a not equal to 0. Then:
(3−1+4−1+5−1) = 1 ---> (Ignore the expression inside the bracket and use a0 = 1 immediately.)
(iv) We know from the property of powers that for every natural number a, a−1 = 1/a. Then:
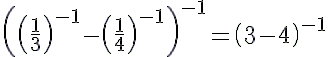 ---> (a−1 = 1/a)
---> (a−1 = 1/a)
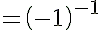
= 1
Q.3. Find the value of each of the following:
(i)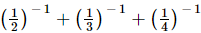
(ii)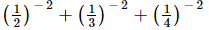
(iii) (2−1 × 4−1) ÷ 2−2
(iv) (5−1 × 2−1) ÷ 6−1
Ans:
(i)
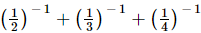 =
= 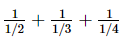 ---> (a−1 = 1/a)
---> (a−1 = 1/a)
= 2 + 3 + 4
= 12
(ii)
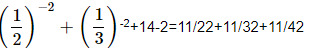
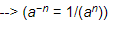
= 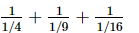
= 4 + 9 +16
= 29
(iii)
(2−1 × 4−1) ÷ 2−2 = 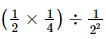
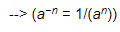
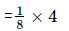
= 2
(iv)
(5−1 × 2−1) ÷ 6−1 = 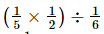
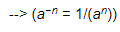
= 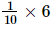
= 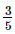
Q.4. Simplify:
(i) (4−1 × 3−1)2
(ii) (5−1 ÷ 6−1)3
(iii) (2−1 + 3−1)−1
(iv) (3−1 × 4−1)−1 × 5−1
Ans:
(i) (4−1 × 3−1)2
= 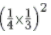
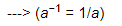
= 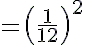
= 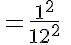
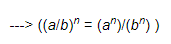
= 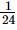
(ii)
(5−1 ÷ 6−1)3
= 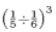
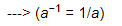
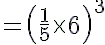
= 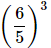
= 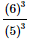
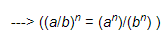
= 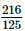
(iii)
(2−1 + 3−1)−1
= 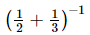
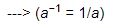
= 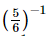
= 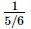
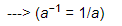
= 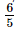
(iv)
(3−1 × 4−1)−1 × 5−1
= 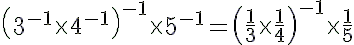
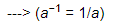
= 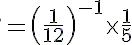
=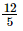
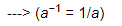
Q.5. Simplify:
(i)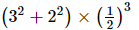
(ii)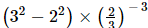
(iii)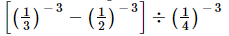
(iv)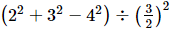
Ans:
(i)
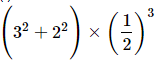 =
= 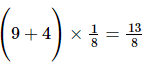
(ii)
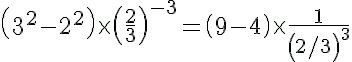
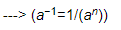
= 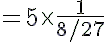
= 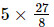
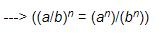
= 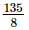
(iii)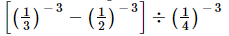
= 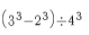
= (27−8)÷64
=19 × 1/64
=19/64
(iv)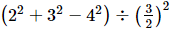
=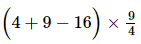
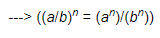
= 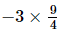
= 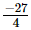
Q.6. By what number should 5−1 be multiplied so that the product may be equal to (−7)−1?
Ans: Using the property a−1 = 1/a for every natural number a, we have 5−1 = 1/5 and (−7)−1 = −1/7. We have to find a number x such that
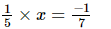
Multiplying both sides by 5, we get:
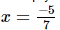
Hence, the required number is −5/7.
Q.7. By what number should (1/2)−1 be multiplied so that the product may be equal to (−4/7)−1?
Ans: Using the property a−1 = 1/a for every natural number a, we have (1/2)−1 = 2 and (−4/7)−1 = −7/4. We have to find a number x such that
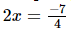
Dividing both sides by 2, we get:
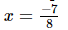
Hence, the required number is −7/8.
Q.8. By what number should (−15)−1 be divided so that the quotient may be equal to (−5)−1?
Ans: Using the property a−1 = 1/a for every natural number a, we have (−15)−1 = −1/15 and (−5)−1 = −1/5. We have to find a number x such that
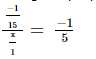
or 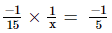
or 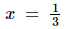
Hence, (−15)−1 should be divided by 1/3 to obtain (−5)−1.
|
79 videos|408 docs|31 tests
|
FAQs on Exercise 2.1 - Powers RD Sharma Solutions - Mathematics (Maths) Class 8
| 1. What are powers in mathematics? |  |
| 2. How do you calculate the value of a power? |  |
| 3. What is the difference between a base and an exponent in powers? |  |
| 4. What is the significance of powers in real-life applications? |  |
| 5. Can negative numbers be raised to a power? |  |

|
Explore Courses for Class 8 exam
|

|

















