Class 9 Exam > Class 9 Notes > Mathematics (Maths) Class 9 > RD Sharma Solutions: Rationalisation- 1
Rationalisation- 1 RD Sharma Solutions | Mathematics (Maths) Class 9 PDF Download
RD Sharma Solutions: Exercise 3.1 - Rationalisation
Q.1. Simplify each of the following:
(i)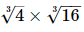
(ii)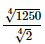
Proof: (i) We know that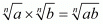
. We will use this property to simplify the expression
.
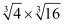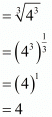
Hence the value of the given expression is 4.
(ii) We know that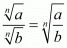
. We will use this property to simplify the expression
.
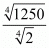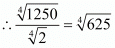
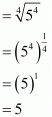
Hence the value of the given expression is 5.
Q.2. Simplify the following expressions:
(i) (4+√7) (3+√2)
(ii) (3+√3) (5−√2)
(iii) (√5−2) (√3−√5)
Proof: (i) We can simplify the expression (4+√7) (3+√2) as
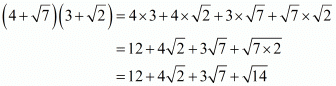
Hence the value of the expression is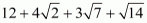
.
(ii) We can simplify the expression (3+√3) (5−√2) as
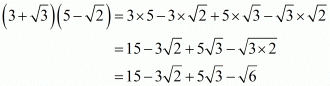
Hence the value of the expression is
.
(iii) We can simplify the expression (√5−2) (√3−√5) as
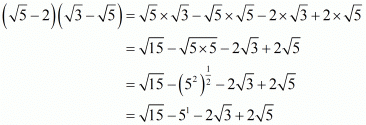
Hence the value of the expression is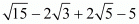
.
Q.3. Simplify the following expressions:
(i) (11+√11) (11−√11)
(ii) (5+√7) (5−√7)
(iii) (√8−√2) (√8+√2)
(iv) (3+√3)(3−√3)
(v) (√5−√2) (√5+√2)
Proof: (i) We know that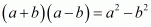
. We will use this property to simplify the expression (11+√11) (11−√11)
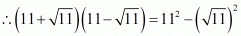
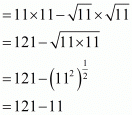
= 110
Hence the value of expression is 110.
(ii) We know that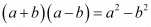
. We will use this property to simplify the expression (5+√7) (5−√7) .
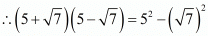
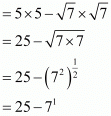
= 18
Hence the value of expression is 18.
(iii) We know that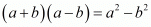
. We will use this property to simplify the expression
.
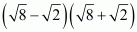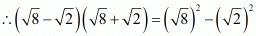
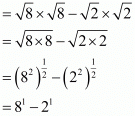
= 6
Hence the value of expression is 6
(iv) We know that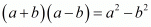
. We will use this property to simplify the expression
.
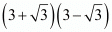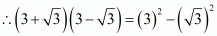
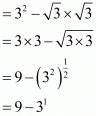
= 6
Hence the value of expression is 6.
(v) We know that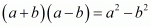
. We will use this property to simplify the expression
.
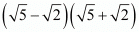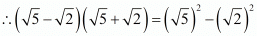
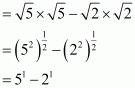
= 3
Hence the value of expression is 3.
Q.4. Simplify the following expressions:
(i) (√3+√7)2
(ii) (√5−√3)2
(iii) (2√5+3√2)2
Proof: (i) We know that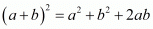
. We will use this property to simplify the expression
.
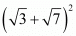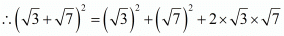
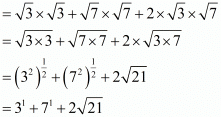
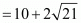
Hence the value of expression is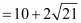
(ii) We know that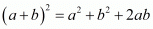
. We will use this property to simplify the expression
.
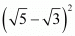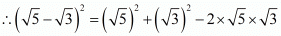
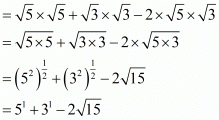
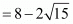
Hence the value of expression is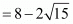
(iii) We know that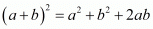
. We will use this property to simplify the expression
.
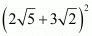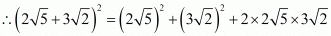
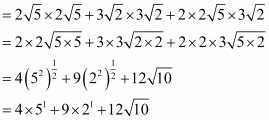
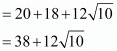
Hence the value of expression is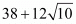
The document Rationalisation- 1 RD Sharma Solutions | Mathematics (Maths) Class 9 is a part of the Class 9 Course Mathematics (Maths) Class 9.
All you need of Class 9 at this link: Class 9
|
44 videos|412 docs|54 tests
|
FAQs on Rationalisation- 1 RD Sharma Solutions - Mathematics (Maths) Class 9
| 1. What is rationalisation and why is it important in mathematics? |  |
Ans. Rationalisation is the process of eliminating radicals or irrational numbers from the denominator of a fraction. It is important in mathematics because it helps simplify the expressions, makes them easier to work with, and often provides a more accurate representation of the numbers involved.
| 2. How do you rationalise the denominator of a fraction? | |
Ans. To rationalise the denominator of a fraction, you multiply both the numerator and denominator by a suitable expression such that the denominator no longer contains square roots or other radicals. This is done to eliminate any irrational numbers from the denominator and simplify the fraction.
| 3. Can you provide an example of rationalising the denominator? | |
Ans. Certainly! Let's consider the fraction 1 / (√2 + √3). To rationalise the denominator, we multiply both the numerator and denominator by the conjugate of the denominator, which is (√2 - √3). After simplifying, we get (√2 - √3) / (2 - √6).
| 4. Are there any limitations or restrictions when rationalising the denominator? | |
Ans. Yes, there are certain limitations or restrictions when rationalising the denominator. One restriction is that the expression inside the radical should not have any perfect square factors in the denominator. If it does, then the rationalisation process may not be applicable or may need to be modified.
| 5. Can you rationalise the denominator if it contains a cube root or higher roots? | |
Ans. No, the process of rationalising the denominator is specifically for eliminating square roots or radicals with index 2. It does not apply to cube roots or higher roots. For expressions containing cube roots or higher roots, a different approach or method needs to be used to simplify the expression.
Related Exams
About this Document
|
4.87/5 Rating |
|
Dec 23, 2024 Last updated |
Document Description: RD Sharma Solutions: Rationalisation- 1 for Class 9 2024 is part of Mathematics (Maths) Class 9 preparation.
The notes and questions for RD Sharma Solutions: Rationalisation- 1 have been prepared according to the Class 9 exam syllabus. Information about RD Sharma Solutions: Rationalisation- 1 covers topics
like RD Sharma Solutions: Exercise 3.1 - Rationalisation and RD Sharma Solutions: Rationalisation- 1 Example, for Class 9 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for RD Sharma Solutions: Rationalisation- 1.
Introduction of RD Sharma Solutions: Rationalisation- 1 in English is available as part of our Mathematics (Maths) Class 9
for Class 9 & RD Sharma Solutions: Rationalisation- 1 in Hindi for Mathematics (Maths) Class 9 course.
Download more important topics related with notes, lectures and mock test series for Class 9
Exam by signing up for free. Class 9: Rationalisation- 1 RD Sharma Solutions | Mathematics (Maths) Class 9
Description
Full syllabus notes, lecture & questions for Rationalisation- 1 RD Sharma Solutions | Mathematics (Maths) Class 9 - Class 9 | Plus excerises question with solution to help you revise complete syllabus for Mathematics (Maths) Class 9 | Best notes, free PDF download
Information about RD Sharma Solutions: Rationalisation- 1
In this doc you can find the meaning of RD Sharma Solutions: Rationalisation- 1 defined & explained in the simplest way possible. Besides explaining types of
RD Sharma Solutions: Rationalisation- 1 theory, EduRev gives you an ample number of questions to practice RD Sharma Solutions: Rationalisation- 1 tests, examples and also practice Class 9
tests
|
Explore Courses for Class 9 exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
Rationalisation- 1 RD Sharma Solutions | Mathematics (Maths) Class 9
,past year papers
,Extra Questions
,practice quizzes
,Free
,Important questions
,Rationalisation- 1 RD Sharma Solutions | Mathematics (Maths) Class 9
,Rationalisation- 1 RD Sharma Solutions | Mathematics (Maths) Class 9
,study material
,Exam
,Semester Notes
,Previous Year Questions with Solutions
,ppt
,video lectures
,Summary
,Sample Paper
,mock tests for examination
,shortcuts and tricks
,Viva Questions
,Objective type Questions
,MCQs
;
Additional Information about RD Sharma Solutions: Rationalisation- 1 for Class 9 Preparation
RD Sharma Solutions: Rationalisation- 1 Free PDF Download
The RD Sharma Solutions: Rationalisation- 1 is an invaluable resource that delves deep into the core of the Class 9 exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the RD Sharma Solutions: Rationalisation- 1 now and kickstart your journey towards success in the Class 9 exam.
Importance of RD Sharma Solutions: Rationalisation- 1
The importance of RD Sharma Solutions: Rationalisation- 1 cannot be overstated, especially for Class 9 aspirants.
This document holds the key to success in the Class 9 exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
RD Sharma Solutions: Rationalisation- 1 Notes
RD Sharma Solutions: Rationalisation- 1 Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to RD Sharma Solutions: Rationalisation- 1.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, RD Sharma Solutions: Rationalisation- 1 Notes on EduRev are your ultimate resource for success.
RD Sharma Solutions: Rationalisation- 1 Class 9 Questions
The "RD Sharma Solutions: Rationalisation- 1 Class 9 Questions" guide is a valuable resource for all aspiring students preparing for the
Class 9 exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study RD Sharma Solutions: Rationalisation- 1 on the App
Students of Class 9 can study RD Sharma Solutions: Rationalisation- 1 alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the RD Sharma Solutions: Rationalisation- 1,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of RD Sharma Solutions: Rationalisation- 1 is prepared as per the latest Class 9 syllabus.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup