Rational and Irrational Numbers - Number Systems, Class 9, Mathematics PDF Download
Rational numbers:– These are real numbers which can be expressed in the form of  , where p and q are integers and
, where p and q are integers and  .
.
Ex.  –3, 0, 10, 4.33, 7.123123123.........
–3, 0, 10, 4.33, 7.123123123.........
|

Irrational numbers:– A number is called irrational number if it cannot be written in the form  , where p & q are integers and
, where p & q are integers and  . All Non-terminating & Non-repeating decimal numbers are Irrational numbers.
. All Non-terminating & Non-repeating decimal numbers are Irrational numbers.

(vi) Real numbers :– The totality of rational numbers and irrational numbers is called the set of real numbers.
i.e. rational numbers and irrational numbers taken together are called real numbers.
Every real number is either a rational number or an irrational number.
FINDING RATIONAL NUMBERS BETWEEN TWO NUMBERS
(A) 1st method : Find a rational number between x and y then,  is a rational number lying between x and y.
is a rational number lying between x and y.
(B) 2nd method : Find n rational number between x and y (when x and y is non fraction number)
then we use formula.
(C) 3rd method : Find n rational number between x and y (when x and y is fraction Number) then we use formula,
then n rational number lying between x and y are (x + d), (x + 2d), (x + 3d) .....(x + nd)
Remark : x = First Rational Number, y = Second Rational Number, n = No. of Rational Number.
Ex. Find 3 rational numbers between 2 and 5.
Sol. Let, a = first rational number.
b = second rational number.
n = number of rational number.

Hence, three rational numbers between 2 and 5 are :  Ans.
Ans.
RATIONAL NUMBER IN DECIMAL REPRESENTATION:
Every rational number can be expressed as terminating decimal or non-terminating decimal.
(i) Terminating Decimal : The word "terminate" means "end". A decimal that ends is a terminating decimal.
OR
A terminating decimal doesn't keep going. A terminating decimal will have a finite number of digits after the decimal point.

Ex. Express  in the decimal form by long division method.
in the decimal form by long division method.
Sol. We have,

∴ 
(ii) Non terminating & Repeating (Recurring decimal) :–
A decimal in which a digit or a set of finite number of digits repeats periodically is called Non-terminating repeating (recurring) decimals.


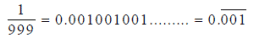
Ex. Find the decimal representation of  .
.
Sol. By long division, we have


COMPETITION WINDOW NATURE OF THE DECIMAL EXPANSION OF RATIONAL NUMBERS Theorem-1 : Let x be a rational number whose decimal expansion terminates. Then we can express x in the form Theorem-2 : Let x = Theorem-3 : Let x = Ex. (i) we observe that the prime factorisation of the denominators of these rational numbers are of the form 2m × 5n, where m,n are non-negative integers. Hence, (ii) we observe that the prime factorisation of the denominator of these rational numbers are not of the form 2m × 5n, where m,n are non-negative integers. Hence 17/6 has non-terminating and repeating decimal expansion. (iii) So, the denominator 8 of 17/8 is of the form 2m × 5n, where m,n are non-negative integers. Hence 17/8 has terminating decimal expansion. (iv) Clearly, 455 is not of the form 2m × 5n. So, the decimal expansion of 64/455 is non-terminating repeating. |
REPRESENTATION OF RATIONAL NUMBERS ON A NUMBER LINE
We have learnt how to represent integers on the number line. Draw any line. Take a point O on it. Call it 0(zero). Set of equal distances on the right as well as on the left of O.
Such a distance is known as a unit length. Clearly, the points A, B, C, D represent the integers 1, 2, 3, 4 respectively and the point A', B', C' D' represent the integers –1, –2, –3, –4 respectively

Thus, we may represent any integer by a point on the number line. Clearly, every positive integer lies to the right of O and every negative integer lies to the left of O.
Similarly we can represent rational numbers.
Ex. Represent 1/2 and -1/2 on the number line.
Sol. Draw a line. Take a point O on it. Let it represent 0. Set off unit length OA and OA' to the right as well as to the left of O.
The, A represents the integer 1 and A' represents the integer –1.

Now, divide OA into two equal parts. Let OP be the first part out of these two parts.
Then, the point P represents the rational number 1/2.
Again, divide OA' into two equal parts. Let OP' be the first part out of these 2 parts. Then the point P' represents the rational number -1/2.
FAQs on Rational and Irrational Numbers - Number Systems, Class 9, Mathematics
| 1. What are rational numbers? |  |
| 2. What are irrational numbers? |  |
| 3. How can we differentiate between rational and irrational numbers? |  |
| 4. Can an irrational number be converted into a rational number? |  |
| 5. What is the significance of rational and irrational numbers in mathematics? |  |

|
Explore Courses for Class 9 exam
|

|


















