Scatter Graphs, Bivariate Data & Correlation | Mathematics for Grade 10 PDF Download
| Table of contents |

|
| Introduction |

|
| Bivariate Data |

|
| Scatterplots |

|
| Shape |

|
| Trend |

|
| Strength |

|
| What is correlation? |

|
Introduction
- Professionals often want to know how two (or more) numeric variables are related. For example, is there a relationship between the grade on the second math exam a student takes and the grade on the final exam? If there is a relationship, what is the relationship and how strong is it?
- In another example, your income may be determined by your education, your profession, your years of experience, and your ability. The amount you pay a repair person for labor is often determined by an initial amount plus an hourly fee.
- The type of data described in these examples is bivariate data — “bi” for two variables. In this chapter, you will be studying the “simple linear regression”. Note that this does not imply that these ideas are “simple” but just that we are working with one independent variable (x) and a linear relationship. This involves data that fits a line in two dimensions.
Bivariate Data
When we are looking at bivariate data we first need to decide, if possible, does changing one variable seems to lead to a change in the other. A response variable (also called y, dependent variable, predicted variable) measures or records an outcome of a study. An explanatory variable (also called x, independent variable, predictor variable) explains changes in the response variable.
When considering the relationship between two quantitative variables:
- Start with a graph (scatterplot)
- Look for an overall pattern and deviations from the pattern
- Use numerical descriptions of the data and overall pattern (correlation, coefficient of determination)
- Consider a mathematical model (regression)
Scatterplots
Before we take up the discussion of linear regression and correlation, we need to examine a way to display the relation between two variables x and y. The most common and easiest way is a scatter plot. A scatter plot shows a lot about the relationship between the variables. When you look at a scatterplot, you want to notice the overall pattern and any potential deviations from the pattern. You can determine the strength of the relationship by looking at the scatter plot and seeing how close the points are together. When looking at a scatterplot you always want to note:
- Shape
- Trend
- Strength
The following scatterplot examples illustrate these concepts.
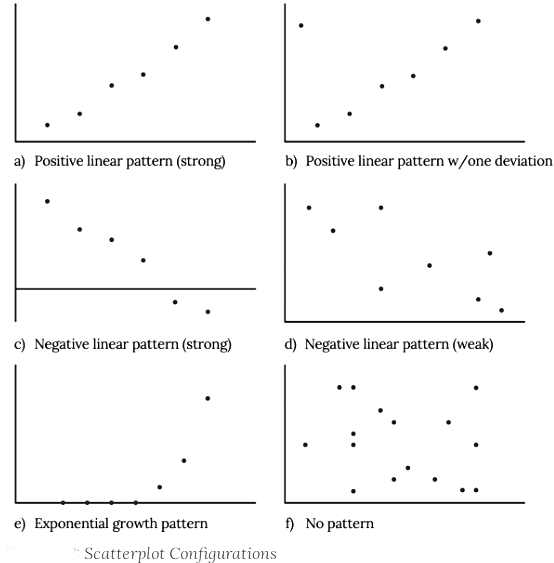
Shape
Although we may see other shapes in a scatter plot, at this point we are only interested in applying these ideas when we see a linear pattern. Linear patterns are quite common. The linear relationship is strong if the points are close to a straight line, except in the case of a horizontal line where there is no relationship. If we think that the points show a linear relationship, we would like to draw a line on the scatter plot. This line can will later be calculated through a process called linear regression. However, we only calculate a regression line if one of the variables helps to explain or predict the other variable.
Trend
If we do see a linear pattern, what sort of relationship is there? A positive trend is seen when increasing x also increases y. On the other hand a negative (inverse) trend is seen when increasing x appears to cause y to decrease. In other words:
- High values of one variable occurring with high values of the other variable or low values of one variable occurring with low values of the other variable.
- High values of one variable occurring with low values of the other variable.
Strength
At this point we can think about the strength of a relationship as how tightly do the points on a scatterplot fit the linear pattern. A stronger relationship has points clustered together closely while in a weaker one, points are more spread out. The strength of a relationship is not always apparent in a scatterplot but we will see numerical measures of this in the future.
Example 1: Does the scatter plot appear linear? Strong or weak? Positive or negative?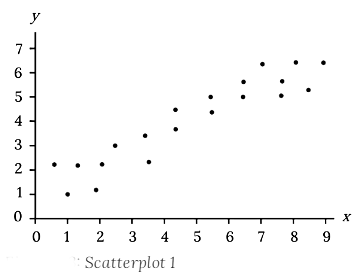
The data appear to be linear with a strong, positive correlation.
Example 2: Does the scatter plot appear linear? Strong or weak? Positive or negative?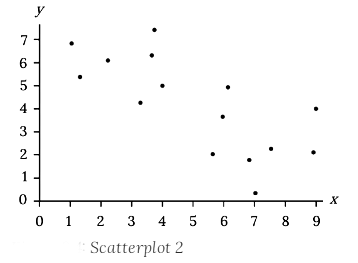
The data appear to be linear with a weak, negative correlation.
Example 3: Does the scatter plot appear linear? Strong or weak? Positive or negative?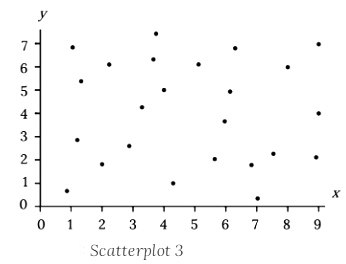
The data appear to have no correlation.
What is correlation?
Correlation is the relationship between the two variables in a bivariate data set. Such relationship can have certain tendencias that would allow us to see if the variables have an interdependency or if they are affected by the other in any way.
Correlation can be positive, negative, zero (or no correlation) and curvilinear. In the next figure you can see an example of the three most common types of data correlation:
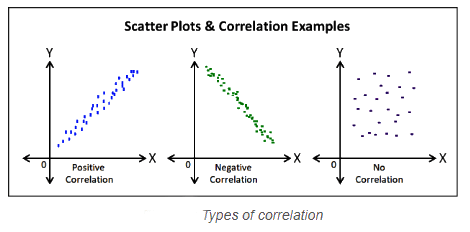
To explain the three main types of correlation of the variables in a bivariate data set we have that:
- If the value of the data points increase for the variable in the horizontal axis, just as it increases for the variable in the vertical axis, then it is said the two variables have a positive correlation.
- If the value of the data points increase for the variable in the horizontal axis, just as it decreases for the variable in the vertical axis (or vice versa), then it is said the two variables have a negative correlation.
- When no relationship or tendency is seen between the two variables, we say there is no correlation between them.
A positive and negative correlation are types of linear correlations, and the strength of them is measured by a value known as the correlation coefficient (ρ):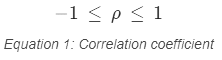
When the correlation coefficient is negative, the bivariate variables have a negative correlation (one increases while the other decreases). When the correlation coefficient is positive, the bivariate variables have a positive correlation (one increases as the other increases too).
Example: State whether each of the following bivariate data will most likely be positively correlated, negatively correlated or have no correlation:
a) Amount of gas put into a cars gas tank and the distance that car will travel
(i) Notice the first variable for this case is the amount of gas put into a cars gas tank (not the level of gas in the cars tank) versus the distance that the car will travel. For that matter, since a car needs gasoline to run, the car will cover a certain amount of kilometers per each litre of gasoline spent depending on the efficiency of the cars engine. Therefore, the more gasoline is put into the tank, the more distance the car will be able to cover with it, and so, these two variables are positively correlated.
(ii) On the other hand, if the first variable of this question was the level of gas in the cars tank, the situation would be quite different. A car spends gasoline as it runs; therefore, as the driver accumulates more distance traveled, the car is spending more and more gas until a certain point when its tank is emptied. Since one variable is increasing in value while the other one is decreasing, these two variables would be negatively correlated to each other.
b) Amount of cigarettes smoked and your life expectancy
Just as before, one variable is decreasing one other one is increasing in this case since the life expectancy of a person goes down as more cigarettes have been smoked by the person (due to the accumulation of about 7000 different chemicals in your body, some of them carcinogens).
Therefore, these two variables have a negative correlation with each other.
c) The amount of time you spend watching TV and the price of rice in China
The two variables in this case have no relation to each other whatsoever, they produce no effect on one another in any way; therefore, there is no correlation between the amount of time you watch TV and the price of rice in China.
|
138 videos|66 docs|43 tests
|



















