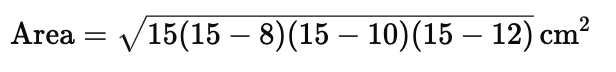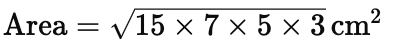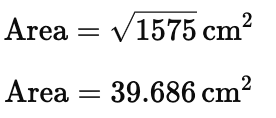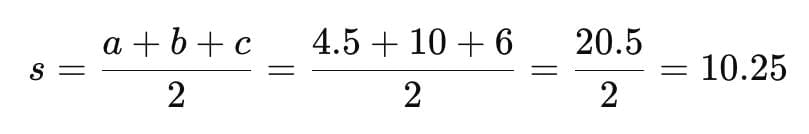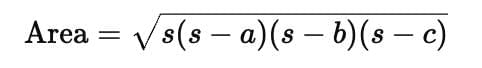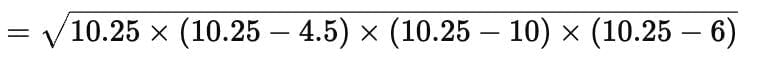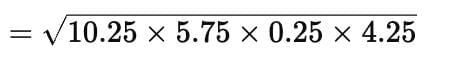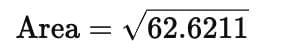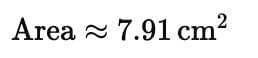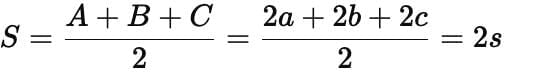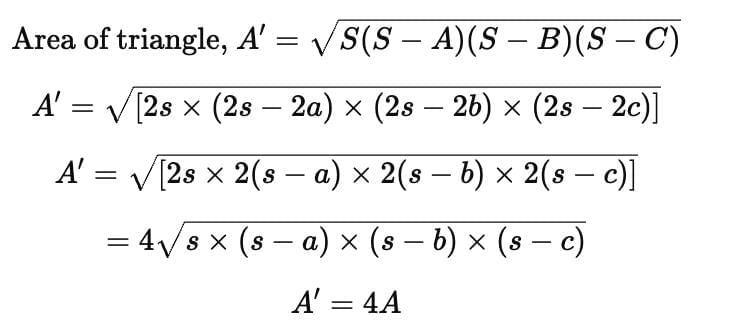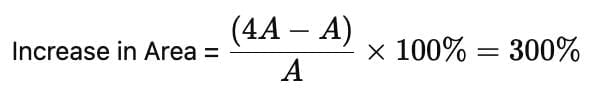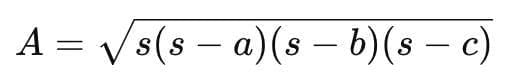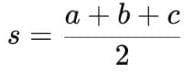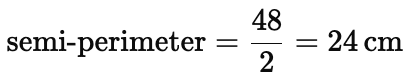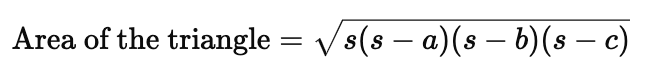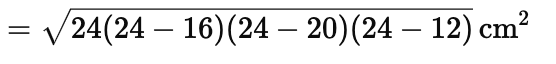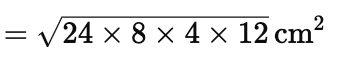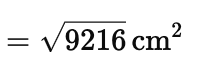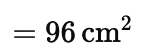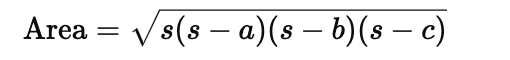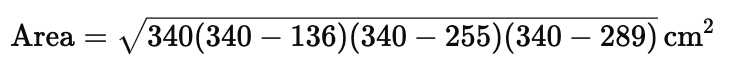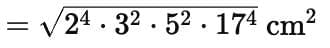Class 9 Maths Chapter 10 Question Answers - Heron’s Formula

Q1. Find the area of a triangle whose sides are 8 cm, 10 cm, and 12 cm.

Sol: Let the semi-perimeter $s$s be:Heron’s formula for the area of the triangle:Substituting the values:

Q2. Find the area of a triangle whose sides are 4.5 cm and 10 cm and perimeter 20.5 cm.

Sol:Given,

Side a = 4.5 cm

Side b = 10 cm

Perimeter of triangle = 20.5 cm

a+b+c = 20.5

4.5+10+c = 20.5

14.5+c = 20.5

c = 20.5 – 14.5

c = 6 cm

Semiperimeter, s = (4.5+10+6)/2 = 20.5/2 = 10.25 cm

Using Heron’s formula,

Area of the triangle = √[s (s-a) (s-b) (s-c)]

Area = √[10.25(10.25-4.5) (10.25-10) (10.25-6)]

Area = 7.91 cm²

Q3. If every side of a triangle is doubled, by what percentage is the area of the triangle increased?

Sol:Let a, b and c be the sides of a triangle.

Semiperimeter, s = (a+b+c)/2

Now, if each of the side is doubled, then the new sides of a triangle are:

A = 2a, B = 2b, C = 2c

Semiperimeter, S = (A+B+C)/2 = (2a + 2b + 2c)/2 = 2s

By Heron’s formula,

Area of triangle, A’ = √[S (S-A) (S-B) (S-C)]

A’ = √[2s(2s-2a)(2s-2b)(2s-2c)]

A’ = √[2s2(s-a)2(s-b)2(s-c)]

= 4√s(s-a)(s-b)(s-c)

A’ = 4A

Increase in Area = (4A – A)/A x 100% = 300%

Hence, the area is increased by 300%, if the sides of the triangle are doubled.

Q4.A triangular field has sides 150 m, 120 m, and 100 m. A gardener has to put a fence all around it and also plant grass inside. How much area does he need to plant grass?

Sol:Given,

Sides of triangular park are 120m, 80m and 50m.

Semiperimeter, s = (150+120+100)/2 = 185 m
Using Heron’s formula, we have;

Area of triangle = √(s(s-a)(s-b)(s-c))
The area that needs to be planted with grass is 2400m².

Q5. Find the area of a triangle whose two sides are 16 cm and 20 cm, and the perimeter is 48 cm.

Sol:Assume that the third side of the triangle is $x$x.

Now, the three sides of the triangle are 16 cm, 20 cm, and $x$x cm.

It is given that the perimeter of the triangle is 48 cm:

x=48−(16+20) =48−36 =12cm

Q6. The sides of a triangle are in the ratio 8: 15: 17 and its perimeter is 680 cm. Find its area.

Sol: Given:The ratio of the sides of the triangle is given as 8: 15: 17.
Let the common ratio between the sides of the triangle be "x".

Thus, the sides are $8x,\; 15x,$8x,15x, and 17x.

It is also given that the perimeter of the triangle is 680 cm:

$\hspace{0.17em}cm8x\; +\; 15x\; +\; 17x\; =\; 680\; \backslash ,\; \backslash text\{cm\}$8x+15x+17x=680cm
40x=680cm
$x\; =\; 17$x=17
Now, the sides of the triangle are:
$\hspace{0.17em}cm8\; \backslash times\; 17\; =\; 136\; \backslash ,\; \backslash text\{cm\},\; \backslash quad\; 15\; \backslash times\; 17\; =\; 255\; \backslash ,\; \backslash text\{cm\},\; \backslash quad\; 17\; \backslash times\; 17\; =\; 289\; \backslash ,\; \backslash text\{cm\}$8×17=136cm, 15×17=255cm, 17×17=289cm
the semi-perimeter of the triangle $s=\frac{680}{2}=340\hspace{0.17em}cms\; =\; \backslash frac\{680\}\{2\}\; =\; 340\; \backslash ,\; \backslash text\{cm\}$
Using Heron's formula:
Area = 4760cm²

Q7. The lengths of sides of a triangle are in the ratio 3 : 4 : 5 and its perimeter is 120 cm, find its area. 

The sides are in the ratio of 3 : 4 : 5.
Let the sides be 3x, 4x and 5x.
∴ Perimeter = 3x + 4x + 5x = 12x
Now 12x = 120                [Perimeter = 120 cm]
⇒   x =(120/12) = 10
∴ Lengths are: a = 3x = 3 x 10 = 30 cm
b = 4x = 4 x 10 = 40 cm
c = 5x = 5 x 10 = 50 cm
Now, semi-perimeter (s) = (120/12)
cm = 60 cm
∵ (s – a) = 60 – 30 = 30 cm
(s – b) = 60 – 40 = 20 cm
(s – c) = 60 – 50 = 10 cm

Using Heron’s formula, we have  

Area of the triangle =  

= 2 x 30 x 10 cm² = 600 cm² Thus, the required area of the triangle = 600 cm².

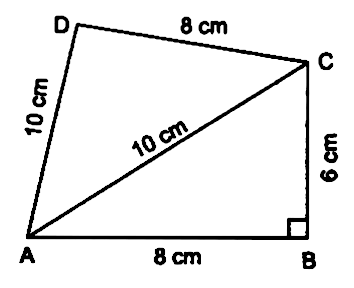
Q8. Find the area of a quadrilateral ABCD in which AB = 8 cm, BC = 6 cm, CD = 8 cm, DA = 10 cm and AC = 10 cm.

In ΔABC, ∠B = 90°

∴ area of right (rt ΔABC) = (1/2) x 8 x 6 cm² = 24 cm²
In ΔACD,
a = AC = 10 cm b = AD = 10 cm c = CD = 8 cm

∴Area of ΔACD 

= 2 x 4√21 = 8√21 cm²

= 8 x 4,58 cm² = 36.64 cm²

Now, area of quadrilateral ABCD = ar (ΔABC) + ar (ΔACD)
= 24 cm² + 8√21 cm² 
= 24 cm² + 36.64 cm²
= 60.64 cm²

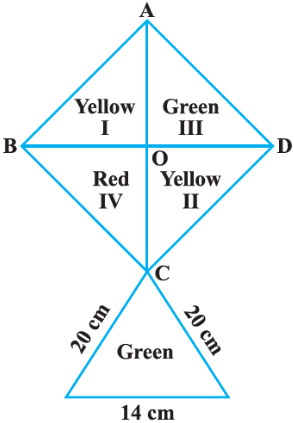
Q9. How much paper of each shade is needed to make a kite given in the figure, in which ABCD is a square of diagonal 44 cm.

∵ The diagonals of a square bisect each other at right angles

∴ OB = OD = OA = OC = (44/2) = 22 cm
Now, ar rt (Δ –I) = (1/2)× OB × OA
= (1/2) × 22 × 22 cm² = 242 cm²
Similarly ar rt (Δ –II) = arrt(Δ–III) = ar rt (Δ –IV) = 242 cm²

∵ Sides of ΔCEF are 20 cm, 20 cm and 14 cm

⇒ Area of ΔCEF 

Now, area of yellow paper = ar (Δ – I) + ar (Δ – II)
= 242 cm² + 242 cm² = 484 cm²
Area of red paper = ar (Δ – IV) = 242 cm²
Area of green paper = ar (Δ – III) + ar ΔCEF
= 242 cm² + 131.14 cm²
= 373.14 cm²