Class 9 Maths Chapter 6 Question Answers - Triangles
Q1: ABCD is a quadrilateral in which AD = BC and ∠DAB = ∠CBA. Prove that
(i) ΔABD ≅ ΔBAC
(ii) BD = AC
(iii) ∠ABD = ∠BAC.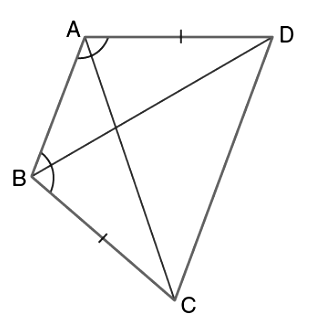 Sol:
Sol:
As per given in the question,
∠DAB = ∠CBA and AD = BC.
(i) ΔABD and ΔBAC are similar by SAS congruency as
AB = BA (common arm)
∠DAB = ∠CBA and AD = BC (given)
So, triangles ABD and BAC are similar
i.e. ΔABD ≅ ΔBAC. (Hence proved).
(ii) As it is already proved,
ΔABD ≅ ΔBAC
So,
BD = AC (by CPCT)
(iii) Since ΔABD ≅ ΔBAC
So, the angles,
∠ABD = ∠BAC (by CPCT).
Q2: Line l is the bisector of an angle ∠A and B is any point on l. BP and BQ are perpendiculars from B to the arms of ∠A. Show that:
(i) ΔAPB ≅ ΔAQB
(ii) BP = BQ or B is equidistant from the arms of ∠A.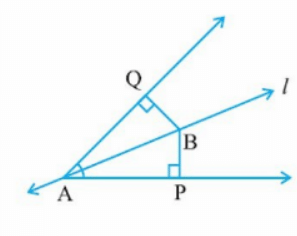 Sol:
Sol:
It is given that the line “l” is the bisector of angle ∠A and the line segments BP and BQ are perpendiculars drawn from l.
(i) ΔAPB and ΔAQB are similar by AAS congruency because;
∠P = ∠Q (both are right angles)
AB = AB (common arm)
∠BAP = ∠BAQ (As line l is the bisector of angle A)
So, ΔAPB ≅ ΔAQB.
(ii) By the rule of CPCT, BP = BQ. So, we can say point B is equidistant from the arms of ∠A.
Q3: In right triangle ABC, right-angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see the figure). Show that:
(i) ΔAMC ≅ ΔBMD
(ii) ∠DBC is a right angle.
(iii) ΔDBC ≅ ΔACB
(iv) CM = 1/2 AB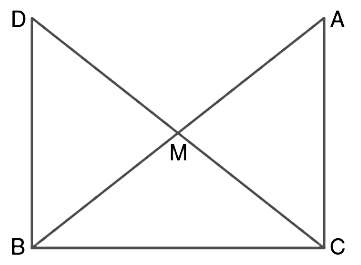 Sol:
Sol:
It is given that M is the mid-point of the line segment AB, ∠C = 90°, and DM = CM
(i) Consider the triangles ΔAMC and ΔBMD:
AM = BM (Since M is the mid-point)
CM = DM (Given)
∠CMA = ∠DMB (Vertically opposite angles)
So, by SAS congruency criterion, ΔAMC ≅ ΔBMD.
(ii) ∠ACM = ∠BDM (by CPCT)
∴ AC ∥ BD as alternate interior angles are equal.
Now, ∠ACB + ∠DBC = 180° (Since they are co-interiors angles)
⇒ 90° + ∠B = 180°
∴ ∠DBC = 90°
(iii) In ΔDBC and ΔACB,
BC = CB (Common side)
∠ACB = ∠DBC (Both are right angles)
DB = AC (by CPCT)
So, ΔDBC ≅ ΔACB by SAS congruency.
(iv) DC = AB (Since ΔDBC ≅ ΔACB)
⇒ DM = CM = AM = BM (Since M the is mid-point)
So, DM + CM = BM + AM
Hence, CM + CM = AB
⇒ CM = (½) AB
Q4: ΔABC is an isosceles triangle in which AB = AC. Side BA is produced to D such that AD = AB. Show that ∠BCD is a right angle.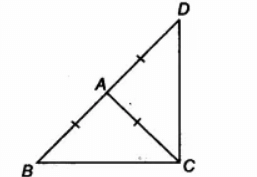 Sol:
Sol:
Given, AB = AC and AD = AB
To prove: ∠BCD is a right angle.
Proof:
Consider ΔABC,
AB = AC (Given)
Also, ∠ACB = ∠ABC (Angles opposite to equal sides)
Now, consider ΔACD,
AD = AC
Also, ∠ADC = ∠ACD (Angles opposite to equal sides)
Now,
In ΔABC,
∠CAB + ∠ACB + ∠ABC = 180°
So, ∠CAB + 2∠ACB = 180°
⇒ ∠CAB = 180° – 2∠ACB — (i)
Similarly in ΔADC,
∠CAD = 180° – 2∠ACD — (ii)
Also,
∠CAB + ∠CAD = 180° (BD is a straight line.)
Adding (i) and (ii) we get,
∠CAB + ∠CAD = 180° – 2∠ACB + 180° – 2∠ACD
⇒ 180° = 360° – 2∠ACB – 2∠ACD
⇒ 2(∠ACB + ∠ACD) = 180°
⇒ ∠BCD = 90°
Q5: Two sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of ΔPQR (see the figure). Show that:
(i) ΔABM ≅ ΔPQN
(ii) ΔABC ≅ ΔPQR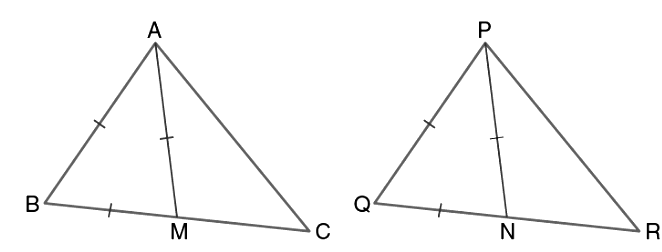 Sol:
Sol:
Given;
AB = PQ,
BC = QR and
AM = PN
(i) 1/2 BC = BM and 1/2QR = QN (Since AM and PN are medians)
Also, BC = QR
So, 1/2 BC = 1/2QR
⇒ BM = QN
In ΔABM and ΔPQN,
AM = PN and AB = PQ (Given)
BM = QN (Already proved)
∴ ΔABM ≅ ΔPQN by SSS congruency.
(ii) In ΔABC and ΔPQR,
AB = PQ and BC = QR (Given)
∠ABC = ∠PQR (by CPCT)
So, ΔABC ≅ ΔPQR by SAS congruency.
Q6: AD and BC are equal perpendiculars to a line segment AB. Show that CD bisects AB.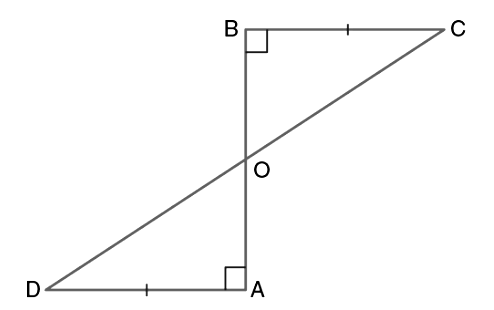 Sol:
Sol:
Given, AD and BC are two equal perpendiculars to AB.
To prove: CD is the bisector of AB
Proof:
Triangles ΔAOD and ΔBOC are similar by AAS congruency
Since:
(i) ∠A = ∠B (perpendicular angles)
(ii) AD = BC (given)
(iii) ∠AOD = ∠BOC (vertically opposite angles)
∴ ΔAOD ≅ ΔBOC.
So, AO = OB ( by CPCT).
Thus, CD bisects AB (Hence proved).
Q7: AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that ∠BAD = ∠ABE and ∠EPA = ∠DPB. Show that
(i) ΔDAP ≅ ΔEBP
(ii) AD = BE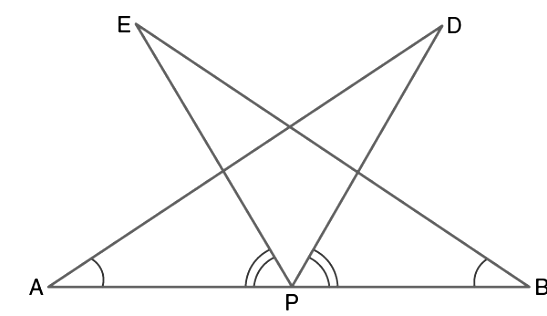
Sol:
Given, P is the mid-point of line segment AB.
Also, ∠BAD = ∠ABE and ∠EPA = ∠DPB
(i) Given, ∠EPA = ∠DPB
Now, add ∠DPE on both sides,
∠EPA + ∠DPE = ∠DPB + ∠DPE
This implies that angles DPA and EPB are equal
i.e. ∠DPA = ∠EPB
Now, consider the triangles DAP and EBP.
∠DPA = ∠EPB
AP = BP (Since P is the mid-point of the line segment AB)
∠BAD = ∠ABE (given)
So, by ASA congruency criterion,
ΔDAP ≅ ΔEBP.
(ii) By the rule of CPCT,
AD = BE
Q8: ABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively. Show that these altitudes are equal.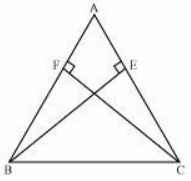 Sol:
Sol:
Given:
(i) BE and CF are altitudes.
(ii) AC = AB
To prove:
BE = CF
Proof:
Triangles ΔAEB and ΔAFC are similar by AAS congruency, since;
∠A = ∠A (common arm)
∠AEB = ∠AFC (both are right angles)
AB = AC (Given)
∴ ΔAEB ≅ ΔAFC
and BE = CF (by CPCT).
Q9: ΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see the figure). If AD is extended to intersect BC at P, show that
(i) ΔABD ≅ ΔACD
(ii) ΔABP ≅ ΔACP
(iii) AP bisects ∠A as well as ∠D.
(iv) AP is the perpendicular bisector of BC.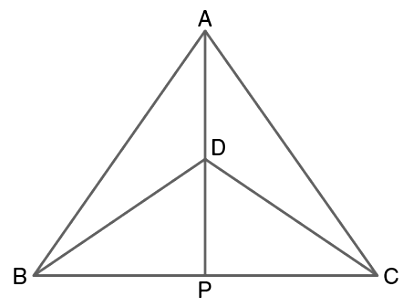 Sol:
Sol:
In the above question, it is given that ΔABC and ΔDBC are two isosceles triangles.
(i) ΔABD and ΔACD are similar by SSS congruency because:
AD = AD (It is the common arm)
AB = AC (Since ΔABC is isosceles)
BD = CD (Since ΔDBC is isosceles)
∴ ΔABD ≅ ΔACD.
(ii) ΔABP and ΔACP are similar as:
AP = AP (common side)
∠PAB = ∠PAC ( by CPCT since ΔABD ≅ ΔACD)
AB = AC (Since ΔABC is isosceles)
So, ΔABP ≅ ΔACP by SAS congruency.
(iii) ∠PAB = ∠PAC by CPCT as ΔABD ≅ ΔACD.
AP bisects ∠A. ………… (1)
Also, ΔBPD and ΔCPD are similar by SSS congruency as
PD = PD (It is the common side)
BD = CD (Since ΔDBC is isosceles.)
BP = CP (by CPCT as ΔABP ≅ ΔACP)
So, ΔBPD ≅ ΔCPD.
Thus, ∠BDP = ∠CDP by CPCT. ……………. (2)
Now by comparing equation (1) and (2) it can be said that AP bisects ∠A as well as ∠D.
(iv) ∠BPD = ∠CPD (by CPCT as ΔBPD ≅ ΔCPD)
and BP = CP — (1)
also,
∠BPD + ∠CPD = 180° (Since BC is a straight line.)
⇒ 2∠BPD = 180°
⇒ ∠BPD = 90° —(2)
Now, from equations (1) and (2), it can be said that
AP is the perpendicular bisector of BC.
Q10: In the Figure, PR > PQ and PS bisect ∠QPR. Prove that ∠PSR > ∠PSQ.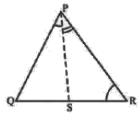 Sol:
Sol:
Given, PR > PQ and PS bisects ∠QPR
To prove: ∠PSR > ∠PSQ
Proof:
∠QPS = ∠RPS — (1) (PS bisects ∠QPR)
∠PQR > ∠PRQ — (2) (Since PR > PQ as angle opposite to the larger side is always larger)
∠PSR = ∠PQR + ∠QPS — (3) (Since the exterior angle of a triangle equals the sum of opposite interior angles)
∠PSQ = ∠PRQ + ∠RPS — (4) (As the exterior angle of a triangle equals to the sum of opposite interior angles)
By adding (1) and (2)
∠PQR + ∠QPS > ∠PRQ + ∠RPS
Now, from (1), (2), (3) and (4), we get
∠PSR > ∠PSQ.
|
40 videos|471 docs|57 tests
|
FAQs on Class 9 Maths Chapter 6 Question Answers - Triangles
| 1. What are the different types of triangles based on their sides? |  |
| 2. How do you calculate the area of a triangle? |  |
| 3. What is the Pythagorean theorem and how is it related to triangles? |  |
| 4. How can you determine if a triangle is a right triangle? |  |
| 5. What are the angle classifications of triangles? |  |

















