Points to Remember: Linear Equations in One Variable | Mathematics (Maths) Class 8 PDF Download
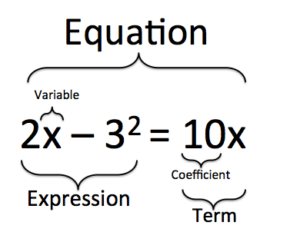
Algebraic Expression
Any expression containing constants, variables, and the operations like addition, subtraction, etc. is called an algebraic expression.
Examples: 5x, 2x - 3, x2 + 1 etc.

- A variable is an unknown number, and generally, it is represented by a letter like x, y, n, etc.
- Any number without any variable is called Constant.
- A number followed by a variable is called the Coefficient of that variable.
- A term is any number or variable separated by operators.
Equation
Any mathematical expression equating one algebraic expression to another is called an equation.
Examples: 5x = 25, 2x - 3 = 9, x2 + 1 = 0 etc.
Linear Expression
A linear expression is an expression whose highest power of the variable is one only.
Example
2x + 5, 3y etc.
The expressions like x2 + 1, z2 + 2z + 3 are not the linear expressions as their highest power of the variable is greater than 1.
Linear Equations
The equation of a straight line is the linear equation. It could be in one variable or two variables.
Linear Equation in One Variable
If there is only one variable in the equation, then it is called a linear equation in one variable.
The general form is
ax + b = c, where a, b and c are real numbers and a ≠ 0.
Example
x + 5 = 10
y – 3 = 19
- These are called linear equations in one variable because the highest degree of the variable is one and there is only one variable.
- For any linear equation, there will be a presence of an equal to sign in the equation.
- The quantity on the left of the equality sign is called the Left Hand Side (LHS) of the equation and that on the right side is called the Right Hand Side (RHS) of the equation.
Examples: Consider a linear equation x - 3 = 5.
Here, (x - 3) is the LHS of the equation and ‘5’ is the RHS of the equation.
- The values of the expression on the LHS and RHS are equal and become true only for certain values of the variable. These certain values are called the solutions of the equation.
Examples: In equation x - 3 =5, the equation will be true for x = 8 i.e. for x = 8 LHS will be equal to RHS. - Transposing mean taking a term from one side of an equation to the other side with its sign changed.
- Some equations may not be linear in the beginning, but they can be brought to be linear by using the usual methods.
- Equations help us to solve different problems on numbers, ages, perimeters, combination of coins or currency notes, etc.
Finding the Solution of Linear Equations having Variable on Both the Sides
To find the solution of such equations, bring all quantities with variables on one side of the equation and all the other quantities on the other side. Now, solve the equation to obtain the solution.
Example 1: Solve 2x - 5 = x + 3
Solution: Firstly, we will transpose x from RHS to LHS
2x - 5 - x = 3
Now, we will transpose the integer - 5 from LHS to RHS
2x - x = 3 + 5
On solving,
x = 8
Example 2: Solve 2x + 53 = 263 - x and verify the result.
Solution: Transposing x to the LHS and 53 to the RHS, we get:
2x + x = 263 - 53
So, 3x = 213 = 7
Dividing both sides by 3, we get:
3x3 = 73
⇒ x = 73
Verification:
Substituting the value of x into the LHS and RHS:
LHS = 2x + 53 = 2 × 73 + 53 = 143 + 53 = 193
RHS = 263 - x = 263 - 73 = 193
Since LHS = RHS, the result is correct.
Reducing Complex Linear Equations to Simpler Form
Example 1: x + 7 - 8x3 = 176 - 5x2
Solution: Firstly, we will transpose 5x2 on LHS:
x + 7 - 8x3 + 5x2 = 176
Now, let us transpose 7 from LHS to RHS:
x - 8x3 + 5x2 = 176 - 7
On solving both sides, we get:
5x6 = -256
Thus, x = -5 is the required solution.
Example 2: The denominator of a rational number is greater than its numerator by 8. If the numerator is increased by 17 and the denominator is decreased by 1, the number obtained is 32 . Find the rational number.
Solution: Suppose the numerator of the rational number is x. Hence, its denominator will be x + 8.
The rational number will be xx + 8.
Given, if the numerator is increased by 17 and the denominator is decreased by 1, the number obtained is 32, thus we get:
x + 17 x + 8 -1 = 32
Or, x + 17x + 7 = 32
Multiplying both sides by 2(x + 7), we get:
2(x + 17) = 3(x + 7)
⇒ 2x + 34 = 3x + 21
Transposing 3x on LHS and 34 on RHS, we get:
2x - 3x = 21 - 34
⇒ -x = -13
Dividing by -1 on both sides,
x = 13
x + 8 = 13 + 8 = 21
Thus, the rational number will be 1321.
|
79 videos|408 docs|31 tests
|
FAQs on Points to Remember: Linear Equations in One Variable - Mathematics (Maths) Class 8
| 1. What is a linear equation in one variable? |  |
| 2. How do you solve a linear equation in one variable? |  |
| 3. What are some common examples of linear equations in one variable? |  |
| 4. What is the importance of linear equations in real life? |  |
| 5. Can a linear equation in one variable have more than one solution? |  |

|
Explore Courses for Class 8 exam
|

|


















