Points to Remember: Rational Numbers | Mathematics (Maths) Class 8 PDF Download
- Natural Numbers: The counting numbers 1, 2, 3, 4, 5, … are called ‘natural numbers’. The smallest natural number is 1, but there is no last (or the greatest) natural number. There are infinitely many natural numbers.
- Whole Numbers: The number ‘0’ together with the natural numbers give us the numbers 0, 1, 2, 3, 4, ... which are called ‘whole numbers’. Every natural number is a whole number but every whole number is not a natural number (because ‘0’ is not a natural number).
- Integers: The whole numbers together with the negatives of counting numbers are known as ‘integers’. Thus, …, –4, –3, –2, –1, 0, 1, 2, 3, 4, … are integers.
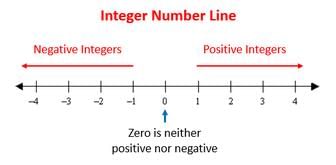
Key Points:
I. The positive integers are 1, 2, 3, 4, …, i.e. the positive integers are the same as natural numbers.
II. The negative integers are …, –4, –3, –2, –1.
III. The greatest negative integer is –1, and the smallest positive integer is 1.
IV. ‘0’ is neither positive nor negative. It is greater than every negative integer and smaller than every positive integer.
- Rational Numbers: The numbers which can be expressed as the ratio of integers are known as rational numbers. The examples of rational numbers will be 1/4, 2/7, - 3/10, 34/7, etc. On solving equations like 3x + 5 = 0, we get the solution as x = -5/3. The solution -5/3 is neither a natural number or whole number or integer.
This leads us to the collection of Rational Numbers. These are the numbers that can be expressed in x/y form; where y ≠ 0. - Properties of Rational Numbers: Rational numbers, expressed as fractions, possess key properties. They exhibit proprties such as closure, commutative and associative under basic arithmetic operations.
Let us discuss these properties of Rational numbers.
(i) Closure Property: When any operation is performed between two or more rational numbers and their result is also a rational number then we say that the rational numbers follow the closure property for that operation.
Operation | Numbers | Remark |
Addition | (a) 5/3 + 3/2 = 19/6 (Rational No); | We can observe that addition of two rational numbers x and y, i.e. x + y is always a rational number. |
Subtraction | (a) 5/3 - 3/2 = 1/6 (Rational No); | We can observe that subtraction of two rational numbers x and y, i.e. x - y is always a rational number. |
Multiplication | (a) 5/ 3 × 3/2 = 5/2 (Rational No); (b) -2/7 × 14/5 = -4/5 (Rational No); | We can observe that multiplication of two rational numbers x and y, i.e. x × y is always a rational number. |
Division | (a) 5/3 ÷ 3/2 = 10/9 (Rational No); ….. | We can observe that division of two rational numbers x and y, i.e. x ÷ y is not always a rational number. |
(ii) Commutative Property: When two rational numbers are swapped between one operator and still their result does not change then we say that the rational numbers follow the commutative property for that operation.
Operation | Numbers | Remark |
Addition | (a) 5/3 + 3/2 = 19/6; | We can observe that addition of two rational numbers x and y when inter changed yields the same answer, i.e. x + y = y + x. |
Subtraction | (a) 5/3 - 3/2 = 1/6; | We can observe that subtraction of two rational numbers x and y when inter changed does not yield the same answer, i.e. x - y ≠ y - x. |
Multiplication | (a) 5/3 × 3/2 = 5/2; | We can observe that multiplication of two rational numbers x and y when inter changed yields the same answer, i.e. x × y = y × x. |
Division | (a) 5/3 ÷ 3/2 = 10/9; | We can observe that division of two rational numbers x and y when inter changed does not yield the same answer, i.e. x ÷ y ≠ y ÷ x. |
(iii) Associative Property: When rational numbers are rearranged among two or more same operations and still their result does not change then we say that the rational numbers follow the associative property for that operation.
Operation | Numbers | Remark |
Addition | (a) 5/3 + (3/2 + 1/3) = 7/2; | We can observe that addition of rational numbers x, y, and z in any order yields the same answer, i.e. x + (y + z) = (x + y) + z. |
Subtraction | (a) 5/3 – (3/2 – 1/3) = 3/2; | We can observe that subtraction of rational numbers x, y, and z in any order does not yields the same answer, i.e. x - (y - z) ≠ (x - y) - z. |
Multiplication | (a) 5/ 3 × (3/2 × 2/3) = 5/3; | We can observe that multiplication of rational numbers x, y, and z in any order yields the same answer, i.e. x × (y × z) = (x × y) × z. |
Division | (a) 5/3 ÷ (3/2 ÷ 1/4) = 5/18; | We can observe that division of rational numbers x, y, and z in any order does not yields the same answer, i.e. x ÷ (y ÷ z) ≠ (x ÷ y) ÷ z. |
The Role of Zero(0) and One(1)
(i) The zero is called the identity element for the addition of rational numbers, i.e. the sum of 0 and a rational number is the rational number itself.
Note: Zero is also the additive identity for integers and whole numbers as well.
(ii) 1 is the multiplicative identity for rational numbers, i.e. the product of 1 and a rational number is the rational number itself.
Note: 1 is the multiplicative identity for integers and whole numbers also.
- Distributivity of Multiplication over Addition and Subtraction: If the rational numbers a, b, and c obey the property of a × (b + c) = ab + ac, then it is said to follow the distributive property of multiplication over addition.
Example:
1/3 × (2/3 + 1/4) = 1/3 × 11/12 = 11/36…………………..(i)
(1/3 × 2/3) + (1/3 × 1/4) = 2/9 + 1/12 = 11/36…………(ii)
Here, answer for both the equations (i) and (ii) are same.
Hence, rational numbers follow the distributive property of multiplication over addition.
Recap:
A number of the form p/q , where ‘p’ and ‘q’ are any integers and q ≠ 0 is called a rational number.
- Rational numbers are closed under the operations of addition, subtraction and multiplication.
- Rational numbers are commutative under addition and multiplication.
- Rational numbers are associative under addition and multiplication.
- The rational number ‘0’ is the additive identity for rational numbers i.e. x/y + 0 = x/y.
- The rational number 1 is the multiplicative identity for rational numbers i.e. x/y × 1 = x/y.
- For all rational numbers, a, b and c, we have:
a(b + c) = ab + ac
a(b – c) = ab – ac
|
79 videos|408 docs|31 tests
|
FAQs on Points to Remember: Rational Numbers - Mathematics (Maths) Class 8
| 1. What are natural numbers? |  |
| 2. What are whole numbers? |  |
| 3. What are integers? |  |
| 4. What are rational numbers? |  |
| 5. How are rational numbers different from other types of numbers? |  |

|
Explore Courses for Class 8 exam
|

|


















