Triangles Class 9 Notes Maths Chapter 6
| Table of contents |

|
| What is a Triangle? |

|
| Types of Triangle |

|
| Congruence |

|
| Congruence of Triangles |

|
| Some Properties of a Triangle |

|
| Inequalities in a Triangle |

|
What is a Triangle?
A closed figure with three sides is called a Triangle. It has three vertex, sides and Angles.
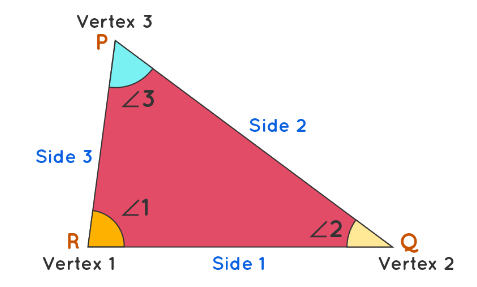
Types of Triangle
1. There are three types of triangles on the basis of the length of the sides.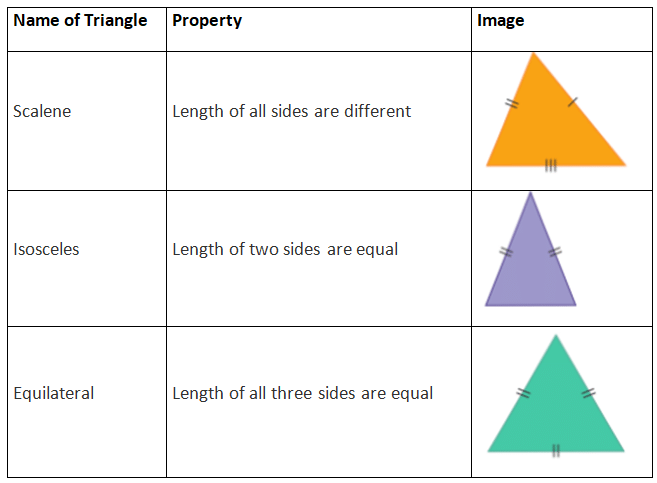
2. There are three types of triangles on the basis of angles.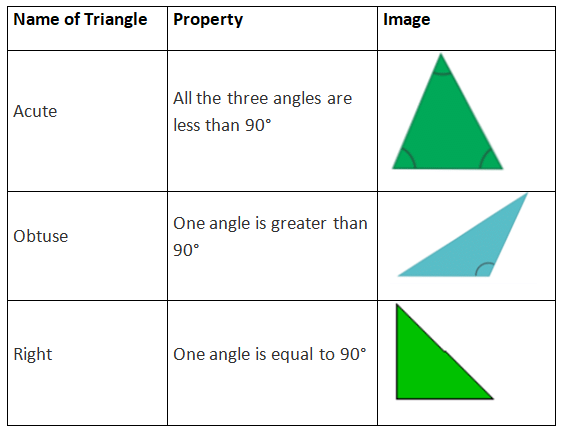
Congruence
If the shape and size of two figures are same then these are called Congruent.
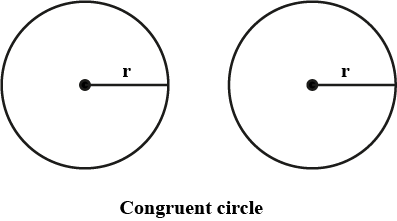
1. Two circles are congruent if their radii are same.
2. Two squares are congruent if their sides are equal.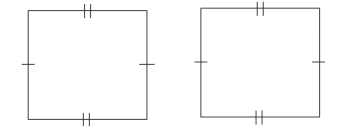
Congruence of Triangles
A triangle will be congruent if its corresponding sides and angles are equal.
The symbol of congruent is “≅”.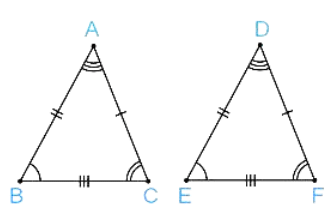
AB = DE, BC = EF, AC = DF
m∠A = m∠D, m∠B = m∠E, m∠C = m∠F
Here ∆ABC ≅ ∆DEF
Criteria for Congruence of Triangles
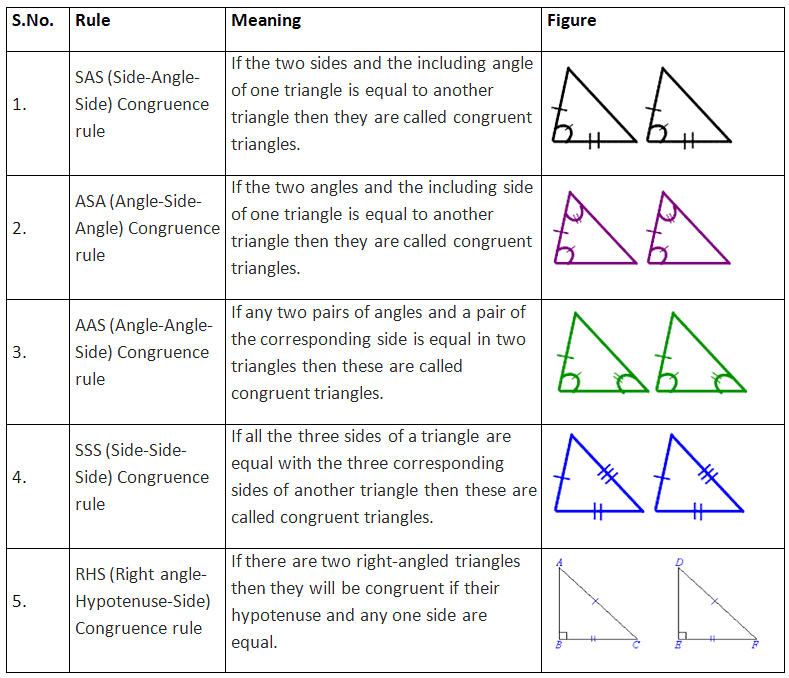
Remark
- SSA and ASS do not show the congruency of triangles.
- AAA is also not the right condition to prove that the triangles are congruent.
Example: Find the ∠P, ∠R, ∠N and ∠M if ∆LMN ≅ ∆PQR.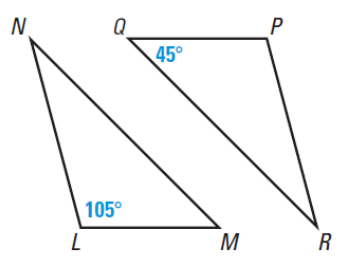
Sol:
If ∆ LMN ≅ ∆PQR, then
∠L=∠P
∠M =∠Q
∠N =∠R
So,
∠L=∠P = 105°
∠M =∠Q = 45°
∠M + ∠N + ∠L = 180° (Sum of three angles of a triangle is 180°)
45° + 105° + ∠N = 180°
∠N = 180°- 45° + 105°
∠N = 30°
∠N = ∠R = 30°
Some Properties of a Triangle
If a triangle has two equal sides then it is called an Isosceles Triangle.
1. Two angles opposite to the two equal sides of an isosceles triangle are also equal.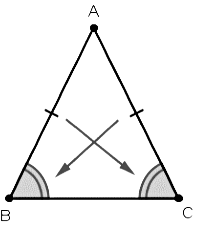
2. Two sides opposite to the equal angles of the isosceles triangle are also equal. This is the converse of the above theorem.
Inequalities in a Triangle
Theorem 1: In a given triangle if two sides are unequal then the angle opposite to the longer side will be larger.
a > b, if and only if ∠A > ∠B
Longer sides correspond to larger angles.
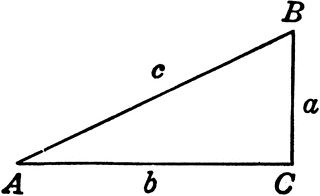
Theorem 2: In the given triangle, the side opposite to the larger angle will always be longer. This is the converse of above theorem.
Theorem 3: The sum of any two sides of a triangle will always be greater than the third side.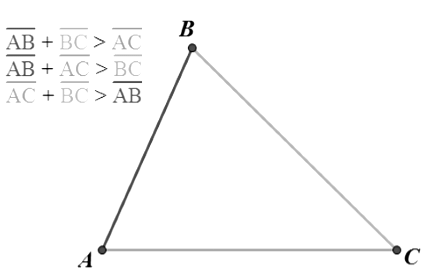
Example: Show whether the inequality theorem is applicable to this triangle or not?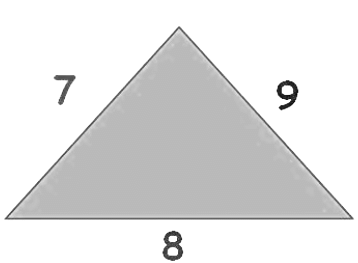
Sol:
The three sides are given as 7, 8 and 9.
According to inequality theorem, the sum of any two sides of a triangle will always be greater than the third side.
Let’s check it
7 + 8 > 9
8 + 9 > 7
9 + 7 > 8
This shows that this theorem is applicable to all the triangles irrespective of the type of triangle.
|
40 videos|471 docs|57 tests
|















