This EduRev document offers 10 Multiple Choice Questions (MCQs) from the topic Simple & Compound Interest (Level - 3). These questions are of Level - 3 difficulty and will assist you in the preparation of CAT & other MBA exams. You can practice/attempt these CAT Multiple Choice Questions (MCQs) and check the explanations for a better understanding of the topic.
Question for Practice Questions Level 3: Simple & Compound Interest - 1
Try yourself:Mr. Khanna pays 10% pa interest on an amount borrowed and lends this amount at 12% pa. If at the end of the year, he earns Rs. 2.4 crore as simple interest in the transaction, then what is the sum transacted?
Explanation
Net rate = 2%
SI at 2% of money transacted = 
x = 120 crore
Report a problem
Question for Practice Questions Level 3: Simple & Compound Interest - 1
Try yourself:Amit invested two equal sums of Rs. 5000 for 2 years at 10%, one at compound interest payable yearly and the other at compound interest payable half-yearly. Find the difference between amounts that he will get at the end of 2 years.
Explanation
Report a problem
Question for Practice Questions Level 3: Simple & Compound Interest - 1
Try yourself:A certain sum of money lent at simple interest amounts to Rs. 1,300 in 4 years and Rs. 1,525 in 7 years. Find the sum.
Explanation
Let principal = P
Amount after 4 years = Rs. 1300
Amount after 7 years = Rs. 1525
Simple interest for 3 years = 1525 - 1300 = Rs. 225
Simple interest for 1 year = 225/3 = Rs. 75
Simple interest for 4 years = 4 × 75 = Rs. 300
Principal = Amount - SI
P = 1300 - 300 = Rs. 1000
Report a problem
Question for Practice Questions Level 3: Simple & Compound Interest - 1
Try yourself:A man borrows a certain sum at 20% compounded annually and lends it at 25% simple interest. What is the maximum number of years for which he can wait, so that he does not bear any loss?
Explanation
Let principal = P
Required time = t
Amount at 25% simple interest = P + (P × 25 × t)/100
Amount at 20% compound interest =
According to the question,
If he waits up to 4 years, he will bear a loss.
So, he can wait for up to 3 years.
Report a problem
Question for Practice Questions Level 3: Simple & Compound Interest - 1
Try yourself:Vivek purchased a bike costing Rs. 51,250 under finance with a down payment (which has to be paid at the beginning) of Rs. 9,575. Next month onwards, he has to pay Rs. 1,300 every month up to 36 months to clear the remaining payment. What is the rate of interest under simple interest?
Explanation
Cost of bike = Rs. 51,250
Down payment = Rs. 9,575
Monthly installment = Rs. 1300 for 36 Months
Amount paid for total installments = 36 × 1300 = Rs. 46,800
Total Amount paid = Rs. 46,800 + Rs. 9,575 = Rs. 56, 375
So, S. I. = Rs. 56,375 - Rs. 51,250 = Rs. 5125
Now,
R = 10%
Report a problem
Question for Practice Questions Level 3: Simple & Compound Interest - 1
Try yourself:Raghav has to repay a loan of Rs. 12,820 in three annual instalments. The first instalment is to be half of the second and one-third of the third. If the compound interest is calculated annually at a rate of 10%, then find the value of the third instalment that Raghav has to pay.
Explanation
Given, initial amount = Rs. 12,820
Amount to be paid after one year = Rs. 12,820 + Rs. 1282 = Rs.14,102.
If Raghav`s first year instalment is `Rs. a`, then the principal for second year = Rs. (14,102 - a).
Amount to be paid after two years = Rs. 11/10 x (14,102 - a) = Rs. (15,512.2 - 1.1a)
If Raghav's second year instalment is 'Rs. 2a', then principal for third year = Rs. ( 15,512.2 - 1.1a - 2a) = Rs. (15,512.2 - 3.1a)
∴ Amount to be paid after three years = Rs. 11/10 x (15,512.2 - 3.1a) = Rs. (17,063.42 - 3.41a)
Also, amount to be paid in third year = Rs. 3a
⇒ 17,063.42 - 3.41a = 3a
6.41a = 17063.42
a = 2662
Thus, third instalment = Rs. 3a = Rs. 7986
Report a problem
Question for Practice Questions Level 3: Simple & Compound Interest - 1
Try yourself:For a loan of Rs. 32,400 that I gave to my friend Anil, he promised to pay me the amount in monthly installments, starting with a certain amount and increasing the installments every month with a fixed amount of Rs. 100. In this way, he could clear off the loan in 24 months. How much was the first installment?
Explanation
Let the 1st installment be Rs. x.
According to the given condition, = Rs. 32,400
2x + 2300 = Rs. 2700
2x = Rs. 400
x = Rs. 200
Report a problem
Question for Practice Questions Level 3: Simple & Compound Interest - 1
Try yourself:A sum of Rs. 9400 is divided into three parts, such that the simple interests accrued on these sums for three, four and five years are equal. Find the amount deposited for five years.
Explanation
Let the amount deposited for 4 years be 15d.
Let the rate of deposited per annum be i.
Let the amount deposited for 3 years be a.
Let the amount deposited for 5 years be c.
So, a x 3 x i = 15 x d x 4 x i
Or, a = 20d ... (i)
Also,
c x 5i = 15d x 4 x i
Or, c = 12d ... (ii)
Now, a + 15d + c = Rs. 9400 ... (iii)
20d + 15d + 12d = 9400
d = 200
Plugging in values of a, and c from equations (i) and (ii) into equation (iii), and solving for d, we get d = Rs. 200
Thus, amount deposited for 5 years = 12d = 12 x Rs. 200 = Rs. 2400
Report a problem
Question for Practice Questions Level 3: Simple & Compound Interest - 1
Try yourself:If a person repaid Rs. 22,500 after 10 years of borrowing a loan, at 10% per annum simple interest find out what amount did he take as a loan?
Explanation
At 10% simple interest per year, the amount would double in 10 years. Thus, the original borrowin would be 22500/2 = 11250.
Report a problem
Question for Practice Questions Level 3: Simple & Compound Interest - 1
Try yourself:Mr. X , a very industrious person, wants to establish his own unit. For this he needs an instant loan of Rs. 5,00,000 and, every five years he requires an additional loan of Rs.100,000. If he had to clear all his outstandings in 20 years, and he repays the principal of the first loan equally over the 20 years, find what amount he would have to pay as interest on his initial borrowing if the rate of interest is 10% p.a. Simple Interest.
Explanation
The simple interest would be defined on the basis of the sum of the AP. 50000 + 47500 + 45000 + … + 2500 = 525000.
Report a problem
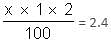
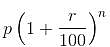
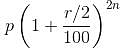
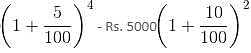
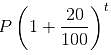
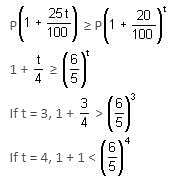
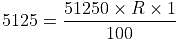
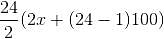= Rs. 32,400
