Solution of Triangles | Mathematics (Maths) for JEE Main & Advanced PDF Download
Solution of Triangles
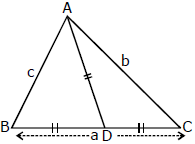
In a triangle ABC, the vertices and the angles are denoted by capital letters and the sides by small letters.
In the figure given below, the sides opposite to angles A, B, and C are denoted by a, b, c, respectively. The area of the triangle is denoted by S or Δ.
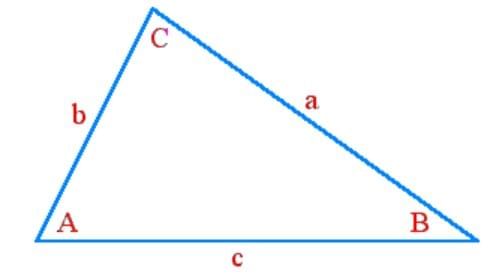
Basic Formulae and Results:
Some of the basic trigonometry formulae depicting the relationship between the sides and angles of a triangle are listed below:
A. Sine Formula
In any triangle, the sides are proportional to the sines of the opposite angles, i.e.
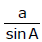 =
= 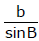 =
= 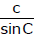
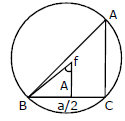
(1) Let the triangle ABC be acute-angled.
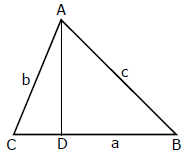
From A draw AD perpendicular to the opposite side; then
AD = AB sin ( ABD) = c sin B and AD = AC sin (
ABD) = c sin B and AD = AC sin ( ABCD) = b sin C
ABCD) = b sin C
 b sin C = c sin B i.e.
b sin C = c sin B i.e. 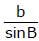 =
= 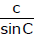
(2) Let the triangle ABC have an obtuse angle at B
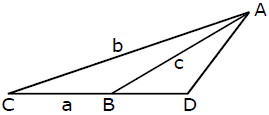
Draw AD perpendicular to CB produced; then
AD = AC sin  ACD = b sin C and AD = AB sin
ACD = b sin C and AD = AB sin  ABD
ABD
= c sin (180º - B) = c sin B;
 b sin C = c sin B i.e.
b sin C = c sin B i.e. 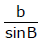 =
= 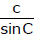
In a similar manner it may be proved that either of these ratios is equal to 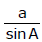
Thus 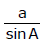 =
= 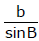 =
= 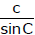 .
.
Ex.1 If the angles of a DABC are 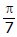 ,
, 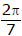 and
and 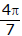 and R is the radius of the circumcircle then a2 + b2 + c2 has the value equal to
and R is the radius of the circumcircle then a2 + b2 + c2 has the value equal to
Sol. a2 + b2 + c2 = 4R2 (sin2A + sin2B + sin2C) = 2R2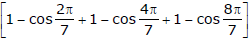
= 2R2 [3 - (cosθ + cos2θ + cos4θ)] where θ = 2π/7
now let S = cosθ + cos 2θ + cos 3θ (cos4θ = cos 3θ)
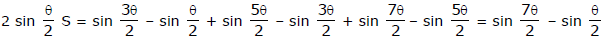
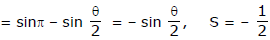 ⇒
⇒ 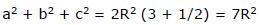
Ex.2 In a triangle ABC,  A is twice that of show
A is twice that of show 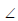 B. Whose that a2 = b(b + c).
B. Whose that a2 = b(b + c).
Sol. First assume that in the triangle ABC, A = 2B. Produce CA to D such that AD = AB, join BD.
By construction, it is clear that ABD is an isosceles triangle and so  ADB =
ADB =  ABD.
ABD.
But 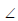 ADB +
ADB + 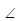 ABD +
ABD + 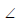 BAC (the external angle)
BAC (the external angle)
Hence 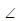 ADB =
ADB =  ABD =
ABD =  = B.
= B.
In triangles ABC and BDC we have  ABC =
ABC =  BDC and
BDC and  C is common. So
C is common. So 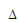 ABC is similar to
ABC is similar to 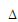 BDC. Therefore
BDC. Therefore 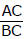 =
= 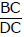
If follows that a2 = b(b + c)
Now we will prove the converse. Assume that a2 = b(b + c). We refer to the same figure. As before, in the isosceles triangle ABD, we have 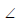 ABD =
ABD = 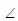 ADB. So each of these angles is equal to half of their sum which is A. Thus, in particular,
ADB. So each of these angles is equal to half of their sum which is A. Thus, in particular, 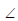 ADB =
ADB = 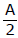 ....(1)
....(1)
On the other hand, in triangles ACB and BCD, we have, as a consequence of the assumption a2 = b(b + c), 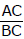 =
= 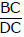 , and
, and 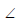 C is common. So the two triangles are similar and
C is common. So the two triangles are similar and 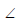 CDB =
CDB = 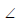 CBA = B. ....(2)
CBA = B. ....(2)
From (1) and (2), it follows that B = A/2, as desired.
Aliter : We may use the Sine rule for a triangle to dispose of both the implications simultaneously.
A = 2B ⇔ A - B = B ⇔ sin (A - B) = sin B ⇔ sin (A - B) sin (A + B) = sin B sin C
⇔ sin2A - sin2B = sin B sin C ⇔ (2R sinA)2 - (2R sin B)2 = (2R sin B) (2R sinC)
⇔ a2 - b2 = bc ⇔ a2 = b(b + c)
Ex.3 In a triangle ABC, a cos A + b cos B + c cos C = s. Prove that the triangle is equilateral.
Sol. The given result can be written as 2a cos A + 2b cos B + 2c cos C = a + b + c
Using sine rule we get 2 sin A cos A + 2 sin B cos B + 2 sin C cos C = sin A + sin B + sin C
⇒ sin 2A + sin 2B + sin 2C = sin A + sin B + sin C ⇒ 4 sinA sin B sin C = 4 cos 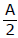 cos
cos 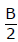 cos
cos 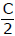
⇒ 8 sin 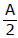 sin
sin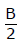 sin
sin 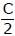 = 1 ⇒ 4
= 1 ⇒ 4 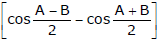 sin
sin 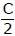 = 1
= 1
⇒ 4 sin2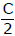 - 4 cos
- 4 cos 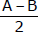 sin
sin 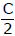 +1 = 0. This is a quadratic equation in sin
+1 = 0. This is a quadratic equation in sin 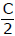 which must have real roots.
which must have real roots.
Hence 16 cos2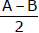
 1 ⇒ cos2
1 ⇒ cos2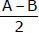
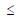 1. But cos2
1. But cos2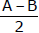
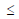 1 ⇒ cos2
1 ⇒ cos2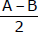 =1 ⇒ A = B
=1 ⇒ A = B
Similarly it can be prove that B = C ⇒ A = B = C
B. Cosine Formula
To find an expression for one side (c) of a triangle in terms of other to sides and the included angle (C).
(1) Let C be an acute angle.
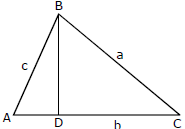
Draw BD perpendicular to AC ;
AB2 = BC2 + CA2 - 2AC . CD;
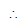 c2 = a2 + b2 - 2ba cos C
c2 = a2 + b2 - 2ba cos C
= a2 + b2 - 2ab cos C.
(2) Let C be an obtuse angle.
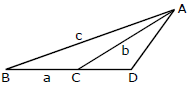
Draw BD perpendicular to AC produced ;
AB2 = BC2 + CA2 + 2AC . CD ;
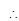 c2 = a2 + b2 + 2ba cos BCD
c2 = a2 + b2 + 2ba cos BCD
= a2 + b2 + 2ab cos (180º - C)
= a2 + b2 - 2ab cos C
Hence in each case, c2 = a2 + b2 - 2ab cos C
Similarly it may be shown that
a2 = b2 + c2 - 2bc cos A and b2 = c2 + a2 - 2ac cos B
From the above formulae we obtain
cos A = 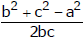 ; cos B =
; cos B = 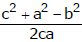 ; cos C =
; cos C = 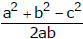
These results enable us to find the cosines of the angles when the numerical values of the sides are given.
Ex.4 If the sides a, b, c of a 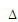 ABC satisfy the relation, a4 + b4 + c4 = 2c2 (a2 + b2), find the possible values of the angle C.
ABC satisfy the relation, a4 + b4 + c4 = 2c2 (a2 + b2), find the possible values of the angle C.
Sol. Solving as a quadratic equation in c we get, c2 = a2 + b2 ± 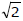 ab
ab
or a2 + b2 - c2 = ± 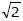 ab =
ab = 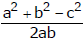 = ±
= ± 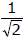 ⇒ C =
⇒ C = 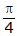 or
or 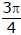
Ex.5 Let a, b, c be the sides of a triangle and D is its area. Prove that a2 + b2 + c2 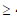 4
4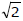
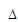 . When does the equality hold ?
. When does the equality hold ?
Sol. TPT : a2 + b2 + (a2 + b2 - 2ab cos C) 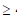 4
4 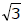 sin C or
sin C or 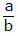 +
+ 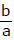
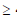
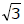 sin C + cos C
sin C + cos C
⇒ 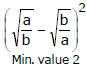 +
+ 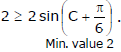 Equality occurs when a = b and C = π/3
Equality occurs when a = b and C = π/3
Ex.6 In a 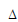 ABC, prove that a (b cos C - c cos B) = b2 - c2
ABC, prove that a (b cos C - c cos B) = b2 - c2
Sol.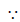 We have to prove a (b cos C - c cos B) = b2 - c2
We have to prove a (b cos C - c cos B) = b2 - c2
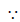 from cosine rule we know that cos C =
from cosine rule we know that cos C = 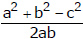 & cos B =
& cos B = 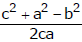
 L.H.S. = a
L.H.S. = a 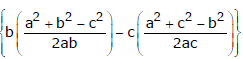 =
= 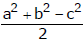 -
- 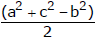 = (b2 - c2) = R.H.S.
= (b2 - c2) = R.H.S.
Ex.7 If in 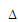 ABC,
ABC, 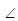 A = 60º then find the value of
A = 60º then find the value of 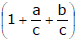
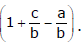 .
.
Sol.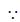
 A = 60º
A = 60º
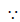
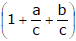
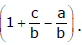 =
= 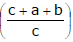
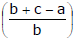
= 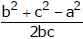 =
= 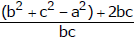 =
= 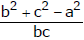 + 2 = 2
+ 2 = 2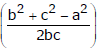 + 2
+ 2
= 2 cos A + 2 = 3
(
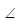 A = 60º ⇒ cos A =
A = 60º ⇒ cos A = 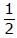 )
)
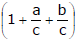
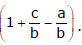 = 3
= 3
C. Projection Formula
To express one side of a triangle in terms of the adjacent angles and the other two sides.
(1) Let ABC be an acute-angled triangle
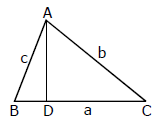
Draw AD perpendicular to BC;
then BC = BD + CD = AB cos  ABD + AC cos
ABD + AC cos 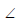 ACD;
ACD;
i.e. a = c cos B + cos C
(2) Let the triangle ABC have an obtuse angle C.
Draw AD perpendicular to BC produced; then
BC = BD - CD = AB cos  ABD - AC cos
ABD - AC cos 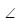 ACD ;
ACD ;
 a = cos B - b cos (180º - C) = c cos B + b cos C
a = cos B - b cos (180º - C) = c cos B + b cos C
Thus in each each case a = b cos C + c cos B.
Similarly it may be shown that
b = c cos A + a cos C, and c = a cos B + b cos A
Ex.8 In a 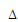 ABC prove that (b + c) cos A + (c + a) cos B + (a + b) cos C = a + b + c
ABC prove that (b + c) cos A + (c + a) cos B + (a + b) cos C = a + b + c
Sol.  L.H.S. = (b + c) cos A + (c + a) cos B + (a + B) cosC
L.H.S. = (b + c) cos A + (c + a) cos B + (a + B) cosC
= b cos A + c cos A + c cos B + a cos B + a cos C + b cos C
= (b cos A + a cos B) + (c cos A + a cos C) + (c cos B + b cos C) = a + b + c = R.H.S.
Ex.9 In a 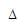 ABC perpendiculars are drawn from angles A, B, C of an acute angled triangle on the opposite sides and produced to meet the circumscribing circle. If these produced points be α, β, γ respectively, show that
ABC perpendiculars are drawn from angles A, B, C of an acute angled triangle on the opposite sides and produced to meet the circumscribing circle. If these produced points be α, β, γ respectively, show that 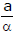 +
+ 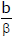 +
+ 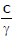 = 2 π tan A, where π denotes the continued product.
= 2 π tan A, where π denotes the continued product.
Sol. Using the property of cyclic quadrilateral,
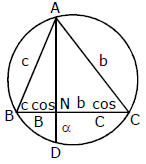
c sin B . α = bc cos B . cos C
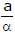 =
= 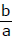
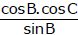 =
= 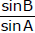 .
. 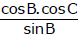 =
= 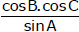
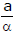 =
= 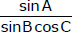 =
= 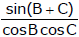 =
= 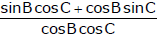
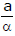 = tan B + tanC ....(1)
= tan B + tanC ....(1)
similarly 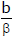 = tan C + tan A ....(2)
= tan C + tan A ....(2)
and 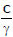 = tan A + tan B ....(3)
= tan A + tan B ....(3)
adding 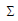
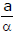 = 2
= 2 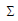 tan A = 2 π tan A
tan A = 2 π tan A
Ex.10 In any triangle ABC, (a + b)2 sin2 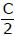 + (a - b)2 cos2
+ (a - b)2 cos2 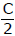 =
=
Sol. a2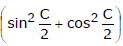 + b2
+ b2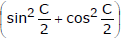 - 2ab
- 2ab 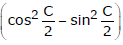 = a2 +b2 - 2ab cos C = c2
= a2 +b2 - 2ab cos C = c2
D. NapieR's Analogy - Tangent Rule
(i) tan 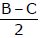 =
=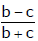 cot
cot 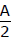
(ii) tan 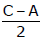 =
=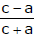 cot
cot 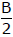
(iii) tan 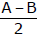 =
=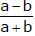 cot
cot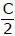
Ex.11 Find the unknown elements of the 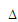 ABC in which a =
ABC in which a = 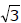 + 1, b =
+ 1, b = 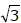 - 1, C = 60º
- 1, C = 60º
Sol. a =
a = 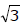 + 1, b =
+ 1, b = 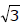 - 1, C = 60º
- 1, C = 60º  A + B + C = 180º
A + B + C = 180º  A + B = 120º ....(i)
A + B = 120º ....(i)
 From law of tangent, we know that tan
From law of tangent, we know that tan 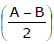 =
= 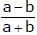 cot
cot 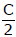 =
= 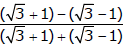 cot 30º
cot 30º
= 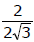 cot 30º ⇒ tan
cot 30º ⇒ tan 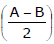 = 1
= 1 
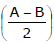 =
= 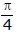 = 45º ⇒ A - B = 90º ....(ii)
= 45º ⇒ A - B = 90º ....(ii)
From equation (i) and (ii); we get A = 105º and B = 15º. Now,
 From sine-rule, we know that
From sine-rule, we know that  =
= 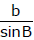 =
= 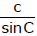
 c =
c = 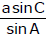 =
= 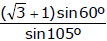 =
= 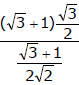
 sin 105º =
sin 105º = 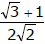
⇒ c = 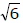
 c =
c = 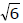 , A = 105º, B = 15º
, A = 105º, B = 15º
E. Area of a triangle
To find the area of a triangle. Let 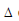 denote the area of the triangle ABC.Draw AD perpendicular to BC.
denote the area of the triangle ABC.Draw AD perpendicular to BC.
The area of a triangle is half the area of a rectangle on the same base and of the same altitude
 =
= 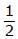 (base × altitude)
(base × altitude)
= 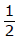 BC . AD =
BC . AD = 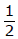 BC .AB sin B =
BC .AB sin B = 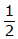 ca sin B
ca sin B
 R =
R = 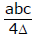 ⇒ abc = 4R
⇒ abc = 4R
 LHS =
LHS = 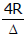
Ex.12 Find the area of a triangle with angles α, β and γ knowing that the distances from an arbitrary point M taken inside the triangle to its sides are equal to m,nand k. (fig.)
Sol.

The area S of the triangle ABC can be found by the formula S = 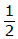 AC. BC. sin γ, for which purpose we have to find AC and BC. Let BC = x. Then, by the law of sines, we have
AC. BC. sin γ, for which purpose we have to find AC and BC. Let BC = x. Then, by the law of sines, we have 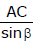 =
= 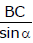 =
=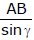 ,
,
whence we find that AC = 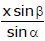 and AB =
and AB = 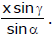 .
.
Thus, the problem is reduced to finding x. To set up an equation, we are going to apply the method of areas,
taking the area S of the triangle ABC as a reference element.
We have :
S = 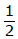 AC . BC . sin γ =
AC . BC . sin γ = 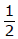
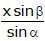 . x sin γ =
. x sin γ = 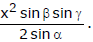 . On the other hand,
. On the other hand,
S = SAMB + SBMC + SAMC =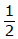 AB . k +
AB . k +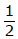 BC . n +
BC . n +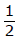 AC . m =
AC . m =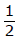 .
.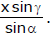 k +
k +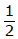 xn +
xn + 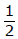 .
.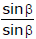 .m
.m
= 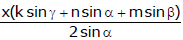
Hence, 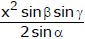 =
=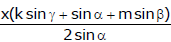 , whence we get : x =
, whence we get : x =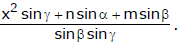 .
.
Substituting this value of x into the first of the above formulas for the area of the triangle ABC, we obtain : S = 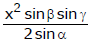 =
= 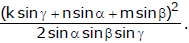
F. Trigonometric Functions of Half Angles
(i) sin 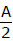 =
= 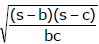 ; sin
; sin 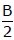 =
= 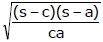 ; sin
; sin 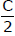 =
= 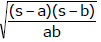
(ii) cos 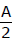 =
= 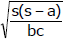 ; cos
; cos 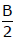 =
= 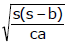 ; cos
; cos 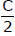 =
= 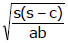
(iii) tan 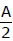 =
= 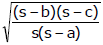 =
= 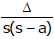 where s =
where s = 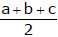 &
& 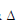 = area of triangle
= area of triangle
(iv) Area of triangle = 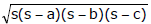
Ex.13 If a, b, c are in A.P., then the numerical value of tan 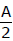 tan
tan 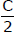 is
is
Sol.
Given 2b = a + c ⇒ 3b = 2s = a + b + c
tan 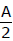 tan
tan 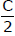 =
= 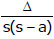 .
.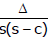 .
. 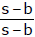 =
= 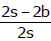 =
= 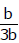 =
= 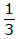
Ex.14 In a 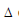 ABC, if cos A + cos B = 4 sin2
ABC, if cos A + cos B = 4 sin2 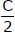 , prove that tan
, prove that tan 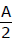 . tan
. tan 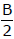 =
= 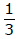 . Hence deduce that the sides of the triangle are in A.P.
. Hence deduce that the sides of the triangle are in A.P.
Sol. 2 cos 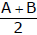 cos
cos  = 4 sin2
= 4 sin2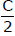 or cos
or cos 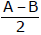 = 2 sin
= 2 sin 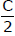
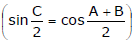
= 2 cos 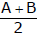 or cos
or cos 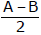 - cos
- cos 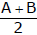 = cos
= cos 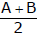
2 sin 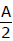 . sin
. sin 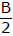 = cos
= cos  . cos
. cos 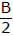 - sin
- sin 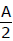 . sin
. sin 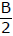
3 sin 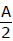 . sin
. sin 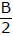 = cos
= cos 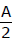 . cos
. cos 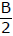 or tan
or tan 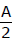 . tan
. tan 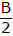 =
= 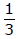
Now 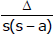 .
. 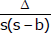 =
= 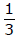 ⇒
⇒ 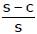 =
= 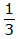
 2s = 3c ⇒ a + b + c = 3c ⇒ a + b = 2c ⇒ a, c, b are in A.P.
2s = 3c ⇒ a + b + c = 3c ⇒ a + b = 2c ⇒ a, c, b are in A.P.
Ex.15 With usual notions, prove that in a triangle ABC, cot 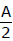 + cot
+ cot 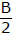 + cot
+ cot 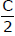 =
= 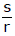 .
.
Sol. Using cot 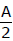 =
= 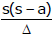 etc.
etc.
LHS = 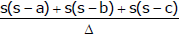 =
= 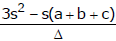 =
= 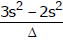 =
= 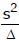 =
= 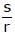
G. M-N Rule
In any triangle, (m + n) cot θ = m cot α - n cot β = n cot B - m cot C
H. Radius of the incircle
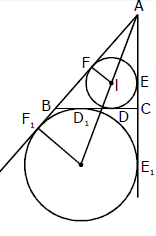
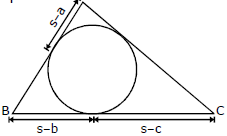
To find the radius of the circle inscribed in a triangle. Let l be the circle inscribed in the triangle ABC, and D, E, F the points of contact; then ID, IE, IF are perpendicular to the sides.
Now 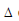 = sum of the areas of the triangles BIC, CIA, AIB
= sum of the areas of the triangles BIC, CIA, AIB
= 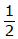 ar +
ar + 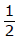 br +
br + 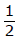 or =
or = 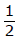 (a + b + c)r = sr ⇒ r =
(a + b + c)r = sr ⇒ r = 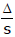
(a) r = 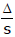 where s =
where s = 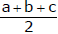
(b) r = (s - a) tan 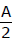 = (s - b) tan
= (s - b) tan 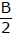 = (s - c) tan
= (s - c) tan 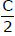
(c) r = 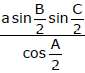 & so on
& so on
(d) r = 4R sin 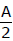 sin
sin 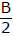 sin
sin 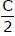
I. Radius of the Ex-circles
A circle which touches one side of a triangle and the other two sides produced is said to be an escribed circle of the triangle. Thus the triangle ABC has three escribed circles, one touching BC, and AB, AC produced, a second touching CA, and BC produced ; a third touching AB, and CA, CB produced.
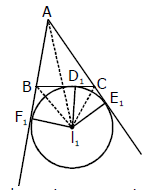
To find the radius of an escribed circle of a triangle. Let I1 be the centre of the circle touching the side BC and the two sides AB and AC produced. Let D1, E1, F1 be the points of contact; then the lines joining I1 to these points are perependicular to the sides.
Let r1 be the radius ; then
 = area ABC = area ABl1C - area Bl1C = area Bl1A + area Cl1A - area Bl1C
= area ABC = area ABl1C - area Bl1C = area Bl1A + area Cl1A - area Bl1C
=  cr1 +
cr1 +  br1 -
br1 - 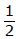 ar1 =
ar1 = 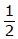 (c + b + a) r1 = (s - a) r1
(c + b + a) r1 = (s - a) r1  r1 =
r1 = 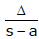
Similarly, if r2, r3 be the radii of the escribed circles opposite to the angles B and C respectively
r2 = 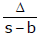 , r3 =
, r3 = 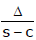 .
.
Many important relations connecting a triangle and its circles may be established by elementary geometry.
With the notation of previous articles, since tangents to a circle from the same point are equal.
we have, AF = AE, BD = BF, CD = CE;
 AF + (BD + CD) = half the sum of the sides ;
AF + (BD + CD) = half the sum of the sides ;
 AF + a = s
AF + a = s  AF = s - a = AE
AF = s - a = AE
Similarly, BD = BF = s - b, CD = CE = s - c. Also r = AF tan 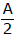 =(s - a) tan
=(s - a) tan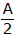
Similarly, r= (s - b) tan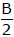 , r = (s - c) tan
, r = (s - c) tan 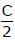 .
.
Again, AF1 = AE1, BF1 = BD1, CE1 = CD1
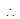 2AF1 = AF1 + AE1 = (AB + BD1) + (AC + CD1) = sum of the sides
2AF1 = AF1 + AE1 = (AB + BD1) + (AC + CD1) = sum of the sides
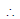 AF1 = s = AE1
AF1 = s = AE1 BD1 = BF1 = s - c, CD1 = CE1 = s - b
BD1 = BF1 = s - c, CD1 = CE1 = s - b
Also r1 = AF1 tan 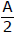 = s tan
= s tan 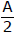 Similarly, r2 = s tan
Similarly, r2 = s tan 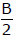 , r3 = s tan
, r3 = s tan 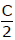 .
.
Note : r1 = 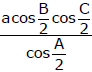 = 4R sin . cos
= 4R sin . cos 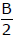 . cos
. cos 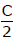 & so on
& so on
Ex.16 With usual notation in a triangle ABC, prove that r2 + s2 + 4Rr = ab + bc + ca.
Sol. r2 + s2 + 4Rr = 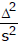 + s2 +
+ s2 + 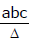 .
. 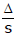
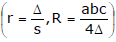
= 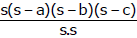 + s2 +
+ s2 + 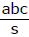 =
= 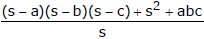
= 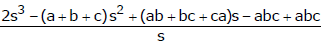 = ab + bc + ca (proved)
= ab + bc + ca (proved)
Ex.17 Find the area of a right triangle if it is known that the radius of the circle inscribed in the triangle is r and that of the circumscribed circle is R.
Sol.
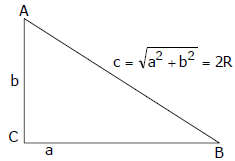
r = 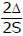 =
= 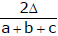 =
= 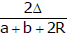
r (a + b) = 2D - 2Rr
r2 [a2 + b2 2ab] = 4(D - Rr)2
r2 [4R2 + 4D] = 4(D - Rr)2 [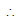 ab = 2D]
ab = 2D]
r2 [R2 + D2] = D2 + R2r2 - 2Dr
Dr2 = D2 - 2Dr ⇒ D = r2 + 2Rr = r(r + 2R)
Ex.18 If α, β, γ be the distances of the angular points of a triangle from the points of contact of the incircle with the sides of the triangle, then show that r = 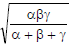
Sol.
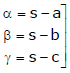 ⇒ a + b + g = s
⇒ a + b + g = s
Now r2 = 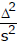 =
= 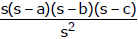 =
= 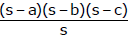 =
= 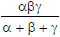 . Hence r =
. Hence r = 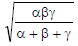
Ex.19 In an isosceles  ABC if the altitudes intersect on the inscribed circle then the cosine of the vertical angle 'A' is
ABC if the altitudes intersect on the inscribed circle then the cosine of the vertical angle 'A' is
Sol. A = x ⇒
A = x ⇒  BOI =
BOI = 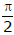 -
- 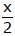 and
and 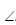 BIM =
BIM = 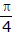 +
+ 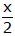 ; tan
; tan 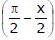 =
= 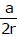 = cot
= cot 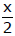
and tan 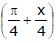 =
= 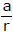 =
= 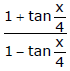
⇒ 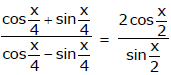
⇒ sin 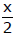 =
= 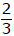
⇒ cos x = 1 - 2 sin2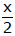 =
= 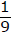
Ex.20 Consider an acute angled triangle ABC. Let AD, BE and CF be the altitudes drawn from the vertices to the opposite sides. Prove that, 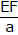 +
+ 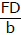 +
+ 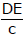 =
= 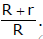
Sol.
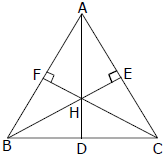
BD = AB cos B = c cos B also 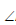 BHD =
BHD = 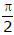 -
-  EBC =
EBC = 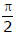 -
- 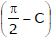 = C
= C
⇒ BH = 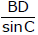 =
= 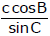 = 2R cos B
= 2R cos B
Now the points H, D, B and F are concyclic and BH is the diameter of the circle passing through these four points. In fact this circle is also the circumcircle of
triangle BFD ⇒ 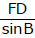 = BH = 2R cos B
= BH = 2R cos B
⇒ FD = 2R sin B cos B = b cos B ⇒ 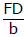 = cos B. Similarly
= cos B. Similarly 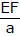 = cos A and
= cos A and 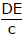 = cos C
= cos C
Thus 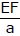 +
+ 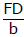 +
+ 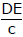 = cos A + cos B + cos C = 1 + 4 sin
= cos A + cos B + cos C = 1 + 4 sin 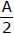 sin
sin 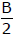 sin
sin 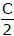 = 1 +
= 1 + 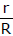 =
= 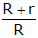 .
.
Ex.21 If the excircle touching the side c of the triangle ABC passes through its circumcentre, then prove that, sin A + sin B + sin C = 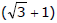 cot
cot 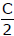 .
.
Sol. Distance between the circumcentre and the excentre l3 is d = 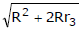 . As the excircle passes through its circumcentre ⇒ d = r3 ⇒ r32 = R2 + 2Rr3 ⇒ 2r32 = (R + r3)2
. As the excircle passes through its circumcentre ⇒ d = r3 ⇒ r32 = R2 + 2Rr3 ⇒ 2r32 = (R + r3)2
Ex.22 The radii r1, r2, r3 of described circles of a triangle ABC are in harmonic progression. If its area is 24 sq. cm and its perimeter is 24 cm, find the lengths of its sides.
Sol.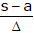 ,
, 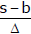 ,
, 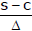 are in A.P. ⇒ a, b, c are in A.P.
are in A.P. ⇒ a, b, c are in A.P.
⇒ 2b = a + c ⇒ 2s = 24 ⇒ s = 12
⇒ 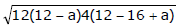 = 24 ⇒ 12 × 4(12 - a) (a - 4) = 24 × 24 ⇒ -a2 + 16a - 48 = 12
= 24 ⇒ 12 × 4(12 - a) (a - 4) = 24 × 24 ⇒ -a2 + 16a - 48 = 12
⇒ a2 - 16a + 60 = 0 ⇒ (a - 10) (a - 6) = 0 ⇒ a = 10, a = 6 6, 8, 10 cms
Ex.23 ABC is a triangle with l as its incentre. The radii of the incircles of the triangles BIC, AIB and AIC are r1, r2, and r3 respectively. Prove that Al + Bl + Cl = 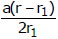 +
+ 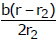 +
+ 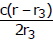 .
.
Sol. The area of the triangle BIC = 
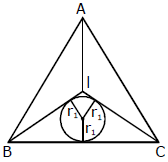
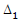 =
= 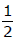 ar1 +
ar1 + 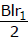 +
+ 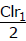 ⇒
⇒ 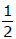 ar =
ar =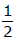 (a + Bl + Cl) r1 ⇒
(a + Bl + Cl) r1 ⇒ 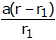 = Bl + Cl ....(1)
= Bl + Cl ....(1)
Similarly 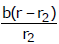 = Al + Bl and
= Al + Bl and 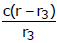 = Al + Cl ....(2)
= Al + Cl ....(2)
From (1) and (2), we get Al + Bl + Cl = 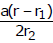 +
+ 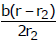 +
+ 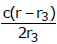 .
.
J. Length of angle bisectors, medians & altitude
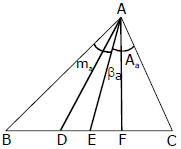
(i) Length of an angle bisector from the angle A = βa = 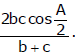 .
.
(ii) Length of median from the angle A = ma = 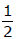
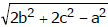
(iii) Length of altitude from the angle A = Aa = 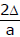 .
.
Note : ma2 + mb2 + mc2 = 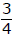 (a2 + b2 + c2)
(a2 + b2 + c2)
To find the radius of the circle circumscribing a triangle. Let S be the centre of the circle circumscribing the triangle ABC, and R its radius.
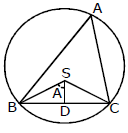
Bisect 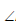 BSC by SD, which will also bisect BC at right angles.
BSC by SD, which will also bisect BC at right angles.  BSC at centre = twice
BSC at centre = twice  BAC = 2A and
BAC = 2A and 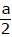 = BD = BS sin BSD = R sin A
= BD = BS sin BSD = R sin A  R =
R = 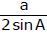
Thus 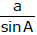 =
= 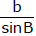 =
= 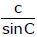 = 2R or a = 2R sinA, b = 2R sin B, c = 2R sin C
= 2R or a = 2R sinA, b = 2R sin B, c = 2R sin C
The circum-radius may be expressed in a form not involving the angles, as
R = 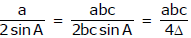
Ex.24 Show that 2R2 sin A sin B sin C 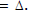
Sol. The first side = 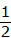 . 2R sin A. 2R sin B. sin C =
. 2R sin A. 2R sin B. sin C = 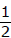 ab sin C
ab sin C 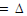
Ex.25 The medians of a triangle ABC are 9 cm, 12 cm and 15 cm respectively. Then the area of the triangle i
Sol. Produce the median AM to D such that GM = MD. Join D to B and C.
Now GBDC is a parallelogram. Note that the sides of the DGDC are 6, 8, 10
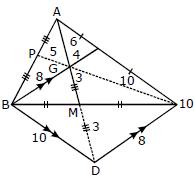
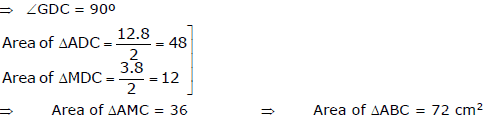
Ex.26 In 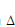 ABC, in the usual notation, the area is
ABC, in the usual notation, the area is 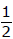 be sq. units AD is the median to BC.
be sq. units AD is the median to BC.
Prove that 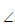 ABC =
ABC = 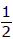
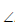 ADC.
ADC.
Sol. ⇒ sin A = 1 ⇒  A = 90º
A = 90º
Since AD is the median and  A = 90º, D, the midpoint of BC is the centre of the circumcircle of
A = 90º, D, the midpoint of BC is the centre of the circumcircle of  ABC.
ABC.
So AD = BD = DC ⇒ 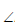 ABC =
ABC = 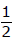
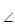 ADC
ADC
(angle subtended by AC at the circumference = 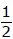 angle subtended by AC at the centre).
angle subtended by AC at the centre).
Ex.27 Prove that of all the triangles with a given base and a given vertex angle, an isosceles triangle has the greatest bisector of the vertex angle.
Sol. Let us give a geometrical proof which is considerably briefer and more elegant than the first method.
Circumscribe a circle above the triangle ABC with the angle bisector BD (fig.). The vertices of all the rest of triangles with a given base and a given vertex angle lie on the arc ABC. Let us take an isosceles triangle AB1C, draw the angle bisector B1D1 in it, and prove that BD < B1D1 in it, and prove that BD < B1D1.
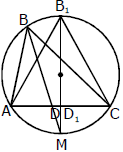
Extend both angle bisectors BD and B1D1 to intersect the circle. Both of them will intersect the circle. Both of them will intersect the circle at one and the same point M which is the midpoint of the arc AC. Since B1M is a diameter of the circle, we have : BM < B1M. From the triangle DD1M. From these inequalities it follows that BM - DM < B1M - D1M, that is BD < B1D1.
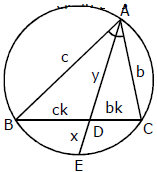
Ex.28 In a 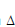 ABC, the bisector of the angle A meets the side BC in D and the circumscribed circle in E. Show that, DE =
ABC, the bisector of the angle A meets the side BC in D and the circumscribed circle in E. Show that, DE = 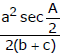 .
.
Sol.
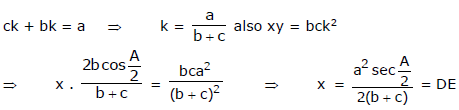
Ex.29 In a 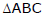 internal angle bisector Al, Bl and Cl are produced to meet opposite sides in A', B' and C' respectively. Prove that the maximum value of
internal angle bisector Al, Bl and Cl are produced to meet opposite sides in A', B' and C' respectively. Prove that the maximum value of 
Sol.
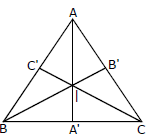
Since angle bisector divides opposite side in ratio of sides containing the angle
⇒ BA' = 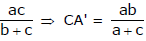
Now Bl is also angle bisector of 
K. Orthocentre and Pedal Triangle
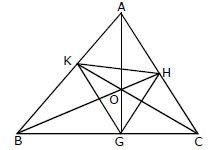
Let G, H, K be the feet of the perpendiculars from the angular points on the opposite sides of the triangle ABC, then GHK is called the Pedal triangle of ABC. The three perpendiculars AG, BH, CK meet in a point O which is called the orthocentre of the triangle ABC.
To find the sides and angles of the pedal triangle.
In the figure, the points K, O, G, B are concyclic 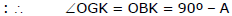
Also the points H, O, G, C are concyclic : 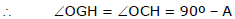
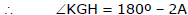
Thus the angles of the pedal triangle are 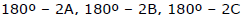
Again, the triangles AKH, ABC are similar : 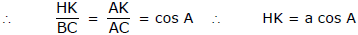
Thus the sides of the pedal triangle are a cos A, b cos B, c cos C.
In terms of R, the equivalent forms become R sin 2A, R sin 2B, R sin 2C.
If the angle ACB of the given triangle is obtuse, the expression 180º - 2C, and c cos C are both negative, and the values we have obtained required some modification. We have the student to show that in this case the angles are 2A, 2B, 2C - 180º, and the sides a cos A, b cos B, - c cos C.
Remarks :
(i) The distances of the orthocentre from the angular points of the DABC are 2 R cos A, 2R cos B and 2R cos C.
(ii) The distances of P from sides are 2R cos B cos C, 2R cos C cos A & 2R cos B cos C.
(iii) Circumradii of the triangles PBC, PCA, PAB and AABC are equal.
To find the area and circum-radius of the pedal triangle.
Area =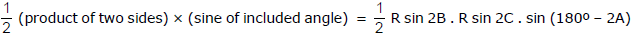
= 
Ex.30 The point H is the orthocentre of the triangle ABC. A point K is taken on the straight line CH such that ABK is a right triangle. Prove that the area of the triangle ABK is the geometric mean
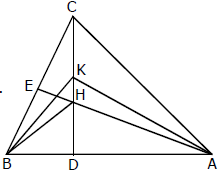
between the area of the triangles ABC and ABH.
Sol. We introduce the following notation : SABK = S, SABC = S1, SABH = S2.
Then S = 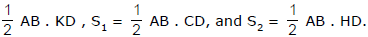
We have to prove that S = 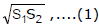
i.e. 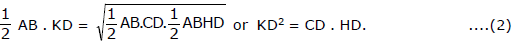
But ABK is a triangle, and therefore, KD2 = BD. AD. Thus, equality (2) will be ascertained if we prove that BD . AD = CD . DH, or that 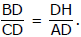 The last equality obviously follows from and HDA (in these triangles the angles BCD and HAD are equal as angles with mutually perpendicular sides since AE is the altitude of the triangle). Hence, Equality (2) as well as equality (1) have been proved.
The last equality obviously follows from and HDA (in these triangles the angles BCD and HAD are equal as angles with mutually perpendicular sides since AE is the altitude of the triangle). Hence, Equality (2) as well as equality (1) have been proved.
Ex.31 If f, g, h denote sides, the pedal triangle of a DABC, then show that 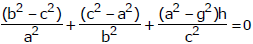
Sol. Sides are a cos A, b cos B, c cos C. Hence LHS 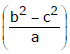 cos A +
cos A + 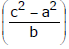 cos B +
cos B + 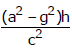 cos C
cos C
Put the values of cos A etc get the result.
Ex.32 Vertex A of a variable triangle ABC, inscribed in a circle of radius R, is a fixed point. If the angles subtended by the side BC at orthocentre (H), circumcentre (O) and incentre (I) are equal than identify the locus of orthocentre of triangle ABC.
Sol. The angles subtended by the side BC at points H, O and I are B + C, 2A and 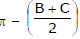 respectively.
respectively.
 Also in triangle ABC, HA = 2R cos A = R
Also in triangle ABC, HA = 2R cos A = R
⇒ HA is contant. ⇒ locus of orthocentre is a circle having centre at the vertex A.
L. Excentral Triangle
Let ABC be a triangle l1, l2, l3 its ex-centres ; then l1l2l3 is called the Ex-central triangle of ABC. Let l be the in-centre ; then from the construction for finding the positions of the in-centre and ex-centres, it follows that :
(i) The points l, l1 lie on the line bisecting the angle BAC; the points l, l2 lie on the line bisecting the angle ABC; the points l, l3 lie on the line bisecting the angle ACB.
(ii) The points l2, l3 lie on the line bisecting the angle BAC externally; the points l3,l1 lie on the line bisecting the angle ABC externally ; the points l1, l2 lie on the line bisecting the angle ABC externally.
(iii) The line Al1 is perpendicular to l2l3; the line Bl2 is perpendicular to l3l1 ; the line Cl3 is perpendicular to l1l2. Thus the triangle ABC is the Pedal triangle of its ex-central triangle l1l2l3.
(iv) The angles IBl1 and ICl1 are right angles; hence the points B, l, C, l1 are concyclic . Similarly, the points C, l, A, l2, and the points A, l, B, l3 are concyclic.
(v) The lines AI1, BI2, CI3 meet at the in-centre l, which is therefore the orthocentre of the ex-central triangle l1l2l3.
(vi) The lines Al1, Bl2, Cl3 meet at the in-centre l, which is therefore the orthocentre of the ex-central triangle l1l2l3.
(vii) Each of the four points l, l1,l2,l3 is the orthocentre of the triangle formed by joining the other three points.
To find the sides and angles of the ex-central triangle. With the figure of the-last article.
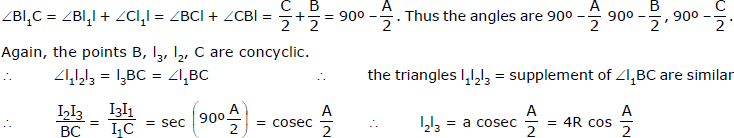
Thus the sides are 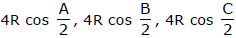
To find the area and circum-radius of the ex-central triangle.
The area = 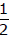 (product of two sides) × (sine of included angle)
(product of two sides) × (sine of included angle)
= 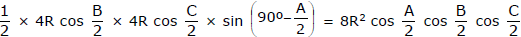
The circum-radius =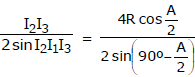 = 2R
= 2R
To find the distances between the in-centre and ex-centres.

We have proved that OG, OH, OK bisect the angles HGK, KHG, GHK respectively, so that O is the in-centre of the triangle GHK. Thus the orthocentre of a triangle is the in-centre of the pedal triangle.
Again, the line CGB which is at right angles to OG bisect ∠HGK externally. Similarly the lines AHC and BKA bisect  externally, so that ABC is the ex-central triangle of its pedal triangle GHK.
externally, so that ABC is the ex-central triangle of its pedal triangle GHK.
Ex.33 If l is the incentre and l1, l2, l3 are the centre of escribed circles of the DABC, Prove that
(i) II1, II2, II3 = 16R2r
(ii) II12 + I2I32 = II22 + I3I12 = I1I22 + II32
Sol. (i)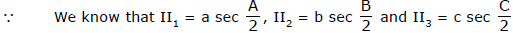
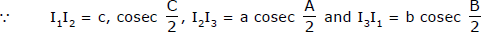
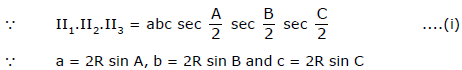
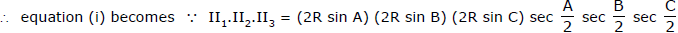

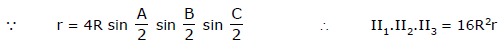
(ii)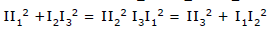
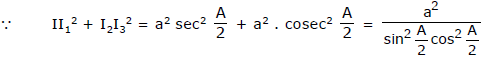
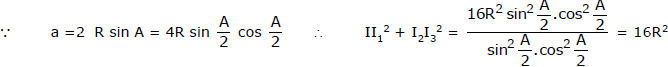
Hence II12 +I2I32 = II22 + I3I12 = II32 + I1I22
M. Distances of Special Points from vertices and sides of a triangle
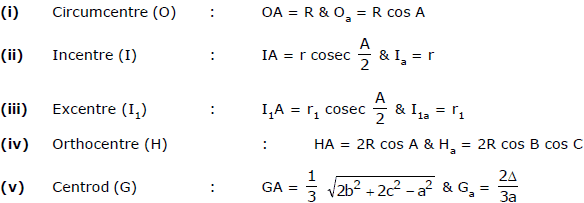
N. Distances between special points
(a) The distance between circumcentre and orthocentre is = 
(b) The distance between circumcentre and incentre is = 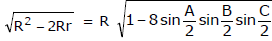
(c) Distance between circumcentre and centroid OG = 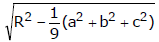
(d) The distance between incentre and orthocentre is 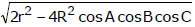
To find the distance between the in-centre and circum-centre.
Let S be the circum-centre and I the in-centre.Produce Al to meet the circum-circle in H; join CH and Cl.
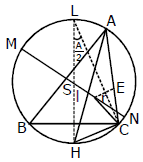
Draw IE perpendicular to AC. Produce HS to meet the circumference in L, and join CL. Then
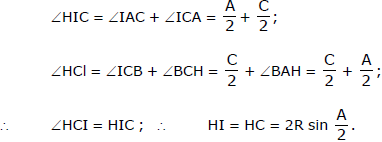
Also 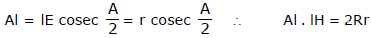
Produce Sl to meet the circumference in M and N. Since MIN, AIH are chords of the circle.
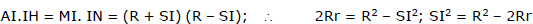
To find the distance of the orthocentre from the circum-centre.
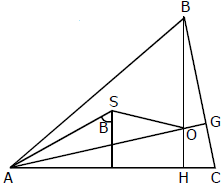
With the usual notation, we have SO2 = SA2 + AO2 - 2SA . AO cos SAO.
Now AS = R; AO = AH cosec C = c cos A cosec C
= 2R sin C cos A cosec C = 2R cos A;
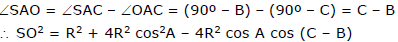
= 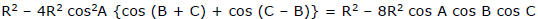
Ex.34 If r and R are radii of the incircle and circumcircle of  prove that
prove that
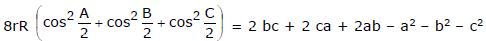
Sol.
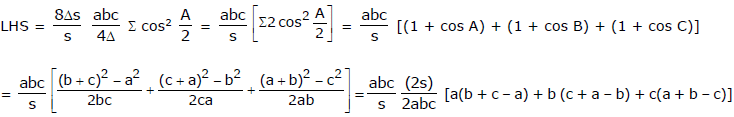
Ex.35 If the angle A of triangle ABC is 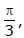 then prove that the vertices B, C orthocentre, circumcentre and incentre are concylic.
then prove that the vertices B, C orthocentre, circumcentre and incentre are concylic.
Sol. The angle subtended by the side BC at the orthocentre, the circumcentre and the incentre are B + C, 2A and 90 + 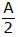 respectively. If
respectively. If 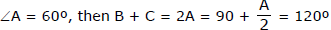
⇒ Angle subtended by BC at orthocentre, circumcentre and incentre are equal
⇒ B, C, orthocentre, circumcentre and incentre are angle C.
Ex.36 A quadrilateral ABCD is such that one circle can be inscribed in it and another circle circumscribed about it ; show that 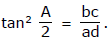
Sol. If a circle can be inscribed in a quadrilateral, the sum of one pair of the opposite sides is equal to that of the other pair : a + c = b + d. Since the quadrilateral is cyclic, cos A = 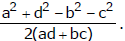 .
.
But a - d = b - c, so that a2 -2ad + d2 = b2 - 2bc + c2 ;
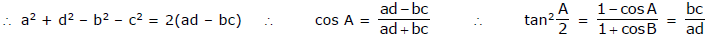
P. Inscribed and circumscribed polygons
To find the perimeter and area of a regular polygon of n sides inscribed a circle.
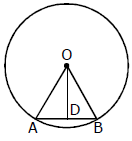
Let r be the radius of the circle, and AB a side of the polygon.
Join OA, OB, and draw OD bisecting ∠AOB; then AB is bisected at right angles in D.
And 
Perimeter of polygon = 
Area of polygon = 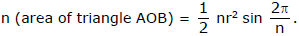
To find the perimeter and area of a regular polygon of n sides circumscribed about a given circle.
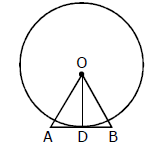
Let r be the radius of the circle, and AB a side of the polygon. Let AB touch the circle at D. Join OA, OB, OD; then OD bisects AB at right angles, and also bisects 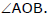
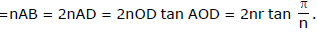
Area of polygon = n (area of triangle AOB)
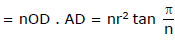
Q. Miscellaneous Questions
Ex.37 If a, b, c denote the sides of a DABC, show that the value of the expression,
a3 (p - q) (p - r) + b2 (q - r) + b2 (q - r) (q - p) + c2 (r - p) (r - q) cannot be negative where p, q, 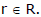
Sol. Let p > q > r and 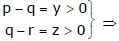 p - r = y + z > 0
p - r = y + z > 0
Consider 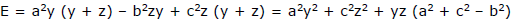
Now 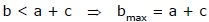
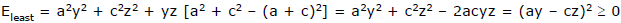
Ex.38 In a triangle ABC is 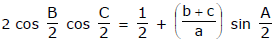 then find the measure of angle A.
then find the measure of angle A.
Sol. Given
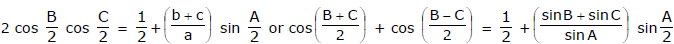
or 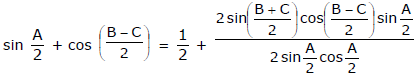
or 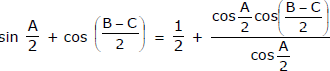
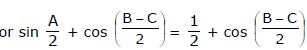

Ex.39 Suppose ABCD is a rectangle and P,Q,R,S are points on the sides AB, BC, CD, DA respectively. Show that PQ + QR + RS + SP  AC.
AC.
Sol.
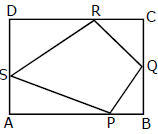
We have (see figure) PQ. QR > BQ . QC, QR . RS > CR . RD, etc.
Therefore, (PQ + QR + RS + SP)2 = PQ2 + ... + 2PQ . QR + ....
> (PB2 + BQ2) + 2BQ . QC +.....
= (PA + PB)2 + (BQ + QC)2 + (CR + RD)2 + (DS + SA)2
= AB2 + BC2 + CD2 + DA2 = AC2 + BD2 = 2AC2
Hence PQ + QR + RS + SP > AC.
Ex.40 In a triangle ABC the angle A is twice the angle C, the side BC is 2 cm longer than the side AB, and AC = 5 cm. Find AB and BC.
Sol. Drawing the bisector AD of the angle A, we get : 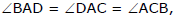
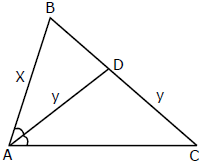
In a triangles ADC, the base angles are equal to each other, and hence, this is an isosceles triangles :
AD = DC. Setting AB = x and AD = DC = y, we find the BC = x + 2 and BD = x + 2 - y.
The triangles ABD and ABC are similar, since 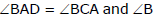 is a common angle. From the similarity of these triangles we conclude that
is a common angle. From the similarity of these triangles we conclude that 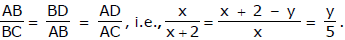
For finding x & y we have system of two equation in two variables : 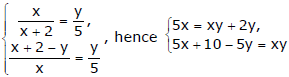
Subtracting the second equation from the first, we get : 5y - 10 = 2y
and 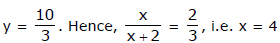
Ex.41 In triangle ABC, cos A . cos B + cos B. cos C + cos C, cos A = 1 - 2cos A . cos B . cos C. Prove that it is possible if and only if DABC is equilateral.
Sol. ∑cosA . cos B = 1 – 2 cos A. cos B. cos C = 1 – cos C (cos (A + B) + cos (A – B))
= 1 + cos2 A – sin2B + cos2 C = cos2 A + cos2 B + cos2 C = ∑ cos2 A
= 1 +cos2 A – sin2B + cos2 C = cos2 + cos2 B + cos2 B + cos2 C = ∑ cos2 A
Thus we have, 2∑cos2A – 2∑ cos A . cos B = 0
(cos A – cos B)2 + (cos B – cos C)2 + (cos C – cos A)2 = 0
cos A = cos B = cos C ⇒ ∠A = ∠B = ∠C. Thus triangle ABCis equilateral
Now if Δ is equilateral ∠A = ∠B = ∠C = p/3 ⇒ ∑cos A cos B = 3/4 and 1 – 2 cos A cos B cos C = 1– 2/8 = 3/4 .
Hence the given expression is true if and only if DABC is equilateral.
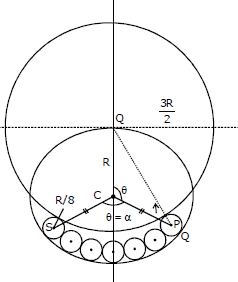
Ex.42 A point 'O' is situated on a circle of radius R and with centre O, another circle of radius 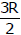 is described inside the crescent shaped area intercepted between these circles, a circle of radius R/8 is placed. If the same circle moves in centroid with the original circle of radius R, the length of the arc described by its centre in moving from one extreme position to the other is
is described inside the crescent shaped area intercepted between these circles, a circle of radius R/8 is placed. If the same circle moves in centroid with the original circle of radius R, the length of the arc described by its centre in moving from one extreme position to the other is
Sol.

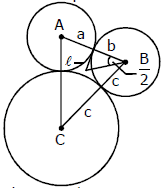
Ex.43 Three circles whose radii are a, b and c and c touch one other externally and the tangents at their points of contact meet in a point. Prove that the distance of this point from either of their points of contact is 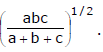
Sol.
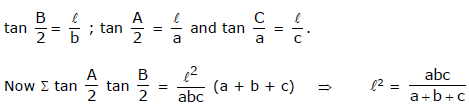
Ex.44 Inscribed in a circle is an isosceles triangle ABC whose base AC = b and the base angle is a. A second circle touches the first circle and the base of the triangle at its midpoint D, and is situated outside the triangle. Find the radius of the second circle.
Sol.
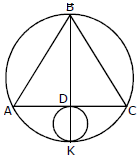
Let us take advantage of the fact that AD.DC = BD.DK
(Theorem 16a). Since 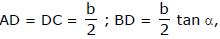
and 
R. Ambiguous case of the solution of a triangle
To solve a triangle having given two sides and an angle opposite to one of them.
(a) If a < b sin A, then b sinA/a > 1, so that sin B > 1, which is impossible. Thus, there is no solution.
(b) If a = b sin A, then b sin A/a = 1, so that sin B = 1 and B has only the value 90º.
(c) If a > b sin A, then b sin A/a < 1, and two values for B may be found from sin B = b sin A/a. These values are supplementary, so that one angle is acute, the other obtuse.
(1) If a < b, then A < B, and therefore B may either be acute or obtuse, so that both values are admissible. This is known as the ambiguous case.
(2) If a = b, then A = B; and if a > b, then A > B; in either case, B cannot be obtuse, and therefore only the smaller value of B is admissible. When B is found, C is determined from C = 180º – A – B.
Finally, c may be found from the equation c = aSinC/sinA
From the foregoing investigation, it appears that the only case in which an ambiguous solution can arise is when the smaller of the two given sides is opposite to the given angle.
To discuss the Ambiguous case geometrically.
Let a, b be the given parts. Take a line AX unlimited towards X; mae 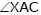 equal to A, and AC equal to b.
equal to A, and AC equal to b.
Draw CD perpendicular to AX, then CD = b sin A. With centre C and radius equal to describe a circle.
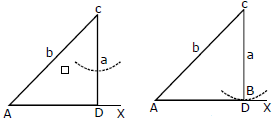
(a) If a < b sin A, the circle will not meet AX; thus, no triangle can be constructed with the given parts.
(b) If a = b sin A, the circle will touch AX at D; thu,s there is right-angled triangle with the given parts.
(c) If a > b sin A, the circle will cut AX in two points, B1, B2.
(1) These points will be both on the same side of A, when a < b, in which case there are two solutions
namely the triangles AB1C, AB2C This the Ambiguous case.
(2) The points B1, B2, will be on opposite sides of A when a >b. In this case there is only one solution, for the angle CAB2 is the supplement of the given angle, and thus the triangle AB2C does not satisfy the data.
(3) If a = b, the point B2 coincides with A, so that there is only one solution.
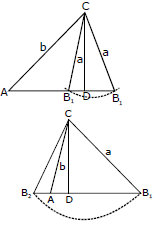
Ex.45 Given 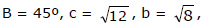 solve the triangle.
solve the triangle.
Sol. We have sin 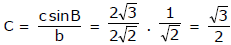
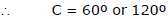 and
and
since b < c, both these values are admissible.
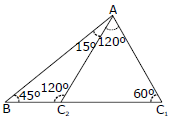
The two triangles which satisfy the data are shown in the figure.
Denote the sides BC1, BC2 by a1, a2 and the angles BAC1, BAC2 by A1, A2 respectively
(a)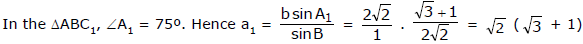
(b)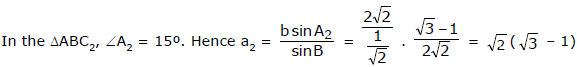
Thus, the complete solution is 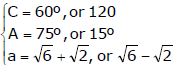
The ambiguous case may also be discussed by first finding the third side.
As before, let a, b, A be given, then cos A = 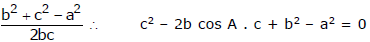
By solving this quadratic equation in c, we obtain
c = 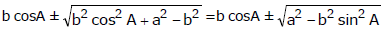
(a) When a < b sin A, the quantity under the radical is negative, and the values of c are impossible, so that there is no solution.
(b) When a = b sin A, the quantity under the radical is zero, and c= b cos A. Since sin A < 1, it follows that a < b, and therefore A < B. Hence the triangle is impossible unless the angle A is acute,m in which case c is positive and there is one solution.
(c) When a < b sin A, there are three cases to consider.
(1) Suppose a < b, then A < B, and as before the triangle is impossible unless A is acute.
In this case b cos A is positive. Also 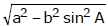 is real and
is real and 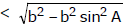
i.e. 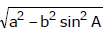 < b cos A
< b cos A
Hence both values of c are real and positive, so that there are two solutions.
(2) Suppose a > b, then 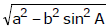 >
> 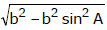 i.e.
i.e. 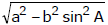 > b cos A
> b cos A
Hence one value of c is positive and one value is negative, whether A is acute or obtuse, and in each case there is only one solution.
(3) Suppose a = b, then 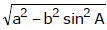 = b cos A i.e. c = 2b cos A or 0;
= b cos A i.e. c = 2b cos A or 0;
hence there is only one solution when A is acute, and when A is obtuse the triangle is impossible.
|
177 videos|620 docs|160 tests
|
FAQs on Solution of Triangles - Mathematics (Maths) for JEE Main & Advanced
| 1. What is the Law of Sines? |  |
| 2. How do you solve a triangle using the Law of Sines? |  |
| 3. What is the Law of Cosines? |  |
| 4. How do you use the Law of Cosines to solve a triangle? |  |
| 5. What is the difference between the Law of Sines and the Law of Cosines? |  |






















