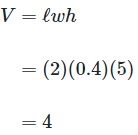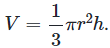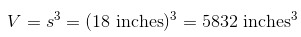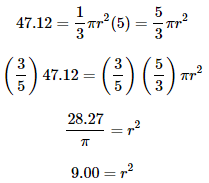Solved Examples: Area and Volume | Mathematics for Digital SAT PDF Download
What are area and volume problems?
Area and volume problems focus on using the relevant formulas for various two- and three-dimensional shapes. We'll be expected to calculate the length, area, surface area, and volume of shapes, as well as describe how changes in side length affect area and volume.Solved Examples
Q1: What is the volume, in cubic meters, of a right rectangular prism that has a length of 2 meters, a width of 0.4 meter, and a height of 5 meters?
Ans: 4 cubic meters
The formula for V , the volume of a rectangular prism with length l, width w, and height h is V = lwh.For l = 2 meters, w = 0.4 meter, and h = 5 meters, the volume V, in cubic meters, is:The volume of the right rectangular prism is 4 cubic meters.
Q2: The volume of a right circular cone A is 225 cubic inches. What is the volume, in cubic inches, of a right circular cone with twice the radius and twice the height of cone A?
(a) 450
(b) 900
(c) 1800
(d) 3600
Ans: (c)
The volume formula for a cone is
Since r is squared in the formula, the area of the cone's base is 22 = 4 times the area of the base of cone A.
The cone is also 2 times as tall.
This means the volume of the cone is 4 x 2 = 8 times the volume of cone A, or 8 x 225 = 1,800 cubic inches.
Q3: Find the volume of a cube in inches with a side of 1.5 \textup{ feet}.
(a) 54 inche3
(b) 40.5 inche3
(c) 5832 inche3
(d) 13458 inche3
(e) 0.001953 inche3
Ans: (c)
Convert the side dimension to inches first before finding the volume.
Write the volume for a cube and substitute the new side to obtain the volume in inches.
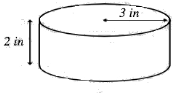
Q4: 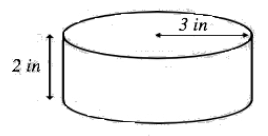
What is the volume of the cylinder above?
(a) 94.25 in3
(b) 66.13 in3
(c) 48.79 in3
(d) 56.55 in3
(e) 52.36 in3
Ans: (d)
In order to find the volume of a cylinder, you find the area of the circular top and multiply it by the height.
Volume = πr2h
π(32)(2) = π(9)(2) = 18π = 56.55
The volume of the cylinder is 56.55 in3
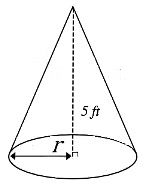
Q5:
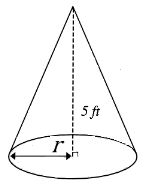
If the volume of the cone above is 47.12 ft3, what is the radius of the base?
(a) 3.5ft
(b) 4 ft
(c) 3 ft
(d) 2 ft
(e) 5 ft
Ans: (c)
Because we have been given the volume of the cone and have been asked to find the radius of the base of the cone, we must work backwards using the volume formula.
Volume = 1/3πr2h
r = 3The radius of the base of the cone is 3 ft.
Q6: The base of a cylinder has a circumference of 5π. The height of the cylinder is 4. What is the volume of the cylinder?
(a) 20π
(b) 25π
(c) 40π
(d) 50π
Ans: (b)
Circumference = 5π
2πr = 5π
Divide both sides by 2π.
r = 2.5
Volume of the cylinder :
= πr2h
Substitute r = 2.5 and h = 4.
= π(2.5)2(4)
= π(6.25)(4)
= 25π cubic units
The correct answer choice is (B).
Q7:
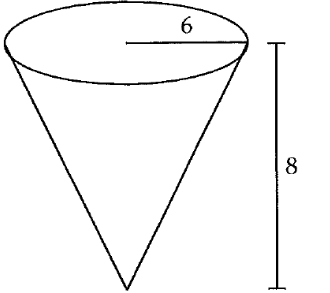
The figure above shows a paper cup in the shape of a right circular cone with a base radius of 6 and a height of 8. The cup is filled with water until its depth reaches half of the height of the cone. What is the volume of the water in the cup?
(a) 12π
(b) 16π
(c) 48π
(d) 96π
Ans: (a)
The height of the cone is 8.
When the cup is filled with water until its depth reaches half of the height of the cone, the depth of water in the cone :
= (1/2) x height of the cone
= (1/2) x 8
= 4
Let x be the radius of the water level, when the depth of the water is 4.
In the figure above, ΔABC and DEC are similar triangles, hence the sides are proportional.
DE/AB = DC/AC
x/6 = 4/8
x/6 = 1/2
x = 3
The radius of the water level is 3.
Since the water is in the cone shaped cup, formula for volume of a cone can be used to find the volume of the water in the cup.
Volume of the water :
= (1/3)πr2h
Substitute r = 3 and h = 4.
= (1/3)π(3)2(4)
= (1/3)π(9)(4)
= 12π cubic units
The correct answer choice is (A).
Q8:
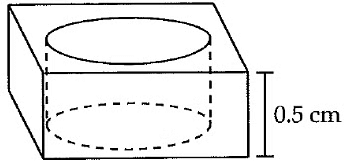
The figure above shows a metal ring with two square faces and a thickness of 0.5 cm. The square faces have a side length of 2.5 cm and the circular hole has a diameter of 2 cm. Which of the following is closest to the volume, in cubic centimeters, of the metal used to form the ring?
(a) 1.07
(b) 1.55
(c) 2.14
(d) 3.11
Ans: (b)
Volume of the box with two square faces :
= base area x height
= (2.5 x 2.5) x 0.5
= 6.25 x 0.5
= 3.125 cm3
To find the volume of the ring, we have to subtract the volume of the metal in the hole from the volume of the box.
The circular hole at the center of the ring is in the shape of the cylinder.
The hole has a diameter of 2 cm. Then the radius of the hole is 1 cm.
Volume of the metal in the hole can be found using the volume of a cylinder formula, as the hole is in the shape of the cylinder.
Volume of the metal in the hole :
= πr2h
= π(1)2(0.5)
= 0.5π
≈ 1.57 cm3
Volume of the metal ring :
= volume of the box - volume of the metal in the hole
= 3.125 - 1.57
= 1.555 cm3
Of the given answer choices, 1.55 is very close to 1.555.
The correct answer choice is (B).
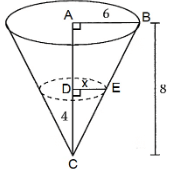
Q9: 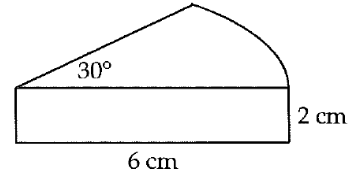
A wedge is formed by a central angle of 30° as shown above was cut from a circular block of cheese with a radius of 6 cm and a thickness of 2 cm. What is the volume, in cubic centimeters, of the wedge?
(a) 3π
(b) 6π
(c) 9π
(d) 12π
Ans: (b)
The base of the wedge above is a sector of a circle.
So, area of the base of the wedge can be found using area of a sector of a circle.
Area of the base of the wedge :
= (θ/360°) x πr2
Substitute θ = 30° and r = 6.
= (30°/360°) x π(6)2
= (1/12) x π(36)
= 3π cm2
Volume of wedge :
= base area x height
= 3π x 2
= 6π cm3
The correct answer choice is (B).
Q10: A rectangular box is 4 in wide, 6 in long, and 8 in deep. If the box is to be filled with cubes, each with a side length of 2 in, until no space is left inside, how many cubes are needed?
(a) 12
(b) 24
(c) 32
(d) 48
Ans: (b)
Volume of the box :
= base area x height
= (4 x 6) x 8
= 24 x 8
= 192 in3
Volume of a cube of side length 2 in :
= 2 x 2 x 2
= 8 in3
Number of cubes of side length 2 in are needed to fill the box :
= 192/8
= 24
The correct answer choice is (B).
|
204 videos|126 docs|75 tests
|
FAQs on Solved Examples: Area and Volume - Mathematics for Digital SAT
| 1. What are some common types of area and volume problems encountered in SAT exams? |  |
| 2. How can I approach solving area and volume problems on the SAT? |  |
| 3. What are some tips for tackling challenging area and volume problems on the SAT? |  |
| 4. Can I use a calculator to solve area and volume problems on the SAT? |  |
| 5. How important is it to memorize area and volume formulas for the SAT? |  |